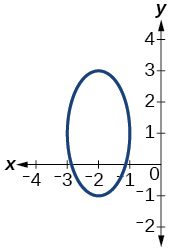2.5e: Exercises Inverse Functions
- Page ID
- 45445
A: Concepts
Exercise \(\PageIndex{A}\)
1) Describe why the horizontal line test is an effective way to determine whether a function is one-to-one?
2) Why do we restrict the domain of the function \(f(x)=x^2\) to find the function’s inverse?
3) Can a function be its own inverse? Explain.
4) Are one-to-one functions either always increasing or always decreasing? Why or why not?
5) How do you find the inverse of a function algebraically?
- Answers to Odd Exercises:
-
1. Each output of a function must have exactly one output for the function to be one-to-one. If any horizontal line crosses the graph of a function more than once, that means that \(y\)-values repeat and the function is not one-to-one. If no horizontal line crosses the graph of the function more than once, then no \(y\)-values repeat and the function is one-to-one.
3. Yes. For example, \(f(x)=\dfrac{1}{x}\) is its own inverse.
5. Given a function \(y=f(x)\), solve for \(x\) in terms of \(y\). Interchange the \(x\) and \(y\). Solve the new equation for \(y\). The expression for \(y\) is the inverse, \(y=f^{-1}(x)\).
B: Horizontal Line Test
Exercise \(\PageIndex{B}\))
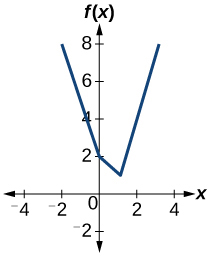
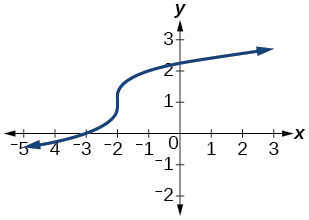
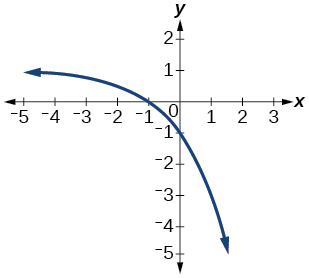
\( \bigstar \) For the following exercises, use the horizontal line test to determine whether each of the given graphs is one-to-one.
|
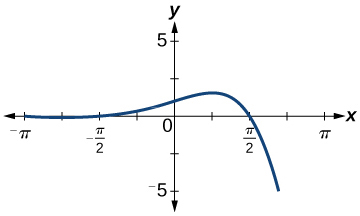
7.  |
8. 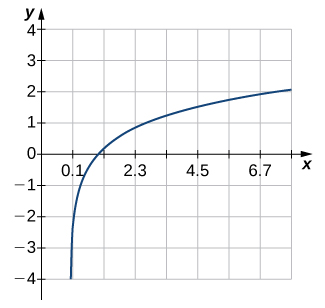 |
9. 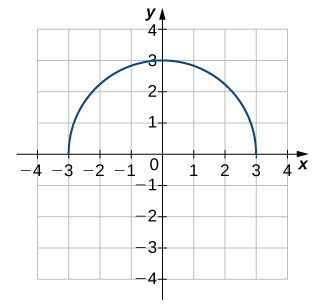 |
10. 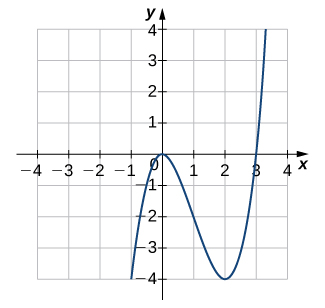 |
|
11. 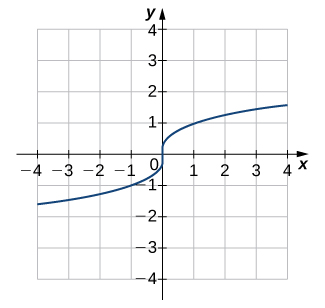 |
12.  |
13.
|
14.
|
|
15.
|
16.
|
17.
|
18.
|
- Answers to Odd Exercises:
-
7. Not one-to-one, \(\;\) 9. Not one-to-one, \(\;\) 11. One-to-one, \(\;\) 13. Not one-to-one, \(\;\) 15. One-to-one, \(\;\) 17. Not one-to-one
C: Graphs of Inverse Functions
Exercise \(\PageIndex{C}\)
\( \bigstar \) For the following exercises, use the graph of \(f\) to sketch the graph of its inverse function.
19)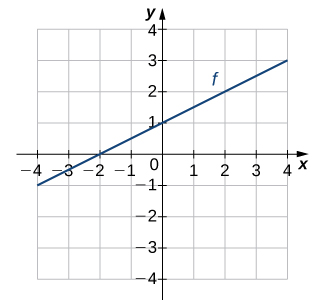 |
20)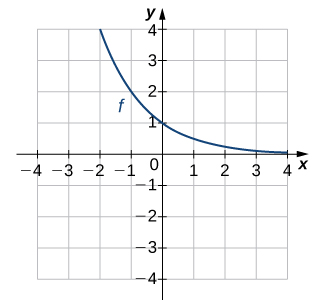 |
21)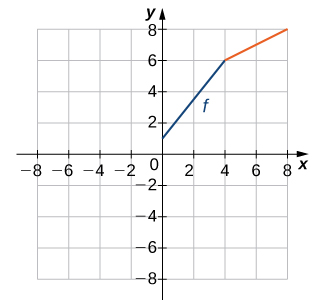 |
22) |
\( \bigstar \) Use the graph of the one-to-one function shown in the Figure to answer the following questions.
|
23) Find \(f(0) \\[4pt] \). 24) Solve \(f(x)=0 \\[4pt] \). 25) Find \(f^{-1}(0) \\[4pt] \). 26) Solve \(f^{-1}(x)=0\). |
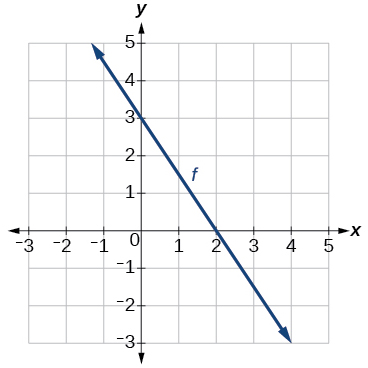 |
27) Sketch the graph of \(f^{-1} \\[4pt] \). 28) Find \(f(6)\) and \(f^{-1}(2) \\[4pt] \). 29) If the complete graph of \(f\) is shown, find the domain of \(f \\[4pt] \). 30) If the complete graph of \(f\) is shown, find the range of \(f\) |
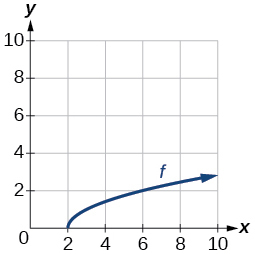 |
- Answers to Odd Exercises:
-
19)
21)
\(\;\)
23. \(3\)
25. \(2\)
29. \([2, \infty ) \)
27.
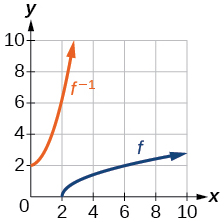
D: Inverse Function Values
Exercise \(\PageIndex{D}\)
\( \bigstar \) Find values of a function from its inverse and vice versa.
|
31) If \(f(6)=7\), find \(f^{-1}(7)\). 32) If \(f(3)=2\), find \(f^{-1}(2)\). |
32.1) If \(f(4)=5\), find \(f^{-1}(4)\). 32.2) If \(f^{-1}(9)=3\), find \(f(9)\). |
33) If \(f^{-1}(−4)=−8\), find \(f(−8)\). 34) If \(f^{-1}(−2)\)=−1, find \(f(−1)\). |
\( \bigstar \) Given the table of values for \(f\), determine the following.
|
\( \begin{array}{ll} |
|
- Answers to Odd Exercises:
-
31. \(6\) \(\qquad\) 33. \(-4\), \(\qquad\) 35. \(12\), \(\qquad\) 37. \(12\), \(\qquad\) 39. Undefined
E: Verify Two Functions are Inverses
Exercise \(\PageIndex{E}\)
\( \bigstar \) For the following exercises, use composition to determine which pairs of functions are inverses.
|
41) \(f(x)=8x, \; g(x)=\dfrac{x}{8}\) 42) \(f(x)=\dfrac{2}{3}x+2, \; g(x)=\dfrac{3}{2}x+3\) 43) \(f(x)=5x−7, \; g(x)=\dfrac{x+5}{7}\) 44) \(f(x)=8x+3, \; g(x)=\dfrac{x-3}{8}\) 45) \(f(x)=\dfrac{1}{x−1}, x≠1, \; g(x)=\dfrac{1}{x}+1,x≠0 \) |
46) \(f(x)=−3x+5\), \(g(x)=\dfrac{x-5}{-3}\) 47) \(f(x)=\dfrac{x}{2+x}\), \(g(x)=\dfrac{2x}{1-x} \\[2pt] \) 48) \(f(x)=x^3+1, \; g(x)=(x−1)^{1/3} \\[2pt] \) 49) \(f(x)=x^2+2x+1,x≥−1, \; g(x)=−1+\sqrt{x},x≥0 \\[2pt] \) 50) \(f(x)=\sqrt{4−x^2},0≤x≤2, \; g(x)=\sqrt{4−x^2},0≤x≤2 \\[2pt] \) |
- Answers to Odd Exercises:
-
41) inverses. 43) not inverses. 45) inverses. 47) inverses 49) inverses
F: Find inverses of linear and rational functions
Exercise \(\PageIndex{F}\)
\( \bigstar \) Find the formula for the inverse function \(f^{−1}(x)\).
|
51) \(f(x)=x+3 \\[2pt] \) 52) \(f(x)=x+5 \\[2pt] \) 53) \(f(x)=2−x \\[2pt] \) 54) \(f(x)=3−x \\[2pt] \) 55) \(f(x) = 7x−9 \\[2pt] \) |
56) \(f(x) = 6x−4 \\[2pt] \) 57) \(f(x) = −5x+2 \\[2pt] \) 58) \(f(x) = 6x+8\) 59) \(f(x)=\dfrac{3}{x+2} \\[3pt] \) 60) \(f(x)=\dfrac{x}{x-2} \\[3pt] \) |
61) \(f(x)= \dfrac{x-4}{x+2} \\[3pt] \) 62) \(f(x)= \dfrac{2x-7}{x+6} \\[3pt] \) 63) \(f(x)= \dfrac{2x+3}{5x+4} \\[3pt] \) 64) \(f(x)= \dfrac{2x+6}{x-3} \\[3pt] \) 65) \(f(x) = \dfrac{9x−3}{9x+7} \) |
66) \(f(x) = \dfrac{3x+7}{2x+8} \\[3pt] \) 67) \(f(x) = \dfrac{4x+2}{4x+3} \\[3pt] \) 68) \(f(x) = \dfrac{8x−7}{3x−6} \\[3pt] \) 69) \(f(x) = \dfrac{4x−1}{2x+2} \\[3pt] \) 70) \(f(x)=−\dfrac{9x−3}{7x+6}\) |
- Answers to Odd Exercises:
-
51. \(f^{-1}(x)=x−3 \) 53. \(f^{-1}(x)=2−x\)
55. \(f^{-1}(x)=\frac{x+9}{7}\) 57. \(f^{-1}(x)=−\frac{x−2}{5}\) 59. \(f^{-1}(x) = \frac{3}{x} - 2 \) 61. \(f^{-1}(x) = -2\frac{x+2}{x-1} \)
63. \(f^{-1}(x) = \frac{-4x+3}{5x-2} \) 65. \(f^{-1}(x)=−\frac{7x+3}{9x−9}\) 67. \(f^{-1}(x)=−\frac{3x−2}{4x−4}\) 69. \(f^{-1}(x)=−\frac{2x+1}{2x−4}\)
G: Find inverses of odd degree power and root functions
Exercise \(\PageIndex{G}\)
\( \bigstar \) Find the formula for the inverse function \(f^{−1}(x)\).
|
71) \(f(x)=x^3+1 \\[2pt] \) 72) \(f(x)=x^3−27 \\[2pt] \) 73) \(f(x)=(x-4)^3 \\[2pt] \) 74) \(y=(x+8)^3+3\) |
75) \(f(x) = 5x^3−5 \\[2pt] \) 76) \(f(x) = 4x^5−9 \\[2pt] \) 77) \(f(x) = 3x^5−9 \\[2pt] \) 78) \(f(x) = 5x^7+4\) |
79) \(f(x) = 9x^9+5 \\[2pt] \) 80) \(f(x) = 4x^7−3 \\[2pt] \) 81) \(f(x)=\sqrt[3]{x−4} \\[2pt] \) 82) \(f(x)=\sqrt[3]{3x+1}\) |
83) \(f(x)=\sqrt[3]{x} + 5 \\[2pt] \) 84) \(f(x)=\sqrt[3]{x} - 8 \\[2pt] \) 85) \(f(x) = \sqrt[3]{−6x−4} \\[2pt] \) 86) \(f(x) = \sqrt[3]{9x−7}\) |
87) \(f(x) = \sqrt[7]{−3x−5} \\[2pt] \) 88) \(f(x) = \sqrt[7]{8x−3} \\[2pt] \) 89) \(f(x) = \sqrt[3]{6x+7} \\[2pt] \) 90) \(f(x) = \sqrt[9]{8x+2}\) |
- Answers to Odd Exercises:
-
71. \(f^{−1}(x)=\sqrt[3]{x-1} \) 73. \(f^{−1}(x) = 4 + \sqrt[3]{x}\) 75. \(\sqrt[3]{\frac{x+5}{5}}\) 77. \(\sqrt[5]{\frac{x+9}{3}}\) 79. \(\sqrt[9]{\frac{x−5}{9}} \)
81. \(f^{-1}(x) = x^3+4 \) 83. \(f^{-1}(x) = (x-5)^3 \) 85. \(−\frac{x^3+4}{6}\) 87. \(−\frac{x^7+5}{3}\) 89. \(\frac{x^3−7}{6}\)
H: Find inverses of even degree power and root functions
Exercise \(\PageIndex{H}\) ]
\( \bigstar \) Find \(f^{-1}(x)\) for each function below. In #105-108 also state the restrictions for \(x\) in \(f^{-1}\).
|
91) \(f(x) = x^4, \;\) \(x \le 0 \\[2pt] \) 92) \(f(x) = x^4, \;\) \(x \ge 0 \\[2pt] \) 93) \(f(x) = x^2−1, \;\) \(x \le 0 \\[2pt] \) 94) \(f(x) = x^2+2, \;\) \(x \ge 0 \\[2pt] \) 95) \(f(x)=x^2−4, \; x≥0 \\[2pt] \) 96) \(f(x)=x^2+11, \;x≤0\) |
97) \(f(x) = x^4+3, \;\) \(x \le 0 \\[2pt] \) 98) \(f(x) = x^4−5, \;\) \(x \ge 0 \\[2pt] \) 99) \(f(x)=(x−1)^2, \; x≥1 \\[2pt] \) 100) \(f(x)=(x+3)^2, \; x≥-3 \\[2pt] \) 101) \(f(x) = (x−1)^2, \;\) \(x \le 1 \\[2pt] \) 102) \(f(x) = (x+2)^2, \;\) \(x \ge −2\) |
103) \(f(x)=x^2-8x+3, \; x≤4 \\[2pt] \) 104) \(f(x)=x^2+2x+50, \; x≥-1 \\[2pt] \) 105) \(f(x)=\sqrt{x−1} \\[2pt] \) 106) \(f(x)=\sqrt{x+2} \\[2pt] \) 107) \(f(x)=\sqrt{x}+9 \\[2pt] \) 108) \(f(x)=\sqrt{x}-1\) |
- Answers to Odd Exercises:
-
91. \(f^{-1}(x) =−\sqrt[4]{x}\)
93. \(f^{-1}(x) =−\sqrt{x+1}\)
95. \(f^{-1}(x) = \sqrt{x+4} \)
97. \(f^{-1}(x) =−\sqrt[4]{x−3}\)
99. \(f^{-1}(x) = 1+ \sqrt{x} \)
101. \(f^{-1}(x) =−\sqrt{x}+1\)
103. \(f^{-1}(x) = 4-\sqrt{x+13} \)
105. \(f^{−1}(x)=x^2+1\), \(x≥0\)
107. \(f^{-1}(x) = (x-9)^2,\) \(x≥9\)
I: Find the inverse and its domain and range
Exercise \(\PageIndex{I}\)
\( \bigstar \) Find a domain on which \(f\) is one-to-one and non-decreasing. Then find the inverse of \(f\).
| 111) \(f(x)=(x+7)^2\) | 112) \(f(x)=(x−6)^2\) | 113) \(f(x)=x^2−5\) | 114) \( f(x)=3(x−4)^2+1\) |
- Answers to Odd Exercises
-
111. domain of \(f(x)\): \(\left[−7,\infty\right)\); \(\quad\) \(f^{-1}(x)=\sqrt{x}−7\) 113. domain of \(f(x)\): \(\left[0,\infty\right)\); \(\quad\) \(f^{-1}(x)=\sqrt{x+5}\)