2.2e: Exercises - Attributes of Functions
- Page ID
- 38322
A: Concepts
Exercise \(\PageIndex{A}\)
1) Why does the domain differ for different functions?
2) How do we determine the domain of a function defined by an equation?
3) Explain why the domain of \(f(x)=\sqrt[3]{x}\) is different from the domain of \(f(x)=\sqrt{x}\).
4) When describing sets of numbers using interval notation, when do you use a parenthesis and when do you use a bracket?
5) How do you graph a piecewise function?
- Answers to Odd Exercises:
-
1. The domain of a function depends upon what values of the independent variable make the function undefined or imaginary.
3. There is no restriction on x for \(f(x)=\sqrt[3]{x}\) because you can take the cube root of any real number. So the domain is all real numbers, \((−∞,∞)\). When dealing with the set of real numbers, you cannot take the square root of negative numbers. So x-values are restricted for \(f(x)=\sqrt{x}\) to nonnegative numbers and the domain is \([0,∞)\).
5. Graph each formula of the piecewise function over its corresponding domain. Use the same scale for the x-axis and y-axis for each graph. Indicate inclusive endpoints with a solid circle and exclusive endpoints with an open circle. Use an arrow to indicate \(−∞\) or \(∞\). Combine the graphs to find the graph of the piecewise function.
B: Find the Domain given an Equation
Exercise \(\PageIndex{B}\)
\( \bigstar \) Find the domain of each function. State the domain in interval notation.
|
7) \(f(x)=5−2x^2 \\[3pt]\) 8) \(f(x)=−2x(x−1)(x−2) \\[3pt]\) 9) \(f(x)=3\sqrt{x-2} \\[3pt]\) 10) \(f(x)=3-\sqrt{6-2x} \\[3pt]\) 11) \(f(x)=\sqrt{4-3x} \\[3pt]\) 12) \(f(x)=\sqrt{x^2+4} \\[3pt]\) 13. \( f(x)=\sqrt{12-3x}+\sqrt{x-1} \\[3pt]\) 14. \(f(x)=\sqrt[3]{1-2x} \\[3pt]\) 15) \(f(x)=\sqrt[3]{x-1} \\[3pt] \) 16) \(f(x)=\dfrac{9}{x-6} \) |
17) \(f(x)=\dfrac{1}{x^2-x-6} \\[5pt]\) 18) \(f(x)=\dfrac{x}{x} \\[5pt]\) 19) \(f(x)=\dfrac{3x+1}{4x+2} \\[5pt]\) 20) \(f(x)=\dfrac{x-3}{x^2+9x-22} \\[5pt]\) 21) \(f(x)=\dfrac{x^2-9x}{x^2-81} \\[5pt]\) 22) \(f(x)=\dfrac{2x^3−250}{x^2−2x−15} \\[6pt]\) 23) \(f(x)=\dfrac{\sqrt{x+4}}{x-4} \\[6pt]\) 24) \( f(x)=\dfrac{x}{x-5}+\dfrac{\sqrt{x-3}}{2x-7}\) |
25) \( f(x)=\dfrac{5}{\sqrt{x-3}} \\[6pt]\) 26) \( f(x)=\dfrac{2x+1}{\sqrt{5-x}} \\[6pt]\) 27) \( f(x)=\dfrac{\sqrt{x-6}}{\sqrt{x-4}} \\[6pt] \) 28) \( f(x)=\dfrac{\sqrt{x-4}}{\sqrt{6-x}} \) 30) \(f(x)= \sqrt{x^2-6x+8} \\[3pt]\) 30.1) \(f(x)= \sqrt{x^2(x-1)(x+3)} \\[3pt]\) 30.2) \(f(x)= \sqrt{\dfrac{(x-1)}{(x+2)(x+5)^2}} \\[3pt]\) 30.3) \(f(x)= \sqrt{\dfrac{(x-1)^2}{(x-2)^2(x+7)}} \\[3pt]\) |
- Answers to Odd Exercises:
-
7. \((-\infty,\infty)\)
9. \( [2,\infty)\)
11. \( \left(-\infty,\dfrac{4}{3} \right] \)
13. \( [1,4] \)
15. \((-\infty,\infty)\)
17. \((-\infty,-2)\cup(-2,3)\cup(3,\infty)\)
19. \(\left (-\infty,-\dfrac{1}{2} \right )\cup \left (-\dfrac{1}{2},\infty \right )\)
21. \((-\infty,-9)\cup(-9,9)\cup(9,\infty)\)
23. \( [-4,4)\cup(4,\infty)\)
25. \( (3,\infty) \)
27. \(\left[6,\infty\right)\)
C: Find the Domain, Range, and Intercepts given a Continuous Graph
Exercise \(\PageIndex{C}\)
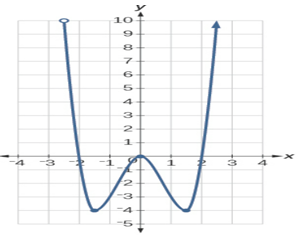
\( \bigstar \) For each function whose graph is illustrated below, (a) Determine the domain and range and state results in interval notation, and (b) State the \(x\)- and \(y\)-intercepts.
|
31)
|
32)
|
33)
|
|
|
35)
|
36) 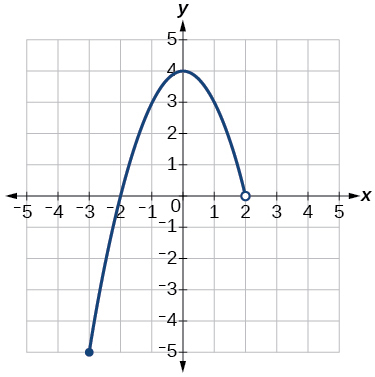 |
37)
|
38)
|
|
39)
|
40)
|
41) 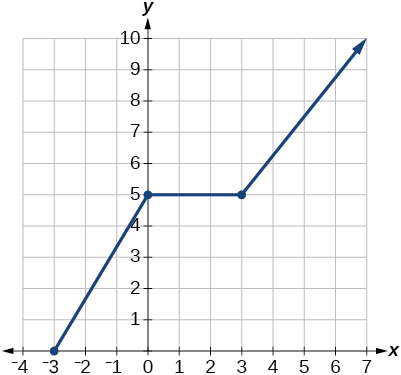 |
- Answers to Odd Exercises:
-
31. (a) domain: \((2,8]\); range: \([6,8) \);
(b) no intercepts33. (a) domain: \( [-4,4] \); range: \( [0,2] \);
(b) intercepts: \((-4,0)\), \((4,0)\), \((0,2)\)35. (a) domain: \([−5,3)\); range: \([0,2]\);
(b) intercepts: \((-1,0)\), \((0, \approx .1)\)37. (a) domain: \(\left(−\infty,1\right]\), range: \(\left[0,\infty\right)\);
(b) intercepts: \( (1,0) \), \( (0,1.5) \)39. (a) domain: \([−6,−\frac{1}{6}]\cup[\frac{1}{6},6]\);
range: \([−6,−\frac{1}{6}]\cup[\frac{1}{6},6]\);
(b) no intercepts41. (a) domain: \(\left[−3, \infty\right)\); range: \(\left[0,\infty\right)\);
(b) intercepts: \( (-3,0) \), \( (0,5) \)
D: Find Domain and Range given a Piecewise Graph
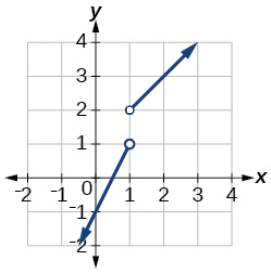
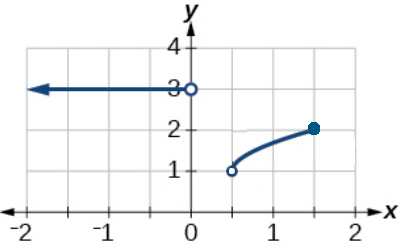
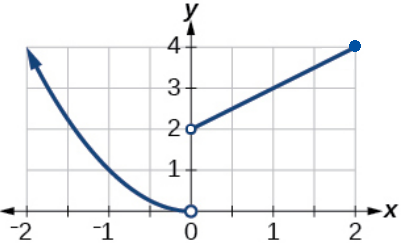
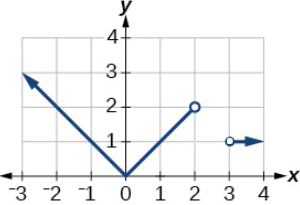
Exercise \(\PageIndex{D}\)
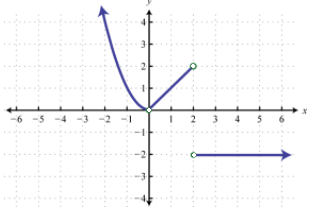
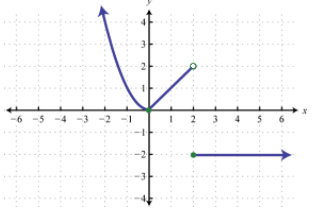
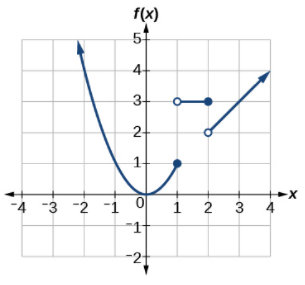
\( \bigstar \) Find the domain and range of the following graphs.
|
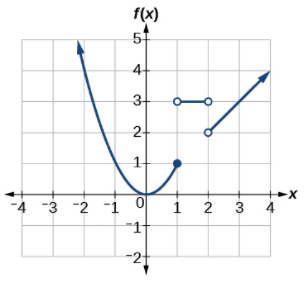
48. (a)
|
(b)
|
49. (a) |
(b) |
|
50. (a) |
(b) |
51. (a) |
(b) |
|
52.
|
53.
|
54.
|
55.
|
|
56.
|
57.
|
- Answers to Odd Exercises:
-
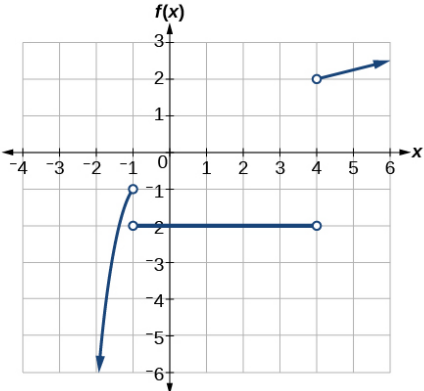
49. (a) domain: \( ( -\infty, \infty) \), range: \( [0, \infty ) \) (b) domain: \( ( -\infty, 2) \cup (2,\infty) \), range: \( [0, \infty ) \)
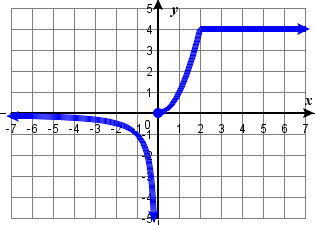
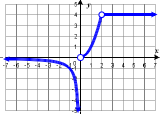
51. (a) domain: \( ( -\infty, -4) \cup (-4, \infty ) \), range: \( [-2, \infty) \) (b) domain: \( ( -\infty, -2 ) \cup (-2, \infty ) \), range: \( [-2, \infty) \)
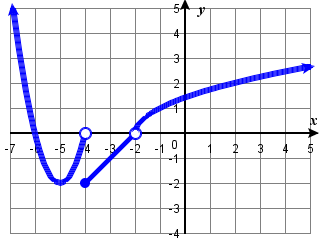
53. domain: \( ( -\infty, 0) \cup (1/2, 3/2] \), range: \( (1,2] \cup [3, 3] \) 55. domain: \( ( -\infty, 2) \cup (3, \infty) \), range: \( [0, \infty) \)
57. domain: \( ( -\infty, -2) \cup (-2,0) \cup (0,2) \cup (2, \infty ) \), range: \( ( 0, \infty) \)
E: Find Zeros and Intercepts given a Function
Exercise \(\PageIndex{E}\): Find Zeros and Intercepts for a Function
\( \bigstar \) Find the zeros and intercepts of each function below.
|
63) \(f ( x ) = 9 x ^ { 2 } - 12 x + 4\) 64) \(p ( x ) = 64 x ^ { 2 } - 1\) 65) \(f ( x ) = ( x + 5 ) ^ { 2 } + 1\) 66) \(f ( x ) = ( 3 x - 4 ) ^ { 2 } + 7\) 67) \(g ( x ) = - ( x + 1 ) ^ { 4 } + 16\) 68) \(f ( x ) = ( 2x -3 ) ^ { 2 } - 4\) 68.1) \(f ( x ) = \frac { 1 } { 3 } x ^ { 3 } + \frac { 1 } { 2 } x ^ { 2 } + \frac { 4 } { 3 } x + 2\) 69.1) \( f(x) = 3x^3 - 2x^2 +27x -18 \) |
69.2) \(f ( x ) = \dfrac { 4 x ^ { 2 } - 9 } { 2 x - 3 }\) 70) \(f ( x ) = \dfrac { 3 x ^ { 2 } - 2 x - 1 } { x ^ { 2 } - 1 }\) 71) \(f ( x ) = \dfrac { 1 } { x } - 3\) 72) \(f(x)=\dfrac{x}{x^2−16}\) 73) \(f ( x ) = \dfrac { 1 } { x + 5 }\) 74) \(h(x)=\dfrac{3}{x^2+4}\) |
75) \(f ( x ) = \sqrt { 2 x - 3 } - 1\) 76) \(f ( x ) = 3 \sqrt { x - 7 } - 6\) 77) \(f ( x ) = 2 \sqrt { x + 2 } - 8\) 78) \(f(x)=−1+\sqrt{x+2}\) 79) \(f ( x ) = \sqrt [ 3 ] { x -8 } - 2\) 80) \(f ( x ) = 2 \sqrt [ 3 ] { x - 1 } + 6\) 81) \(g(x)=\sqrt{\dfrac{7}{x−5}}\) 82) \(f(x)=4|x^2+5|\) |
- Answers to Odd Exercises:
-
63). zeros: {2/3}; \(x\)-intercepts: (2/3,0); \(y\)-intercepts: (0,4) 65. {\(-5 \pm i\)}; no \(x\) intercepts; (0, 26)
67). { \(\pm 2\), \(\pm 2i\) }; (2,0), (-2,0); (0,15) 69.1. zeros: \( \{ \pm 3i, \frac{2}{3} \); \(x\)-intercepts: (3/2,0); \(y\)-intercepts: (0,-18)
69.2). {-3/2}; (-3/2,0); (0,3) 71). {1/3}; (1/3, 0); no \(y\)-intercepts 73). no zeros; no \(x\)-intercepts; (0, \(\frac{1}{5}\) )
75). {2}; (2,0); no \(y\)-intercept 77). {14}; (14,0); (0, \(2\sqrt{2}-8\) )
79). {16}; (16,0); (0, -4) 81). no zeros; no \(x\)-intercepts; no \(y\)-intercept.
F: Find Zeros, Intercepts, and Symmetry given an Equation
Exercise \(\PageIndex{F}\): Find Zeros, Intercepts and Symmetry for a graph given its equation
\( \bigstar \) For each equation below (a) Find the zeros and \(x\)- and \(y\)-intercept(s) of the graph, if any exist and (b) Test for symmetry.
|
|
|
|
- Answers to odd exercises.
-
83. (a) The graph has no \(x\)-intercepts, \(y\)-intercept: \( (0, 1) \), zeros: \( \{ \pm i \} \).
(b) \(x\)-axis symmetry: no. \(y\)-axis symmetry: yes. Origin symmetry: no.85. \(x\)-intercepts: \( (-1, 0), (0, 0), (1, 0) \), \(y\)-intercept: \( (0, 0) \), zeros: \( \{ -1,\; 0,\; 1\} \).
(b) \(x\)-axis symmetry: no. \(y\)-axis symmetry: no. Origin symmetry: yes.87. \(x\)-intercept: \( (2, 0) \), The graph has no \(y\)-intercepts, zeros: \( \{ 2 \} \).
(b) \(x\)-axis symmetry: no. \(y\)-axis symmetry: no. Origin symmetry: no.89. \(x\)-intercept: \( (\frac{7}{3}, 0) \), \(y\)-intercept: \( (0, -7) \), zeros: \( \{ \frac{7}{3} \} \).
(b) \(x\)-axis symmetry: no. \(y\)-axis symmetry: no. Origin symmetry: no.91. \(x\)-intercepts: \( (-6, 0) \), \( (2,0) \), \(y\)-intercepts: \( \left(0, \pm 2\sqrt{3}\right) \), zeros: \( \{ 2, -6 \} \).
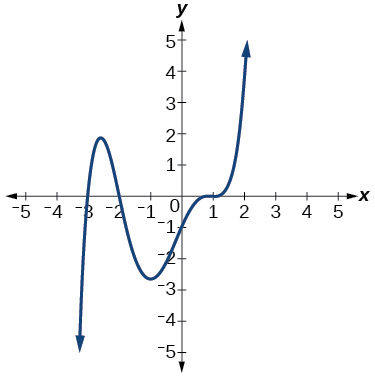
(b) \(x\)-axis symmetry: yes. \(y\)-axis symmetry: no. Origin symmetry: no.93. The graph has no \(x\)-intercepts, \(y\)-intercepts: \( (0, \pm 3) \), zeros: \( \{ \pm 2i \} \).
(b) \(x\)-axis symmetry: yes. \(y\)-axis symmetry: yes. Origin symmetry: yes.
G: Find Symmetry for an Equation or a Function
Exercise \(\PageIndex{G}\)
\( \bigstar \) Determine the symmetry for the following equations
|
|
|
- Answers to Odd Exercises:
-
97) no symmetry 99) x-axis symmetry 101) y-axis symmetry 103) x-axis symmetry 105) origin symmetry
|
|
|
117. \(h(x)=\dfrac{1}{x}+3x \\[2pt] \) |
- Answers to Odd Exercises:
-
109) (a) y-axis symmetry, (b) even function 111) (a) neither y-axis or origin symmetry, (b) neither even nor odd
113) (a) y-axis symmetry, (b) even function 115) (a) y-axis symmetry, (b) even function 117) (a) origin symmetry, (b) odd function,
H: Attributes from Graphs
Exercise \(\PageIndex{H}\)
\( \bigstar \) Determine the following for each graph below. These graphs do not have arrows, but assume that a graph continues at both ends if it extends beyond the given grid.
- Domain and range
- \(x\) -intercept, if any (estimate where necessary)
- \(y\)-Intercept, if any (estimate where necessary)
- The intervals for which the function is increasing (only if a function)
- The intervals for which the function is decreasing (only if a function)
- The intervals for which the function is constant (only if a function)
- Symmetry about any axis and/or the origin
- Whether the function is even, odd, or neither (only if a function)
|
121)
|
122)
|
123)
|
124)
|
|
125)
|
126)
|
127)
|
128)
|
- Answers to Odd Exercises:
-
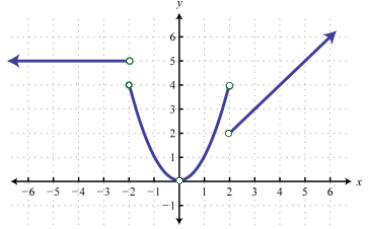
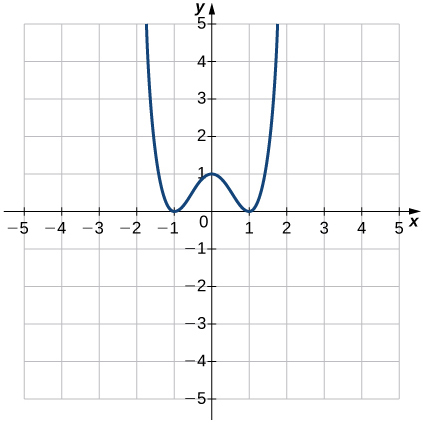
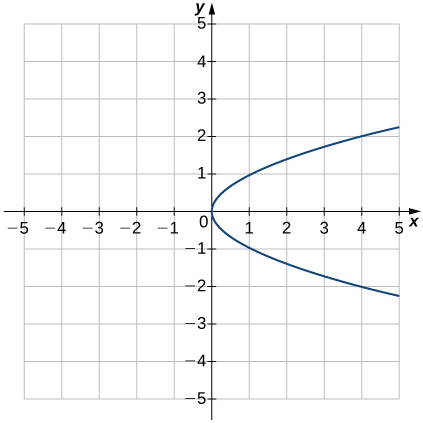
121) Solution: Function; a. Domain: \( ( -\infty, \infty) \), range: \( [0, \infty ) \) b. \( (-1, 0)\), \( (1, 0)\) c. \( (0,1) \) d. \(−1<x<0\) and \( x > 1 \) e. \( x<−1\) and \(0<x<1\) f. Not constant g. \(y\)-axis h. Even
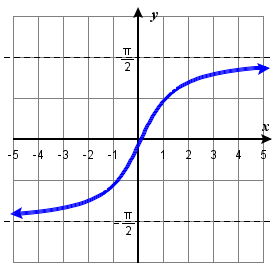
123) Solution: Function; a. Domain: \( ( -\infty, \infty) \), range: \( (−\frac{\pi}{2}, \frac{\pi}{2}) \) b. \( (0, 0) \) c. \( (0, 0) \) d. all real numbers e. None f. Not constant g. Origin h. Odd
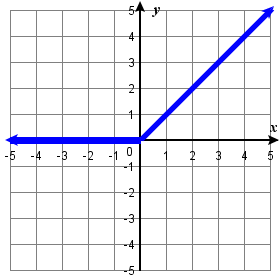
125) Solution: Function; a. Domain: \( ( -\infty, \infty) \), range: \( [0, \infty ) \) b. \( \{ (x,0) | \; x ≤ 0 \} \) c. \( (0, 0) \) d. \(x>0\) e. Not decreasing f. \(x<0\) g. No Symmetry h. Neither
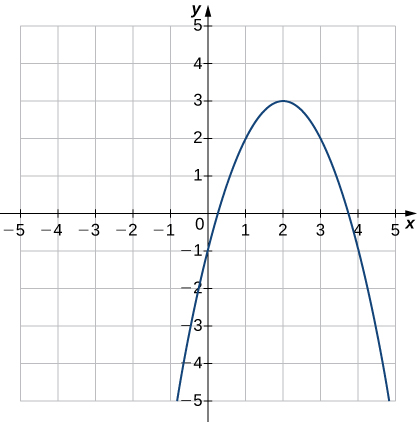
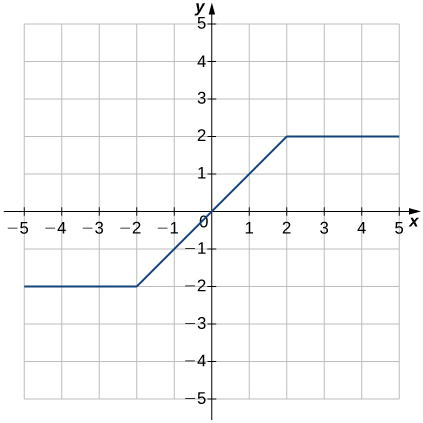
127) Solution: Function; a. Domain: \( [-4, 4 ] \), range: \( [-4, 4 ] \) b. \( ( \approx 1.2, 0) \) c. \( (0, 4) \) d. Not increasing e. \(0<x<4\) f. \(−4<x<0\) g. No Symmetry h. Neither
I: Extrema and Continuity given a Graph
Exercise \(\PageIndex{I}\)
\( \bigstar \) Find the following attributes in the graphs below.
Assume that graphs do NOT extend beyond the given grid unless arrows are drawn. For minima and maxima, state the coordinates of the point or the label on the point as appropriate. Estimate if needed.
a) Local minima b) Absolute minima c) Local maxima d) Absolute maxima
e) Places where the function is discontinuous
| 131) | 132) | ||
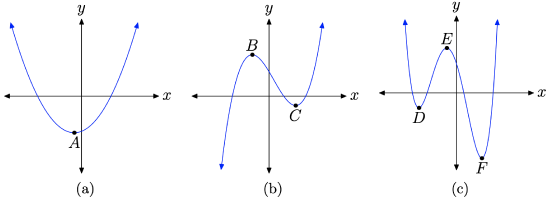 |
 |
||
Assume that graphs do NOT extend beyond the given grid unless arrows are drawn. Estimate if needed.
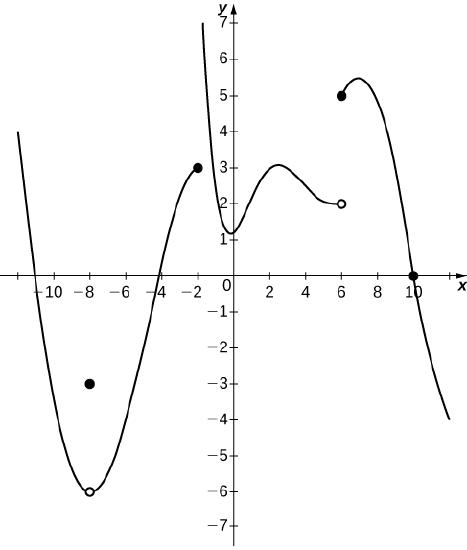
| 133) | 134) | 135) | 136) |
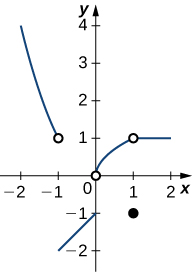 |
 |
 |
 |
- Answers to Odd Exercises:
-
131a) (a) A, (b) A, (c) none, (d) none (e) none; 131b) (a) C, (b) none, (c) B, (d) none, (e) none
131c) (a) D,F, (b) F, (c) E, (d) none, (e) none
133) (a) (1,-1), (b) (-1,-2); (c) none, (d) (-2,4); (e) x=-1, x=0, x=1
135) (a) (0, 1.2), (b) none; (c) (-8, -3), (2,3), (7, 5.5) (d) (-2,7) (e) x=-8, x=-2, x=6.
.


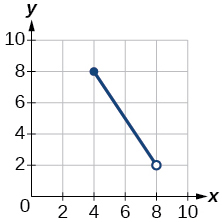
![Graph of a function from \(\left(2, 8\right]\).](https://math.libretexts.org/@api/deki/files/1092/CNX_Precalc_Figure_01_02_202.jpg?revision=1)
![Graph of a function [-4,4]](https://math.libretexts.org/@api/deki/files/1094/CNX_Precalc_Figure_01_02_204.jpg?revision=1&size=bestfit&height=135)

![Graph of a function from (-infinity, 2].](https://math.libretexts.org/@api/deki/files/1098/CNX_Precalc_Figure_01_02_208.jpg?revision=1)

![Graph of a function from [-6, -1/6]U[1/6, 6]/.](https://math.libretexts.org/@api/deki/files/1100/CNX_Precalc_Figure_01_02_210.jpg?revision=1)