Chapter 7 Review Exercises

Last updated
Save as PDF

Page ID: 10777

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

In exercises 1 - 4, determine whether the statement is true or false. Justify your answer with a proof or a counterexample.

1) $\displaystyle ∫e^x\sin(x)\,dx$ cannot be integrated by parts.

2) $\displaystyle ∫\frac{1}{x^4+1}\,dx$ cannot be integrated using partial fractions.

Answer: False

3) In numerical integration, increasing the number of points decreases the error.

4) Integration by parts can always yield the integral.

Answer: False

In exercises 5 - 10, evaluate the integral using the specified method.

5) $\displaystyle ∫x^2\sin(4x)\,dx,$ using integration by parts

6) $\displaystyle ∫\frac{1}{x^2\sqrt{x^2+16}}\,dx,$ using trigonometric substitution

Answer: $\displaystyle ∫\frac{1}{x^2\sqrt{x^2+16}}\,dx = −\frac{\sqrt{x^2+16}}{16x}+C$

7) $\displaystyle ∫\sqrt{x}\ln x\,dx,$ using integration by parts

8) $\displaystyle ∫\frac{3x}{x^3+2x^2−5x−6}\,dx,$ using partial fractions

Answer: $\displaystyle ∫\frac{3x}{x^3+2x^2−5x−6}\,dx = \frac{1}{10}\big(4\ln|2−x|+5\ln|x+1|−9\ln|x+3|\big)+C$

9) $\displaystyle ∫\frac{x^5}{(4x^2+4)^{5/2}}\,dx,$ using trigonometric substitution

10) $\displaystyle ∫\frac{\sqrt{4−\sin^2(x)}}{\sin^2(x)}\cos(x)\,dx,$ using a table of integrals or a CAS

Answer: $\displaystyle ∫\frac{\sqrt{4−\sin^2(x)}}{\sin^2(x)}\cos(x)\,dx = −\frac{\sqrt{4−\sin^2(x)}}{\sin(x)}−\frac{x}{2}+C$

In exercises 11 - 15, integrate using whatever method you choose.

11) $\displaystyle ∫\sin^2 x\cos^2 x\,dx$

12) $\displaystyle ∫x^3\sqrt{x^2+2}\,dx$

Answer: $\displaystyle ∫x^3\sqrt{x^2+2}\,dx = \frac{1}{15}(x^2+2)^{3/2}(3x^2−4)+C$

13) $\displaystyle ∫\frac{3x^2+1}{x^4−2x^3−x^2+2x}\,dx$

14) $\displaystyle ∫\frac{1}{x^4+4}\,dx$

Answer: $\displaystyle ∫\frac{1}{x^4+4}\,dx = \frac{1}{16}\ln(\frac{x^2+2x+2}{x^2−2x+2})−\frac{1}{8}\tan^{−1}(1−x)+\frac{1}{8}\tan^{−1}(x+1)+C$

15) $\displaystyle ∫\frac{\sqrt{3+16x^4}}{x^4}\,dx$

In exercises 16 - 18, approximate the integrals using the midpoint rule, trapezoidal rule, and Simpson’s rule using four subintervals, rounding to three decimals.

16) [T] $\displaystyle ∫^2_1\sqrt{x^5+2}\,dx$

Answer: $M_4=3.312,$
$T_4=3.354,$
$S_4=3.326$

17) [T] $\displaystyle ∫^{\sqrt{π}}_0e^{−\sin(x^2)}\,dx$

18) [T] $\displaystyle ∫^4_1\frac{\ln(1/x)}{x}\,dx$

Answer: $M_4=−0.982,$
$T_4=−0.917,$
$S_4=−0.952$

In exercises 19 - 20, evaluate the integrals, if possible.

19) $\displaystyle ∫^∞_1\frac{1}{x^n}\,dx,$ for what values of $n$ does this integral converge or diverge?

20) $\displaystyle ∫^∞_1\frac{e^{−x}}{x}\,dx$

Answer: approximately 0.2194

In exercises 21 - 22, consider the gamma function given by $\displaystyle Γ(a)=∫^∞_0e^{−y}y^{a−1}\,dy.$

21) Show that $\displaystyle Γ(a)=(a−1)Γ(a−1).$

22) Extend to show that $\displaystyle Γ(a)=(a−1)!,$ assuming $a$ is a positive integer.

The fastest car in the world, the Bugati Veyron, can reach a top speed of 408 km/h. The graph represents its velocity.

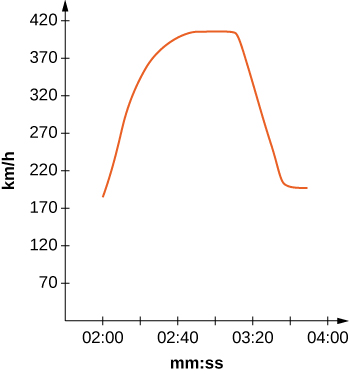
$This figure has a graph in the first quadrant. It increases to where x is approximately 03:00 mm:ss and then drops off steep. The maximum height of the graph, here the drop occurs is approximately 420 km/h.$

23) [T] Use the graph to estimate the velocity every 20 sec and fit to a graph of the form $v(t)=ae^{bx}\sin(cx)+d.$ (Hint: Consider the time units.)

24) [T] Using your function from the previous problem, find exactly how far the Bugati Veyron traveled in the 1 min 40 sec included in the graph.

Answer: Answers may vary. Ex: $9.405$ km