0.3: Functions

 

Definition:  Cartesian Product

Let $A$ be a set. Then $A \times A= \{(a_1,a_2)| a_1, a_2 \in A  \}$ is the Cartesian product of $A$.

Example $\PageIndex{1}$

If $A = \mathbb{R}$ then this is the Cartesian plane -  Like $\{x,y: x,y \in A\}$.

Definition: Function

A function $f$ is a subset (symbol is $\subseteq$) of $A \times B$ such that for every $a \in A$ there exists a unique $b \in B$ such that $f(a)=b$. 

Denoted by $f: A \rightarrow B$ or $A \xrightarrow{f} B$.

$A$ is the domain of the function $f$.  

$B$ is the co-domain of the function $f$.

The range of $f$ is $f(A)=\big{\{}f(x): x \in A \big{\}} \subseteq B = Im(f)$, the image of $f.$

A well-defined function is one that for every value of $A$, there is one and only one value of $B$.

 

Definition: 

Let $f$ be a function from $A \rightarrow B$.

1. $f$ is called onto (surjective) if $f(A)=B$.

2. $f$ is called one to one (injective) if $f(x_1)=f(x_2), x_1,x_2 \in A$ then $x_1=x_2$.

3. $f$ is bijective if it is surjective and injective.

4. A function can be injective, surjective, bijective (both injective & surjective), or neither.

 Note: 

Let $f: A \rightarrow B$.  If $f$ is 1-1 and onto then $f$ is a bijection from $A \rightarrow B.$ 

If there is a bijection between two finite sets, the number of elements in each set is the same.  Notationally:  |A| = |B|.

Example $\PageIndex{2}$

Example $\PageIndex{3}$

Consider the mapping:

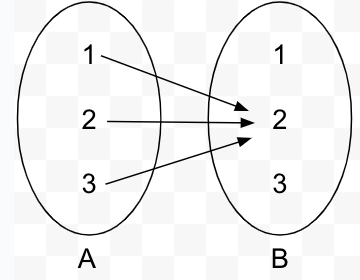
Is this mapping 

a) Well defined?

b)  Is it surjective? 

c) Is it injective? 

d) Is it bijective?

Solution

The function is well-defined. Not injective. Not subjective, not bijective.

 

Example $\PageIndex{4}$

Consider the mapping:

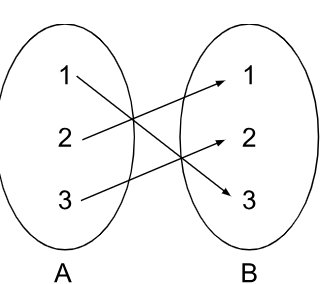
Is this mapping 

a) Well defined?

b)  Is it surjective? 

c) Is it injective? 

d) Is it bijective?

Solution

This is well defined bijective function.

Example $\PageIndex{5}$

Given $f: \mathbb{Q} \rightarrow \mathbb{Z}$ where $\mathbb{Q}=\{\frac{a}{b}:a,b \in \mathbb{Z}, b \ne 0 \}$ and $f(\frac{a}{b})=a$.  Is this a well-defined function?  

Solution

Let $A$ and $B$ be sets such that $A=\{\frac{a}{b}:a,b \in \mathbb{Z}, a \ne 0\}$ and $B=\mathbb{Z}$.

Using a counterexample, $f(\frac{a}{b})$ is not a well-defined function since $f(\frac{1}{2})=1$ and $f(\frac{2}{4})=2$, but $\frac{1}{2}=\frac{2}{4}$.  This is an example of an element in $A$ that maps to more than one element in $B$.

 

Example $\PageIndex{6}$

Let

 $\mathbb{Q}=\big{\{}\frac{a}{b}: b> 0,\text{and } gcd(a,b)=1 \big{\}}$

and $f: \mathbb{Q} \rightarrow \mathbb{Z}$ that is defined by

 $f(\frac{a}{b})=a.$

 a) Is $f$ a well-defined function? b)  Is it surjective?  c) Is it injective?  d) Is it bijective?

Solution

1. The function is well-defined.

Let $A$ and $B$ be set.

Let $a \in A$.

We need to show that if $f(a)=b$ then $b\in B$ and if $f(a)=b_1$ that $b=b_1$.

We are given $f: \mathbb{Q} \rightarrow \mathbb{Z}$, which means that this is a function thus $f(a)$ will map to one and only one element of $B$.  Thus if $f(a)=b$ and if $f(a)=b_1$ then $b=b_1$.

In b) below, we have shown that the function is surjective, thus $f(a)=b$,

 

2. The function is surjective

   Proof:

We shall show that $f(\mathbb{Q})=\mathbb{Z}$ by showing that  $f(\mathbb{Q}) \subseteq \mathbb{Z}$ and  $\mathbb{Z} \subseteq f(\mathbb{Q})$.

Since we are given $f: \mathbb{Q} \rightarrow \mathbb{Z}$,  $f(\mathbb{Q}) \subseteq \mathbb{Z}$.

Next, we shall show that $\mathbb{Z} \subseteq f(\mathbb{Q})$.

Let $n \in \mathbb{Z}$, then $\frac{n}{1} \in \mathbb{Q}$ and $f\big(\frac{n}{1}\big)=n$.

Hence $n \in f(\mathbb{Q})$.

Hence $\mathbb{Z} \subseteq f(\mathbb{Q})$.

Since $\mathbb{Z} \subseteq f(\mathbb{Q})$ and $f(\mathbb{Q}) \subseteq \mathbb{Z}, f(\mathbb{Q})=\mathbb{Z}$.◻

 

3. The function is not injective.

   Counterexample:

Let $A=\big{\{}\frac{a}{b}: b> 0,\text{and } gcd(a,b)=1 \big{\}}$ and $B=\mathbb{Z}$.

Note $\frac{1}{4},  \frac{1}{3} \in A$ and that $f(\frac{1}{4}),  f(\frac{1}{3}) \in B$ and that $f(\frac{1}{4})=f(\frac{1}{3})=1$. 

Since $\frac{1}{4} \ne \frac{1}{3}$ , the function is not injective.

 

4. The function is not bijective since it has been shown not to be both injective and surjective.

 

Operation on functions: Composition

 

Definition:Composition of functions

Let $f: A \rightarrow B$ and $g: B \rightarrow C$. Then the composition functions of $g$ and $f$, $g \circ f: A \to C$, defined by 

$(g \circ f)(a)=g(f(a)), \; \forall a \in A$.

Definition: Identity function

Identity function $id_A: A \rightarrow A$ defined by $id_A(x)=x, \forall x \in A$.

Definition: Inverse of a function

Let $f: A \rightarrow B$ then $f$ has an inverse $g: B \rightarrow A$ if $(f \circ g)= id_B$ and $(g \circ f)=id_A$.  Note the domain of $f \circ g$ is the domain of $g$.

Let $f:A \rightarrow B$ be a bijection.  Then the inverse of $f$, denoted by $f^{-1}:B \rightarrow A$ defined by $f^{-1}(b)=a$ if $f(a)=b$ if $a \in A$ and $b \in B$. In this case, $f \circ f^{-1}=id_A,$ and $f^{-1}\circ f =id_B.$

 

 

Example $\PageIndex{7}$

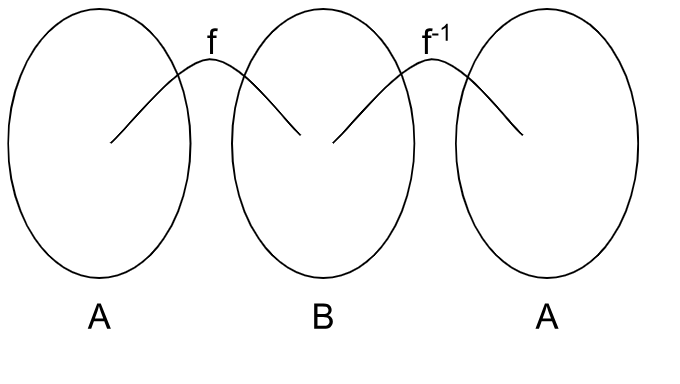
Let $f: A \rightarrow B$ and $g: B \rightarrow C$. Then $g \circ f: A \to C.$ 

1. If $f$ and $g$ are surjective, then $g \circ f$ is surjective.

Proof:

We will show that $g \circ f$ is surjective.

Let $c \in C$ . Since g is surjective, there exists $b \in B$  such that $g(b)=c.$

Since f is surjective, there exists $a \in A$  such that $f(b)=a$.

Hence there exists $a \in A$ such that $gf(f(a))=c.$

Therefore, if $f$ and $g$ are surjective, then $g \circ f$  is surjective.◻

(better proof) If $f$ is onto and $g$ is onto then $g \circ f$ is onto.

Proof:

We shall show that $(g \circ f)(A)=C$.

Since $f$ and $g$ are onto, by definition of a function, $(g \circ f)(A) \subseteq C$.

Now we need to show that $C \subseteq (g \circ f)(A)$.

Let $c \in C$.

Since $g$ is onto $\exists b \in B$ s.t. $c=g(b)$.

Since $f$ is onto, $\exists a \in A$ s.t. $b=f(a)$.

Consider $(g \circ f)(a)=g(f(a))$ (by definition)

        $=g(b)$

        $=c$.

Therefore $c \in (g \circ f)(A)$.

Hence $C \subseteq (g \circ f)(A)$.

Since $(g \circ f)(A) \subseteq C$ and $C \subseteq (g \circ f)(A)$, then $g \circ f$ is onto.◻

 

Example $\PageIndex{8}$

1. If $f$ and $g$ are injective, then $g \circ f$ is injective. That is,

If $f$ is 1-1 and $g$ is 1-1 then $g \circ f$ is 1-1.

Proof:

Let   $a_1,a_2 \in A$  such that $g \circ f (a_1)= g\circ f(a_2).$  Then $g(f(a_1))=g(f(a_2)).$

Since  $g$ is 1-1, $f(a_1)=f(a_2)$.

Since,  $f$ is 1-1, $a_1=a_2.$

 

Hence $g \circ f$  is injective.

 

 

Example $\PageIndex{9}$

Given $A$ is a matrix and $T_A:\mathbb{R}^2 \rightarrow \mathbb{R}^2$ defined by $T_A \begin{bmatrix} x\\ y\end{bmatrix} = \begin{bmatrix} 1 &1\\ 0 & 1\end{bmatrix} \begin{bmatrix} x\\ y\end{bmatrix}$.  Note this is a matrix transformation which is linear.

1. Is $T_A$ 1-1?

Yes, $T_A$ is 1-1.

Proof:

Let $\begin{bmatrix} x_1\\ y_1\end{bmatrix}$and $\begin{bmatrix} x_2\\ y_2\end{bmatrix} \in \mathbb{R}^2$ s.t. $T_A \begin{bmatrix} x_1\\ y_1\end{bmatrix} =T_A \begin{bmatrix} x_2\\ y_2\end{bmatrix}$.

We will show that $\begin{bmatrix} x_1\\ y_1\end{bmatrix} =\begin{bmatrix} x_2\\ y_2\end{bmatrix}$.

$T_A \begin{bmatrix} x\\ y\end{bmatrix} =\begin{bmatrix} x+y\\ y\end{bmatrix}$.

Thus  $\begin{bmatrix} x_1 +y_1\\ y_1\end{bmatrix} =\begin{bmatrix} x_2+y_2\\ y_2\end{bmatrix}$.

Thus $x_1+y_1=x_2+y_2$ and $y_1=y_2$.

Thus $x_1=x_2$ and $y_1=y_2$.

Hence $\begin{bmatrix} x_1\\ y_1\end{bmatrix} =\begin{bmatrix} x_2\\ y_2\end{bmatrix}$.

Thus $T_A$ is 1-1.◻

 

2. Is $T_A \begin{bmatrix} x\\ y\end{bmatrix}$ onto?

Yes, $T_A \begin{bmatrix} x\\ y\end{bmatrix}$ is onto.

Proof:

We know by definition that $T_A \begin{bmatrix} x\\ y\end{bmatrix} \subseteq \mathbb{R}^2$.

We need to show that $\mathbb{R}^2 \subseteq T_A \begin{bmatrix} x\\ y\end{bmatrix}$.

Let $\begin{bmatrix} a\\ b\end{bmatrix} \in \mathbb{R}^2$.

Find $\begin{bmatrix} x\\ y\end{bmatrix} \in \mathbb{R}^2$ s.t. $\begin{bmatrix} 1 &1\\ 0 & 1\end{bmatrix} \begin{bmatrix} x\\ y\end{bmatrix}=\begin{bmatrix} a\\ b\end{bmatrix}$.

$\begin{bmatrix} x+y\\ y\end{bmatrix} =\begin{bmatrix} a\\ b\end{bmatrix}$.

Thus $y=b$ and $x=a-b$.

Thus $T_A \begin{bmatrix} a-b\\ b\end{bmatrix} =\begin{bmatrix} a\\ b\end{bmatrix}$.

Thus $T_A$ is onto.◻

 

3. Does $T_A$ have an inverse?  If so, what is it?

a) Yes $T_A$ has an inverse since it is a bijection (1-1 and onto) ∴ $T_A^{-1}$ exists.

Proof:  By definition?  Obvious?

b) $T_A^{-1}: \mathbb{R}^2 \rightarrow \mathbb{R}^2$.

$T_A^{-1} \begin{bmatrix} x\\ y\end{bmatrix} =\begin{bmatrix} 1 & -1\\ 0 & 1\end{bmatrix} \begin{bmatrix} x\\ y\end{bmatrix}$

    $=\begin{bmatrix} x-y\\ y\end{bmatrix}$.

Check: $T_A^{-1} \cdot T_A=\begin{bmatrix} 1 & -1\\ 0 & 1 \end{bmatrix} \cdot \begin{bmatrix} 1 & 1\\ 0 & 1\end{bmatrix} =\begin{bmatrix} 1 & 0\\ 0 & 1 \end{bmatrix}$.

Example $\PageIndex{10}$

Let $f: A \rightarrow B$ and $g: B \rightarrow C$. Then $g \circ f:A \to C$  . Given $g \circ f$ is injective and $f$ is onto, prove that $g$ is injective.

Proof:

Assume $g \circ f$ is injective.  (by definition)

Let $x_1, x_2 \in B$ s.t. $g(x_1)=g(x_2)$.

We shall show that $x_1=x_2$.

Let $a_1, a_2 \in A$.

Since $f$ is surjective (by definition), we know that $f(a_1)=x_1$ and $f(a_2)=x_2$.

Since $x_1 \in B$ and $f$ is surjective, $\exists a_1 \in A$ s.t. $f(a_1)=x_1$.

Since $x_2 \in B$ and $f$ is surjective, $\exists a_2 \in A$ s.t. $f(a_2)=x_2$.

Since $g(x_1)=g(x_2)$, then $g(f(a_1))=g(f(a_2))$ and $g \circ f)(a_1)=(g \circ f)(a_2)$.

Since $g \circ f)$ is injective, $a_1=a_2$.

Thus $f(a_1)=f(a_2)$ since $f$ is a function.

Therefore, $x_1=x_2$.

Hence $g$ is injective.

Example $\PageIndex{11}$

Consider the mapping $\alpha$:

 

Find $\alpha^2$ , $\alpha^3$ and $\alpha^{-1}$.