Skip to main content
Mathematics LibreTexts

5.1: Ratios, Rates, Proportions

  1. Last updated
  2. Save as PDF
  • Page ID
    142721

    • \( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

    Ratios & Rates

    A ratio is the quotient of two numbers or the quotient of two quantities with the same units.

    When writing a ratio as a fraction, the first quantity is the numerator and the second quantity is the denominator.

    Exercise \(\PageIndex{1}\)

    1. Find the ratio of \(45\) minutes to \(2\) hours. Simplify the fraction, if possible.

    joshua-hoehne-1RGvrDGu9kA-unsplash-scaled.jpg

    Answer

    1. \(\dfrac{3}{8}\)

    A rate is the quotient of two quantities with different units. You must include the units.

    When writing a rate as a fraction, the first quantity is the numerator and the second quantity is the denominator. Simplify the fraction, if possible. Include the units in the fraction.

    Exercise \(\PageIndex{1}\)

    2. A car travels \(105\) miles in \(2\) hours. Write the rate as a fraction.

    Answer

    2. \(\dfrac{105\text{ mi}}{2\text{ hr}}\)

    A unit rate has a denominator of \(1\). If necessary, divide the numerator by the denominator and express the rate as a mixed number or decimal.

    Exercise \(\PageIndex{1}\)

    3. A car travels \(105\) miles in \(2\) hours. Write as a unit rate.

    Answer

    3. \(\dfrac{52.5\text{ mi}}{1\text{ hr}}\) or \(52.5\) miles per hour

    A unit price is a rate with the price in the numerator and a denominator equal to \(1\). The unit price tells the cost of one unit or one item. You can also simply divide the cost by the size or number of items.

    Exercises \(\PageIndex{1}\)

    4. An \(18\)-ounce box of cereal costs $ \(3.59\). Find the unit price.

    5. A \(12\)-ounce box of cereal costs $ \(2.99\). Find the unit price.

    6. Which box has a lower unit price?

    franki-chamaki-wkvKZR4e2OI-unsplash-scaled.jpg

    Answer

    4. $ \(0.199\)/oz, or around \(20\) cents per ounce

    5. $ \(0.249\)/oz, or around \(25\) cents per ounce

    6. the \(18\)-ounce box has the lower unit price

    Proportions

    A proportion says that two ratios (or rates) are equal.

    Exercises \(\PageIndex{1}\)

    Determine whether each proportion is true or false by simplifying each fraction.

    7. \(\dfrac{6}{8}=\dfrac{21}{28}\)

    8. \(\dfrac{10}{15}=\dfrac{16}{20}\)

    Answer

    7. \(\dfrac{3}{4}=\dfrac{3}{4}\); true

    8. \(\dfrac{2}{3} \neq \dfrac{4}{5}\); false

    A common method of determining whether a proportion is true or false is called cross-multiplying or finding the cross products. We multiply diagonally across the equal sign. In a true proportion, the cross products are equal.

    \(\dfrac{a}{b}=\dfrac{c}{d}\) → \({a}\cdot{d}={b}\cdot{c}\)
    Exercises \(\PageIndex{1}\)

    Determine whether each proportion is true or false by cross-multiplying.

    9. \(\dfrac{6}{8}=\dfrac{21}{28}\)

    10. \(\dfrac{10}{15}=\dfrac{16}{20}\)

    11. \(\dfrac{14}{4}=\dfrac{15}{5}\)

    12. \(\dfrac{0.8}{4}=\dfrac{5}{25}\)

    Answer

    9. \(168 = 168\); true

    10. \(200 \neq 240\); false

    11. \(70 \neq 60\); false

    12. \(20 = 20\); true

    As we saw in a previous module, we can use a variable to stand for a missing number. If a proportion has a missing number, we can use cross multiplication to solve for the missing number. This is as close to algebra as we get in this textbook.

    To solve a proportion for a variable:

    1. Set the cross products equal to form an equation of the form \({a}\cdot{d}={b}\cdot{c}\).
    2. Isolate the variable by rewriting the multiplication equation as a division equation.
    3. Check the solution by substituting the answer into the original proportion and finding the cross products.

    You may discover slightly different methods that you prefer.[1] If you think “Hey, can’t I do this a different way?”, you may be correct.

    Exercises \(\PageIndex{1}\)

    Solve for the variable.

    13. \(\dfrac{8}{10}=\dfrac{x}{15}\)

    14. \(\dfrac{3}{2}=\dfrac{7.5}{n}\)

    15. \(\dfrac{3}{k}=\dfrac{18}{24}\)

    16. \(\dfrac{w}{6}=\dfrac{15}{9}\)

    17. \(\dfrac{5}{4}=\dfrac{13}{x}\)

    18. \(\dfrac{3.2}{7.2}=\dfrac{m}{4.5}\) (calculator recommended)

    Answer

    13. \(x = 12\)

    14. \(n = 5\)

    15. \(k = 4\)

    16. \(w = 10\)

    17. \(x = 10.4\)

    18. \(m = 2.0\)

    Now, let's try some with more than one variable in parts of the proportion.

    Example \(\PageIndex{3}\)

    \(\dfrac{n}{n+14}=\dfrac{5}{7}.\)

        .
    Multiply both sides by the LCD.   .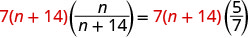
    Remove common factors on each side.   .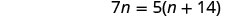
    Simplify.   .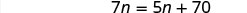
    Solve for n.   .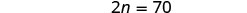
        .
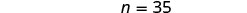    Check.    
      .  
    . .  
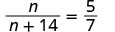    Simplify. .  
    Show common factors. .  
    Simplify. .  
    TRy it \(\PageIndex{5}\)

    \(\dfrac{y}{y+55}=\dfrac{3}{8}\).

    Answer

    33

    TRy it \(\PageIndex{6}\)

    \(\dfrac{z}{z−84}=−\dfrac{1}{5}\).

    Answer

    14

    Example \(\PageIndex{4}\)

    \(\dfrac{p+12}{9}=\dfrac{p−12}{6}\).

        .
    Multiply both sides by the LCD, 18.   .
    Simplify.   .
    Distribute.   .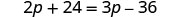
    Solve fpr p.   .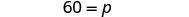
    Check.    
      .  
    . .  
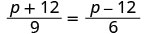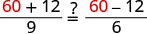    Simplify. .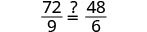  
    Divide. .  
    Try it \(\PageIndex{7}\)

    \(\dfrac{v+30}{8}=\dfrac{v+66}{12}\).

    Answer

    42

    Try it \(\PageIndex{8}\)

    \(\dfrac{2x+15}{9}=\dfrac{7x+3}{15}\).

    Answer

    6

    1. The steps in the box are designed to avoid mentioning the algebraic step of dividing both sides of the equation by a number. If you are comfortable with basic algebra, then you would phrase step 2 differently. ↵

    This page titled 5.1: Ratios, Rates, Proportions is shared under a CC BY-SA 4.0 license and was authored, remixed, and/or curated by Morgan Chase (OpenOregon) via source content that was edited to the style and standards of the LibreTexts platform; a detailed edit history is available upon request.