1.5E: Exercises
- Last updated
- Save as PDF
- Page ID
- 20547
1.5: Exponential and Logarithmic Functions
Exercise:
For the following exercises, evaluate the given exponential functions as indicated, accurate to two significant digits after the decimal.
229) \(f(x)=5^x\) a. \(x=3\) b. \(x=\frac{1}{2}\) c. \(x=\sqrt{2}\)
Solution: \(a. 125 b. 2.24 c. 9.74\)
230) \(f(x)=(0.3)^x\) a. \(x=−1\) b. \(x=4\) c. \(x=−1.5\)
231) \(f(x)=10^x\) a. \(x=−2\) b. \(x=4\) c. \(x=\frac{5}{3}\)
Solution: \(a. 0.01 b. 10,000 c. 46.42\)
232) \(f(x)=e^x\) a. \(x=2\) b. \(x=−3.2\) c. \(x=π\)
For the following exercises, match the exponential equation to the correct graph.
a. \(y=4^{−x}\)
b. \(y=3^{x−1}\)
c. \(y=2^{x+1}\)
d. \(y=(\frac{1}{2})^x+2\)
e. \(y=−3^{−x}\)
f. \(y=1−5^x\)
233)
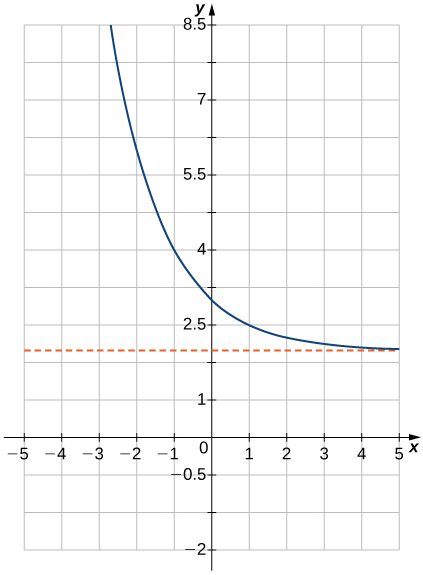
Solution: d
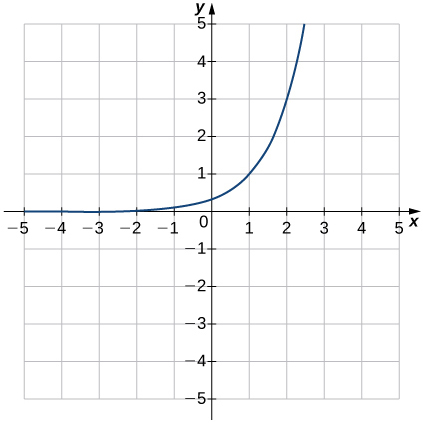
234)
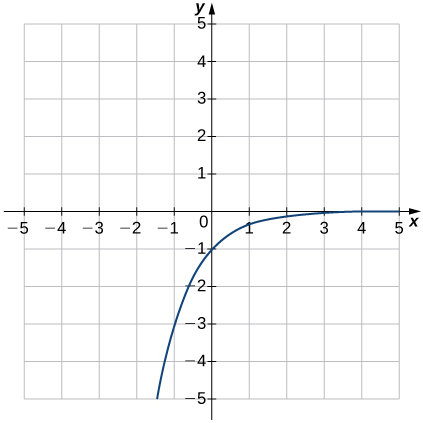
235)
Solution: b
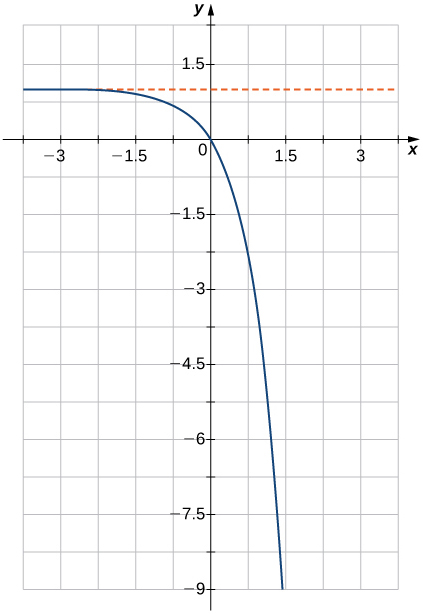
236)

237)
Solution: e
238)

For the following exercises, sketch the graph of the exponential function. Determine the domain, range, and horizontal asymptote.
239) \(f(x)=e^x+2\)

Solution: Domain: all real numbers, range: \((2,∞),y=2\)
240) \(f(x)=−2^x\)
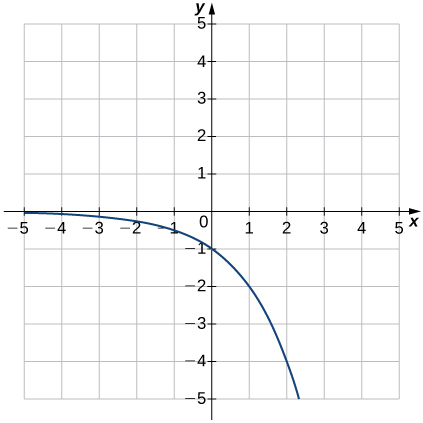
241) \(f(x)=3^{x+1}\)
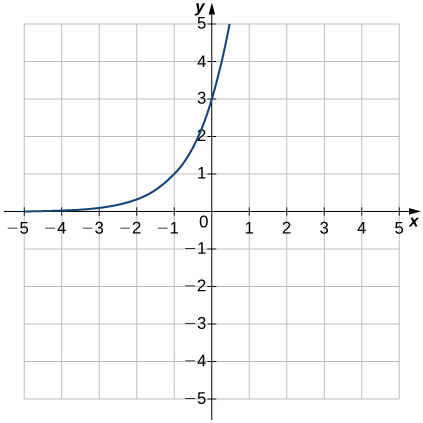
Solution: Domain: all real numbers, range: \((0,∞),y=0\)
242) \(f(x)=4^x−1\)
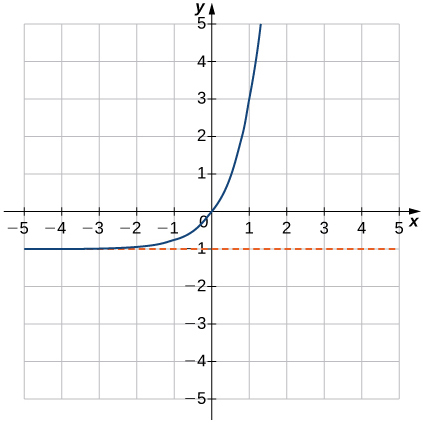
243) \(f(x)=1−2^{−x}\)

Solution: Domain: all real numbers, range: \((−∞,1),y=1\)
244) \(f(x)=5^{x+1}+2\)
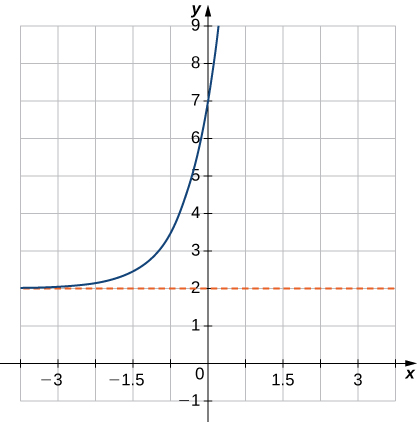
245) \(f(x)=e^{−x}−1\)

Solution: Domain: all real numbers, range: \((−1,∞),y=−1\)
For the following exercises, write the equation in equivalent exponential form.
246) \(log_381=4\)
247) \(log_82=\frac{1}{3}\)
Solution: \(8^{1/3}=2\)
248) \(log_51=0\)
249) \(log_525=2\)
Solution: \(5^2=25\)
250) \(log0.1=−1\)
251) \(ln(\frac{1}{e^3})=−3\)
Solution: \(e^{−3}=\frac{1}{e^3}\)
252) \(log_93=0.5\)
253) \(ln1=0\)
Solution: \(e^0=1\)
For the following exercises, write the equation in equivalent logarithmic form.
254) \(2^3=8\)
255) \(4^{−2}=\frac{1}{16}\)
Solution: \(log_4(\frac{1}{16})=−2\)
256) \(10^2=100\)
257) \(9^0=1\)
Solution: \(log_91=0\)
258) \((\frac{1}{3})^3=\frac{1}{27}\)
259) \(\sqrt[3]{64}=4\)
Solution: \(log_{64}4=\frac{1}{3}\)
260) \(e^x=y\)
261) \(9^y=150\)
Solution: \(log_9150=y\)
262) \(b^3=45\)
263) \(4^{-3/2}=0.125\)
Solution: \(log_40.125=−\frac{3}{2}\)
For the following exercises, sketch the graph of the logarithmic function. Determine the domain, range, and vertical asymptote.
264) \(f(x)=3+lnx\)
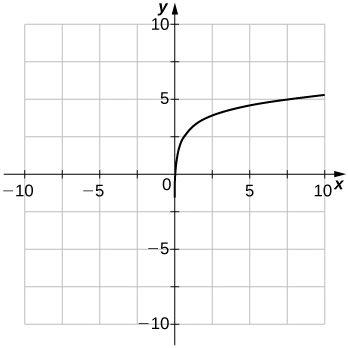
265) \(f(x)=ln(x−1)\)
Solution: Domain: \((1,∞)\), range: \((−∞,∞),x=1\)
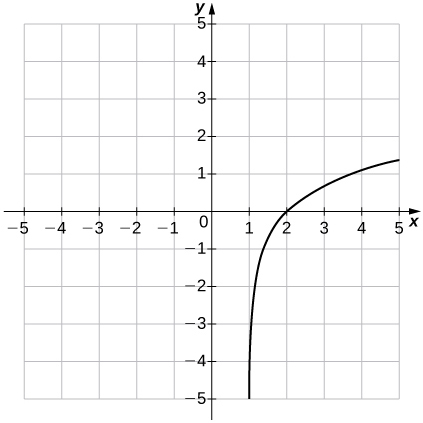
266) \(f(x)=ln(−x)\)
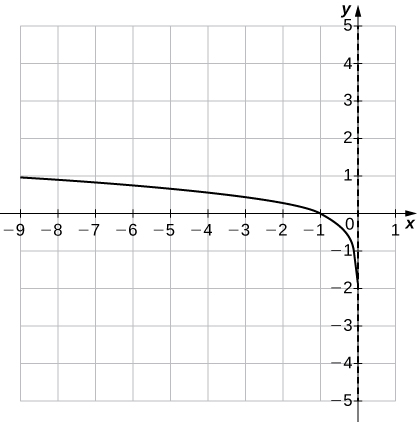
267) \(f(x)=1−lnx\)
Solution: Domain: \((0,∞)\), range: \((−∞,∞),x=0\)
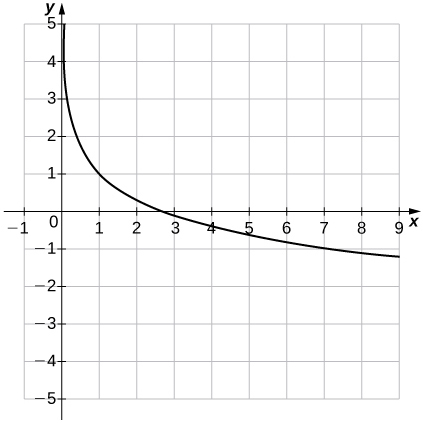
268) \(f(x)=logx−1\)

269) \(f(x)=ln(x+1)\)
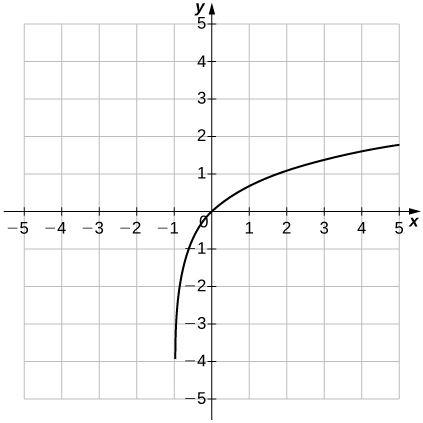
Solution: Domain: \((−1,∞)\), range: \((−∞,∞)\), \(x=−1\)
For the following exercises, use properties of logarithms to write the expressions as a sum, difference, and/or product of logarithms.
270) \(logx^4y\)
271) \(log_3\frac{9a^3}{b}\)
Solution: \(2+3log_3a−log_3b\)
272) \(lna\sqrt[3]{b}\)
273) \(log_5\sqrt{125xy^3}\)
Solution: \(\frac{3}{2}+\frac{1}{2}log_5x+\frac{3}{2}log_5y\)
274) \(log_\frac{\sqrt[3]{xy}}{64}\)
275) \(ln(\frac{6}{\sqrt{e^3}})\)
Solution: \(−\frac{3}{2}+ln6\)
For the following exercises, solve the exponential equation exactly.
276) \(5^x=125\)
277) \(e^{3x}−15=0\)
Solution: \(\frac{ln15}{3}\)
278) \(8^x=4\)
279) \(4^{x+1}−32=0\)
Solution: \(\frac{3}{2}\)
280) \(3^{x/14}=\frac{1}{10}\)
281) \(10^x=7.21\)
Solution: \(log7.21\)
282) \(4⋅2^{3x}−20=0\)
283) \(7^{3x−2}=11\)
Solution: \(\frac{2}{3}+\frac{log11}{3log7}\)
For the following exercises, solve the logarithmic equation exactly, if possible.
284) \(log_3x=0\)
285) \(log_5x=−2\)
Solution: \(x=\frac{1}{25}\)
286) \(log_4(x+5)=0\)
287) \(log(2x−7)=0\)
Solution: \(x=4\)
288) \(ln\sqrt{x+3}=2\)
289) \(log_6(x+9)+log_6x=2\)
Solution: \(x=3\)
290) \(log_4(x+2)−log_4(x−1)=0\)
291) \(lnx+ln(x−2)=ln4\)
Solution: \(1+\sqrt{5}\)
(#292 - #298 removed)
299) [T] The number of bacteria N in a culture after t days can be modeled by the function \(N(t)=1300⋅(2)^{t/4}\). Find the number of bacteria present after 15 days.
Solution: \(~17,491\)
300) [T] The demand D (in millions of barrels) for oil in an oil-rich country is given by the function \(D(p)=150⋅(2.7)^{−0.25p}\), where p is the price (in dollars) of a barrel of oil. Find the amount of oil demanded (to the nearest million barrels) when the price is between $15 and $20.
301) [T] The amount A of a $100,000 investment paying continuously and compounded for t years is given by \(A(t)=100,000⋅e^{0.055t}\). Find the amount A accumulated in 5 years.
Solution: Approximately $131,653 is accumulated in 5 years.
302) [T] An investment is compounded monthly, quarterly, or yearly and is given by the function \(A=P(1+\frac{j}{n})^{nt}\), where \(A\) is the value of the investment at time \(t\), \(P\) is the initial principle that was invested, \(j\) is the annual interest rate, and n is the number of time the interest is compounded per year. Given a yearly interest rate of 3.5% and an initial principle of $100,000, find the amount \(A\) accumulated in 5 years for interest that is compounded a. daily, b., monthly, c. quarterly, and d. yearly.
303) [T] The concentration of hydrogen ions in a substance is denoted by \([H+]\), measured in moles per liter. The pH of a substance is defined by the logarithmic function \(pH=−log[H+]\). This function is used to measure the acidity of a substance. The pH of water is 7. A substance with a pH less than 7 is an acid, whereas one that has a pH of more than 7 is a base.
a. Find the pH of the following substances. Round answers to one digit.
b. Determine whether the substance is an acid or a base.
i. Eggs: \([H+]=1.6×10^{−8}\) mol/L
ii. Beer: \([H+]=3.16×10^{−3}\) mol/L
iii. Tomato Juice: \([H+]=7.94×10^{−5}\) mol/L
Solution: i. a. pH = 8 b. Base ii. a. pH = 3 b. Acid iii. a. pH = 4 b. Acid
304) [T] Iodine-131 is a radioactive substance that decays according to the function \(Q(t)=Q_0⋅e^{−0.08664t}\), where \(Q_0\) is the initial quantity of a sample of the substance and t is in days. Determine how long it takes (to the nearest day) for 95% of a quantity to decay.
305) [T] According to the World Bank, at the end of 2013 \((t=0)\) the U.S. population was 316 million and was increasing according to the following model:
\(P(t)=316e^{0.0074t}\),
where P is measured in millions of people and t is measured in years after 2013.
a. Based on this model, what will be the population of the United States in 2020?
b. Determine when the U.S. population will be twice what it is in 2013.
Solution: a. \(~333\) million b. 94 years from 2013, or in 2107
306) [T] The amount A accumulated after 1000 dollars is invested for t years at an interest rate of 4% is modeled by the function \(A(t)=1000(1.04)^t\).
a. Find the amount accumulated after 5 years and 10 years.
b. Determine how long it takes for the original investment to triple.
307) [T] A bacterial colony grown in a lab is known to double in number in 12 hours. Suppose, initially, there are 1000 bacteria present.
a. Use the exponential function \(Q=Q_0e^{kt}\)to determine the value \(k\), which is the growth rate of the bacteria. Round to four decimal places.
b. Determine approximately how long it takes for 200,000 bacteria to grow.
Solution: a. \(k≈0.0578\) b. ≈\(92\) hours
308) [T] The rabbit population on a game reserve doubles every 6 months. Suppose there were 120 rabbits initially.
a. Use the exponential function \(P=P_0a^t\) to determine the growth rate constant \(a\). Round to four decimal places.
b. Use the function in part a. to determine approximately how long it takes for the rabbit population to reach 3500.
309) [T] The 1906 earthquake in San Francisco had a magnitude of 8.3 on the Richter scale. At the same time, in Japan, an earthquake with magnitude 4.9 caused only minor damage. Approximately how much more energy was released by the San Francisco earthquake than by the Japanese earthquake?
Solution: The San Francisco earthquake had \(10^{3.4} or ~2512\) times more energy than the Japan earthquake.
Chapter Review Exercises
True or False? Justify your answer with a proof or a counterexample.
310) A function is always one-to-one.
311) \(f∘g=g∘f\), assuming f and g are functions.
Solution: False
312) A relation that passes the horizontal and vertical line tests is a one-to-one function.
313) A relation passing the horizontal line test is a function.
Solution: False
For the following problems, state the domain and range of the given functions:
\(f=x^2+2x−3\), \(g=ln(x−5)\), \(h=\frac{1}{x+4}\)
314) h
315) g
Solution: Domain: \(x>5\), range: all real numbers
316) \(h∘f\)
317) \(g∘f\)
Solution: Domain: \(x>2\) and \(x<−4\), range: all real numbers
Find the degree, y-intercept, and zeros for the following polynomial functions.
318) \(f(x)=2x^2+9x−5\)
319) \(f(x)=x^3+2x^2−2x\)
Solution: Degree of 3, \(y\)-intercept: 0, zeros: \(0, \sqrt{3}−1,−1−\sqrt{3}\)
Simplify the following trigonometric expressions.
320) \(\frac{tan^2x}{sec^2x}+{cos^2x}\)
321) \(cos(2x)=sin^2x\)
Solution: \(cos(2x)\) or \(\frac{1}{2}(cos(2x)+1)\)
Solve the following trigonometric equations on the interval \(θ=[−2π,2π]\) exactly.
322) \(6cos2x−3=0\)
323) \(sec^2x−2secx+1=0\)
Solution: \(0,±2π\)
Solve the following logarithmic equations.
324) \(5^x=16\)
325) \log_2(x+4)=3\)
Solution: 4
Are the following functions one-to-one over their domain of existence? Does the function have an inverse? If so, find the inverse \(f^{−1}(x)\) of the function. Justify your answer.
326) \(f(x)=x^2+2x+1\)
327) \(f(x)=\frac{1}{x}\)
Solution: One-to-one; yes, the function has an inverse; inverse: \(f^{−1}(x)=\frac{1}{y}\)
For the following problems, determine the largest domain on which the function is one-to-one and find the inverse on that domain.
328) \(f(x)=\sqrt{9−x}\)
329) \(f(x)=x^2+3x+4\)
Solution: \(x≥−\frac{3}{2},f^{−1}(x)=−\frac{3}{2}+\frac{1}{2}\sqrt{4y−7}\)
330) A car is racing along a circular track with diameter of 1 mi. A trainer standing in the center of the circle marks his progress every 5 sec. After 5 sec, the trainer has to turn 55° to keep up with the car. How fast is the car traveling?
For the following problems, consider a restaurant owner who wants to sell T-shirts advertising his brand. He recalls that there is a fixed cost and variable cost, although he does not remember the values. He does know that the T-shirt printing company charges $440 for 20 shirts and $1000 for 100 shirts.
331) a. Find the equation \(C=f(x)\) that describes the total cost as a function of number of shirts and b. determine how many shirts he must sell to break even if he sells the shirts for $10 each.
Solution: a. \(C(x)=300+7x\) b. 100 shirts
332) a. Find the inverse function \(x=f^{−1}(C)\) and describe the meaning of this function. b. Determine how many shirts the owner can buy if he has $8000 to spend.
For the following problems, consider the population of Ocean City, New Jersey, which is cyclical by season.
333) The population can be modeled by \(P(t)=82.5−67.5cos[(π/6)t]\), where \(t\) is time in months (\(t=0\) represents January 1) and \(P\) is population (in thousands). During a year, in what intervals is the population less than 20,000? During what intervals is the population more than 140,000?
Solution: The population is less than 20,000 from December 8 through January 23 and more than 140,000 from May 29 through August 2
334) In reality, the overall population is most likely increasing or decreasing throughout each year. Let’s reformulate the model as \(P(t)=82.5−67.5cos[(π/6)t]+t\), where t is time in months (\(t=0\) represents January 1) and \(P\) is population (in thousands). When is the first time the population reaches 200,000?
For the following problems, consider radioactive dating. A human skeleton is found in an archeological dig. Carbon dating is implemented to determine how old the skeleton is by using the equation \(y=e^{rt}\), where \(y\) is the percentage of radiocarbon still present in the material, t is the number of years passed, and \(r=−0.0001210\) is the d78.51%ecay rate of radiocarbon.
335) If the skeleton is expected to be 2000 years old, what percentage of radiocarbon should be present?
Solution: 78.51%
336) Find the inverse of the carbon-dating equation. What does it mean? If there is 25% radiocarbon, how old is the skeleton?

