2.2E: Limits of Functions Exercises
- Last updated
- Jun 6, 2019
- Save as PDF
- Page ID
- 20560
( \newcommand{\kernel}{\mathrm{null}\,}\)
2.2: The Limit of a Function
Estimating limits from TABLES
For the following exercises, consider the function f(x)=∣x2−1∣x−1 .
30) [T] Complete the following table for the function. Round your solutions to four decimal places.
| x | f(x) | x | f(x) |
|---|---|---|---|
| 0.9 | a. | 1.1 | e. |
| 0.99 | b. | 1.01 | f. |
| 0.999 | c. | 1.001 | g. |
| 0.9999 | d. | 1.0001 | h. |
31) What do your results in the preceding exercise indicate about the two-sided limit limx→1f(x)? Explain your response.
- Answer:
-
limx→1f(x) does not exist because
limx→1−f(x)=−2 ≠ limx→1+f(x)=2.
For the following exercises, consider the function f(x)=(1+x)1/x.
32) [T] Make a table showing the values of f for x=−0.01,−0.001,−0.0001,−0.00001 and for x=0.01,0.001,0.0001,0.00001. Round your solutions to five decimal places.
| x | f(x) | \(x)\ | f(x) |
|---|---|---|---|
| -0.01 | a. | 0.01 | e. |
| -0.001 | b. | 0.001 | f. |
| -0.0001 | c. | 0.0001 | g. |
| -0.00001 | d. | 0.00001 | h. |
33) What does the table of values in the preceding exercise indicate about the function f(x)=(1+x)1/x?
- Answer:
-
It appears that: limx→0(1+x)1/x=2.7183
34) To which mathematical constant does the limit in the preceding exercise appear to be getting closer?
In the following exercises, use the given values to set up a table to evaluate the limits. Round your solutions to eight decimal places.
35) [T] limx→0sin(2x)x;±0.1,±0.01,±0.001,±.0001
| x | sin2xx | x | sin2xx |
|---|---|---|---|
| -0.1 | a. | 0.1 | e. |
| -0.01 | b. | 0.01 | f. |
| -0.001 | c. | 0.001 | g. |
| -0.0001 | d. | 0.0001 | h. |
- Answer:
-
a. 1.98669331
b. 1.99986667
c. 1.99999867
d. 1.99999999
e. 1.98669331
f. 1.99986667
g. 1.99999867
h. 1.99999999
It appears that: limx→0sin2xx=2
36) [T] limx→0sin(3x)x±0.1,±0.01,±0.001,±0.0001
| x | sin3xx | x | sin3xx |
|---|---|---|---|
| -0.1 | a. | 0.1 | e. |
| -0.01 | b. | 0.01 | f. |
| -0.001 | c. | 0.001 | g. |
| -0.0001 | d. | 0.0001 | h. |
37) Use the preceding two exercises to conjecture (guess) the value of the following limit: limx→0sin(ax)x for a, a positive real value.
- Answer:
-
It appears that: limx→0sinaxx=a
[T] In the following exercises, set up a table of values to find the indicated limit. Round to eight digits.
38) limx→2x2−4x2+x−6
| x | x2−4x2+x−6 | x | x2−4x2+x−6 |
|---|---|---|---|
| 1.9 | a. | 2.1 | e. |
| 1.99 | b. | 2.01 | f. |
| 1.999 | c. | 2.001 | g. |
| 1.9999 | d. | 2.0001 | h. |
39) limx→1(1−2x)
| x | 1−2x | x | 1−2x |
|---|---|---|---|
| 0.9 | a. | 1.1 | e. |
| 0.99 | b. | 1.01 | f. |
| 0.999 | c. | 1.001 | g. |
| 0.9999 | d. | 1.0001 | h. |
- Answer:
-
a. −0.80000000
b. −0.98000000
c. −0.99800000
d. −0.99980000
e. −1.2000000
f. −1.0200000
g. −1.0020000
h. −1.0002000;It appears that: limx→1(1−2x)=−1
40) limx→051−e1/x
| x | 51−e1/x | x | 51−e1/x |
|---|---|---|---|
| -0.1 | a. | 0.1 | e. |
| -0.01 | b. | 0.01 | f. |
| -0.001 | c. | 0.001 | g. |
| -0.0001 | d. | 0.0001 | h. |
41) limz→0z−1z2(z+3)
| z | z−1z2(z+3) | z | z−1z2(z+3) |
|---|---|---|---|
| -0.1 | a. | 0.1 | e. |
| -0.01 | b. | 0.01 | f. |
| -0.001 | c. | 0.001 | g. |
| -0.0001 | d. | 0.0001 | h. |
- Answer:
-
a. −37.931934
b. −3377.9264
c. −333,777.93
d. −33,337,778
e. −29.032258
f. −3289.0365
g. −332,889.04
h. −33,328,889It appears that: limx→0z−1z2(z+3)=−∞
42) limt→0+cos(t)t
| \(t) | costt |
|---|---|
| 0.1 | a. |
| 0.01 | b. |
| 0.001 | c. |
| 0.0001 | d. |
43) limx→21−2xx2−4
| x | 1−2xx2−4 | x | 1−2xx2−4 |
|---|---|---|---|
| 1.9 | a. | 2.1 | e. |
| 1.99 | b. | 2.01 | f. |
| 1.999 | c. | 2.001 | g. |
| 1.9999 | d. | 2.0001 | h. |
- Answer:
-
a. 0.13495277
b. 0.12594300
c. 0.12509381
d. 0.12500938
e. 0.11614402
f. 0.12406794
g. 0.12490631
h. 0.12499063It appears that: limx→21−2xx2−4=0.1250=18
[T] In the following exercises, set up a table of values and round to eight significant digits. Based on the table of values, make a guess about what the limit is. Then, use a calculator to graph the function and determine the limit. Was the conjecture correct? If not, why does the method of tables fail?
44) \displaystyle \lim_{θ \to 0}sin(\frac{π}{θ})
| θ | sin(\frac{π}{θ}) | θ | sin(\frac{π}{θ}) |
|---|---|---|---|
| -0.1 | a. | 0.1 | e. |
| -0.01 | b. | 0.01 | f. |
| -0.001 | c. | 0.001 | g. |
| -0.0001 | d. | 0.0001 | h. |
45) \displaystyle \lim_{α \to 0^+} \frac{1}{α}\cos(\frac{π}{α})
| a | \frac{1}{α}cos(\frac{π}{α}) |
|---|---|
| 0.1 | a. |
| 0.01 | b. |
| 0.001 | c. |
| 0.0001 | d. |
- Answer:
-
a. −10.00000; b. −100.00000; c. −1000.0000; d. −10,000.000
It appears from the table: \displaystyle \lim_{α→0^+}\frac{1}{α}\cos(\frac{π}{α})=-∞
actual answer (see graph): DNE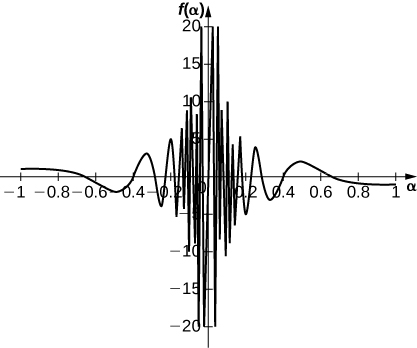
Estimating limits from GRAPHS
Note: Exercises #46 - #49 moved to section 2.4 Exercises
In the following exercises, use the following graph of the function y=f(x) to find the values, if possible. Estimate when necessary.
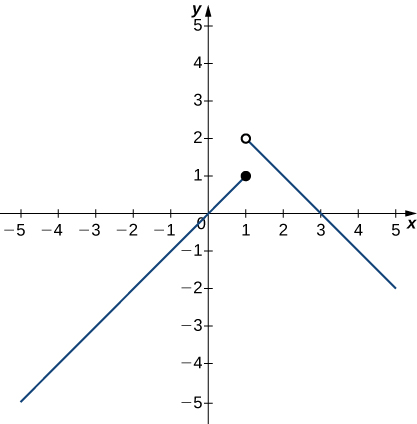
50) \displaystyle \lim_{x→1^−}f(x)
51) \displaystyle \lim_{x→1^+}f(x)
- Answer:
-
2
52) \displaystyle \lim_{x→1}f(x)
53) \displaystyle \lim_{x→2}f(x)
- Answer:
-
1
54) f(1)
In the following exercises, use the graph of the function y=f(x) shown here to find the values, if possible. Estimate when necessary.
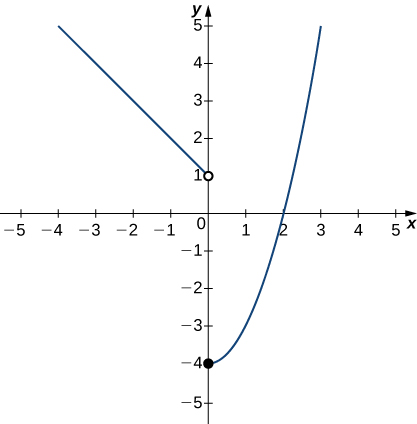
55) \displaystyle \lim_{x→0^−}f(x)
- Answer:
-
1
56) \displaystyle \lim_{x→0^+}f(x)
57) \displaystyle \lim_{x→0}f(x)
- Answer:
-
DNE
58) \displaystyle \lim_{x→2}f(x)
In the following exercises, use the graph of the function y=f(x) shown here to find the values, if possible. Estimate when necessary.
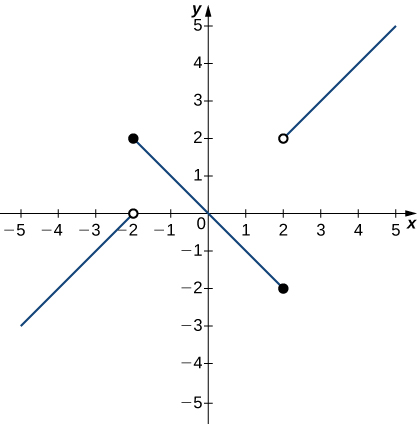
59) \displaystyle \lim_{x→−2^−}f(x)
- Answer:
-
0
60) \displaystyle \lim_{x→−2^+}f(x)
61) \displaystyle \lim_{x→−2}f(x)
- Answer:
-
DNE
62) \displaystyle \lim_{x→2^−}f(x)
63) \displaystyle \lim_{x→2^+}f(x)
- Answer:
-
2
64) \displaystyle \lim_{x→2}f(x)
In the following exercises, use the graph of the function y=g(x) shown here to find the values, if possible. Estimate when necessary.
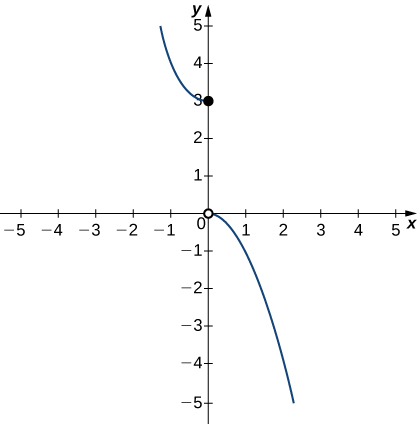
65) \displaystyle \lim_{x→0^−}g(x)
- Answer:
-
3
66) \displaystyle \lim_{x→0^+}g(x)
67) \displaystyle \lim_{x→0}g(x)
- Answer:
-
DNE
In the following exercises, use the graph of the function y=h(x) shown here to find the values, if possible. Estimate when necessary.
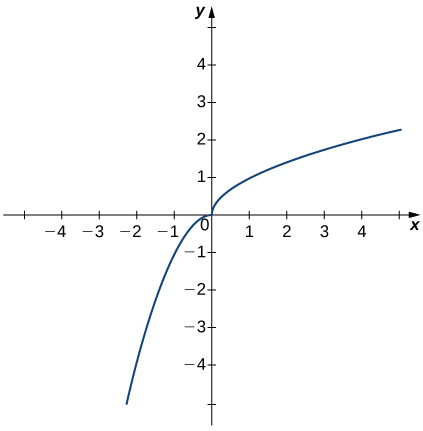
68) \displaystyle \lim_{x→0^−}h(x)
69) \displaystyle \lim_{x→0^+}h(x)
- Answer:
-
0
70) \displaystyle \lim_{x→0}h(x)
In the following exercises, use the graph of the function y=f(x) shown here to find the values, if possible. Estimate when necessary.
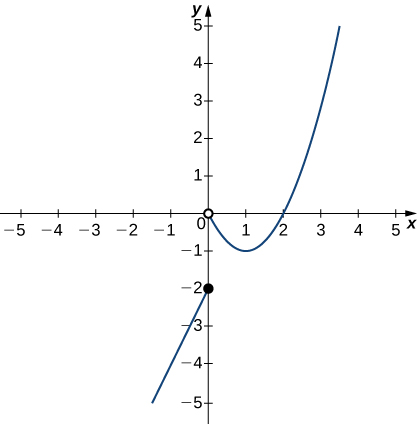
71) \displaystyle \lim_{x→0^−}f(x)
- Answer:
-
−2
72) \displaystyle \lim_{x→0^+}f(x)
73) \displaystyle \lim_{x→0}f(x)
- Answer:
-
DNE
74) \displaystyle \lim_{x→1}f(x)
75) \displaystyle \lim_{x→2}f(x)
- Answer:
-
0
In the following exercises, sketch the graph of a function with the given properties.
76) \displaystyle \lim_{x→2}f(x)=1, \displaystyle \lim_{x→4^−}f(x)=3, \displaystyle \lim_{x→4^+}f(x)=6,x=4 is not defined.
77) \displaystyle \lim_{x→−∞}f(x)=0, \displaystyle \lim_{x→−1^−}f(x)=−∞, \displaystyle \lim_{x→−1^+}f(x)=∞, \displaystyle \lim_{x→0}f(x)=f(0), f(0)=1, \displaystyle \lim_{x→∞}f(x)=−∞
- Answer:
-
Answer may vary
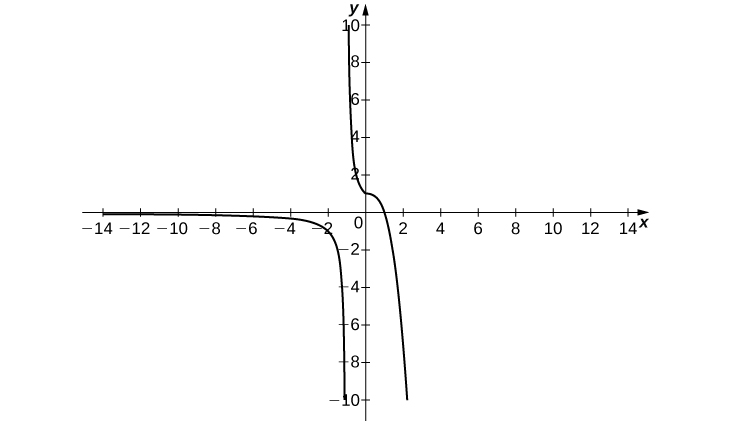
78) \displaystyle \lim_{x→−∞}f(x)=2, \displaystyle \lim_{x→3^−}f(x)=−∞, \displaystyle \lim_{x→3^+}f(x)=∞, \displaystyle \lim_{x→∞}f(x)=2,f(0)=\frac{−1}{3}
79) \displaystyle \lim_{x→−∞}f(x)=2, \displaystyle \lim_{x→−2}f(x)=−∞, \displaystyle \lim_{x→∞} f(x)=2,f(0)=0
- Answer:
-
Answer may vary
80)\displaystyle \lim_{x→−∞}f(x)=0, \displaystyle \lim_{x→−1^−}f(x)=∞, \displaystyle \lim_{x→−1^+}f(x)=−∞, f(0)=−1, \displaystyle \lim_{x→1^−}f(x)=−∞, \displaystyle \lim_{x→1^+}f(x)=∞, \displaystyle \lim_{x→∞}f(x)=0
81) Shock waves arise in many physical applications, ranging from supernovas to detonation waves. A graph of the density of a shock wave with respect to distance, x, is shown here. We are mainly interested in the location of the front of the shock, labeled xSF in the diagram.
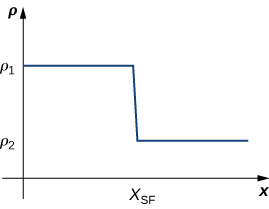
a. Evaluate \displaystyle \lim_{x→x_{SF}^+}ρ(x).
b. Evaluate \displaystyle \lim_{x→x_{SF}^−}ρ(x).
c. Evaluate \displaystyle \lim_{x→x_{SF}}ρ(x). Explain the physical meanings behind your answers.
- Answer:
-
a. ρ_2
b. ρ_1
c. DNE unless ρ_1=ρ_2. As you approach xSF from the right, you are in the high-density area of the shock. When you approach from the left, you have not experienced the “shock” yet and are at a lower density.
82) A track coach uses a camera with a fast shutter to estimate the position of a runner with respect to time. A table of the values of position of the athlete versus time is given here, where x is the position in meters of the runner and t is time in seconds. What is \displaystyle \lim_{t→2}x(t)? What does it mean physically?
| t(sec) | x(m) |
|---|---|
| 1.75 | 4.5 |
| 1.95 | 6.1 |
| 1.99 | 6.42 |
| 2.01 | 6.58 |
| 2.05 | 6.9 |
| 2.25 | 8.5 |

