1.14: The Metric System
- Page ID
- 56853
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)
You will NOT need a calculator for this module.
The metric system was first implemented following the French Revolution; if we’re overthrowing the monarchy, why should we use a unit of a “foot” that is based on the length of a king’s foot?
The metric system was designed to be based on the natural world, and different units are related to each other by powers of \(10\) instead of weird numbers like \(3\), \(12\), \(16\), and \(5,280\)… This makes converting between metric units incredibly simple.
| kilo- (k) | hecta- (h) | deka- (da) | [base unit] | deci- (d) | centi- (c) | milli- (m) |
| \(1,000\) | \(100\) | \(10\) | \(1\) | \(0.1\) | \(0.01\) | \(0.001\) |
| \(1,000\) | \(100\) | \(10\) | \(1\) | \(\dfrac{1}{10}\) | \(\dfrac{1}{100}\) | \(\dfrac{1}{1,000}\) |
| \(10^{3}\) | \(10^{2}\) | \(10^{1}\) | \(10^{0}\) | \(10^{-1}\) | \(10^{-2}\) | \(10^{-3}\) |
Notice that because deka- and deci- both start with d, the abbreviation for deka- is da.
Metric System: Measurements of Length
The base unit of length is the meter, which is a bit longer than a yard (three feet). Because the prefix kilo- means one thousand, \(1\) kilometer is \(1,000\) meters. (One kilometer is around six tenths of a mile.) Similarly, because the prefix centi- means one hundredth, \(1\) centimeter is \(\dfrac{1}{100}\) of a meter, or \(1\) meter is \(100\) centimeters. (One centimeter is roughly the thickness of a pen.) And because the prefix milli- means one thousandth, \(1\) millimeter is \(\dfrac{1}{1,000}\) of a meter, or \(1\) meter is \(1,000\) millimeters. (One millimeter is roughly the thickness of a credit card.)
From each of the four choices, choose the most reasonable measure.
1. The length of a car:
\(5\) kilometers, \(5\) meters, \(5\) centimeters, \(5\) millimeters
2. The height of a notebook:
\(28\) kilometers, \(28\) meters, \(28\) centimeters, \(28\) millimeters
3. The distance to the next town:
\(3.8\) kilometers, \(3.8\) meters, \(3.8\) centimeters, \(3.8\) millimeters
4. An adult woman’s height:
\(1.6\) kilometers, \(1.6\) meters, \(1.6\) centimeters, \(1.6\) millimeters
5. An adult woman’s height:
\(160\) kilometers, \(160\) meters, \(160\) centimeters, \(160\) millimeters
6. The thickness of a pane of glass:
\(3\) kilometers, \(3\) meters, \(3\) centimeters, \(3\) millimeters
- Answer
-
1. \(5\) m
2. \(28\) cm
3. \(3.8\) km
4. \(1.6\) m
5. \(160\) cm
6. \(3\) mm
| kilo- (km) | hecta- (hm) | deka- (dam) | meter (m) | deci- (dm) | centi- (cm) | milli- (mm) |
| \(1,000\) | \(100\) | \(10\) | \(1\) | \(0.1\) | \(0.01\) | \(0.001\) |
| \(1,000\) | \(100\) | \(10\) | \(1\) | \(\dfrac{1}{10}\) | \(\dfrac{1}{100}\) | \(\dfrac{1}{1,000}\) |
| \(10^{3}\) | \(10^{2}\) | \(10^{1}\) | \(10^{0}\) | \(10^{-1}\) | \(10^{-2}\) | \(10^{-3}\) |
To convert metric units, you can simply move the decimal point left or right the number of places indicated in the table above. No calculator required!
7. Convert \(3.7\) meters to centimeters.
8. Convert \(3.7\) meters to millimeters.
9. Convert \(2.45\) kilometers to meters.
10. Convert \(2.45\) kilometers to centimeters.
11. Convert \(342\) millimeters to meters.
12. Convert \(342\) millimeters to centimeters.
13. Convert \(528\) meters to kilometers.
14. Convert \(45\) centimeters to meters.
- Answer
-
7. \(370\) cm
8. \(3,700\) mm
9. \(2,450\) m
10. \(245,000\) cm
11. \(0.342\) m
12. \(34.2\) cm
13. \(0.528\) km
14. \(0.45\) m
Metric System: Measurements of Weight or Mass
The base unit for mass is the gram, which is approximately the mass of a paper clip. A kilogram is \(1,000\) grams; as we’ll see in the next module, this is around \(2.2\) pounds. The active ingredients in medicines may be measured using the milligram, or possibly the microgram, which we will come back to in a future module. For now, we will focus on the prefixes between kilo- and milli-.
From each of the three choices, choose the most reasonable measure.
15. The mass of an apple:
\(100\) kilograms, \(100\) grams, \(100\) milligrams
16. The mass of an adult man:
\(80\) kilograms, \(80\) grams, \(80\) milligrams
17. The amount of active ingredient in a pain relief pill:
\(500\) kilograms, \(500\) grams, \(500\) milligrams
18. The base vehicle weight of a GMC Sierra 1500:
\(2,000\) kilograms, \(2,000\) grams, \(2,000\) milligrams
- Answer
-
15. \(100\) g
16. \(80\) kg
17. \(500\) mg
18. \(2,000\) kg
| kilo- (kg) | hecta- (hg) | deka- (dag) | gram (g) | deci- (dg) | centi- (cg) | milli- (mg) |
| \(1,000\) | \(100\) | \(10\) | \(1\) | \(0.1\) | \(0.01\) | \(0.001\) |
| \(1,000\) | \(100\) | \(10\) | \(1\) | \(\dfrac{1}{10}\) | \(\dfrac{1}{100}\) | \(\dfrac{1}{1,000}\) |
| \(10^{3}\) | \(10^{2}\) | \(10^{1}\) | \(10^{0}\) | \(10^{-1}\) | \(10^{-2}\) | \(10^{-3}\) |
This table is identical to the previous table; the only difference is that the base unit “meter” has been replaced by “gram”. This means that converting metric units of mass is exactly the same process as converting metric units of length; just move the decimal point!
19. Convert \(0.813\) grams to centigrams.
20. Convert \(0.813\) grams to milligrams.
21. Convert \(1.25\) kilograms to grams.
22. Convert \(1.25\) kilograms to milligrams.
23. Convert \(960\) milligrams to grams.
24. Convert \(960\) milligrams to centigrams.
25. Convert \(1,350\) grams to dekagrams.
26. Convert \(7.5\) centigrams to grams.
- Answer
-
19. \(81.3\) cg
20. \(813\) g
21. \(1,250\) g
22. \(1,250,000\) mg
23. \(0.96\) g
24. \(96\) cg
25. \(135\) dag
26. \(0.075\) g
Metric System: Measurements of Volume or Capacity
The base unit of volume is the liter, which is slightly larger than one quart. The milliliter is also commonly used; of course, there are \(1,000\) milliliters in \(1\) liter.
In case you were wondering, the units of volume, length, and mass are all connected; one cubic centimeter (a cube with each side equal to \(1\) cm) has the same volume as one milliliter, and one milliliter of water has a mass of one gram.
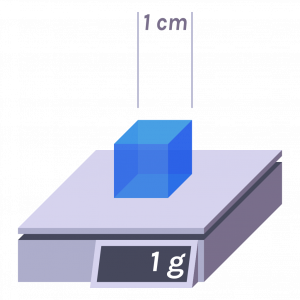
From each of the two choices, choose the more reasonable measure.
27. The capacity of a car’s gas tank: \(50\) liters, \(50\) milliliters
28. A dosage of liquid cough medicine: \(30\) liters, \(30\) milliliters
- Answer
-
27. \(50\) L
28. \(30\) mL
| kilo- (kL) | hecta- (hL) | deka- (daL) | liter (L) | deci- (dL) | centi- (cL) | milli- (mL) |
| \(1,000\) | \(100\) | \(10\) | \(1\) | \(0.1\) | \(0.01\) | \(0.001\) |
| \(1,000\) | \(100\) | \(10\) | \(1\) | \(\dfrac{1}{10}\) | \(\dfrac{1}{100}\) | \(\dfrac{1}{1,000}\) |
| \(10^{3}\) | \(10^{2}\) | \(10^{1}\) | \(10^{0}\) | \(10^{-1}\) | \(10^{-2}\) | \(10^{-3}\) |
Again, this table is identical to the previous tables; just move the decimal point left or right to convert the units.
29. Convert \(2.8\) liters to milliliters.
30. Convert \(2.8\) liters to deciliters.
31. Convert \(15\) dekaliters to liters.
32. Convert \(0.75\) deciliters to milliliters.
33. Convert \(600\) milliliters to centiliters.
34. Convert \(600\) milliliters to liters.
35. Convert \(4.5\) deciliters to liters.
36. Convert \(550\) centiliters to liters.
37. Flying on IcelandAir, you happen to notice that one mini bottle of booze is labeled \(50\text{ mL}\), but another mini bottle is labeled \(5\text{ cL}\). How do the two bottles compare in size?
38. How many \(500\)-milliliter bottles of Coke[1] are equivalent to one \(2\)-liter bottle?
39. The engine displacement of a Yamaha Majesty scooter is \(125\) cc (cubic centimeters), and the engine displacement of a Chevrolet Spark automobile is \(1.4\) L (liters). What is the approximate ratio of these engine displacements?
- Answer
-
29. \(2,800\) mL
30. \(28\) dL
31. \(150\) L
32. \(75\) mL
33. \(60\) cL
34. \(0.6\) L
35. \(0.45\) L
36. \(5.5\) L
37. they are equal in size
38. \(4\) bottles
39. about \(11\) to \(1\)
- (Fun fact: in Spanish, a \(500\)-milliliter bottle is called a medio litro.) ↵


