4.2.2: Case n=2
( \newcommand{\kernel}{\mathrm{null}\,}\)
Consider the initial value problem
vxx+vyy=c−2vttv(x,y,0)=f(x,y)vt(x,y,0)=g(x,y),
where f∈C3, g∈C2.
Using the formula for the solution of the three-dimensional initial value problem we will derive a formula for the two-dimensional case. The following consideration is called Hadamard's method of decent.
Let v(x,y,t) be a solution of (4.2.2.1)-(4.2.2.3), then
$$u(x,y,z,t):=v(x,y,t)\]
is a solution of the three-dimensional initial value problem with initial data f(x,y), g(x,y), independent of z, since u satisfies (4.2.2.1)-(4.2.2.3). Hence, since u(x,y,z,t)=u(x,y,0,t)+uz(x,y,δz,t)z, 0<δ<1, and uz=0, we have
$$v(x,y,t)=u(x,y,0,t).\]
Poisson's formula in the three-dimensional case implies
v(x,y,t)=14πc2∂∂t(1t∫∂Bct(x,y,0) f(ξ,η) dS)+14πc2t∫∂Bct(x,y,0) g(ξ,η) dS.
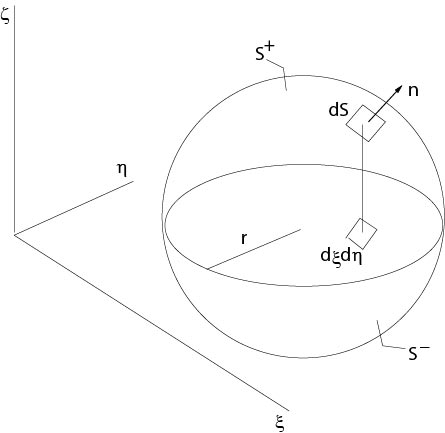
Figure 4.2.2.1: Domains of integration
The integrands are independent on ζ. The surface S is defined by χ(ξ,η,ζ):=(ξ−x)2+(η−y)2+ζ2−c2t2=0. Then the exterior normal n at S is n=∇χ/|∇χ| and the surface element is given by dS=(1/|n3|)dξdη, where the third coordinate of n is
$$n_3=\pm\frac{\sqrt{c^2 t^2-(\xi-x)^2-(\eta-y)^2}}{ct}.\]
The positive sign applies on S+, where ζ>0 and the sign is negative on S− where ζ<0, see Figure 4.2.2.1. We have S=S+∪¯S−.
Set ρ=√(ξ−x)2+(η−y)2. Then it follows from (4.2.2.4)
Theorem 4.3. The solution of the Cauchy initial value problem (4.2.2.1)-(4.2.2.3) is given by
v(x,y,t)=12πc∂∂t∫Bct(x,y) f(ξ,η)√c2t2−ρ2 dξdη+12πc∫Bct(x,y) g(ξ,η)√c2t2−ρ2 dξdη.
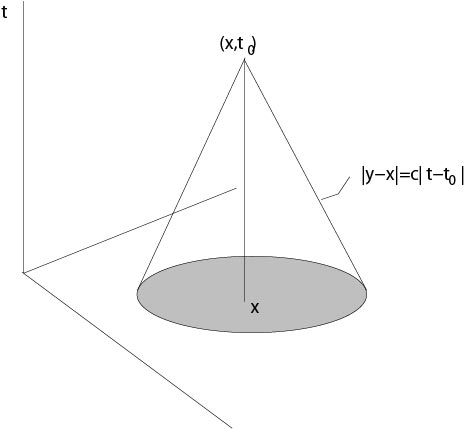
Figure 4.2.2.2: Interval of dependence, case n=2
Corollary. In contrast to the three dimensional case, the domain of dependence is here the disk Bcto(x0,y0) and not the boundary only. Therefore, see formula of Theorem 4.3, if f, g have supports in a compact domain D⊂R2, then these functions have influence on the value v(x,y,t) for all time t>T, T sufficiently large.
Contributors and Attributions
Integrated by Justin Marshall.


