Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

1.7: Decimal Fractions

Last updated

Jun 4, 2023
Save as PDF
- 1.6: Operations with Fractions
- 1.8: Percent

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Overview

Decimal Fractions
Adding and Subtracting Decimal Fractions
Multiplying Decimal Fractions
Dividing Decimal Fractions
Converting Decimal Fractions to Fractions
Converting Fractions to Decimal Fractions

Decimal Fractions

Fractions are one way we can represent parts of whole numbers. Decimal fractions are another way of representing parts of whole numbers.

Decimal Fractions

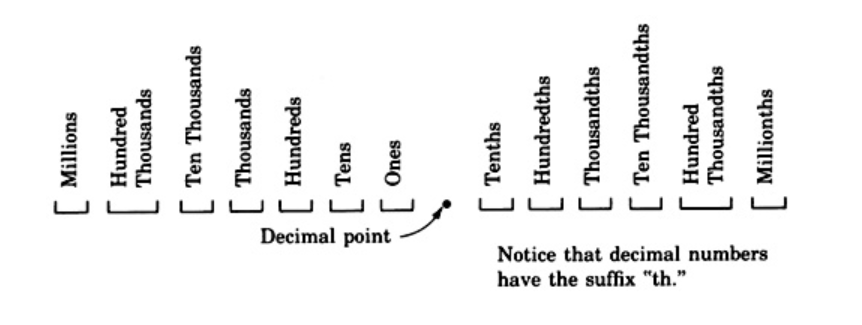
A decimal fraction is a fraction in which the denominator is a power of 10.

A decimal fraction uses a decimal point to separate whole parts and fractional parts. Whole parts are written to the left of the decimal point and fractional parts are written to the right of the decimal point. Just as each digit in a whole number has a particular value, so do the digits in decimal positions.

$clipboard_ea88b986766aeb75f89f9bd0ff13a57fa.png$

Sample Set A

The following numbers are decimal fractions

Example $\PageIndex{1}$

57.9

The 9 is in the tenths position. 57.9 = $10\dfrac{9}{10}$ .

Example $\PageIndex{2}$

6.8014

The 8 is in the tenths position.

The 0 is in the hundredths position.

The 1 is in the thousandths position.

The 4 is in the ten thousandths position.

6.8014 = $6\dfrac{8014}{10000}$ .

Adding and Subtracting Decimal Fractions

Adding/Subtracting Decimal Fractions:

To add or subtract decimal fractions,

Align the numbers vertically so that the decimal points line up under each other and corresponding decimal positions are in the same column. Add zeros if necessary.
Add or subtract the numbers as if they were whole numbers.
Place a decimal point in the resulting sum or difference directly under the other decimal points.

Sample Set B

Find each sum or difference

Example $\PageIndex{3}$

$clipboard_eb8f3187bed3dae27998d45d92fb02733.png$

Example $\PageIndex{4}$

$clipboard_e347c7001e47b6cc97bc4ef4184aad64f.png$

Example $\PageIndex{5}$

$clipboard_e78931fdcd0ab185080223fb89a946e6e.png$

Multiplying Decimal Fractions

Multiplying Decimal Fractions

To multiply decimals,

Multiply the numbers as if they were whole numbers.
Find the sum of the number of decimal places in the factors.
The number of decimal places in the product is the sum found in step 2.

Sample Set C

Find the following products

Example $\PageIndex{6}$

$clipboard_e9a2205a67b0ff9b184b9e94b27f5300c.png$

Example $\PageIndex{7}$

$clipboard_e7fe21e750898d21ed9851636a9a8e0c1.png$

Dividing Decimal Fractions

Dividing Decimal Fractions

To divide a decimal by a nonzero decimal,

Convert the divisor to a whole number by moving the decimal point to the position immediately to the right of the divisor’s last digit.
Move the decimal point of the dividend to the right the same number of digits it was moved in the divisor.
Set the decimal point in the quotient by placing a decimal point directly above the decimal point in the dividend.
Divide as usual.

Sample Set D

Find the following quotients

Example $\PageIndex{8}$

$clipboard_ec5f32196849d2f0f6febf901572c5361.png$

Example $\PageIndex{9}$

$clipboard_e99cc7a86f383544f60a07a618f0dc751.png$

Example $\PageIndex{10}$

$clipboard_eddecf73ac4c0ceee99b1eed28da9da56.png$

Converting Decimal Fractions to Fractions

We can convert a decimal fraction to a fraction by reading it and then writing the phrase we have just read. As we read the decimal fraction, we note the place value farthest to the right. We may have to reduce the fraction.

Sample Set E

Convert each decimal fraction to a fraction

Example $\PageIndex{11}$

$clipboard_ee771b2283db4f531418ef8c383937923.png$

Example $\PageIndex{12}$

$clipboard_ea884cbe3facd91eae60ea788abe93444.png$

Converting Fractions to Decimal Fractions

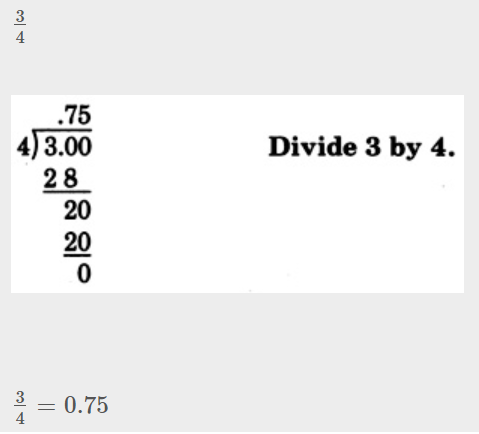
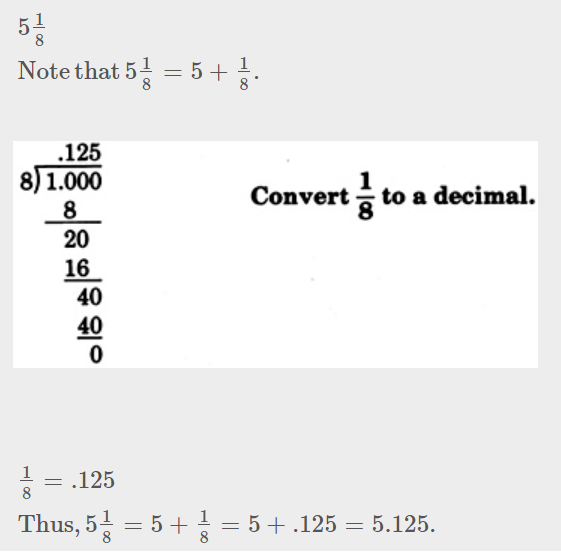
Sample Set F

Convert the following fractions to decimals. If the division is nonterminating, round to 2 decimal places.

Example $\PageIndex{13}$

$clipboard_ef7a4e04a8fa9406d8f6ff9e0bb74ca7d.png$

Example $\PageIndex{14}$

$clipboard_e9f18a5e71406773c7600d66bfb1bca1e.png$

Example $\PageIndex{15}$

$clipboard_e22125b2b49cab4acb3a0cbebed1e39e1.png$

Example $\PageIndex{16}$

$clipboard_ea42278a4fe104364bee6a2d0c91d0e00.png$

Example $\PageIndex{17}$

$clipboard_ecb66dcc55e4885d631a5c0d6cdf38144.png$

Exercises

For the following problems, perform each indicated operation.

Exercise $\PageIndex{1}$

1.84 + 7.11

Answer: 8.95

Exercise $\PageIndex{2}$

15.015 - 6.527

Exercise $\PageIndex{3}$

4.904 - 2.67

Answer: 2.234

Exercise $\PageIndex{4}$

156.33 − 24.095

Exercise $\PageIndex{5}$

.0012 + 1.53 + 5.1

Answer: 6.6312

Exercise $\PageIndex{6}$

44.98 + 22.8 − 12.76

Exercise $\PageIndex{7}$

5.0004 − 3.00004 + 1.6837

Answer: 3.68406

Exercise $\PageIndex{8}$

1.11 + 12.1212 − 13.131313

Exercise $\PageIndex{9}$

4.26 ⋅ 3.2

Answer: 13.632

Exercise $\PageIndex{10}$

2.97 ⋅ 3.15

Exercise $\PageIndex{11}$

23.05 ⋅ 1.1

Answer: 25.355

Exercise $\PageIndex{12}$

5.009 ⋅ 2.106

Exercise $\PageIndex{13}$

0.1 ⋅ 3.24

Answer: 0.324

Exercise $\PageIndex{14}$

100 ⋅ 12.008

Exercise $\PageIndex{15}$

1000 ⋅ 12.008

Answer: 12,008

Exercise $\PageIndex{16}$

10,000 ⋅ 12.008

Exercise $\PageIndex{17}$

75.642 ÷ 18.01

Answer: 4.2

Exercise $\PageIndex{18}$

51.811 ÷ 1.97

Exercise $\PageIndex{19}$

0.0000448 ÷ 0.014

Answer: 0.0032

Exercise $\PageIndex{20}$

0.129516 ÷ 1004

For the following problems, convert each decimal fraction to a fraction.

Exercise $\PageIndex{21}$

0.06

Answer: $\dfrac{3}{50}$

Exercise $\PageIndex{22}$

0.115

Exercise $\PageIndex{23}$

3.7

Answer: $3\dfrac{7}{10}$

Exercise $\PageIndex{24}$

48.1162

Exercise $\PageIndex{25}$

712.00004

Answer: $712\dfrac{1}{25000}$

For the following problems, convert each fraction to a decimal fraction. If the decimal form is nonterminating,round to 3 decimal places.

Exercise $\PageIndex{26}$

$\dfrac{5}{8}$

Exercise $\PageIndex{27}$

$\dfrac{9}{20}$

Answer: 0.45

Exercise $\PageIndex{28$

15 ÷ 22

Exercise $\PageIndex{29}$

$\dfrac{7}{11}$

Answer: 0.636

Exercise $\PageIndex{30}$

$\dfrac{2}{9}$

Overview

Decimal Fractions

Decimal Fractions

Sample Set A

Example 1.7.1\PageIndex{1}

Example 1.7.2\PageIndex{2}

Adding and Subtracting Decimal Fractions

Sample Set B

Example 1.7.3\PageIndex{3}

Example 1.7.4\PageIndex{4}

Example 1.7.5\PageIndex{5}

Multiplying Decimal Fractions

Sample Set C

Example 1.7.6\PageIndex{6}

Example 1.7.7\PageIndex{7}

Dividing Decimal Fractions

Sample Set D

Example 1.7.8\PageIndex{8}

Example 1.7.9\PageIndex{9}

Example 1.7.10\PageIndex{10}

Converting Decimal Fractions to Fractions

Sample Set E

Example 1.7.11\PageIndex{11}

Example 1.7.12\PageIndex{12}

Converting Fractions to Decimal Fractions

Sample Set F

Example 1.7.13\PageIndex{13}

Example 1.7.14\PageIndex{14}

Example 1.7.15\PageIndex{15}

Example 1.7.16\PageIndex{16}

Example 1.7.17\PageIndex{17}

Exercises

Exercise 1.7.1\PageIndex{1}

Exercise 1.7.2\PageIndex{2}

Exercise 1.7.3\PageIndex{3}

Exercise 1.7.4\PageIndex{4}

Exercise 1.7.5\PageIndex{5}

Exercise 1.7.6\PageIndex{6}

Exercise 1.7.7\PageIndex{7}

Exercise 1.7.8\PageIndex{8}

Exercise 1.7.9\PageIndex{9}

Exercise 1.7.10\PageIndex{10}

Exercise 1.7.11\PageIndex{11}

Exercise 1.7.12\PageIndex{12}

Exercise 1.7.13\PageIndex{13}

Exercise 1.7.14\PageIndex{14}

Exercise 1.7.15\PageIndex{15}

Exercise 1.7.16\PageIndex{16}

Exercise 1.7.17\PageIndex{17}

Exercise 1.7.18\PageIndex{18}

Exercise 1.7.19\PageIndex{19}

Exercise 1.7.20\PageIndex{20}

Exercise 1.7.21\PageIndex{21}

Exercise 1.7.22\PageIndex{22}

Exercise 1.7.23\PageIndex{23}

Exercise 1.7.24\PageIndex{24}

Exercise 1.7.25\PageIndex{25}

Exercise 1.7.26\PageIndex{26}

Exercise 1.7.27\PageIndex{27}

Exercise \(\PageIndex{28\)

Exercise 1.7.29\PageIndex{29}

Exercise 1.7.30\PageIndex{30}

Support Center

How can we help?

Example $\PageIndex{1}$

Example $\PageIndex{2}$

Example $\PageIndex{3}$

Example $\PageIndex{4}$

Example $\PageIndex{5}$

Example $\PageIndex{6}$

Example $\PageIndex{7}$

Example $\PageIndex{8}$

Example $\PageIndex{9}$

Example $\PageIndex{10}$

Example $\PageIndex{11}$

Example $\PageIndex{12}$

Example $\PageIndex{13}$

Example $\PageIndex{14}$

Example $\PageIndex{15}$

Example $\PageIndex{16}$

Example $\PageIndex{17}$

Exercise $\PageIndex{1}$

Exercise $\PageIndex{2}$

Exercise $\PageIndex{3}$

Exercise $\PageIndex{4}$

Exercise $\PageIndex{5}$

Exercise $\PageIndex{6}$

Exercise $\PageIndex{7}$

Exercise $\PageIndex{8}$

Exercise $\PageIndex{9}$

Exercise $\PageIndex{10}$

Exercise $\PageIndex{11}$

Exercise $\PageIndex{12}$

Exercise $\PageIndex{13}$

Exercise $\PageIndex{14}$

Exercise $\PageIndex{15}$

Exercise $\PageIndex{16}$

Exercise $\PageIndex{17}$

Exercise $\PageIndex{18}$

Exercise $\PageIndex{19}$

Exercise $\PageIndex{20}$

Exercise $\PageIndex{21}$

Exercise $\PageIndex{22}$

Exercise $\PageIndex{23}$

Exercise $\PageIndex{24}$

Exercise $\PageIndex{25}$

Exercise $\PageIndex{26}$

Exercise $\PageIndex{27}$

Exercise $\PageIndex{29}$

Exercise $\PageIndex{30}$