2.4E: Exercises
( \newcommand{\kernel}{\mathrm{null}\,}\)
Practice Makes Perfect
Solve Equations Using the General Strategy for Solving Linear Equations
In the following exercises, solve each linear equation.
15(y−9)=−60
21(y−5)=−42
- Answer
-
y=3
−9(2n+1)=36
−16(3n+4)=32
- Answer
-
n=−2
8(22+11r)=0
5(8+6p)=0
- Answer
-
p=−43
−(w−12)=30
−(t−19)=28
- Answer
-
t=−9
9(6a+8)+9=81
8(9b−4)−12=100
- Answer
-
b=2
32+3(z+4)=41
21+2(m−4)=25
- Answer
-
m=6
51+5(4−q)=56
−6+6(5−k)=15
- Answer
-
k=32
2(9s−6)−62=16
8(6t−5)−35=−27
- Answer
-
t=1
3(10−2x)+54=0
−2(11−7x)+54=4
- Answer
-
x=−2
23(9c−3)=22
35(10x−5)=27
- Answer
-
x=5
15(15c+10)=c+7
14(20d+12)=d+7
- Answer
-
d=1
18−(9r+7)=−16
15−(3r+8)=28
- Answer
-
r=−7
5−(n−1)=19
−3−(m−1)=13
- Answer
-
m=−15
11−4(y−8)=43
18−2(y−3)=32
- Answer
-
y=−4
24−8(3v+6)=0
35−5(2w+8)=−10
- Answer
-
w=12
4(a−12)=3(a+5)
−2(a−6)=4(a−3)
- Answer
-
a=4
2(5−u)=−3(2u+6)
5(8−r)=−2(2r−16)
- Answer
-
r=8
3(4n−1)−2=8n+3
9(2m−3)−8=4m+7
- Answer
-
m=3
12+2(5−3y)=−9(y−1)−2
−15+4(2−5y)=−7(y−4)+4
- Answer
-
y=−3
8(x−4)−7x=14
5(x−4)−4x=14
- Answer
-
x=34
5+6(3s−5)=−3+2(8s−1)
−12+8(x−5)=−4+3(5x−2)
- Answer
-
x=−6
4(u−1)−8=6(3u−2)−7
7(2n−5)=8(4n−1)−9
- Answer
-
n=−1
4(p−4)−(p+7)=5(p−3)
3(a−2)−(a+6)=4(a−1)
- Answer
-
a=−4
−(9y+5)−(3y−7)=16−(4y−2)
−(7m+4)−(2m−5)=14−(5m−3)
- Answer
-
m=−4
4[5−8(4c−3)]=12(1−13c)−8
5[9−2(6d−1)]=11(4−10d)−139
- Answer
-
d=−3
3[−9+8(4h−3)]=2(5−12h)−19
3[−14+2(15k−6)]=8(3−5k)−24
- Answer
-
k=35
5[2(m+4)+8(m−7)]=2[3(5+m)−(21−3m)]
10[5(n+1)+4(n−1)]=11[7(5+n)−(25−3n)]
- Answer
-
n=−5
5(1.2u−4.8)=−12
4(2.5v−0.6)=7.6
- Answer
-
v=1
0.25(q−6)=0.1(q+18)
0.2(p−6)=0.4(p+14)
- Answer
-
p=−34
0.2(30n+50)=28
0.5(16m+34)=−15
- Answer
-
m=−4
Classify Equations
In the following exercises, classify each equation as a conditional equation, an identity, or a contradiction and then state the solution.
23z+19=3(5z−9)+8z+46
15y+32=2(10y−7)−5y+46
- Answer
-
identity; all real numbers
5(b−9)+4(3b+9)=6(4b−5)−7b+21
9(a−4)+3(2a+5)=7(3a−4)−6a+7
- Answer
-
identity; all real numbers
18(5j−1)+29=47
24(3d−4)+100=52
- Answer
-
conditional equation; d=23
22(3m−4)=8(2m+9)
30(2n−1)=5(10n+8)
- Answer
-
conditional equation; n=7
7v+42=11(3v+8)−2(13v−1)
18u−51=9(4u+5)−6(3u−10)
- Answer
-
contradiction; no solution
3(6q−9)+7(q+4)=5(6q+8)−5(q+1)
5(p+4)+8(2p−1)=9(3p−5)−6(p−2)
- Answer
-
contradiction; no solution
12(6h−1)=8(8h+5)−4
9(4k−7)=11(3k+1)+4
- Answer
-
conditional equation; k=26
45(3y−2)=9(15y−6)
60(2x−1)=15(8x+5)
- Answer
-
contradiction; no solution
16(6n+15)=48(2n+5)
36(4m+5)=12(12m+15)
- Answer
-
identity; all real numbers
9(14d+9)+4d=13(10d+6)+3
11(8c+5)−8c=2(40c+25)+5
- Answer
-
identity; all real numbers
Everyday Math
Fencing Micah has 44 feet of fencing to make a dog run in his yard. He wants the length to be 2.5 feet more than the width. Find the length, L, by solving the equation 2L+2(L−2.5)=44.
Coins Rhonda has $1.90 in nickels and dimes. The number of dimes is one less than twice the number of nickels. Find the
number of nickels, n, by solving the equation 0.05n+0.10(2n−1)=1.90.
- Answer
-
8 nickels
Writing Exercises
Using your own words, list the steps in the general strategy for solving linear equations.
Explain why you should simplify both sides of an equation as much as possible before collecting the variable terms to one side and the constant terms to the other side.
- Answer
-
Answers will vary.
What is the first step you take when solving the equation 3−7(y−4)=38? Why is this your first step?
Solve the equation 14(8x+20)=3x−4 explaining all the steps of your solution as in the examples in this section.
- Answer
-
Answers will vary.
Self Check
ⓐ After completing the exercises, use this checklist to evaluate your mastery of the objective of this section.
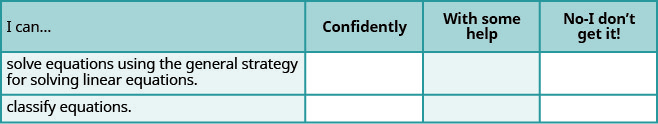
ⓑ On a scale of 1-10, how would you rate your mastery of this section in light of your responses on the checklist? How can you improve this?
Glossary
- conditional equation
- An equation that is true for one or more values of the variable and false for all other values of the variable is a conditional equation.
- contradiction
- An equation that is false for all values of the variable is called a contradiction. A contradiction has no solution.
- identity
- An equation that is true for any value of the variable is called an identity. The solution of an identity is all real numbers.


