10.5E: Exercises

Last updated
Save as PDF

Page ID: 30287

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Practice Makes Perfect

Recognize the Graph of a Quadratic Equation in Two Variables

In the following exercises, graph:

Example $\PageIndex{37}$

$y=x^2+3$

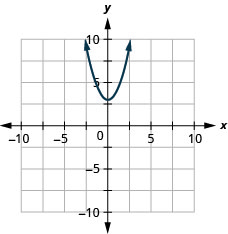
Answer: $This figure shows an upward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 10. The y-axis of the plane runs from -10 to 10. The parabola has a vertex at (0, 3) and goes through the point (1, 4).$

Example $\PageIndex{38}$:

$y=−x^2+1$

In the following exercises, determine if the parabola opens up or down.

Example $\PageIndex{39}$

$y=−2x^2−6x−7$

Answer: down

Example $\PageIndex{40}$:

$y=6x^2+2x+3$

Example $\PageIndex{41}$

y=4x^2+x−4

Answer: up

Example $\PageIndex{42}$:

$y=−9x^2−24x−16$

Find the Axis of Symmetry and Vertex of a Parabola

In the following exercises, find ⓐ the axis of symmetry and ⓑ the vertex.

Example $\PageIndex{43}$

$y=x^2+8x−1$

Answer: ⓐ x=−4 ⓑ (−4,−17)

Example $\PageIndex{44}$:

$y=x^2+10x+25$

Example $\PageIndex{45}$

$y=−x^2+2x+5$

Answer: ⓐ x=1 ⓑ (1,6)

Example $\PageIndex{46}$:

$y=−2x^2−8x−3$

Find the Intercepts of a Parabola

In the following exercises, find the x- and y-intercepts.

Example $\PageIndex{47}$

$y=x^2+7x+6$

Answer: y:(0,6); x:(−1,0),(−6,0)

Example $\PageIndex{48}$:

$y=x^2+10x−11$

Example $\PageIndex{49}$

$y=−x^2+8x−19$

Answer: y:(0,−19); x:none

Example $\PageIndex{50}$:

$y=x^2+6x+13$

Example $\PageIndex{51}$

$y=4x^2−20x+25$

Answer: y:(0,25); x:(52,0)

Example $\PageIndex{52}$:

$y=−x^2−14x−49$

Graph Quadratic Equations in Two Variables

In the following exercises, graph by using intercepts, the vertex, and the axis of symmetry.

Example $\PageIndex{53}$

$y=x^2+6x+5$

Answer

y:(0,5); x:(−1,0),(−5,0);
axis: x=−3; vertex:(−3,−4)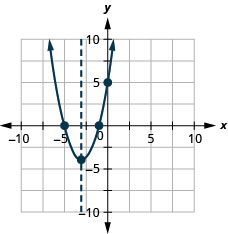

$This figure shows an upward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 10. The y-axis of the plane runs from -10 to 10. The parabola has points plotted at the vertex (-3, -4) and the intercepts (-5, 0), (-1, 0) and (0, 5). Also on the graph is a dashed vertical line representing the axis of symmetry. The line goes through the vertex at x equals -3.$

Example $\PageIndex{54}$:

$y=x^2+4x−12$

Example $\PageIndex{55}$

$y=x^2+4x+3$

Answer

y:(0,3); x:(−1,0),(−3,0);
axis: x=−2; vertex:(−2,−1)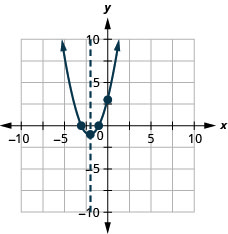

$This figure shows an upward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 10. The y-axis of the plane runs from -10 to 10. The parabola has points plotted at the vertex (-2, -1) and the intercepts (-1, 0), (-3, 0) and (0, 3). Also on the graph is a dashed vertical line representing the axis of symmetry. The line goes through the vertex at x equals -2.$

Example $\PageIndex{56}$:

$y=x^2−6x+8$

Example $\PageIndex{57}$

$y=9x^2+12x+4$

Answer

y:(0,4); x:$(−\frac{2}{3},0)$;
axis: $(−\frac{2}{3}$; vertex:$(−\frac{2}{3},0)$

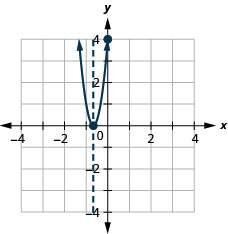
$This figure shows an upward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -5 to 5. The y-axis of the plane runs from -5 to 5. The parabola has points plotted at the vertex (-2 thirds, 0) and the intercept (0, 4). Also on the graph is a dashed vertical line representing the axis of symmetry. The line goes through the vertex at x equals -2 thirds.$

Example $\PageIndex{58}$:

$y=−x^2+8x−16$

Example $\PageIndex{59}$

$y=−x^2+2x−7$

Answer

y:(0,−7); x:none;
axis: x=1; vertex:(1,−6)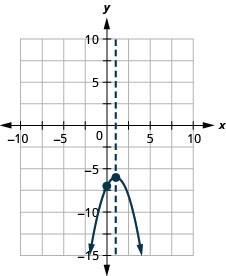

$This figure shows a downward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 10. The y-axis of the plane runs from -15 to 5. The parabola has points plotted at the vertex (1, -6) and the intercept (0, -7). Also on the graph is a dashed vertical line representing the axis of symmetry. The line goes through the vertex at x equals 1.$

Example $\PageIndex{60}$:

$y=5x^2+2$

Example $\PageIndex{61}$

$y=2x^2−4x+1$

Answer: y:(0,1); x:(1.7,0),(0.3,0);
axis: x=1; vertex:(1,−1)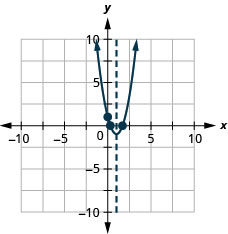
$This figure shows an upward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 10. The y-axis of the plane runs from -10 to 10. The parabola has points plotted at the vertex (1, -1) and the intercepts (1.7, 0), (0.3, 0) and (0, 1). Also on the graph is a dashed vertical line representing the axis of symmetry. The line goes through the vertex at x equals 1.$

Example $\PageIndex{62}$:

$y=−4x^2−6x−2$

Example $\PageIndex{63}$

$y=−x^2−4x+2$

Answer

y:(0,2); x:(−4.4,0),(0.4,0);
axis: x=−2; vertex:(−2,6)

$This figure shows a downward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 10. The y-axis of the plane runs from -10 to 10. The parabola has points plotted at the vertex (-2, 6) and the intercepts (-4.4, 0), (0.4, 0) and (0, 2). Also on the graph is a dashed vertical line representing the axis of symmetry. The line goes through the vertex at x equals -2.$

Example $\PageIndex{64}$:

$y=x^2+6x+8$

Example $\PageIndex{65}$

$y=5x^2−10x+8$

Answer

y:(0,8); x:none;
axis: x=1; vertex:(1,3)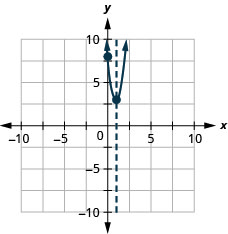

$This figure shows an upward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 10. The y-axis of the plane runs from -10 to 10. The parabola has points plotted at the vertex (1, 3) and the intercept(0, 8). Also on the graph is a dashed vertical line representing the axis of symmetry. The line goes through the vertex at x equals 1.$

Example $\PageIndex{66}$:

$y=−16x^2+24x−9$

Example $\PageIndex{67}$

$y=3x^2+18x+20$

Answer

y:(0,20); x:(−4.5,0),(−1.5,0)
axis: x=−3; vertex:(−3,−7)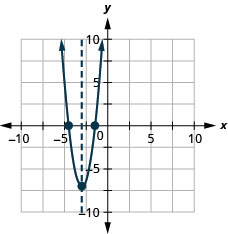

$This figure shows an upward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 10. The y-axis of the plane runs from -10 to 10. The parabola has points plotted at the vertex (-3, -7) and the intercepts (-4.5, 0) and (-1.5, 0). Also on the graph is a dashed vertical line representing the axis of symmetry. The line goes through the vertex at x equals -3.$

Example $\PageIndex{68}$:

$y=−2x^2+8x−10$

Solve Maximum and Minimum Applications

In the following exercises, find the maximum or minimum value.

Example $\PageIndex{69}$

$y=2x^2+x−1$

Answer: The minimum value is $−\frac{9}{8}$ when $x=−\frac{1}{4}$.

Example $\PageIndex{70}$:

$y=−4x^2+12x−5$

Example $\PageIndex{71}$

$y=x^2−6x+15$

Answer: The minimum value is 6 when x=3.

Example $\PageIndex{72}$:

$y=−x^2+4x−5$

Example $\PageIndex{73}$

$y=−9x^2+16$

Answer: The maximum value is 16 when x=0.

Example $\PageIndex{74}$:

$y=4x^2−49$

In the following exercises, solve. Round answers to the nearest tenth.

Example $\PageIndex{75}$

An arrow is shot vertically upward from a platform 45 feet high at a rate of 168 ft/sec. Use the quadratic equation $h=−16t^2+168t+45$ to find how long it will take the arrow to reach its maximum height, and then find the maximum height.

Answer: In 5.3 sec the arrow will reach maximum height of 486 ft.

Example $\PageIndex{76}$:

A stone is thrown vertically upward from a platform that is 20 feet high at a rate of 160 ft/sec. Use the quadratic equation $h=−16t^2+160t+20$ to find how long it will take the stone to reach its maximum height, and then find the maximum height.

Example $\PageIndex{77}$

A computer store owner estimates that by charging x dollars each for a certain computer, he can sell $40−x$ computers each week. The quadratic equation $R=−x^2+40x$ is used to find the revenue, R, received when the selling price of a computer is x. Find the selling price that will give him the maximum revenue, and then find the amount of the maximum revenue.

Answer: 20 computers will give the maximum of $400 in receipt.

Example $\PageIndex{78}$:

A retailer who sells backpacks estimates that, by selling them for x dollars each, he will be able to sell $100−x$ backpacks a month. The quadratic equation $R=−x^2+100x$ is used to find the R received when the selling price of a backpack is x. Find the selling price that will give him the maximum revenue, and then find the amount of the maximum revenue.

Example $\PageIndex{79}$

A rancher is going to fence three sides of a corral next to a river. He needs to maximize the corral area using 240 feet of fencing. The quadratic equation A=x(240−2x) gives the area of the corral, A, for the length, x, of the corral along the river. Find the length of the corral along the river that will give the maximum area, and then find the maximum area of the corral.

Answer: The length of the side along the river of the corral is 120 feet and the maximum area is 7,200 sq ft.

Example $\PageIndex{80}$:

A veterinarian is enclosing a rectangular outdoor running area against his building for the dogs he cares for. He needs to maximize the area using 100 feet of fencing. The quadratic equation $A=x(100−2x)$ gives the area, A, of the dog run for the length, x, of the building that will border the dog run. Find the length of the building that should border the dog run to give the maximum area, and then find the maximum area of the dog run.

Everyday Math

Example $\PageIndex{81}$

In the previous set of exercises, you worked with the quadratic equation $R=−x^2+40x$ that modeled the revenue received from selling computers at a price of x dollars. You found the selling price that would give the maximum revenue and calculated the maximum revenue. Now you will look at more characteristics of this model.
1. Graph the equation $R=−x^2+40x$.

2. Find the values of the x-intercepts.

Answer

1.
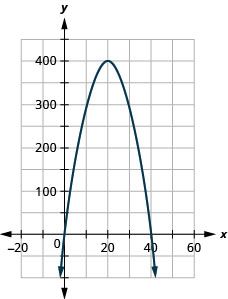
$This figure shows a downward-opening parabola graphed on the x y-coordinate plane. The x-axis of the plane runs from -10 to 60. The y-axis of the plane runs from -50 to 500. The parabola has a vertex at (20, 400) and also goes through the points (0, 0) and (40, 0).$

2. (0,0), (40,0)

Example $\PageIndex{82}$:

the previous set of exercises, you worked with the quadratic equation $R=−x^2+100x$ that modeled the revenue received from selling backpacks at a price of x dollars. You found the selling price that would give the maximum revenue and calculated the maximum revenue. Now you will look at more characteristics of this model.

1. Graph the equation $R=−x^2+100x$.

2.Find the values of the x-intercepts.

Writing Exercises

Example $\PageIndex{83}$

For the revenue model in Exercise and Exercise, explain what the x-intercepts mean to the computer store owner.

Answer: Answers will vary.

Example $\PageIndex{84}$:

For the revenue model in Exercise and Exercise, explain what the x-intercepts mean to the backpack retailer.

Self Check

a. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.

$This table has six rows and four columns. The first row is a header row and it labels each column. The first column is labeled "I can …", the second "Confidently", the third “With some help” and the last "No–I don’t get it". In the “I can…” column the second row reads “solve quadratic equations using the quadratic for recognize the graph of a quadratic equation in two variables.” The third row reads “find the axis of symmetry and vertex of a parabola.” The fourth row reads “find the intercepts of a parabola.” The fifth row reads “graph quadratic equations in two variables.” and the last row reads “solve maximum and minimum applications.” The remaining columns are blank.$

b. What does this checklist tell you about your mastery of this section? What steps will you take to improve?

Practice Makes Perfect

Example \(\PageIndex{37}\)

Example \(\PageIndex{38}\):

Example \(\PageIndex{39}\)

Example \(\PageIndex{40}\):

Example \(\PageIndex{41}\)

Example \(\PageIndex{42}\):

Example \(\PageIndex{43}\)

Example \(\PageIndex{44}\):

Example \(\PageIndex{45}\)

Example \(\PageIndex{46}\):

Example \(\PageIndex{47}\)

Example \(\PageIndex{48}\):

Example \(\PageIndex{49}\)

Example \(\PageIndex{50}\):

Example \(\PageIndex{51}\)

Example \(\PageIndex{52}\):

Example \(\PageIndex{53}\)

Example \(\PageIndex{54}\):

Example \(\PageIndex{55}\)

Example \(\PageIndex{56}\):

Example \(\PageIndex{57}\)

Example \(\PageIndex{58}\):

Example \(\PageIndex{59}\)

Example \(\PageIndex{60}\):

Example \(\PageIndex{61}\)

Example \(\PageIndex{62}\):

Example \(\PageIndex{63}\)

Example \(\PageIndex{64}\):

Example \(\PageIndex{65}\)

Example \(\PageIndex{66}\):

Example \(\PageIndex{67}\)

Example \(\PageIndex{68}\):

Example \(\PageIndex{69}\)

Example \(\PageIndex{70}\):

Example \(\PageIndex{71}\)

Example \(\PageIndex{72}\):

Example \(\PageIndex{73}\)

Example \(\PageIndex{74}\):

Example \(\PageIndex{75}\)

Example \(\PageIndex{76}\):

Example \(\PageIndex{77}\)

Example \(\PageIndex{78}\):

Example \(\PageIndex{79}\)

Example \(\PageIndex{80}\):

Everyday Math

Example \(\PageIndex{81}\)

Example \(\PageIndex{82}\):

Writing Exercises

Example \(\PageIndex{83}\)

Example \(\PageIndex{84}\):

Self Check