22.4: What Fraction of a Group?
- Page ID
- 40242
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Lesson
Let's think about dividing things into groups when we can't even make one whole group.
Exercise \(\PageIndex{1}\): Estimating a Fraction of a Number
- Estimate the quantities:
- What is \(\frac{1}{3}\) of \(7\)?
- What is \(\frac{4}{5}\) of \(9\frac{2}{3}\)?
- What is \(2\frac{4}{7}\) of \(10\frac{1}{9}\)?
- Write a multiplication expression for each of the previous questions.
Exercise \(\PageIndex{2}\): Fractions of Ropes
The segments in the applet represent 4 different lengths of rope. Compare one rope to another, moving the rope by dragging the open circle at one endpoint. You can use the yellow pins to mark off lengths.
- Complete each sentence comparing the lengths of the ropes. Then, use the measurements shown on the grid to write a multiplication equation and a division equation for each comparison.
- Rope B is _______ times as long as rope A.
- Rope C is _______ times as long as rope A.
- Rope D is _______ times as long as rope A.
- Each equation can be used to answer a question about Ropes C and D. What could each question be?
- \(?\cdot 3=9\) and \(9\div 3=?\)
- \(?\cdot 9=3\) and \(3\div 9=?\)
Exercise \(\PageIndex{3}\): Fractional Batches of Ice Cream
One batch of an ice cream recipe uses 9 cups of milk. A chef makes different amounts of ice cream on different days. Here are the amounts of milk she used:
- Monday: \(12\) cups
- Tuesday: \(22\frac{1}{2}\) cups
- Thursday: \(6\) cups
- Friday: \(7\frac{1}{2}\) cups
- How many batches of ice cream did she make on these days? For each day, write a division equation, draw a tape diagram, and find the answer.
- Monday
- Tuesday
- What fraction of a batch of ice cream did she make on these days? For each day, write a division equation, draw a tape diagram, and find the answer.
- Thursday
- Friday
- For each question, write a division equation, draw a tape diagram, and find the answer.
- What fraction of \(9\) is \(3\)?
- What fraction of \(5\) is \(\frac{1}{2}\)?
Summary
It is natural to think about groups when we have more than one group, but we can also have a fraction of a group.
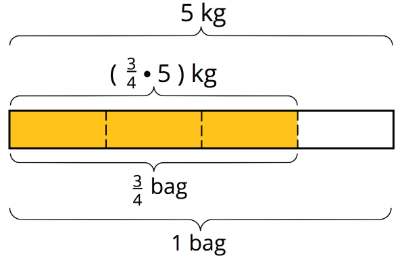
To find the amount in a fraction of a group, we can multiply the fraction by the amount in the whole group. If a bag of rice weighs 5 kg, \(\frac{3}{4}\) of a bag would weigh \(\left(\frac{3}{4}\cdot 5\right)\) kg.
Sometimes we need to find what fraction of a group an amount is. Suppose a full bag of flour weighs 6 kg. A chef used 3 kg of flour. What fraction of a full bag was used? In other words, what fraction of 6 kg is 3 kg?
This question can be represented by a multiplication equation and a division equation, as well as by a diagram.
\(?\cdot 6=3\)
\(3\div 6=?\)
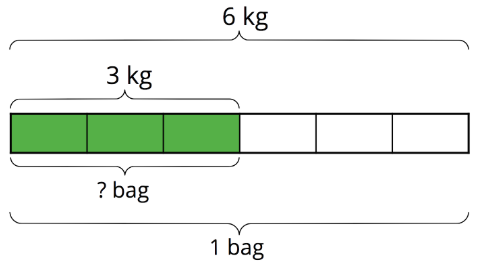
We can see from the diagram that 3 is \(\frac{1}{2}\) of 6, and we can check this answer by multiplying: \(\frac{1}{2}\cdot 6=3\).
In any situation where we want to know what fraction one number is of another number, we can write a division equation to help us find the answer.
For example, “What fraction of 3 is \(2\frac{1}{4}\)?” can be expressed as \(?\cdot 3=2\frac{1}{4}\), which can also be written as \(2\frac{1}{4}\div 3=?\).
The answer to “What is \(2\frac{1}{4}\div 3\)?” is also the answer to the original question.
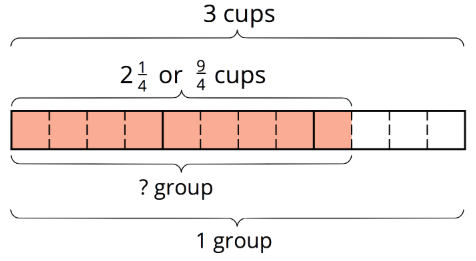
The diagram shows that 3 wholes contain 12 fourths, and \(2\frac{1}{4}\) contains 9 fourths, so the answer to this question is \(\frac{9}{12}\), which is equivalent to \(\frac{3}{4}\).
We can use diagrams to help us solve other division problems that require finding a fraction of a group. For example, here is a diagram to help us answer the question: “What fraction of \(\frac{9}{4}\) is \(\frac{3}{2}\)?,” which can be written as \(\frac{3}{2}\div\frac{9}{4}=?\).
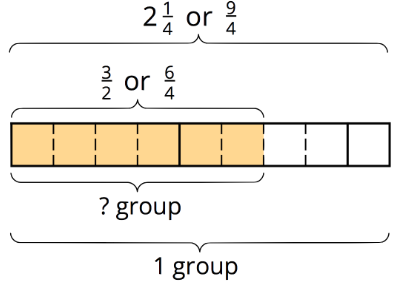
We can see that the quotient is \(\frac{6}{9}\), which is equivalent to \(\frac{2}{3}\). To check this, let’s multiply. \(\frac{2}{3}\cdot\frac{9}{4}=\frac{18}{12}\), and \(\frac{18}{12}\) is, indeed, equal to \(\frac{3}{2}\).
Practice
Exercise \(\PageIndex{4}\)
A recipe calls for \(\frac{1}{2}\) lb of flour for 1 batch. How many batches can be made with each of these amounts?
- \(1\) lb
- \(\frac{3}{4}\) lb
- \(\frac{1}{4}\) lb
Exercise \(\PageIndex{5}\)
Whiskers the cat weighs \(2\frac{2}{3}\) kg. Piglio weighs \(4\) kg. For each question, write a multiplication equation and a division equation, decide whether the answer is greater than 1 or less than 1, and then find the answer.
- How many times as heavy as Piglio is Whiskers?
- How many times as heavy as Whiskers is Piglio?
Exercise \(\PageIndex{6}\)
Andre is walking from his home to a festival that is \(1\frac{5}{8}\) kilometers away. He walks \(\frac{1}{3}\) kilometer and then takes a quick rest. Which question can be represented by the equation \(?\cdot 1\frac{5}{8}=\frac{1}{3}\) in this situation?
- What fraction of the trip has Andre completed?
- What fraction of the trip is left?
- How many more kilometers does Andre have to walk to get to the festival?
- How many kilometers is it from home to the festival and back home?
Exercise \(\PageIndex{7}\)
Draw a tape diagram to represent the question: What fraction of \(2\frac{1}{2}\) is \(\frac{4}{5}\)?
Then find the answer.
Exercise \(\PageIndex{8}\)
How many groups of \(\frac{3}{4}\) are in each of these quantities?
- \(\frac{11}{4}\)
- \(6\frac{1}{2}\)
(From Unit 4.2.3)
Exercise \(\PageIndex{9}\)
Which question can be represented by the equation \(4\div\frac{2}{7}=?\)
- What is \(4\) groups of \(\frac{2}{7}\)?
- How many \(\frac{2}{7}\)s are in \(4\)?
- What is \(\frac{2}{7}\) of \(4\)?
- How many \(4\)s are in \(\frac{2}{7}\)?
(From Unit 4.2.1)