33.4: Equivalent Exponential Expressions
- Page ID
- 40612
Lesson
Let's investigate expressions with variables and exponents.
Exercise \(\PageIndex{1}\): Up or Down?
Find the values of \(3^{x}\) and \(\left(\frac{1}{3}\right)^{x}\) for different values of \(x\). What patterns do you notice?
| \(x\) | \(3^{x}\) | \(\left(\frac{1}{3}\right)^{x}\) |
|---|---|---|
| \(1\) | ||
| \(2\) | ||
| \(3\) | ||
| \(4\) |
Exercise \(\PageIndex{2}\): What's the Value?
Evaluate each expression for the given value of \(x\).
- \(3x^{2}\) when \(x\) is \(10\)
- \(3x^{2}\) when \(x\) is \(\frac{1}{9}\)
- \(\frac{x^{3}}{4}\) when \(x\) is \(4\)
- \(\frac{x^{3}}{4}\) when \(x\) is \(\frac{1}{2}\)
- \(9+x^{7}\) when \(x\) is \(1\)
- \(9+x^{7}\) when \(x\) is \(\frac{1}{2}\)
Exercise \(\PageIndex{3}\): Exponent Experimentation
Find a solution to each equation in the list. (Numbers in the list may be a solution to more than one equation, and not all numbers in the list will be used.)
- \(64=x^{2}\)
- \(64=x^{3}\)
- \(2^{x}=32\)
- \(x=\left(\frac{2}{5}\right)^{3}\)
- \(\frac{16}{9}=x^{2}\)
- \(2\cdot 2^{5}=2^{x}\)
- \(2x=2^{4}\)
- \(4^{3}=8^{x}\)
List:
\(\frac{8}{125}\quad \frac{6}{15}\quad\frac{5}{8}\quad\frac{8}{9}\quad 1\frac{4}{3}\quad 2\quad 3\quad 4\quad 5\quad 6\quad 8\)
Are you ready for more?
This fractal is called a Sierpinski Tetrahedron. A tetrahedron is a polyhedron that has four faces. (The plural of tetrahedron is tetrahedra.)
The small tetrahedra form four medium-sized tetrahedra: blue, red, yellow, and green. The medium-sized tetrahedra form one large tetrahedron.

- How many small faces does this fractal have? Be sure to include faces you can’t see. Try to find a way to figure this out so that you don’t have to count every face.
- How many small tetrahedra are in the bottom layer, touching the table?
- To make an even bigger version of this fractal, you could take four fractals like the one pictured and put them together. Explain where you would attach the fractals to make a bigger tetrahedron.
- How many small faces would this bigger fractal have? How many small tetrahedra would be in the bottom layer?
- What other patterns can you find?
Summary
In this lesson, we saw expressions that used the letter \(x\) as a variable. We evaluated these expressions for different values of \(x\).
- To evaluate the expression \(2x^{3}\) when \(x\) is \(5\), we replace the letter \(x\) with \(5\) to get \(2\cdot 5^{3}\). This is equal to \(2\cdot 125\) or just \(250\). So the value of \(2x^{3}\) is \(250\) when \(x\) is \(5\).
- To evaluate \(\frac{x^{2}}{8}\) when \(x\) is \(4\), we replace the letter \(x\) with \(4\) to get \(\frac{4^{2}}{8}=\frac{16}{8}\), which equals \(2\). So \(\frac{x^{2}}{8}\) has a value of \(2\) when \(x\) is \(4\).
We also saw equations with the variable \(x\) and had to decide what value of \(x\) would make the equation true.
- Suppose we have an equation \(10\cdot 3^{x}=90\) and a list of possible solutions: \(1, 2, 3, 9, 11\). The only value of \(x\) that makes the equation true is \(2\) because \(10\cdot 3^{2}=10\cdot 3\cdot 3\), which equals \(90\). So \(2\) is the solution to the equation.
Practice
Exercise \(\PageIndex{4}\)
Evaluate each expression if \(x=3\).
- \(2^{x}\)
- \(x^{2}\)
- \(1^{x}\)
- \(x^{1}\)
- \(\left(\frac{1}{2}\right)^{x}\)
Exercise \(\PageIndex{5}\)
Evaluate each expression for the given value of each variable.
- \(2+x^{3}\), \(x\) is \(3\)
- \(x^{2}\), \(x\) is \(\frac{1}{2}\)
- \(3x^{2}+y\), \(x\) is \(5\) \(y\) is \(3\)
- \(10y+x^{2}\), \(x\) is \(6\) \(y\) is \(4\)
Exercise \(\PageIndex{6}\)
Decide if the expressions have the same value. If not, determine which expression has the larger value.
- \(2^{3}\) and \(3^{2}\)
- \(1^{31}\) and \(31^{1}\)
- \(4^{2}\) and \(2^{4}\)
- \(\left(\frac{1}{2}\right)^{3}\) and \(\left(\frac{1}{3}\right)^{2}\)
Exercise \(\PageIndex{7}\)
Match each equation to its solution.
- \(7+x^{2}=16\)
- \(5-x^{2}=1\)
- \(2\cdot 2^{3}=2^{x}\)
- \(\frac{3^{4}}{3^{x}}=27\)
- \(x=1\)
- \(x=2\)
- \(x=3\)
- \(x=4\)
Exercise \(\PageIndex{8}\)
An adult pass at the amusement park costs 1.6 times as much as a child’s pass.
- How many dollars does an adult pass cost if a child’s pass costs:
\($5\)?
\($10\)?
\(w\) dollars? - A child's pass costs $15. How many dollars does an adult pass cost?
(From Unit 6.2.1)
Exercise \(\PageIndex{9}\)
Jada reads 5 pages every 20 minutes. At this rate, how many pages can she read in 1 hour?
- Use a double number line to find the answer.
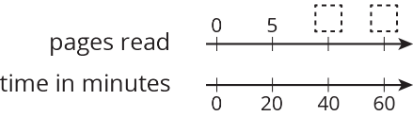
- Use a table to find the answer.
| pages read | time in minutes |
|---|---|
| \(5\) | \(20\) |
Which strategy do you think is better, and why?
(From Unit 2.4.4)

