1.2: Ordinary Differential Equations
( \newcommand{\kernel}{\mathrm{null}\,}\)
Set
$$E(v)=\int_a^bf(x,v(x),v'(x))\ dx\]
and for given ua, ub∈R
$$V=\{v\in C^2[a,b]:\ v(a)=u_a,\ v(b)=u_b\},\]
where y and f is sufficiently regular. One of the basic problems in the calculus of variation is
(P) minv∈VE(v).
Euler equation
Let u∈V be a solution of (P), then
$$\frac{d}{dx}f_{u'}(x,u(x),u'(x))=f_u(x,u(x),u'(x))\]
in (a,b).
Exercise 1.2.1: Proof
For fixed ϕ∈C2[a,b] with ϕ(a)=ϕ(b)=0 and real ϵ, |ϵ|<ϵ0, set g(ϵ)=E(u+ϵϕ). Since g(0)≤g(ϵ) it follows g′(0)=0. Integration by parts in the formula for g′(0) and the following basic lemma in the calculus of variations imply Euler's equation.
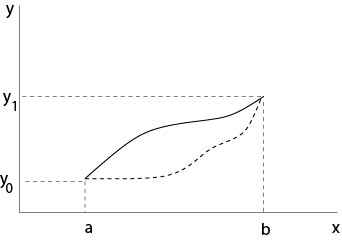
Figure 1.2.1.1: Admissible Variations
Basic lemma in the calculus of variations. Let h∈C(a,b) and
∫bah(x)ϕ(x) dx=0
for all ϕ∈C10(a,b). Then h(x)≡0 on (a,b).
Proof. Assume h(x0)>0 for an x0∈(a,b), then there is a δ>0 such that (x0−δ,x0+δ)⊂(a,b) and h(x)≥h(x0)/2 on (x0−δ,x0+δ).
Set
$$
\phi(x)
=\left\{(δ2−|x−x0|2)2x∈(x0−δ,x0+δ)0x∈(a,b)∖[x0−δ,x0+δ] \right. .
\]
Thus ϕ∈C10(a,b) and
$$\int_a^b h(x)\phi(x)\ dx\ge \frac{h(x_0)}{2}\int_{x_0-\delta}^{x_0+\delta}\phi(x)\ dx>0,\]
which is a contradiction to the assumption of the lemma.
◻
Contributors and Attributions
Integrated by Justin Marshall.


