7.2: The Remaining Inverse Trigonometric Functions

Suggested Prerequisite Topics

This section is designed assuming you understand the following topics from Algebra.

• Piecewise functions
• Set-builder notation

Learning Objectives

• Find the exact value of a non-fundamental inverse trigonometric function.
• Use technology to approximate the value of a non-fundamental inverse trigonometric function.
• Evaluate compositions involving trigonometric functions and non-fundamental inverse trigonometric functions.
• Simplify expressions involving trigonometric functions and non-fundamental inverse trigonometric functions.

In the previous section, we completely ignored the inverses of the secant, cosecant, and cotangent. This is not because they are unimportant - in fact, the inverses of the secant and cosecant are essential parts of Integral Calculus (also known as Calculus II). We have delayed their introduction because it's best to master the inverses of the fundamental trigonometric functions before jumping into the remaining inverse trigonometric functions.

The Inverse Cotangent Function

Like the rest of the trigonometric functions, the cotangent is not naturally one-to-one. Therefore, we restrict the domain of \(g(x) = \cot(x)\) to its principal cycle on \((0,\pi)\) to obtain a one-to-one segment of the cotangent. The vertical asymptotes, \(x=0\) and \(x=\pi\), of the graph of \(g(x) = \cot(x)\) become the horizontal asymptotes, \(y = 0\) and \(y = \pi\), of the graph of \(g^{-1}(x) = \mathrm{arccot}(x)\) (which, recall, is a reflection of the graph of \( y = \cot\left( x \right) \) about the line \( y=x \)).

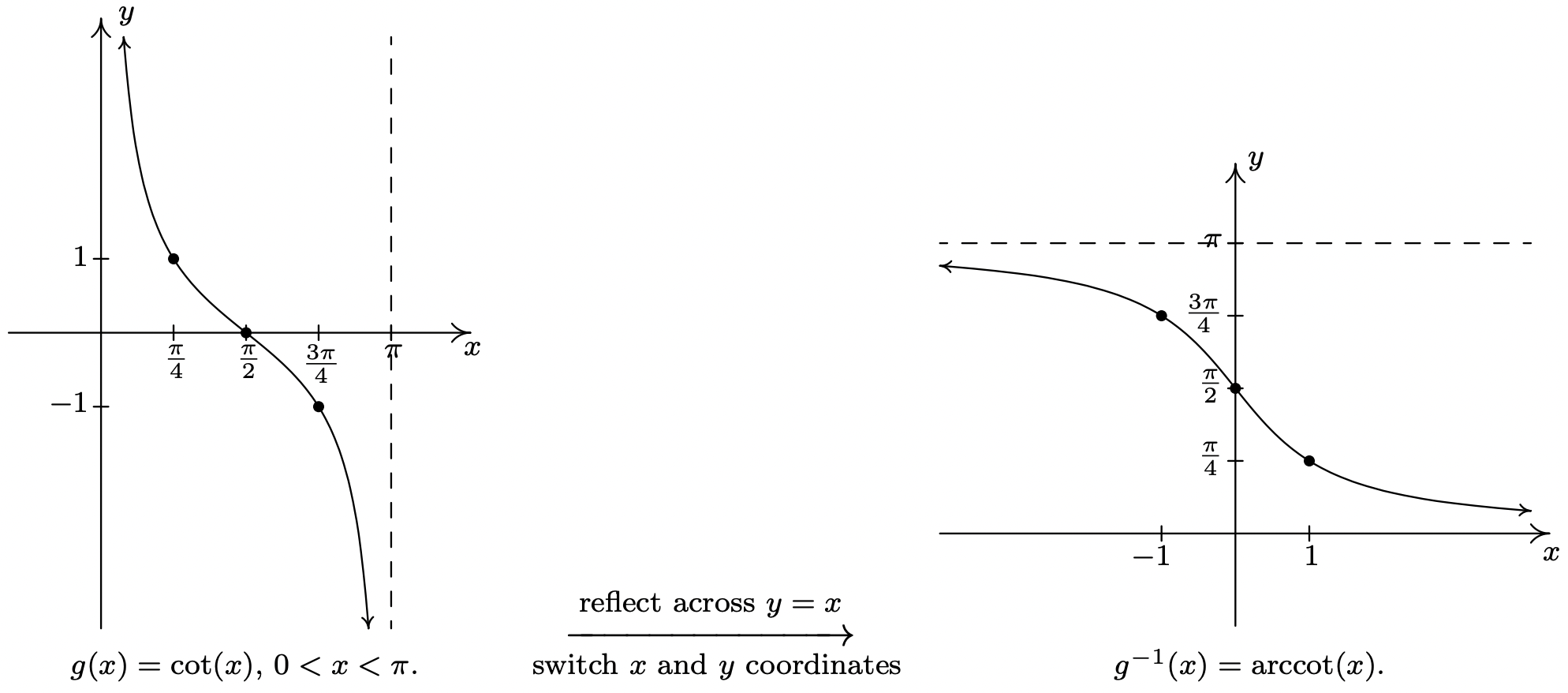
The definition of the arccotangent follows the same pattern as the definitions of our other inverse trigonometric functions.

Definition: The Inverse Cotangent Function

The function \( f(x) = \cot^{-1}(x) \) is defined as follows:¹\[ \cot^{-1}\left( x \right) = \theta \quad \text{if and only if} \quad \cot\left( \theta \right) = x \quad \text{and} \quad 0 \lt \theta \lt \pi. \nonumber \]

Theorem: Properties of the Inverse Cotangent Function

Properties of \(f(x) = \cot^{-1}(x)\)

• \( \theta = \cot^{-1}(x) \) if and only if \( \cot\left( \theta \right) = x \) where \( 0 \lt \theta \lt \pi \)
• Domain: \((-\infty, \infty)\)
• Range: \((0, \pi)\)
• As \(x \to -\infty\), \(\cot^{-1}(x) \to \pi^{-}\), and as \(x \to \infty\), \(\cot^{-1}(x) \to 0^{+}\)
• \(\cot\left(\cot^{-1}(x)\right) = x\) for all \(x \in \mathbb{R} \)
• \(\cot^{-1}(\cot(x)) = x\) provided \(0 \lt x \lt \pi\)

Example \( \PageIndex{ 1 } \)

1. Find the exact values of the following.
   1. \(\mathrm{arccot}(-\sqrt{3})\)
   2. \(\cot(\mathrm{arccot}(-5))\)
2. Rewrite the following as algebraic expressions of \(x\) and state the domain on which the equivalence is valid.\[\cos(\mathrm{arccot}(2x))\nonumber \]

Solutions

1. 1. \(\mathrm{arccot}(-\sqrt{3}) = \theta\) means \(\cot(\theta) = -\sqrt{3}\), where \( \theta \in \left( 0,\pi \right) \).
      
      Quadrant: Since the cotangent is negative, we further restrict the angle to \( \theta \in \left( \frac{\pi}{2} , \pi \right) \), or Quadrant II.
      
      Reference Angle: The reference angle for the cotangent that gives \( \cot\left( \hat{\theta} \right) = \sqrt{3} \) is \( \hat{\theta} = \frac{\pi}{6} \).
      
      Given the quadrant annd reference angle, we get\[\mathrm{arccot}\left(-\sqrt{3}\right) = \dfrac{5\pi}{6}.\nonumber \]
   2. It is helpful to remember that \( \mathrm{trig}\left( \mathrm{trig}^{-1}\left( x \right) \right) = x \) for all of the trigonometric functions as long as \( x \) is in the domain of \( \mathrm{trig}^{-1} \) (which is the range of \( \mathrm{trig} \)). Therefore, \(\cot(\mathrm{arccot}(-5)) = \cot\left( \cot^{-1}\left( -5 \right) \right) = -5\) because \( -5 \) is definitely in the domain of the inverse cotangent.
      
      Another way to think about this is to let \(\theta = \mathrm{arccot}(-5)\). Then \(\theta\) belongs to the interval \((0,\pi)\) and \(\cot(\theta)=-5\). Hence, \(\cot(\mathrm{arccot}(-5)) = \cot(\theta)=-5\).
2. To get started, we let \(\theta = \mathrm{arccot}(2x)\) so that \(\cot(\theta) = 2x\) where \(0 \lt \theta \lt \pi\). We then sketch two reference triangles, one in \( \mathrm{QI} \) and the other in \( \mathrm{QII} \).

           

   In terms of \(\theta\),\[\cos(\mathrm{arccot}(2x)) = \cos(\theta).\nonumber \]Therefore, we can use our reference triangles to evaluate \( \cos\left( \theta \right) \). Looking at the reference triangle in \( \mathrm{QI} \), \( \cos\left( \theta \right) = \frac{2x}{\sqrt{4x^2 + 1}} \). Likewise, the reference triangle from the \( \mathrm{QII} \) sketch yields \( \cos\left( \theta \right) = \frac{2x}{\sqrt{4x^2 + 1}} \). Hence, it appears that\[ \cos\left( \mathrm{arccot}\left( 2x \right) \right) = \cos\left( \theta \right) = \dfrac{2x}{\sqrt{4x^2 + 1}}. \nonumber \]Being good mathematicians, we take a moment to reflect and make sure our logic is solid. What might give us pause is the fact that our second sketch, where \( \theta \in \mathrm{QII} \), leads to cosine being negative. Still, our result, \( \cos\left( \theta \right) = \frac{2x}{\sqrt{4x^2 + 1}} \), looks to be positive; however, looks can be deceiving!
   
   The expression, \( 2x \), in the second sketch, is, in fact, negative. The negativity of it is "hidden" within the variable \( x \). That is, if the \( x \)-value of the point on the upper-left vertex of that reference triangle is \( -4 \), then \( 2x = -4 \implies x = -2 \). Hence, when \( \theta \in \mathrm{QII} \), \( \cos\left( \theta \right) = \frac{2x}{\sqrt{4x^2 + 1}} \) is negative (which is what we want).
   
   We now concern ourselves with the domain of the function. We know that the domain of the arccotangent is all real numbers. Hence, the domain of \( \mathrm{arccot}\left( 2x \right) \) is also all real numbers. Moreover, the range of the arccotangent is \( \left( 0,\pi \right) \). Since the outputs of \( \mathrm{arccot}\left( 2x \right) \) are the inputs of \( \cos\left( \mathrm{arccot}\left( 2x \right) \right) \) and the cosine can take any value as an input, the domain remains unchanged. That is, the domain of \( y = \cos\left( \mathrm{arccot}\left( 2x \right) \right) \) is \( \left( -\infty,\infty \right) \).

Checkpoint \(\PageIndex{1}\)

Find the exact value of the expression.\[ \sin\left( \cot^{-1}\left( -\dfrac{5}{2} \right) \right) \nonumber \]

Answer

\( -\dfrac{5}{\sqrt{29}} \)

The Inverse Secant and Cosecant Functions

The last two functions to invert are secant and cosecant. The principal cycle for each is highlighted in the figure below.

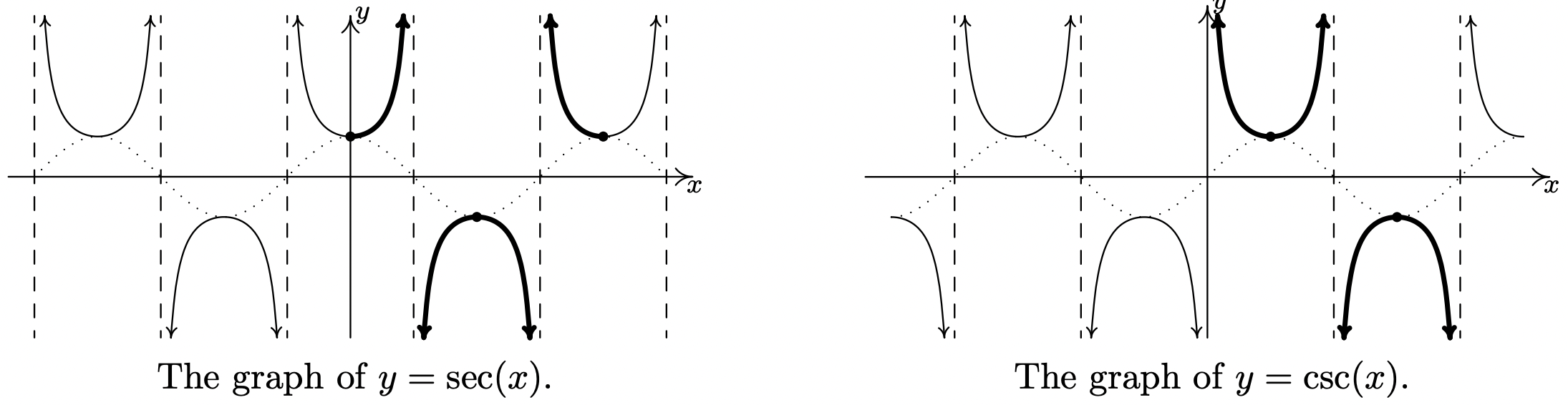
It is clear from the graph of secant that we cannot find one single, continuous piece of its graph which covers its entire range of \((-\infty, -1] \cup [1, \infty)\), and which restricts the domain of the function so that it is one-to-one. The same is true for cosecant. Thus, in order to define the arcsecant and arccosecant functions, we must settle for a piecewise approach wherein we choose one piece to cover the top of the range, namely \([1, \infty)\), and another piece to cover the bottom, namely \((-\infty, -1]\). To make the definitions of the inverse secant and cosecant functions align with our previous work, we restrict their domains to coincide with the restrictions on cosine and sine, respectively.²

When defining the arccosine, we restricted the cosine to the interval \( \left[0,\pi  \right] \); however, such a restriction needs to be modified for the secant because the secant is undefined at \( x = \frac{\pi}{2} \). Thus, we restrict the domain of the secant to \(\left[0, \frac{\pi}{2}\right) \cup \left( \frac{\pi}{2}, \pi\right]\) to help us define the arcsecant.

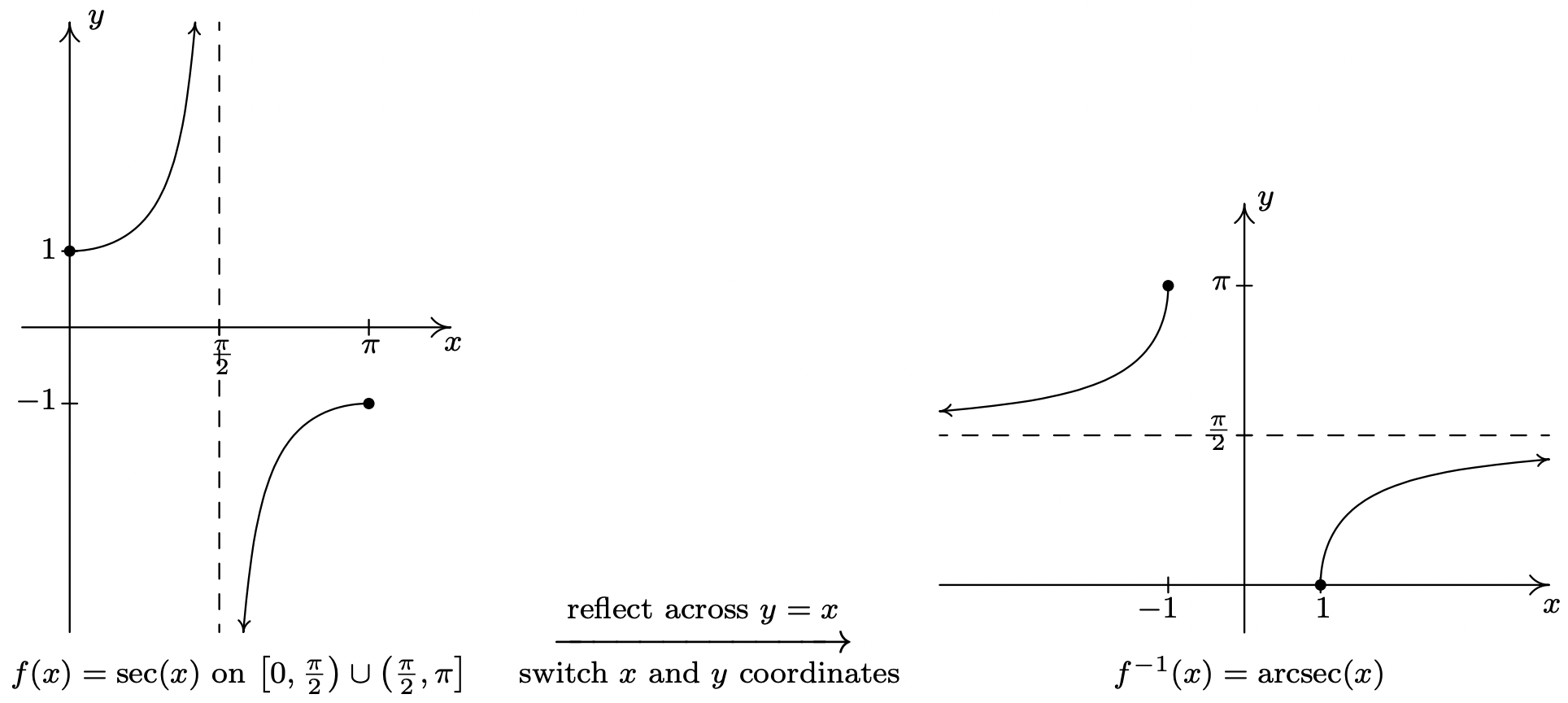
This gives us the following definition.

Definition: The Inverse Secant Function

The function \( f(x) = \sec^{-1}(x) \) is defined as follows:\[ \sec^{-1}\left( x \right) = \theta \quad \text{if and only if} \quad \sec\left( \theta \right) = x \quad \text{and} \quad \theta \in \left[ 0,\dfrac{\pi}{2} \right) \cup \left( \dfrac{\pi}{2}, \pi \right]. \nonumber \]

Likewise, we restricted the sine function to the interval \( \left[ -\frac{\pi}{2}, \frac{\pi}{2} \right] \) when defining the arcsine, but such a restriction will not work for the arccosecant because the cosecant is undefined at \( x = 0 \). Therefore, we restrict \(g(x) = \csc(x)\) to \(\left[-\frac{\pi}{2}, 0\right) \cup \left(0, \frac{\pi}{2}\right]\) to define the arccosecant.

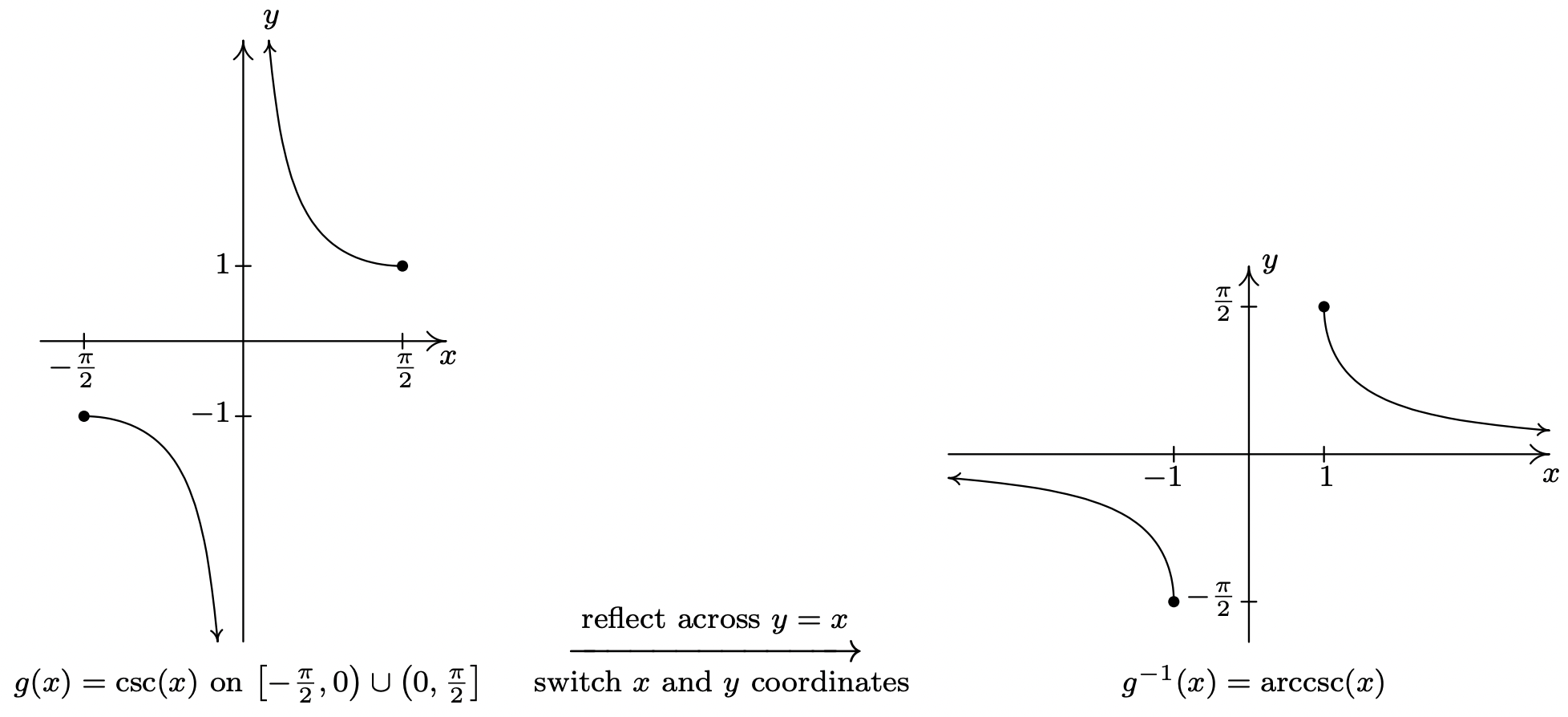
Note that, for both arcsecant and arccosecant, the domain is \((-\infty, -1] \cup [1, \infty)\). We can rewrite this using set-builder notation as \(\left\{ x \mid |x| \geq 1\right\}\). This is often done in Calculus textbooks, so we include it here for completeness.

Definition: The Inverse Cosecant Function

The function \( f(x) = \csc^{-1}(x) \) is defined as follows:\[ \csc^{-1}\left( x \right) = \theta \quad \text{if and only if} \quad \csc\left( \theta \right) = x \quad \text{and} \quad \theta \in \left[ -\dfrac{\pi}{2},0 \right) \cup \left(0, \dfrac{\pi}{2} \right]. \nonumber \]

Using these definitions, we get the following properties of the arcsecant and arccosecant functions.

Theorem: Properties of the Arcsecant and Arccosecant Functions

Properties of \(f(x)= \mathrm{arcsec}(x)\)

• \(\sec^{-1}\left( x \right) = \theta\) if and only if \(\sec\left( \theta \right) = x\) where \(\theta \in \left[ 0,\frac{\pi}{2} \right) \cup \left( \frac{\pi}{2}, \pi \right]\)
• Domain: \(\left\{ x : |x| \geq 1 \right\} = (-\infty, -1] \cup [1,\infty)\)
• Range: \(\left[0, \frac{\pi}{2} \right) \cup \left(\frac{\pi}{2}, \pi\right]\)
• As \(x \to -\infty\), \(\mathrm{arcsec}(x) \to \frac{\pi}{2}^{+}\), and as \(x \to \infty\), \(\mathrm{arcsec}(x) \to \frac{\pi}{2}^{-}\)
• \(\sec\left(\mathrm{arcsec}(x)\right) = x\) provided \(|x| \geq 1\)
• \(\mathrm{arcsec}(\sec(x)) = x\) provided \(0 \leq x < \frac{\pi}{2}\) or \(\frac{\pi}{2} < x \leq \pi\)

Properties of \(f(x) = \mathrm{arccsc}(x)\)

• \(\csc^{-1}\left( x \right) = \theta\) if and only if \(\csc\left( \theta \right) = x\) where \(\theta \in \left[ -\dfrac{\pi}{2},0 \right) \cup \left(0, \dfrac{\pi}{2} \right]\)
• Domain: \(\left\{ x : |x| \geq 1 \right\} = (-\infty, -1] \cup [1,\infty)\)
• Range: \(\left[-\frac{\pi}{2}, 0 \right) \cup \left(0, \frac{\pi}{2} \right]\)
• As \(x \to -\infty\), \(\mathrm{arccsc}(x) \to 0^{-}\), and as \(x \to \infty\), \(\mathrm{arccsc}(x) \to 0^{+}\)
• \(\csc\left(\mathrm{arccsc}(x)\right) = x\) provided \(|x| \geq 1\)
• \(\mathrm{arccsc}(\csc(x)) = x\) provided \(-\frac{\pi}{2} \leq x < 0\) or \(0 < x \leq \frac{\pi}{2}\)
• Additionally, arccosecant is an odd function.

Example \( \PageIndex{ 2 } \)

1. Find the exact values of the following.
   1. \(\sec^{-1}(2)\)
   2. \(\mathrm{arccsc}(-2)\)
   3. \(\mathrm{arcsec}\left( \sec\left( \frac{8\pi}{7} \right) \right)\)
   4. \(\cot\left(\mathrm{arccsc}\left(-3\right)\right)\)
2. Rewrite the following as algebraic expressions of \(x\) and state the domain on which the equivalence is valid.
   1. \(\tan(\mathrm{arcsec}(x))\)
   2. \(\cos(\mathrm{arccsc}(4x))\)

Solutions

1.  
   1. Let \( \theta = \sec^{-1}(2) \). Then \( \sec\left( \theta \right) = 2 \), where \( \theta \in \left[ 0, \frac{\pi}{2} \right) \cup \left( \frac{\pi}{2}, \pi \right] \). Of these two intervals, the only one where secant is positive is \( \left[ 0,\frac{\pi}{2} \right) \). Thus, \( \theta \in \mathrm{QI} \). Moreover, \( \sec\left( \hat{\theta} \right) = 2 \) implies \( \cos\left( \hat{\theta} \right) = \frac{1}{2} \). Hence, \( \hat{\theta} = \frac{\pi}{3} \). Therefore,\[\sec^{-1}(2) = \theta = \dfrac{\pi}{3}.\nonumber \]
   2. We now start to streamline our arguments.\[ \begin{array}{rrclccl}
      & \mathrm{arccsc}(-2) & = & \theta & & \quad & \left( \text{inverse trigonometric functions return angles,} \right) \\
      \implies & \csc(\theta) & = & -2, & \text{ where } \theta \in \left[ -\dfrac{\pi}{2},0 \right) \cup \left( 0, \dfrac{\pi}{2} \right] & \quad & \left( \text{but the angle is restricted} \right) \\
      \implies & \theta & \in & \left[ -\dfrac{\pi}{2},0 \right) & & \quad & \left( \text{cosecant is negative} \right) \\
      \text{and} & \hat{\theta} & = & \dfrac{\pi}{6} & & & \\
      \implies & \mathrm{arccsc}(-2) & = & -\dfrac{\pi}{6} & & \quad & \left( \text{reference angle of }\dfrac{\pi}{6} \text{ in the interval } \left[ -\dfrac{\pi}{2},0 \right) \right) \\
      \end{array} \nonumber \]
   3. Our choice of non-standard angle for this example is purposeful. It's to force you to think about the process instead of focusing solely on computing.\[ \begin{array}{rrcllcl}
      & \mathrm{arcsec}\left( \sec\left( \dfrac{8\pi}{7} \right) \right) & = & \theta, & \text{ where } \theta \in \left[ 0, \dfrac{\pi}{2} \right) \cup \left( \dfrac{\pi}{2}, \pi \right] & \quad & \left( \text{inverse trigonometric functions return restricted angles} \right) \\
      \implies & \sec(\theta) & = & \sec\left( \dfrac{8\pi}{7} \right) & & & \\
      \end{array} \nonumber \]Therefore, the reference angle for \( \theta \) matches the reference angle for \( \frac{8\pi}{7} \). That is,\[ \hat{\theta} = \dfrac{\pi}{7}. \nonumber \]We now need to determine in which quadrant \( \theta \) belongs. Since \( \frac{8\pi}{7} \in \mathrm{QIII} \), \( \sec\left( \frac{8\pi}{7} \right) \) is negative. Moreover, since \( \sec(\theta) = \sec\left( \frac{8\pi}{7} \right) \), \( \sec\left( \theta \right) \) must be negative. Hence, \( \theta \in \left( \frac{\pi}{2}, \pi \right] \). Thus, \( \theta = \frac{6\pi}{7} \). This means that\[ \mathrm{arcsec}\left( \sec\left( \dfrac{8\pi}{7} \right) \right) = \theta = \dfrac{6\pi}{7}. \nonumber \]
   4. As usual, we begin by addressing the fact that the inverse trigonometric function is an angle.\[ \begin{array}{rrcllcl}
      & \mathrm{arccsc}\left( -3 \right) & = & \theta, & \text{ where } \theta \in \left[ -\dfrac{\pi}{2},0 \right) \cup \left(0, \dfrac{\pi}{2} \right] & \quad & \left( \text{inverse trigonometric functions return restricted angles} \right) \\
      \implies & \csc(\theta) & = & -3 & & & \\
      \end{array} \nonumber \]However, we are interested in\[ \cot\left(\mathrm{arccsc}\left(-3\right)\right) = \cot\left( \theta \right). \nonumber \]Since the cosecant is negative, we know that \( \theta \in \left[ -\frac{\pi}{2},0 \right) \).Let sketch a reference triangle in \( \mathrm{QIV} \) to help us determine \( \cot\left( \theta \right) \).

      

      From the reference triangle, we see that\[\cot\left(\mathrm{arccsc}\left(-3\right)\right) = \cot\left( \theta \right) = -2\sqrt{2}.\nonumber \]
2.  
   1. As per usual, let \( \theta = \mathrm{arcsec}(x) \) so that \( \sec\left( \theta \right) = x \), where we restrict \( \theta \) to the interval \( \left[ 0, \frac{\pi}{2} \right) \cup \left( \frac{\pi}{2}, \pi \right] \). We then sketch two reference triangles, one for \( \theta \in \left[ 0,\frac{\pi}{2} \right) \) and the other for \( \theta \in \left( \frac{\pi}{2}, \pi \right] \).

             

      We now use these reference triangles to help us determine the value of \(\tan(\mathrm{arcsec}(x)) = \tan\left( \theta \right)\). In this case, we run into a very interesting problem. In Quadrant I, \( \tan\left( \theta \right) = \sqrt{x^2 - 1} \); however, in Quadrant II, \( \tan\left( \theta \right) = -\sqrt{x^2 - 1} \). Pausing for a moment, let's think about this before going further. Does this make sense?
      
      We know tangent is supposed to be positive in Quadrant I. Since \( \sec\left( \theta \right) = x \), we know either \( x \geq 1 \) or \( x \leq -1 \). Luckily, \( \sqrt{x^2 - 1} \) is definitely positive for these values of \( x \) (unless \( x = \pm1 \), but that would mean \( \tan\left( \theta \right) = 0 \implies \theta = 0 \) which is not in Quadrant I). So the statement, \( \tan\left( \theta \right) = \sqrt{x^2 - 1} \) when \( \theta \in \left( 0,\frac{\pi}{2} \right) \) makes sense.
      
      In Quadrant II, the tangent is supposed to be negative. It's easy to see that \( -\sqrt{x^2 - 1} \) is negative whenever \( \theta \in \left( \frac{\pi}{2},\pi \right) \). Thus, the statement, \( \tan\left( \theta \right) = -\sqrt{x^2 - 1} \) when \( \theta \in \left( \frac{\pi}{2},\pi \right) \) also makes sense. Therefore,\[\tan(\theta) = \left\{ \begin{array}{rr} \sqrt{x^2-1}, & \text{if  } 0 \leq \theta \lt \frac{\pi}{2} \\
      \\
      -\sqrt{x^2-1}, & \text{if  } \frac{\pi}{2} \lt \theta \leq \pi \\
       \end{array}\right.\nonumber\]Now we need to determine what these conditions on \(\theta\) mean for \(x\). Since \(x = \sec(\theta)\), when \(0 \leq \theta < \frac{\pi}{2}\), \(x \geq 1\), and when \(\frac{\pi}{2} \lt \theta \leq \pi\), \(x \leq -1\). Since we encountered no further restrictions on \(\theta\), the equivalence below holds for all \(x\) in \((-\infty, -1] \cup [1, \infty)\).\[\tan(\mathrm{arcsec}(x)) = \left\{ \begin{array}{rr} \sqrt{x^2-1}, & \text{if  } x \geq 1 \\
      \\
      -\sqrt{x^2-1}, & \text{if  } x \leq -1 \\
       \end{array}\right.\nonumber\]
   2. While I always use the graphing tactic, you do not have to think geometrically to work with inverse trigonometric functions. You can rely solely on identities.
      
      For example, to simplify \(\cos(\mathrm{arccsc}(4x))\), we start by letting \(\theta = \mathrm{arccsc}(4x)\). Then \(\csc(\theta) = 4x\) for \(\theta \in \left[-\frac{\pi}{2}, 0 \right) \cup \left(0, \frac{\pi}{2}\right]\).
      
      We now set about finding an expression for \(\cos(\mathrm{arccsc}(4x)) = \cos(\theta)\). Since \(\cos(\theta)\) is defined for all \(\theta\), we do not encounter any additional restrictions on \(\theta\). From \(\csc(\theta) = 4x\), we get \(\sin(\theta) = \frac{1}{4x}\), so to find \(\cos(\theta)\), we can make use of the Pythagorean Identity, \(\cos^{2}(\theta) + \sin^{2}(\theta) = 1\). Substituting \(\sin(\theta) = \frac{1}{4x}\) gives\[\cos^{2}(\theta) + \left(\frac{1}{4x}\right)^2 = 1.\nonumber \]Solving, we get\[\cos(\theta) = \pm \sqrt{\dfrac{16x^2-1}{16x^2}} = \pm \dfrac{\sqrt{16x^2-1}}{4|x|}.\nonumber\]Since \(\theta \in \left[-\frac{\pi}{2}, 0 \right) \cup \left(0, \frac{\pi}{2}\right]\), we know \(\cos(\theta) \geq 0\). Therefore, we choose\[\cos(\theta) = \dfrac{\sqrt{16-x^2}}{4|x|}.\nonumber \](The absolute values here are necessary, since \(x\) could be negative.) To find the values for which this equivalence is valid, we look back at our original substitution, \(\theta = \mathrm{arccsc}(4x)\). Since the domain of \(\mathrm{arccsc}(x)\) requires its argument \(x\) to satisfy \(|x| \geq 1\), the domain of \(\mathrm{arccsc}(4x)\) requires \(|4x| \geq 1\). This implies \(x \leq -\frac{1}{4}\) or \(x \geq \frac{1}{4}\). Since we had no additional restrictions on \(\theta\), the equivalence\[\cos(\mathrm{arccsc}(4x)) = \dfrac{\sqrt{16x^2-1}}{4|x|}\nonumber \]holds for all \(x\) in \(\left(-\infty, -\frac{1}{4} \right] \cup \left[\frac{1}{4}, \infty \right)\).

Checkpoint \(\PageIndex{2}\)

Rewrite the function as one that does not involve trigonometric functions and state the domain.\[ f(x) = \sin\left( \sec^{-1}\left( 2x \right) \right) \nonumber \](Your answer might be a piecewise function or it might involve absolute values.)

Answer

There are two ways to write this function: \[ f(x) = \dfrac{\sqrt{4x^2 - 1}}{2|x|},\nonumber \]or\[ f(x) = \left\{ \begin{array}{rcl}
\dfrac{\sqrt{4x^2 - 1}}{2x}, & \text{ if } & x \geq \dfrac{1}{2} \\
\\
-\dfrac{\sqrt{4x^2 - 1}}{2x}, & \text{ if } & x \lt -\dfrac{1}{2} \\
\end{array}\right. \nonumber \]The domain of this function is all \( x \in \left( -\infty, -\frac{1}{2} \right] \cup \left[ \frac{1}{2}, \infty \right) \).

Calculators and the Non-Fundamental Inverse Trigonometric Functions

In the sections to come, we will need to approximate the values of the inverse trigonometric functions. We have already discussed (in the previous section) how to use technology to approximate values of the arcsine, arccosine, and arctangent functions; however, if we are asked for an approximation of the arccotangent, arcsecant, or arccosecant, how is this to be done when our calculators do not have these functions?

Example \( \PageIndex{ 3 } \)

1. Use a calculator to approximate the following values to four decimal places.
   1. \(\cot^{-1}(2)\)
   2. \(\mathrm{arcsec}(5)\)
   3. \(\mathrm{arccot}(-2)\)
   4. \(\csc^{-1}\left(-\frac{3}{2}\right)\)
2. Find the domain and range of \[f(x) = \frac{\pi}{2} - \sec^{-1}\left(\dfrac{x}{5}\right).\nonumber \]

Solutions

1.  
   1. \( \cot^{-1}\left( 2 \right) = \theta \) means \( \cot\left( \theta \right) = 2 \), where \( \theta \in \left( 0,\pi \right) \).
      
      Quadrant: Since the cotangent is positive, we know \( \theta \in \left( 0,\frac{\pi}{2} \right) \).
      
      Reference Angle: \( 2 \) is not a special ratio for the cotangent, so we will need technology to help us find the reference angle; however, our calculators do not have an inverse cotangent button. What do we do? The answer is quite simple - we use the Reciprocal Identities. Since \( \cot\left( \theta \right) = 2\), \( \tan\left( \theta \right) = \frac{1}{2} \). To compute the reference angle, we evaluate\[ \hat{\theta} = \tan^{-1}\left( \left| \dfrac{1}{2} \right| \right) = \tan^{-1}\left( \dfrac{1}{2} \right) \approx 0.4636 \nonumber \]
      
      Now that we have a reference angle (\( \approx 0.4636 \)) and a quadrant (\( \mathrm{QII} \)), we get\[ \cot^{-1}\left( 2 \right) \approx 0.4636. \nonumber \]
   2. \( \mathrm{arcsec}\left( 5 \right) = \theta \) means \( \sec\left( \theta \right) = 5 \), where \( \theta \in \left[ 0,\frac{\pi}{2} \right) \cup \left( \frac{\pi}{2},\pi \right]\).
      
      Quadrant: Since the secant is positive, we further restrict the angle to \( \theta \in \left( 0,\frac{\pi}{2} \right) \) (Quadrant I).
      
      Reference Angle: Again, since our technology does not have an inverse secant button, we use the Reciprocal Identities.\[ \sec\left( \theta \right) = 5 \implies \cos\left( \theta \right) = \dfrac{1}{5}. \nonumber \]Now we use a calculator to compute\[ \hat{\theta} = \cos^{-1}\left( \left|\frac{1}{5}\right| \right) = \cos^{-1}\left( \frac{1}{5} \right) \approx 1.3694.\nonumber \]
      Using our reference angle (\( \approx 1.3694 \)) and quadrant (\( \mathrm{QI} \)), we get\[\mathrm{arcsec}(5) \approx 1.3694.\nonumber \]
   3. \(\mathrm{arccot}(-2) = \theta\) means \(\cot(\theta) = -2\), where \( \theta \in \left( 0,\pi \right) \).
      
      Quadrant: Since \(\cot(\theta) \lt 0\), we know \(\frac{\pi}{2} \lt \theta \lt \pi\). That is, \(\theta \in \mathrm{QII}\).
      
      Reference Angle: Once again, we do not have an appropriate inverse trigonometric function on the calculator, so we use the Reciprocal Identities.\[ \cot\left( \theta \right) = -2 \implies \tan\left( \theta \right) = -\dfrac{1}{2}. \nonumber \]Thus, we compute the reference angle as follows:\[ \hat{\theta} = \tan^{-1}\left( \left| -\dfrac{1}{2} \right| \right) = \tan^{-1}\left( \dfrac{1}{2} \right) \approx 0.4636. \nonumber \]
      
      We have a reference angle (\( \approx 0.4636 \)) and a quadrant (\( \mathrm{QII} \)). To find the actual angle, we subtract our reference angle from \( \pi \) to get\[\mathrm{arccot}\left( -2 \right) = \theta = \pi - \hat{\theta} = \pi - \arctan\left(\dfrac{1}{2}\right) \approx 2.6779.\nonumber \]
   4. \( \csc^{-1}\left( -\frac{3}{2} \right) = \theta \) means \( \csc\left( \theta \right) = -\frac{3}{2} \), where \( \theta \in \left[ -\frac{\pi}{2},0 \right) \cup \left( 0, \frac{\pi}{2} \right] \).
      
      Quadrant: Since \( \csc\left( \theta \right) \lt 0 \), we further restrict the angle to the interval \( \theta \in \left[ -\frac{\pi}{2},0 \right) \) (Quadrant IV).
      
      Reference Angle: We do not have an appropriate inverse trigonometric button on our calculator for the cosecant. Therefore, we must use the Reciprocal Identities.\[ \csc\left( \theta \right) = -\dfrac{3}{2} \implies \sin\left( \theta \right) = -\dfrac{2}{3}. \nonumber \]Hence,\[ \hat{\theta} = \sin^{-1}\left( \left| -\dfrac{2}{3} \right| \right) = \sin^{-1}\left( \dfrac{2}{3} \right) \approx 0.7297. \nonumber \]
      
      Now that we have a reference angle (\(\approx 0.7297  \)) and a quadrant (\( \mathrm{QIV} \)), we get\[ \csc^{-1}\left( -\dfrac{3}{2} \right) \approx -0.7297. \nonumber \]
2. The domain of the arcsecant is normally \( \left( -\infty,-1 \right] \cup \left[ 1,\infty \right) \). That is, if we were given \( \sec^{-1}\left( r \right) \), we know that allowable values of \( r \) are \( r \in \left( -\infty,-1 \right] \cup \left[ 1,\infty \right) \). In this case, however, the argument of the arcsecant is \( \frac{x}{5} \). Hence, \( \frac{x}{5} \in \left( -\infty,-1 \right] \cup \left[ 1,\infty \right) \). Rewriting this in interval notation will be helpful.\[ \begin{array}{rccccc}
   & \dfrac{x}{5} \leq -1 & \quad & \text{or} & \quad & \dfrac{x}{5} \geq 1 \\
   \implies & x \leq -5 & \quad & \text{or} & \quad & x \geq 5 \\
   \end{array}\nonumber \]Thus, the domain of \( f \) is \( \left( -\infty,-5 \right] \cup \left[ 5,\infty \right) \).
   
   We know the range of the arcsecant is normally \( \left[ 0,\frac{\pi}{2} \right) \cup \left( \frac{\pi}{2}, \pi \right] \). Given the function \( f(x) = \frac{\pi}{2} - \sec^{-1}\left(\frac{x}{5}\right) \), the only transformation that affects the range is the addition of \( \frac{\pi}{2} \) to the output values. Thus, the range of \( f \) is \( \left[ \frac{\pi}{2},\pi \right) \cup \left( \pi, \frac{3\pi}{2} \right] \).

Checkpoint \(\PageIndex{3}\)

Use a calculator to approximate \( \mathrm{arcsec}\left( -\frac{7}{3} \right) \).

Answer

\( \approx 2.0137 \)

The inverse trigonometric functions are typically found in applications whenever the measure of an angle is required. The following example presents one such scenario.

Example \( \PageIndex{ 4 } \)

The roof on the house below has a "\(6/12\) pitch." This means that when viewed from the side, the roof line has a rise of 6 feet over a run of 12 feet. Find the angle of inclination from the bottom of the roof to the top of the roof. Express your answer in decimal degrees, rounded to the nearest hundredth of a degree.

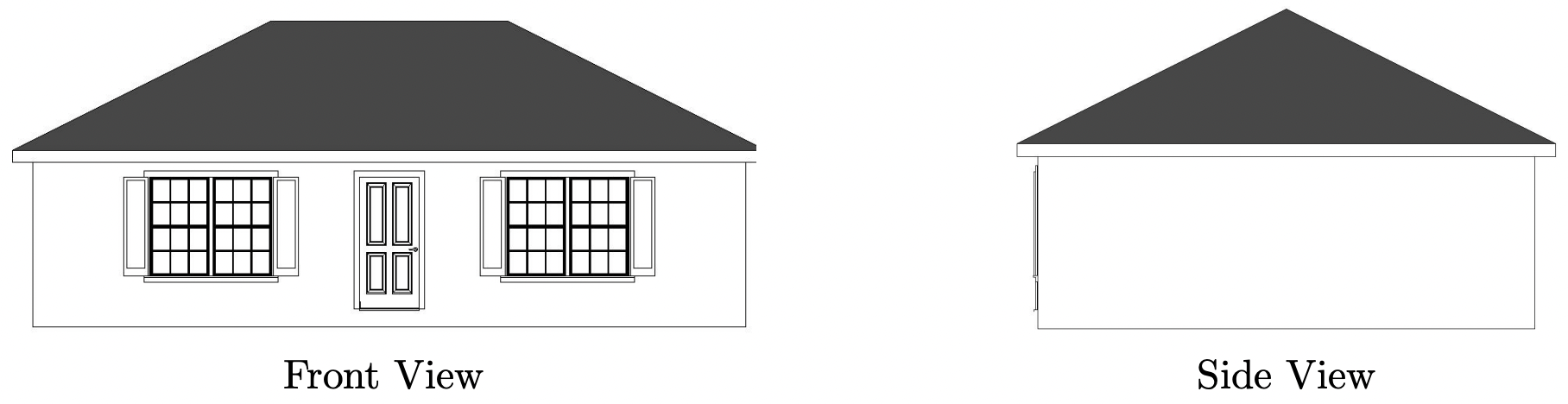
Solution

If we divide the side view of the house down the middle, we find that the roof line forms the hypotenuse of a right triangle with legs of length \(6\) feet and \(12\) feet. We find the angle of inclination, labeled \(\theta\) below, satisfies \(\tan(\theta) = \frac{6}{12} = \frac{1}{2}\). Since \(\theta\) is an acute angle, we can use the arctangent function and we find \(\theta = \arctan\left(\frac{1}{2}\right)\, \text{radians} \, \approx 26.56^{\circ}\).

---

Footnotes

¹ Again, I am mixing notations here. Very technically, the function \( \sin^{-1}(x) \) returns an angle and \( \mathrm{arcsin}(x) \) returns a real number. The same is true for all the inverse trigonometric functions. Therefore, it is valid for \( \cos^{-1}(x) \) to return an angle in degree measure or radian measure; however, it is technically not valid for \( \mathrm{arccos}(x) \) to return a number in degree measure (as this is an angle). Since radians is a unitless measure, a radian is just a real number, so \( \mathrm{arccos}(x) \) can return a number in radian measure. The moral of the story is that you are always safe to work in radian measure when dealing with inverse trigonometric functions, but working in degree measure is a little risky.

² There are two ways to restrict the domains of the secant and cosecant functions. The method shown in this text aligns with all other texts in Trigonometry and Precalculus; however, there is a much better choice of domain restriction for these functions that makes Calculus (specifically, Integral Calculus) work much more smoothly. Despite this, we give the traditional definition here because it aligns with all of our previous work.

---

Homework

Basic Skills

For Problems 1 - 23, find the exact value.

1. \(\mathrm{arccot} \left( \sqrt{3} \right)\)

2. \(\mathrm{arccot} \left( \dfrac{1}{\sqrt{3}} \right)\)

3. \(\mathrm{arcsec} \left( \sqrt{2} \right)\)

4. \(\mathrm{arccsc} \left( \sqrt{2} \right)\)

5. \(\mathrm{arcsec} \left( \dfrac{2}{\sqrt{3}} \right)\)

6. \(\mathrm{arccsc} \left( \dfrac{2}{\sqrt{3}} \right)\)

7. \(\mathrm{arcsec} \left( 2 \right)\)

8. \(\mathrm{arccsc} \left( 2 \right)\)

9. \(\mathrm{arccot} \left( 1 \right)\)

10. \(\mathrm{arcsec} \left( 1 \right)\)

11. \(\mathrm{arccsc} \left( 1 \right)\)

12. \(\mathrm{arccot} \left( 0 \right)\)

13. \(\mathrm{arccot} \left( -\sqrt{3} \right)\)

14. \(\mathrm{arccot} \left( -\dfrac{1}{\sqrt{3}} \right)\)

15. \(\mathrm{arcsec} \left( -2 \right)\)

16. \(\mathrm{arccsc} \left( -2 \right)\)

17. \(\mathrm{arcsec} \left( -\sqrt{2} \right)\)

18. \(\mathrm{arccsc} \left( -\sqrt{2} \right)\)

19. \(\mathrm{arcsec} \left( -\dfrac{2}{\sqrt{3}} \right)\)

20. \(\mathrm{arccsc} \left( -\dfrac{2}{\sqrt{3}} \right)\)

21. \(\mathrm{arcsec} \left( -1 \right)\)

22. \(\mathrm{arccot} \left( -1 \right)\)

23. \(\mathrm{arccsc} \left( -1 \right)\)

For Problems 24 - 38, find the exact value or state that it is undefined.

24. \(\cot\left(\mathrm{arccot}\left(1\right)\right)\)

25. \(\cot\left(\mathrm{arccot}\left(-\sqrt{3}\right)\right)\)

26. \(\cot\left(\mathrm{arccot}\left(-\dfrac{7}{24}\right)\right)\)

27. \(\cot\left(\mathrm{arccot}\left(-0.001\right)\right)\)

28. \(\cot\left(\mathrm{arccot}\left( \dfrac{17\pi}{4} \right)\right)\)

29. \(\sec\left(\mathrm{arcsec}\left(2\right)\right)\)

30. \(\sec\left(\mathrm{arcsec}\left(-1\right)\right)\)

31. \(\sec\left(\mathrm{arcsec}\left(\dfrac{1}{2}\right)\right)\)

32. \(\sec\left(\mathrm{arcsec}\left(0.75\right)\right)\)

33. \(\sec\left(\mathrm{arcsec}\left( 117\pi \right)\right)\)

34. \(\csc\left(\mathrm{arccsc}\left(\sqrt{2}\right)\right)\)

35. \(\csc\left(\mathrm{arccsc}\left(-\dfrac{2\sqrt{3}}{3}\right)\right)\)

36. \(\csc\left(\mathrm{arccsc}\left(\dfrac{\sqrt{2}}{2}\right)\right)\)

37. \(\csc\left(\mathrm{arccsc}\left(1.0001\right)\right)\)

38. \(\csc\left(\mathrm{arccsc}\left( \dfrac{\pi}{4} \right)\right)\)

For Problems 39 - 55, find the exact value or state that it is undefined.

39. \(\mathrm{arccsc}\left(\csc\left(\dfrac{\pi}{6}\right) \right)\)

40. \(\mathrm{arccot}\left(\cot\left(\dfrac{\pi}{3}\right) \right)\)

41. \(\mathrm{arccot}\left(\cot\left(-\dfrac{\pi}{4}\right) \right)\)

42. \(\mathrm{arcsec}\left(\sec\left(\dfrac{\pi}{4}\right) \right)\)

43. \(\mathrm{arccot}\left(\cot\left(\dfrac{\pi}{2}\right) \right)\)

44. \(\mathrm{arcsec}\left(\sec\left(-\dfrac{\pi}{2} \right) \right)\)

45. \(\mathrm{arccsc}\left(\csc\left(-\dfrac{\pi}{2} \right) \right)\)

46. \(\mathrm{arccot}\left(\cot\left(\pi\right) \right)\)

47. \(\mathrm{arccot}\left(\cot\left(\dfrac{2\pi}{3}\right) \right)\)

48. \(\mathrm{arccsc}\left(\csc\left( \dfrac{2\pi}{3} \right) \right)\)

49. \(\mathrm{arcsec}\left(\sec\left( \dfrac{5\pi}{6} \right) \right)\)

50. \(\mathrm{arccsc}\left(\csc\left(\dfrac{5\pi}{4}\right) \right)\)

51. \(\mathrm{arcsec}\left(\sec\left(\dfrac{4\pi}{3}\right) \right)\)

52. \(\mathrm{arcsec}\left(\sec\left(\dfrac{5\pi}{3}\right) \right)\)

53. \(\mathrm{arccsc}\left(\csc\left(\dfrac{11\pi}{6}\right) \right)\)

54. \(\mathrm{arcsec}\left(\sec\left(\dfrac{11\pi}{12}\right) \right)\)

55. \(\mathrm{arccsc}\left(\csc\left(\dfrac{9\pi}{8}\right) \right)\)

In Exercises 56 - 64, find the exact value or state that it is undefined.

56. \(\sin\left(\mathrm{arccot}\left(\sqrt{5}\right)\right)\)

57. \(\sin\left(\mathrm{arccsc}\left(-3\right)\right)\)

58. \(\cos\left(\mathrm{arccot}\left( 3 \right)\right)\)

59. \(\cos\left(\mathrm{arcsec}\left( 5 \right)\right)\)

60. \(\tan\left(\mathrm{arcsec}\left(\dfrac{5}{3}\right)\right)\)

61. \(\tan\left(\mathrm{arccot}\left( 12 \right)\right)\)

62. \(\cot\left(\mathrm{arccsc}\left(\sqrt{5}\right)\right)\)

63. \(\sec\left(\mathrm{arccot}\left(-\dfrac{\sqrt{10}}{10}\right)\right)\)

64. \(\csc\left(\mathrm{arccot}\left(9 \right)\right)\)

Synthesis Questions

In Exercises 65 - 68, find the exact value or state that it is undefined.

65. \(\cos\left( \mathrm{arcsec}(3) + \arctan(2) \right)\)

66. \(\sin\left(2\mathrm{arccsc}\left(\dfrac{13}{5}\right)\right)\)

67. \(\cos\left(2 \mathrm{arcsec}\left(\dfrac{25}{7}\right)\right)\)

68. \(\cos\left(2 \mathrm{arccot}\left(-\sqrt{5}\right)\right)\)

For Problems 69 - 75, find the domain of the given function. Write your answers in interval notation.

69. \(f(x) = \mathrm{arccot}\left(\dfrac{2x}{x^2-9}\right)\)

70. \(f(x) =\arctan(\ln(2x-1))\)

71. \(f(x) = \mathrm{arccot}(\sqrt{2x-1})\)

72. \(f(x) = \mathrm{arcsec}(12x)\)

73. \(f(x) = \mathrm{arccsc}(x+5)\)

74. \(f(x) = \mathrm{arcsec}\left(\dfrac{x^3}{8}\right)\)

75. \(f(x) = \mathrm{arccsc}\left(e^{2x}\right)\)

Applications

76. A guy wire 1000 feet long is attached to the top of a tower. When pulled taut, it touches level ground 360 feet from the tower's base. What angle does the wire make with the ground? Express your answer using degree measure rounded to one decimal place.

77. At Cliffs of Insanity Point, The Great Sasquatch Canyon is 7117 feet deep. From that point, a fire is seen at a location known to be 10 miles away from the base of the sheer canyon wall. What angle of depression is made by the line of sight from the canyon edge to the fire? Express your answer using degree measure rounded to one decimal place.

78. Shelving is being built at the Utility Muffin Research Library, which is to be 14 inches deep. An 18-inch rod will be attached to the wall and the underside of the shelf at its edge away from the wall, forming a right triangle under the shelf to support it. What angle, to the nearest degree, will the rod make with the wall?

79. A parasailor is being pulled by a boat on Lake Ippizuti. The cable is 300 feet long, and the parasailor is 100 feet above the surface of the water. What is the angle of elevation from the boat to the parasailor? Express your answer using degree measure rounded to one decimal place.

80. A tag-and-release program to study the Sasquatch population of the eponymous Sasquatch National Park has begun. From a 200-foot-tall tower, a ranger spots a Sasquatch lumbering through the wilderness directly toward the tower. Let \(\theta\) denote the angle of depression from the top of the tower to a point on the ground. If the range of the rifle with a tranquilizer dart is 300 feet, find the smallest value of \(\theta\) for which the corresponding point on the ground is in range of the rifle. Round your answer to the nearest hundredth of a degree.

Challenge Problems

81. Show that \(\mathrm{arcsec}(x) = \arccos \left( \dfrac{1}{x} \right)\) for \(|x| \geq 1\).

82. Show that \(\mathrm{arccsc}(x) = \arcsin \left( \dfrac{1}{x} \right)\) for \(|x| \geq 1\).