Equations of Lines and Planes in Space
- Last updated
- Save as PDF
- Page ID
- 20991
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Learning Objectives
- Write the vector, parametric, and symmetric equations of a line through a given point in a given direction, and a line through two given points.
- Find the distance from a point to a given line.
- Write the vector and scalar equations of a plane through a given point with a given normal.
- Find the distance from a point to a given plane.
- Find the angle between two planes.
By now, we are familiar with writing equations that describe a line in two dimensions. To write an equation for a line, we must know two points on the line, or we must know the direction of the line and at least one point through which the line passes. In two dimensions, we use the concept of slope to describe the orientation, or direction, of a line. In three dimensions, we describe the direction of a line using a vector parallel to the line. In this section, we examine how to use equations to describe lines and planes in space.
Equations for a Line in Space
Let’s first explore what it means for two vectors to be parallel. Recall that parallel vectors must have the same or opposite directions. If two nonzero vectors, \( \vecs{u}\) and \( \vecs{v}\), are parallel, we claim there must be a scalar, \( k\), such that \( \vecs{u}=k\vecs{v}\). If \( \vecs{u}\) and \( \vecs{v}\) have the same direction, simply choose
\[ k=\dfrac{‖\vecs{u}‖}{‖\vecs{v}‖}.\]
If \( \vecs{u}\) and \( \vecs{v}\) have opposite directions, choose
\[ k=−\dfrac{‖\vecs{u}‖}{‖\vecs{v}‖}.\]
Note that the converse holds as well. If \( \vecs{u}=k \vecs{v}\) for some scalar \( k\), then either \( \vecs{u}\) and \(\vecs{ v}\) have the same direction \( (k>0)\) or opposite directions \( (k<0)\), so \( \vecs{u}\) and \( \vecs{v}\) are parallel. Therefore, two nonzero vectors \( \vecs{u}\) and \(\vecs{ v}\) are parallel if and only if \( \vecs{u}=k\vecs{v}\) for some scalar \( k\). By convention, the zero vector \( \vecs{0}\) is considered to be parallel to all vectors.
Figure \(\PageIndex{1}\): Vector \(\vecs{v}\) is the direction vector for \( \vecd{PQ}\).
As in two dimensions, we can describe a line in space using a point on the line and the direction of the line, or a parallel vector, which we call the direction vector (Figure \(\PageIndex{1}\)). Let \( L\) be a line in space passing through point \( P(x_0,y_0,z_0)\). Let \( \vecs{v}=⟨a,b,c⟩\) be a vector parallel to \( L\). Then, for any point on line \( Q(x,y,z)\), we know that \( \vecd{PQ}\) is parallel to \( \vecs{v}\). Thus, as we just discussed, there is a scalar, \( t\), such that \( \vecd{PQ}=t\vecs{v}\), which gives
\[ \begin{align} \vecd{PQ}&=t\vecs{v} \nonumber \\[5pt] ⟨x−x_0,y−y_0,z−z_0⟩ &=t⟨a,b,c⟩ \nonumber \\[5pt] ⟨x−x_0,y−y_0,z−z_0⟩&=⟨ta,tb,tc⟩. \label{eq1} \end{align}\]
Using vector operations, we can rewrite Equation \ref{eq1}
\[ \begin{align*} ⟨x−x_0,y−y_0,z−z_0⟩&=⟨ta,tb,tc⟩ \\[5pt] ⟨x,y,z⟩−⟨x_0,y_0,z_0⟩&=t⟨a,b,c⟩ \\[5pt] \underbrace{⟨x,y,z⟩}_{\vecs{r}} &=\underbrace{⟨x_0,y_0,z_0⟩}_{\vecs{r}_o}+t\underbrace{⟨a,b,c⟩}_{\vecs{v}}.\end{align*}\]
Setting \( \vecs{r}=⟨x,y,z⟩\) and \( \vecs{r}_0=⟨x_0,y_0,z_0⟩\), we now have the vector equation of a line:
\[ \vecs{r}=\vecs{r}_0+t\vecs{v}. \label{vector}\]
Equating components, Equation \ref{vector} shows that the following equations are simultaneously true: \( x−x_0=ta, y−y_0=tb,\) and \( z−z_0=tc.\) If we solve each of these equations for the component variables \( x,y,\) and \( z\), we get a set of equations in which each variable is defined in terms of the parameter \(t\) and that, together, describe the line. This set of three equations forms a set of parametric equations of a line:
\[ x=x_0+ta \nonumber\]
\[ y=y_0+tb \nonumber\]
\[ z=z_0+tc.\nonumber\]
If we solve each of the equations for \( t\) assuming \( a,b\), and \( c\) are nonzero, we get a different description of the same line:
\[ \begin{align*} \dfrac{x−x_0}{a}&=t \\[5pt] \dfrac{y−y_0}{b}&=t \\[5pt] \dfrac{z−z_0}{c}&=t.\end{align*}\]
Because each expression equals \(t\), they all have the same value. We can set them equal to each other to create symmetric equations of a line:
\[\dfrac{x−x_0}{a}=\dfrac{y−y_0}{b}=\dfrac{z−z_0}{c}. \nonumber\]
We summarize the results in the following theorem.
Theorem: Parametric and Symmetric Equations of a Line
A line \( L\) parallel to vector \( \vecs{v}=⟨a,b,c⟩\) and passing through point \( P(x_0,y_0,z_0)\) can be described by the following parametric equations:
\[ x=x_0+ta, y=y_0+tb,\]
and
\[ z=z_0+tc.\]
If the constants \( a,b,\) and \( c\) are all nonzero, then \( L\) can be described by the symmetric equation of the line:
\[\dfrac{x−x_0}{a}=\dfrac{y−y_0}{b}=\dfrac{z−z_0}{c}.\]
The parametric equations of a line are not unique. Using a different parallel vector or a different point on the line leads to a different, equivalent representation. Each set of parametric equations leads to a related set of symmetric equations, so it follows that a symmetric equation of a line is not unique either.
Example \( \PageIndex{1}\): Equations of a Line in Space
Find parametric and symmetric equations of the line passing through points \( (1,4,−2)\) and \( (−3,5,0).\)
Solution
First, identify a vector parallel to the line:
\[ \vecs v=⟨−3−1,5−4,0−(−2)⟩=⟨−4,1,2⟩. \nonumber\]
Use either of the given points on the line to complete the parametric equations:
\[\begin{align*} x&=1−4t \\[5pt] y&=4+t, \end{align*}\]
and
\[ z=−2+2t. \nonumber\]
Solve each equation for \( t\) to create the symmetric equation of the line:
\[ \dfrac{x−1}{−4}=y−4=\dfrac{z+2}{2}. \nonumber\]
Exercise \( \PageIndex{1}\)
Find parametric and symmetric equations of the line passing through points \( (1,−3,2)\) and \( (5,−2,8).\)
- Hint:
-
Start by finding a vector parallel to the line.
- Answer
-
Possible set of parametric equations: \( x=1+4t,y=−3+t,z=2+6t;\) related set of symmetric equations: \[ \dfrac{x−1}{4}=y+3=\dfrac{z−2}{6} \nonumber\]
Sometimes we don’t want the equation of a whole line, just a line segment. In this case, we limit the values of our parameter \( t\). For example, let \( P(x_0,y_0,z_0)\) and \( Q(x_1,y_1,z_1)\) be points on a line, and let \( \vecs p=⟨x_0,y_0,z_0⟩\) and \( \vecs q=⟨x_1,y_1,z_1⟩\) be the associated position vectors. In addition, let \(\vecs r=⟨x,y,z⟩\). We want to find a vector equation for the line segment between \( P\) and \( Q\). Using \( P\) as our known point on the line, and \( \vecd{PQ}=⟨x_1−x_0,y_1−y_0,z_1−z_0⟩\) as the direction vector equation, Equation \ref{vector} gives
\[\vecs{r}=\vecs{p}+t(\vecd{PQ}). \label{eq10}\]
Equation \ref{eq10} can be expanded using properties of vectors:
\[ \begin{align*} \vecs{r}&=\vecs{p}+t(\vecd{PQ}) \\[5pt] &=⟨x_0,y_0,z_0⟩+t⟨x_1−x_0,y_1−y_0,z_1−z_0⟩ \\[5pt] &=⟨x_0,y_0,z_0⟩+t(⟨x_1,y_1,z_1⟩−⟨x_0,y_0,z_0⟩) \\[5pt] &=⟨x_0,y_0,z_0⟩+t⟨x_1,y_1,z_1⟩−t⟨x_0,y_0,z_0⟩ \\[5pt] &=(1−t)⟨x_0,y_0,z_0⟩+t⟨x_1,y_1,z_1⟩ \\[5pt] &=(1−t)\vecs{p}+t\vecs{q}. \end{align*}\]
Thus, the vector equation of the line passing through \( P\) and \( Q\) is
\[\vecs{r}=(1−t)\vecs{p}+t\vecs{q}.\]
Remember that we did not want the equation of the whole line, just the line segment between \( P\) and \( Q\). Notice that when \( t=0\), we have \(\vecs r= \vecs p\), and when \( t=1\), we have \( \vecs r=\vecs q\). Therefore, the vector equation of the line segment between \( P\) and \( Q\) is
\[\vecs{r}=(1−t)\vecs{p}+t\vecs{q},0≤t≤1.\]
Going back to Equation \ref{vector}, we can also find parametric equations for this line segment. We have
\[ \begin{align*} \vecs{r}&=\vecs{p}+t(\vecd{PQ}) \\[5pt] ⟨x,y,z⟩&=⟨x_0,y_0,z_0⟩+t⟨x_1−x_0,y_1−y_0,z_1−z_0⟩\\[5pt] &=⟨x_0+t(x_1−x_0),y_0+t(y_1−y_0),z_0+t(z_1−z_0)⟩. \end{align*}\]
Then, the parametric equations are
\[ \begin{align} x&=x_0+t(x_1−x_0) \nonumber \\[5pt] y&=y_0+t(y_1−y_0) \nonumber\\[5pt] z &=z_0+t(z_1−z_0),\,0≤t≤1. \nonumber \end{align} \label{para}\]
Example \( \PageIndex{2}\): Parametric Equations of a Line Segment
Find parametric equations of the line segment between the points \( P(2,1,4)\) and \( Q(3,−1,3).\)
Solution
Start with the parametric equations for a line (Equations \ref{para}) and work with each component separately:
\[ \begin{align*} x&=x_0+t(x_1−x_0)\\[5pt] &=2+t(3−2)\\[5pt] &=2+t, \end{align*}\]
\[ \begin{align*} y&=y_0+t(y_1−y_0)\\[5pt] &=1+t(−1−1)\\[5pt] &=1−2t, \end{align*}\]
and
\[ \begin{align*} z&=z_0+t(z_1−z_0)\\[5pt] &=4+t(3−4)\\[5pt] &=4−t. \end{align*}\]
Therefore, the parametric equations for the line segment are
\[ \begin{align*} x&=2+t\\[5pt] y&=1−2t\\[5pt] z&=4−t,\,0≤t≤1.\end{align*}\]
Exercise \( \PageIndex{2}\)
Find parametric equations of the line segment between points \( P(−1,3,6)\) and \( Q(−8,2,4)\).
- Answer
-
\[ x=−1−7t,y=3−t,z=6−2t,0≤t≤1 \nonumber\]
Distance between a Point and a Line
We already know how to calculate the distance between two points in space. We now expand this definition to describe the distance between a point and a line in space. Several real-world contexts exist when it is important to be able to calculate these distances. When building a home, for example, builders must consider “setback” requirements, when structures or fixtures have to be a certain distance from the property line. Air travel offers another example. Airlines are concerned about the distances between populated areas and proposed flight paths.
Let \( L\) be a line in the plane and let \( M\) be any point not on the line. Then, we define distance \( d\) from \( M\) to \( L\) as the length of line segment \( \overline{MP}\), where \( P\) is a point on \( L\) such that \( \overline{MP}\) is perpendicular to \( L\) (Figure \(\PageIndex{2}\)).
When we’re looking for the distance between a line and a point in space, Figure \(\PageIndex{2}\) still applies. We still define the distance as the length of the perpendicular line segment connecting the point to the line. In space, however, there is no clear way to know which point on the line creates such a perpendicular line segment, so we select an arbitrary point on the line and use properties of vectors to calculate the distance. Therefore, let \( P\) be an arbitrary point on line \( L\) and let \(\vecs{v}\) be a direction vector for \( L\) (Figure \(\PageIndex{3}\)).
Vectors \( \vecd{PM}\) and \(\vecs{v}\) form two sides of a parallelogram with area \( ‖\vecd{PM}×\vecs{v}‖\). Using a formula from geometry, the area of this parallelogram can also be calculated as the product of its base and height:
\[‖\vecd{PM}×\vecs{v}‖=‖\vecs v‖d.\]
We can use this formula to find a general formula for the distance between a line in space and any point not on the line.
Distance from a Point to a Line
Let \( L\) be a line in space passing through point \( P\) with direction vector \(\vecs{v}\). If \( M\) is any point not on \( L\), then the distance from \( M\) to \( L\) is
\[d=\dfrac{‖\vecd{PM}×\vecs{v}‖}{‖\vecs{v}‖}.\]
Example \( \PageIndex{3}\): Calculating the Distance from a Point to a Line
Find the distance between the point \( M=(1,1,3)\) and line \( \dfrac{x−3}{4}=\dfrac{y+1}{2}=z−3.\)
Solution:
From the symmetric equations of the line, we know that vector \( \vecs{v}=⟨4,2,1⟩\) is a direction vector for the line. Setting the symmetric equations of the line equal to zero, we see that point \( P(3,−1,3)\) lies on the line. Then,
\[\begin{align*} \vecd{PM}&=⟨1−3,1−(−1),3−3⟩\\[5pt] &=⟨−2,2,0⟩. \end{align*}\]
To calculate the distance, we need to find \( \vecd{PM}×\vecs v:\)
\[\begin{align*} \vecd{PM}×\vecs{v}&=\begin{vmatrix}\mathbf{\hat i}&\mathbf{\hat j}&\mathbf{\hat k}\\−2&2&0\\4&2&1\end{vmatrix} \\[5pt] &=(2−0)\mathbf{\hat i}−(−2−0)\mathbf{\hat j}+(−4−8)\mathbf{\hat k} \\[5pt] &=2\mathbf{\hat i}+2\mathbf{\hat j}−12\mathbf{\hat k}. \end{align*}\]
Therefore, the distance between the point and the line is (Figure \(\PageIndex{4}\))
\[\begin{align*} d&=\dfrac{‖\vecd{PM}×\vecs{v}‖}{‖\vecs{v}‖} \\[5pt] &=\dfrac{\sqrt{2^2+2^2+12^2}}{\sqrt{4^2+2^2+1^2}}\\[5pt] &=\dfrac{2\sqrt{38}}{\sqrt{21}}\\[5pt] &=\dfrac{2\sqrt{798}}{21} \,\text{units} \end{align*}\]
Exercise \( \PageIndex{3}\)
Find the distance between point \( (0,3,6)\) and the line with parametric equations \( x=1−t,y=1+2t,z=5+3t.\)
- Hint
-
Find a vector with initial point \( (0,3,6)\) and a terminal point on the line, and then find a direction vector for the line.
- Answer
-
\[ \sqrt{\dfrac{10}{7}} = \dfrac{\sqrt{70}}{7} \,\text{units} \nonumber\]
Relationships between Lines
Given two lines in the two-dimensional plane, the lines are equal, they are parallel but not equal, or they intersect in a single point. In three dimensions, a fourth case is possible. If two lines in space are not parallel, but do not intersect, then the lines are said to be skew lines (Figure \(\PageIndex{5}\)).
Figure \(\PageIndex{5}\): In three dimensions, it is possible that two lines do not cross, even when they have different directions.
To classify lines as parallel but not equal, equal, intersecting, or skew, we need to know two things: whether the direction vectors are parallel and whether the lines share a point (Figure \(\PageIndex{6}\)).
Example \( \PageIndex{4}\): Classifying Lines in Space
For each pair of lines, determine whether the lines are equal, parallel but not equal, skew, or intersecting.
a.
- \( L_1:x=2s−1,y=s−1,z=s−4\)
- \( L_2:x=t−3,y=3t+8,z=5−2t\)
b.
- \( L_1: x=−y=z\)
- \( L_2:\dfrac{x−3}{2}=y=z−2\)
c.
- \( L_1:x=6s−1,y=−2s,z=3s+1\)
- \( L_2:\dfrac{x−4}{6}=\dfrac{y+3}{−2}=\dfrac{z−1}{3}\)
Solution
a. Line \( L_1\) has direction vector \( \vecs v_1=⟨2,1,1⟩\); line \( L_2\) has direction vector \( \vecs v_2=⟨1,3,−2⟩\). Because the direction vectors are not parallel vectors, the lines are either intersecting or skew. To determine whether the lines intersect, we see if there is a point, \( (x,y,z)\), that lies on both lines. To find this point, we use the parametric equations to create a system of equalities:
\[ 2s−1=t−3;\]
\[ s−1=3t+8;\]
\[ s−4=5−2t.\]
By the first equation, \( t=2s+2.\) Substituting into the second equation yields
\( s−1=3(2s+2)+8\)
\( s−1=6s+6+8\)
\( 5s=−15\)
\( s=−3.\)
Substitution into the third equation, however, yields a contradiction:
\( s−4=5−2(2s+2)\)
\( s−4=5−4s−4\)
\( 5s=5\)
\( s=1.\)
There is no single point that satisfies the parametric equations for \( L_1\) and \( L_2\) simultaneously. These lines do not intersect, so they are skew (see the following figure).
b. Line \( L_1\) has direction vector \( \vecs v_1=⟨1,−1,1⟩\) and passes through the origin, \( (0,0,0)\). Line \( L_2\) has a different direction vector, \( \vecs v_2=⟨2,1,1⟩\), so these lines are not parallel or equal. Let \( r\) represent the parameter for line \( L_1\) and let \(s\) represent the parameter for \( L_2\):
\[\begin{align*} &\text{Line }L_1: & & \text{Line }L_2:\\[4pt] &x = r & & x = 2s + 3\\[4pt] &y = -r & & y = s \\[4pt] &z = r & & z = s + 2 \end{align*}\]
Solve the system of equations to find \( r=1\) and \( s=−1\). If we need to find the point of intersection, we can substitute these parameters into the original equations to get \( (1,−1,1)\) (see the following figure).
c. Lines \( L_1\) and \( L_2\) have equivalent direction vectors: \( \vecs v=⟨6,−2,3⟩.\) These two lines are parallel (see the following figure).
Exercise \( \PageIndex{4}\)
Describe the relationship between the lines with the following parametric equations:
\[ x=1−4t,y=3+t,z=8−6t \nonumber\]
\[x=2+3s,y=2s,z=−1−3s. \nonumber\]
- Hint
-
Start by identifying direction vectors for each line. Is one a multiple of the other?
- Answer
-
These lines are skew because their direction vectors are not parallel and there is no point \( (x,y,z)\) that lies on both lines.
Equations for a Plane
We know that a line is determined by two points. In other words, for any two distinct points, there is exactly one line that passes through those points, whether in two dimensions or three. Similarly, given any three points that do not all lie on the same line, there is a unique plane that passes through these points. Just as a line is determined by two points, a plane is determined by three.
This may be the simplest way to characterize a plane, but we can use other descriptions as well. For example, given two distinct, intersecting lines, there is exactly one plane containing both lines. A plane is also determined by a line and any point that does not lie on the line. These characterizations arise naturally from the idea that a plane is determined by three points. Perhaps the most surprising characterization of a plane is actually the most useful.
Imagine a pair of orthogonal vectors that share an initial point. Visualize grabbing one of the vectors and twisting it. As you twist, the other vector spins around and sweeps out a plane. Here, we describe that concept mathematically. Let \(\vecs{n}=⟨a,b,c⟩\) be a vector and \(P=(x_0,y_0,z_0)\) be a point. Then the set of all points \(Q=(x,y,z)\) such that \(\vecd{PQ}\) is orthogonal to \(\vecs{n}\) forms a plane (Figure \(\PageIndex{7}\)). We say that \(\vecs{n}\) is a normal vector, or perpendicular to the plane. Remember, the dot product of orthogonal vectors is zero. This fact generates the vector equation of a plane:
\[\vecs{n}⋅\vecd{PQ}=0.\]
Rewriting this equation provides additional ways to describe the plane:
\[ \begin{align*} \vecs{n}⋅\vecd{PQ}&=0 \\[5pt] ⟨a,b,c⟩⋅⟨x−x_0,y−y_0,z−z_0⟩&=0 \\[5pt] a(x−x_0)+b(y−y_0)+c(z−z_0)&=0. \end{align*}\]
Definition: Standard Form of the Equation of a Plane
Given a point \(P\) and vector \(\vecs n\), the set of all points \(Q\) satisfying the equation \(\vecs n⋅\vecd{PQ}=0\) forms a plane. The equation
\[\vecs{n}⋅\vecd{PQ}=0 \nonumber\]
is known as the vector equation of a plane.
The standard form of the equation of a plane containing point \(P=(x_0,y_0,z_0)\) with normal vector \(\vec{n}=⟨a,b,c⟩\) is
\[a(x−x_0)+b(y−y_0)+c(z−z_0)=0. \nonumber\]
This equation can be expressed as \(ax+by+cz+d=0,\) where \(d=−ax_0−by_0−cz_0.\) This form of the equation is sometimes called the general form of the equation of a plane.
As the standard and general forms of the equation of a plane no longer contain an explicit vector, they are sometimes called scalar equations of a plane.
As described earlier in this section, any three points that do not all lie on the same line determine a plane. Given three such points, we can find an equation for the plane containing these points.
Example \(\PageIndex{5}\): Writing an Equation of a Plane Given Three Points in the Plane
Write an equation for the plane containing points \(P=(1,1,−2), Q=(0,2,1),\) and \(R=(−1,−1,0)\) in both standard and general forms.
Solution
To write an equation for a plane, we must find a normal vector for the plane. We start by identifying two vectors in the plane:
\[ \begin{align*} \vecd{PQ}&=⟨0−1,2−1,1−(−2)⟩\\[5pt] &=⟨−1,1,3⟩ \\[5pt] \vecd{QR}&=⟨−1−0,−1−2,0−1⟩\\[5pt] &=⟨−1,−3,−1⟩.\end{align*}\]
The cross product \(\vecd{PQ}×\vecd{QR}\) is orthogonal to both \(\vecd{PQ}\) and \(\vecd{QR}\), so it is normal to the plane that contains these two vectors:
\[ \begin{align*} \vecs n&=\vecd{PQ}×\vecd{QR} \\[5pt] &=\begin{vmatrix}\hat{\mathbf{i}}&\hat{\mathbf{j}}&\hat{\mathbf{k}}\\−1&1&3\\−1&−3&−1\end{vmatrix} \\[5pt] &=(−1+9)\hat{\mathbf{i}}−(1+3)\hat{\mathbf{j}}+(3+1)\hat{\mathbf{k}} \\[5pt] &= 8\hat{\mathbf{i}}−4\hat{\mathbf{j}}+4\hat{\mathbf{k}}.\end{align*}\]
Thus, \(\vecs n=⟨8,−4,4⟩,\) and we can choose any of the three given points to write an equation of the plane:
\[ \begin{align*} 8(x−1)−4(y−1)+4(z+2)&=0 \quad \text{(Standard form)}\\[5pt] 8x−4y+4z+4 &=0. \quad \text{(General form)} \end{align*}\]
The scalar equations of a plane vary depending on the normal vector and point chosen.
Example \(\PageIndex{6}\): Writing an Equation for a Plane Given a Point and a Line
Find an equation of the plane that passes through point \((1,4,3)\) and contains the line given by \(x=\dfrac{y−1}{2}=z+1.\)
Solution
Symmetric equations describe the line that passes through point \((0,1,−1)\) parallel to vector \(\vecs v_1=⟨1,2,1⟩\) (see the following figure). Use this point and the given point, \((1,4,3),\) to identify a second vector parallel to the plane:
\[ \vecs v_2=⟨1−0,4−1,3−(−1)⟩=⟨1,3,4⟩. \nonumber\]
Use the cross product of these vectors to identify a normal vector for the plane:
\[ \begin{align*} \vecs n&=\vecs v_1×\vecs v_2 \nonumber \\[5pt] & =\begin{vmatrix}\hat{\mathbf{i}}&\hat{\mathbf{j}}&\hat{\mathbf{k}}\\1&2&1\\1&3&4\end{vmatrix} \nonumber \\[5pt] &=(8−3)\hat{\mathbf{i}}−(4−1)\hat{\mathbf{j}}+(3−2)\hat{\mathbf{k}} \\[5pt] &=5\hat{\mathbf{i}}−3\hat{\mathbf{j}}+\hat{\mathbf{k}}. \nonumber\end{align*} \nonumber\]
The scalar equations for the plane are \(5x−3(y−1)+(z+1)=0\) and \(5x−3y+z+4=0.\)
Exercise \(\PageIndex{6}\)
Find an equation of the plane containing the lines \(L_1\) and \(L_2\):
\[ L_1:x=−y=z \nonumber\]
\[ L_2:\dfrac{x−3}{2}=y=z−2. \nonumber\]
- Hint
-
Hint: The cross product of the lines’ direction vectors gives a normal vector for the plane.
- Answer:
-
\[ −2(x−1)+(y+1)+3(z−1)=0 \quad \text{(Standard form)} \nonumber\]
\[ −2x+y+3z=0 \quad \quad \text{(General form)} \nonumber\]
Distance Between a Plane and a Point
Now that we can write an equation for a plane, we can use the equation to find the distance \(d\) between a point \(P\) and the plane. It is defined as the shortest possible distance from \(P\) to a point on the plane.
Just as we find the two-dimensional distance between a point and a line by calculating the length of a line segment perpendicular to the line, we find the three-dimensional distance between a point and a plane by calculating the length of a line segment perpendicular to the plane. Let \(R\) be the point in the plane such that \(\vecd{RP}\) is orthogonal to the plane, and let \(Q\) be an arbitrary point in the plane. Then the projection of vector \(\vecd{QP}\) onto the normal vector describes vector \(\vecd{RP}\), as shown in Figure \(\PageIndex{8}\).
The Distance between a Plane and a Point
Suppose a plane with normal vector \(\vecs{n}\) passes through point \(Q\). The distance \(d\) from the plane to a point \(P\) not in the plane is given by
\[d=‖\text{proj}_\vecs{n}\,\vecd{QP}‖=∣\text{comp}_\vecs{n}\, \vecd{QP}∣=\dfrac{∣\vecd{QP}⋅\vecs{n}∣}{‖\vecs{n}‖}. \label{distanceplanepoint}\]
Example \(\PageIndex{7}\): Distance between a Point and a Plane
Find the distance between point \(P=(3,1,2)\) and the plane given by \(x−2y+z=5\) (see the following figure).
Solution
The coefficients of the plane’s equation provide a normal vector for the plane: \(\vecs{n}=⟨1,−2,1⟩\). To find vector \(\vecd{QP}\), we need a point in the plane. Any point will work, so set \(y=z=0\) to see that point \(Q=(5,0,0)\) lies in the plane. Find the component form of the vector from \(Q\) to \(P\):
\[ \vecd{QP}=⟨3−5,1−0,2−0⟩=⟨−2,1,2⟩. \nonumber \]
Apply the distance formula from Equation \ref{distanceplanepoint}:
\[\begin{align*} d &=\dfrac{\lvert\vecd{QP}⋅\vecs n\rvert}{‖\vecs n‖} \\[5pt] &=\dfrac{|⟨−2,1,2⟩⋅⟨1,−2,1⟩|}{\sqrt{1^2+(−2)^2+1^2}} \\[5pt] &=\dfrac{|−2−2+2|}{\sqrt{6}} \\[5pt] &=\dfrac{2}{\sqrt{6}} = \dfrac{\sqrt{6}}{3}\,\text{units}. \end{align*}\]
Exercise \(\PageIndex{7}\)
Find the distance between point \(P=(5,−1,0)\) and the plane given by \(4x+2y−z=3\).
- Hint
-
Point \((0,0,−3)\) lies on the plane.
- Answer:
-
\( \dfrac{15}{\sqrt{21}} = \dfrac{5\sqrt{21}}{7}\,\text{units} \)
Intersection of a Line and a Plane
A given line and a given plane may or may not intersect. If the line does intersect with the plane, it's possible that the line is completely contained in the plane as well. How can we differentiate between these three possibilities?
Example \(\PageIndex{8}\): Finding the intersection of a Line and a plane
Determine whether the following line intersects with the given plane. If they do intersect, determine whether the line is contained in the plane or intersects it in a single point. Finally, if the line intersects the plane in a single point, determine this point of intersection.
\[\begin{align*} \text{Line:}\quad x &=2 - t & \text{Plane:} \quad 3x - 2y + z = 10 \\[5pt] y &= 1 + t \\[5pt] z &= 3t \end{align*}\nonumber\]
Solution
Notice that we can substitute the expressions of \(t\) given in the parametric equations of the line into the plane equation for \(x\), \(y\), and \(z\).
\[3(2-t) - 2(1+t) + 3t = 10\nonumber\]
Solving this equation for \(t\):
\[6 - 3t -2 - 2t + 3t = 10\nonumber\]
\[4 - 2t = 10\nonumber\]
\[-2t = 6\nonumber\]
\[t = -3\nonumber\]
Since we found a single value of \(t\) from this process, we know that the line should intersect the plane in a single point, here where \(t = -3\). So the point of intersection can be determined by plugging this value in for \(t\) in the parametric equations of the line.
Here: \(x = 2 - (-3) = 5,\quad y = 1 + (-3) = -2, \,\text{and}\quad z = 3(-3) = -9\).
So the point of intersection of this line with this plane is \(\left(5, -2, -9\right)\). We can verify this by putting the coordinates of this point into the plane equation and checking to see that it is satisfied.
Check: \(3(5) - 2(-2) + (-9) = 15 + 4 - 9 = 10\quad\checkmark\)
Now that we have examined what happens when there is a single point of intersection between a line and a point, let's consider how we know if the line either does not intersect the plane at all or if it lies on the plane (i.e., every point on the line is also on the plane).
Example \(\PageIndex{9}\): Other relationships between a line and a plane
Determine whether the following line intersects with the given plane. If they do intersect, determine whether the line is contained in the plane or intersects it in a single point. Finally, if the line intersects the plane in a single point, determine this point of intersection.
\[\begin{align*} \text{Line:}\quad x &=1 + 2t & \text{Plane:} \quad x + 2y - 2z = 5 \\[5pt] y &= -2 + 3t \\[5pt] z &= -1 + 4t \end{align*}\nonumber\]
Solution
Substituting the expressions of \(t\) given in the parametric equations of the line into the plane equation gives us:
\[(1+2t) +2(-2+3t) - 2(-1 + 4t) = 5\nonumber\]
Simplifying the left side gives us:
\[1 + 2t -4 + 6t + 2 - 8t = 5\nonumber\]
Collecting like terms on the left side causes the variable \(t\) to cancel out and leaves us with a contradiction:
\[-1 = 5\nonumber\]
Since this is not true, we know that there is no value of \(t\) that makes this equation true, and thus there is no value of \(t\) that will give us a point on the line that is also on the plane. This means that this line does not intersect with this plane and there will be no point of intersection.
How can we tell if a line is contained in the plane?
What if we keep the same line, but modify the plane equation to be \( x + 2y - 2z = -1\)? In this case, repeating the steps above would again cause the variable \(t\) to be eliminated from the equation, but it would leave us with an identity, \(-1 = -1\), rather than a contradiction. This means that every value of \(t\) will produce a point on the line that is also on the plane, telling us that the line is contained in the plane whose equation is \( x + 2y - 2z = -1\).
Parallel and Intersecting Planes
We have discussed the various possible relationships between two lines in two dimensions and three dimensions. When we describe the relationship between two planes in space, we have only two possibilities: the two distinct planes are parallel or they intersect. When two planes are parallel, their normal vectors are parallel. When two planes intersect, the intersection is a line (Figure \(\PageIndex{9}\)).
We can use the equations of the two planes to find parametric equations for the line of intersection as shown below in Example \(\PageIndex{10}\).
Example \(\PageIndex{10}\): Finding the Line of Intersection for Two Planes
Determine the parametric equations for the line of intersection of the planes given by \(x+y+z=0\) and \(2x−y+z=0\). See the following figure.
Solution
Finding the line of intersection:
We will use two steps to solve for the line of intersection.
1. Find a point on the line of intersection (i.e., a point that lies on both planes).
2. Find a direction vector for the line of intersection.
1. To find a point that lies on both planes, we first use the elimination method for solving a system of equations to eliminate one of the variables, in this case, \(y\). For this pair of plane equations, we just have to add the equations to eliminate a variable, but sometimes we may need to multiply one or both equations by a factor to make it easier to eliminate a variable when we add the equations.
Adding these equations gives us:
\(x+y+z=0\)
\(2x−y+z=0\)
________________
\(3x+2z=0\).
Solve for one of the variables. If we solve for \(x\), we find \(x=−\dfrac{2}{3}z.\) Now choose any value for \(z\), say \( z = 3\). Then \( x = -2\).
Now plugging these two values into one of the plane equations, we can solve for the corresponding value of \(y\) that will give us a point that should satisfy both planes (i.e., it will lie on the line of intersection).
Plugging into the equation \(x + y + z = 0\) gives us \(-2 + y + 3 = 0\quad\rightarrow\quad y = -1\). So a point on the line of intersection is \(\left(-2, -1, 3\right)\).
Verify that this point satisfies both plane equations: \(-2 + (-1) + 3 = 0 \, \checkmark\) and \(2(-2) - (-1) + 3 = -4 + 1 + 3 = 0 \, \checkmark\)
2. Now we are ready to find a direction vector for the line of intersection. Since this line must lie in both planes, the direction vector must be orthogonal to the normal vector s of both planes. Since we need a vector that is orthogonal to both normal vectors, we use the cross product of the normal vectors to obtain one. Thus:
Direction vector of the line of intersection: \(\vecs v = \vecs n_1 \times \vecs n_2 = \begin{vmatrix}\mathbf{\hat i}&\mathbf{\hat j}&\mathbf{\hat k}\\1&1&1\\2&−1&1\end{vmatrix} = \left(1 - (-1)\right)\mathbf{\hat i}- (1-2)\mathbf{\hat j}+(-1 - 2)\mathbf{\hat k} = 2\mathbf{\hat i}+ \mathbf{\hat j}- 3\mathbf{\hat k}\).
Now the line of intersection will contain the point \(\left(-2, -1, 3\right)\) and have direction vector \(\vecs v = 2\mathbf{\hat i}+ \mathbf{\hat j}- 3\mathbf{\hat k}\).
So a possible set of parametric equations of the line of intersection are: \( x = -2 + 2t, \quad y = -1 + t, \quad z = 3 - 3t\).
To check, we could either find another point on this line (for \(t \neq 0\) and verify that it satisfies both plane equations, or we could substitute these expressions of \(t\) into both plane equations and show that we obtain an identity in both cases (such as \(5 = 5\) or \(0 = 0\)).
For example, if we let \(t = 1\), the line's equations give us the origin \(\left(0,0,0\right)\) as a second point on the intersection line. It's easy to see that this point satisfies both plane equations.
Exercise \(\PageIndex{8}\)
Find parametric equations for the line formed by the intersection of planes \(x+y−z=3\) and \(3x−y+3z=5.\)
- Hint
-
Add the two equations, to eliminate the variable \(y\). Then use the resulting equation to determine a point on the line of intersection. Then find the direction vector, remembering it will be orthogonal to the normal vectors of both planes.
- Answer:
-
\( x=t, \; y=7−3t, \; z=4−2t \)
In addition to finding the equation of the line of intersection between two planes, we may need to find the angle formed by the intersection of two planes. For example, builders constructing a house need to know the angle where different sections of the roof meet to know whether the roof will look good and drain properly.
When two planes intersect, note that there are two supplementary angles formed between the planes (See Figure \(\PageIndex{9}\)). Typically the acute angle between two planes is the one desired. We can determine the acute angle between the two planes by finding the angle between their normal vectors and forcing it to be acute (by taking its supplement, if necessary). Figure \(\PageIndex{10}\) shows why this is true.
To be sure we obtain the acute angle between the two planes, we take the absolute value of the dot product of the two normal vectors, thus forcing the resulting angle \(\theta\) to be acute.
So, to find the acute angle \(\theta\) between two planes with normal vectors \(\vecs n_1\) and \(\vecs n_2\), we use the definition of the dot product and add the absolute values mentioned above to first find the cosine of the angle:
\[\cos θ=\dfrac{|\vecs{n}_1⋅\vecs{n}_2|}{‖\vecs{n}_1‖‖\vecs{n}_2‖}.\]
Example \(\PageIndex{11}\): Finding the Angle between Two Planes
Find the angle between the planes given by \(x+y+z=0\) and \(2x−y+z=0\) for which we found the line of intersection in Example \(\PageIndex{10}\).
Solution
Finding the angle between the planes:
Note that the two planes have nonparallel normals, so the planes intersect. They intersect forming two angles, one obtuse and one acute. We choose to find the acute angle, so we use the modified version of the definition of the dot product that we stated above.
We can read the components of a normal vector from the coefficients of the variables in each plane equation.
Thus: \(\vecs n_1 = \lt 1, 1, 1\gt\) and \(\vecs n_2 = \lt 2, -1, 1\gt\).
Now
\[\vecs n_1 \cdot \vecs n_2 = 2 + (-1) + 1 = 2. \nonumber\]
Their magnitudes are:
\[\| \vecs n_1 \| = \sqrt{1^2 + 1^2 + 1^2} = \sqrt{3} \nonumber\]
\[\| \vecs n_2 \| = \sqrt{2^2 + (-1)^2 + 1^2} = \sqrt{6} \nonumber\]
Then
\[\cos \theta = \dfrac{|\vecs n_1 \cdot \vecs n_2|}{\|\vecs n_1\| \|\vecs n_2\|} = \frac{|2|}{\sqrt{3}\sqrt{6}} = \frac{2}{\sqrt{18}}.\]
So the acute angle between these two planes is given by \(\theta = \arccos \left(\frac{2}{\sqrt{18}}\right) \approx 61.9° \approx 1.08\) radians.
Once we find the angle between two planes we can use it to determine whether the two planes are parallel or orthogonal or if they intersect at some other angle.
Example \(\PageIndex{12}\): determine if planes are Parallel, Orthogonal, or neither
Determine whether each pair of planes is parallel, orthogonal, or neither. If the planes are intersecting, but not orthogonal, find the measure of the angle between them. Give the answer in radians and round to two decimal places.
- \(x+2y−z=8\) and \(2x+4y−2z=10\)
- \(2x−3y+2z=3\) and \(6x+2y−3z=1\)
- \(x+y+z=4\) and \(x−3y+5z=1\)
Solution:
- The normal vectors for these planes are \(\vecs{n}_1=⟨1,2,−1⟩\) and \(\vecs{n}_2=⟨2,4,−2⟩.\) These two vectors are scalar multiples of each other. The normal vectors are parallel, so the planes are parallel.
- The normal vectors for these planes are \(\vecs{n}_1=⟨2,−3,2⟩\) and \(\vecs{n}_2=⟨6,2,−3⟩\). Taking the dot product of these vectors, we have \[\begin{align*} \vecs{n}_1⋅\vecs{n}_2&=⟨2,−3,2⟩⋅⟨6,2,−3⟩\\[5pt] &=2(6)−3(2)+2(−3)=0.\end{align*} \] The normal vectors are orthogonal, so the corresponding planes are orthogonal as well.
- The normal vectors for these planes are \(\vecs n_1=⟨1,1,1⟩\) and \(\vecs n_2=⟨1,−3,5⟩\): \[\begin{align*} \cos θ &=\dfrac{|\vecs{n}_1⋅\vecs{n}_2|}{‖\vecs{n}_1‖‖\vecs{n}_2‖} \\[5pt] &=\dfrac{|⟨1,1,1⟩⋅⟨1,−3,5⟩|}{\sqrt{1^2+1^2+1^2}\sqrt{1^2+(−3)^2+5^2}} \\ &=\dfrac{3}{\sqrt{105}} \end{align*}\]
Then \(\theta =\arccos {\frac{3}{\sqrt{105}}} \approx 1.27\) rad.
Thus the angle between the two planes is about \(1.27\) rad, or approximately \(73°\).
Exercise \(\PageIndex{9}\)
Find the measure of the angle between planes \(x+y−z=3\) and \(3x−y+3z=5.\) Give the answer in radians and round to two decimal places.
- Hint
-
Use the coefficients of the variables in each equation to find a normal vector for each plane.
- Answer:
-
\( 1.44\, \text{rad} \)
When we find that two planes are parallel, we may need to find the distance between them. To find this distance, we simply select a point in one of the planes. The distance from this point to the other plane is the distance between the planes.
Previously, we introduced the formula for calculating this distance in Equation \ref{distanceplanepoint}:
\[d=\dfrac{\vecd{QP}⋅\vecs{n}}{‖\vecs{n}‖},\]
where \(Q\) is a point on the plane, \(P\) is a point not on the plane, and \(\vec{n}\) is the normal vector that passes through point \(Q\). Consider the distance from point \((x_0,y_0,z_0)\) to plane \(ax+by+cz+k=0.\) Let \((x_1,y_1,z_1)\) be any point in the plane. Substituting into the formula yields
\[\begin{align*}d&=\dfrac{|a(x_0−x_1)+b(y_0−y_1)+c(z_0−z_1)|}{\sqrt{a^2+b^2+c^2}} \\[5pt] &=\dfrac{|ax_0+by_0+cz_0+k|}{\sqrt{a^2+b^2+c^2}}.\end{align*}\]
We state this result formally in the following theorem.
Distance from a Point to a Plane
Let \(P(x_0,y_0,z_0)\) be a point. The distance from \(P\) to plane \(ax+by+cz+k=0\) is given by
\[d=\dfrac{|ax_0+by_0+cz_0+k|}{\sqrt{a^2+b^2+c^2}}.\]
Example \(\PageIndex{13}\): Finding the Distance between Parallel Planes
Find the distance between the two parallel planes given by \(2x+y−z=2\) and \(2x+y−z=8.\)
Solution
Point \((1,0,0)\) lies in the first plane. The desired distance, then, is
\[\begin{align*} d&=\dfrac{|ax_0+by_0+cz_0+k|}{\sqrt{a^2+b^2+c^2}} \\[5pt] &= \dfrac{|2(1)+1(0)+(−1)(0)+(−8)|}{\sqrt{2^2+1^2+(−1)^2}} \\[5pt] &= \dfrac{6}{\sqrt{6}}=\sqrt{6} \,\text{units} \end{align*}\]
Exercise \(\PageIndex{10}\):
Find the distance between parallel planes \(5x−2y+z=6\) and \(5x−2y+z=−3\).
- Hint
-
Set \(x=y=0\) to find a point on the first plane.
- Answer
-
\(\dfrac{9}{\sqrt{30}} = \dfrac{3\sqrt{30}}{10}\,\text{units} \)
Distance between Two Skew Lines
Finding the distance from a point to a line or from a line to a plane seems like a pretty abstract procedure. But, if the lines represent pipes in a chemical plant or tubes in an oil refinery or roads at an intersection of highways, confirming that the distance between them meets specifications can be both important and awkward to measure. One way is to model the two pipes as lines, using the techniques in this chapter, and then calculate the distance between them. The calculation involves forming vectors along the directions of the lines and using both the cross product and the dot product.
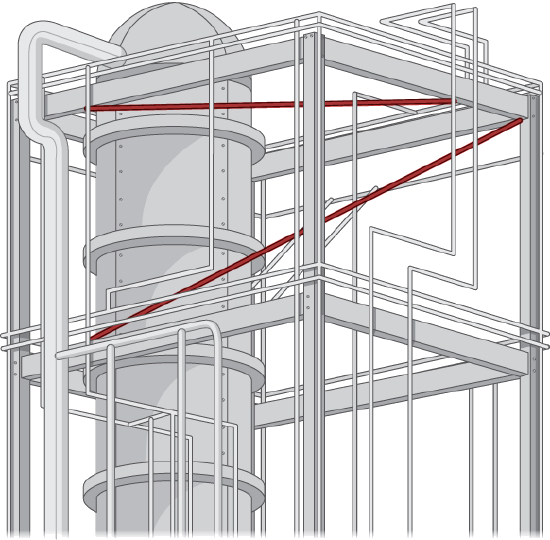
The symmetric forms of two lines, \(L_1\) and \(L_2\), are
\[L_1:\dfrac{x−x_1}{a_1}=\dfrac{y−y_1}{b_1}=\dfrac{z−z_1}{c_1}\]
\[L_2:\dfrac{x−x_2}{a_2}=\dfrac{y−y_2}{b_2}=\dfrac{z−z_2}{c_2}.\]
You are to develop a formula for the distance \(d\) between these two lines, in terms of the values \(a_1,b_1,c_1;a_2,b_2,c_2;x_1,y_1,z_1;\) and \(x_2,y_2,z_2.\) The distance between two lines is usually taken to mean the minimum distance, so this is the length of a line segment or the length of a vector that is perpendicular to both lines and intersects both lines.
1. First, write down two vectors, \(\vecs{v}_1\) and \(\vecs{v}_2\), that lie along \(L_1\) and \(L_2\), respectively.
2. Find the cross product of these two vectors and call it \(\vecs{n}\). This vector is perpendicular to \(\vecs{v}_1\) and \(\vecs{v}_2\), and hence is perpendicular to both lines.
3. Use symmetric equations to find a convenient vector \(\vecs{v}_{12}\) that lies between any two points, one on each line. Again, this can be done directly from the symmetric equations.
4. Find the projection of vector \(\vecs{v}_{12}\) found in step \(3\) onto unit vector \(\vecs{n}\) found in step \(2\). This projection is perpendicular to both lines, and hence its length must be the perpendicular distance \(d\) between them.
5. Check that your formula gives the correct distance of \(d = \frac{25}{\sqrt{198}} = \frac{25\sqrt{22}}{66}\,\text{units}\,≈1.78\,\text{units}\) between the following two lines:
\[L_1:\dfrac{x−5}{2}=\dfrac{y−3}{4}=\dfrac{z−1}{3}\]
\[L_2:\dfrac{x−6}{3}=\dfrac{y−1}{5}=\dfrac{z}{7}.\]
6. Is your general expression valid when the lines are parallel? If not, why not? (Hint: What do you know about the value of the cross product of two parallel vectors? Where would that result show up in your expression for \(d\)?)
7. Demonstrate that your expression for the distance is zero when the lines intersect. Recall that two lines intersect if they are not parallel and they are in the same plane. Hence, consider the direction of \(\vecs{n}\) and \(\vecs{v}_{12}\). What is the result of their dot product?
8. Consider the following application. Engineers at a refinery have determined they need to install support struts between many of the gas pipes to reduce damaging vibrations. To minimize cost, they plan to install these struts at the closest points between adjacent skewed pipes. Because they have detailed schematics of the structure, they are able to determine the correct lengths of the struts needed, and hence manufacture and distribute them to the installation crews without spending valuable time making measurements.
The rectangular frame structure has the dimensions \(4.0×15.0×10.0\,\text{m}\) (height, width, and depth). One sector has a pipe entering the lower corner of the standard frame unit and exiting at the diametrically opposed corner (the one farthest away at the top); call this \(L_1\). A second pipe enters and exits at the two different opposite lower corners; call this \(L_2\) (Figure \(\PageIndex{12}\)).

Write down the vectors along the lines representing those pipes, find the cross product between them from which to create the mutually orthogonal vector \(\vecs n\), define a vector that spans two points on each line, and finally determine the minimum distance between the lines. (Take the origin to be at the lower corner of the first pipe.) Similarly, you may also develop the symmetric equations for each line and substitute directly into your formula.
Key Concepts
- In three dimensions, the direction of a line is described by a direction vector. The vector equation of a line with direction vector \(\vecs v=⟨a,b,c⟩\) passing through point \(P=(x_0,y_0,z_0)\) is \(\vecs r=\vecs r_0+t\vecs v\), where \(\vecs r_0=⟨x_0,y_0,z_0⟩\) is the position vector of point \(P\). This equation can be rewritten to form the parametric equations of the line: \(x=x_0+ta,y=y_0+tb\), and \(z=z_0+tc\). The line can also be described with the symmetric equations \[\dfrac{x−x_0}{a}=\dfrac{y−y_0}{b}=\dfrac{z−z_0}{c}.\]
- Let \(L\) be a line in space passing through point \(P\) with direction vector \(\vecs v\). If \(Q\) is any point not on \(L\), then the distance from \(Q\) to \(L\) is \[d=\dfrac{‖\vecd{PQ}×\vecs v‖}{‖\vecs v‖}. \nonumber\]
- In three dimensions, two lines may be parallel but not equal, equal, intersecting, or skew.
- Given a point \(P\) and vector \(\vecs n\), the set of all points \(Q\) satisfying equation \(\vecs n⋅\vecd{PQ}=0\) forms a plane. This equation is known as the vector equation of a plane.
- The scalar equation of a plane containing point \(P=(x_0,y_0,z_0)\) with normal vector \(\vecs n=⟨a,b,c⟩\) is \[a(x−x_0)+b(y−y_0)+c(z−z_0)=0 \nonumber\]. This equation can be expressed as \[ax+by+cz+d=0, \nonumber \] where \(d=−ax_0−by_0−cz_0.\) This form of the equation is sometimes called the general form of the equation of a plane.
- Suppose a plane with normal vector \(n\) passes through point \(Q\). The distance \(D\) from the plane to point \(P\) not in the plane is given by \[D=‖\text{proj}_\vecs{n}\vecd{QP}‖=∣\text{comp}_\vecs{n}\vec{QP}∣=\dfrac{∣\vec{QP}⋅\vecs n∣}{‖\vecs n‖.} \nonumber\]
- The normal vectors of parallel planes are parallel. When two planes intersect, they form a line.
- The measure of the angle \(θ\) between two intersecting planes can be found using the equation: \[\cos θ=\dfrac{|\vecs{n}_1⋅\vecs n_2|}{‖\vecs n_1‖‖\vecs n_2‖} \nonumber\] where \(\vecs n_1\) and \(\vecs n_2\) are normal vectors to the planes.
- The distance \(D\) from point \((x_0,y_0,z_0)\) to plane \(ax+by+cz+d=0\) is given by
\[D=\dfrac{|a(x_0−x_1)+b(y_0−y_1)+c(z_0−z_1)|} {\sqrt{a^2+b^2+c^2}}=\dfrac{|ax_0+by_0+cz_0+d|}{\sqrt{a^2+b^2+c^2}} \nonumber\].
Key Equations
- Vector Equation of a Line \[\vecs r=\vecs r_0+t\vecs v \nonumber\]
- Parametric Equations of a Line \[x=x_0+ta,y=y_0+tb, \nonumber \] and \[z=z_0+tc\nonumber\]
- Symmetric Equations of a Line \[\dfrac{x−x_0}{a}=\dfrac{y−y_0}{b}=\dfrac{z−z_0}{c}\nonumber\]
- Vector Equation of a Plane \[\vecs n⋅\vecd{PQ}=0\nonumber\]
- Scalar Equation of a Plane \[\nonumber a(x−x_0)+b(y−y_0)+c(z−z_0)=0\]
- Distance between a Plane and a Point \[d=‖\text{proj}_\vecs{n}\vecd{QP}‖=∣\text{comp}_\vecs{n}\vecd{QP}∣=\dfrac{∣\vecd{QP}⋅\vecs n∣}{‖\vecs n‖}\nonumber\]
Glossary
- direction vector
- a vector parallel to a line that is used to describe the direction, or orientation, of the line in space
- general form of the equation of a plane
- an equation in the form \(ax+by+cz+d=0,\) where \(\vecs n=⟨a,b,c⟩\) is a normal vector of the plane, \(P=(x_0,y_0,z_0)\) is a point on the plane, and \(d=−ax_0−by_0−cz_0\)
- normal vector
- a vector perpendicular to a plane
- parametric equations of a line
- the set of equations \(x=x_0+ta, y=y_0+tb,\) and \(z=z_0+tc\) describing the line with direction vector \(v=⟨a,b,c⟩\) passing through point \((x_0,y_0,z_0)\)
- scalar equation of a plane
- the equation \(a(x−x_0)+b(y−y_0)+c(z−z_0)=0\) used to describe a plane containing point \(P=(x_0,y_0,z_0)\) with normal vector \(n=⟨a,b,c⟩\) or its alternate form \(ax+by+cz+d=0\), where \(d=−ax_0−by_0−cz_0\)
- skew lines
- two lines that are not parallel but do not intersect
- symmetric equations of a line
- the equations \(\dfrac{x−x_0}{a}=\dfrac{y−y_0}{b}=\dfrac{z−z_0}{c}\) describing the line with direction vector \(v=⟨a,b,c⟩\) passing through point \((x_0,y_0,z_0)\)
- vector equation of a line
- the equation \(\vecs r=\vecs r_0+t\vecs v\) used to describe a line with direction vector \(\vecs v=⟨a,b,c⟩\) passing through point \(P=(x_0,y_0,z_0)\), where \(\vecs r_0=⟨x_0,y_0,z_0⟩\), is the position vector of point \(P\)
- vector equation of a plane
- the equation \(\vecs n⋅\vecd{PQ}=0,\) where \(P\) is a given point in the plane, \(Q\) is any point in the plane, and \(\vecs n\) is a normal vector of the plane
Contributors
Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.
- Edited by Paul Seeburger (Monroe Community College)


