In this section we will look at an assortment of practical problems involving percent.
Example 4
35 millilitres of a 60 millilitre solution is hydrochloric acid. What percent of the solution is hydrochloric acid?
Solution
Let p represent the percent of the percent of the solution that is hydrochloric acid. Then we can translate the problem statement into words and symbols.
\[ \begin{array}{c c c c c} \colorbox{cyan}{Amount of hydrochloric acid} & \text{ is } & \colorbox{cyan}{what percent} & \text{ of } & \colorbox{cyan}{the tota amount of solution} \\ 35 & = & p & \cdot & 60 \end{array}\nonumber \]
Because multiplication is commutative, we can write the right-hand side of the last equation as follows.
\[35 = 60p\nonumber \]
Now we can solve for p.
\[ \begin{aligned} \frac{35}{60} = \frac{60p}{60} ~ & \textcolor{red}{ \text{ Divide both sides by 60.}} \\ \frac{7}{12} = p ~ & \textcolor{red}{ \text{ Reduce: Divide numerator and denominator by 5.}} \end{aligned}\nonumber \]
Now we must change p to a percent. We can do this exactly by creating an equivalent fraction with a denominator of 100.
\[ \frac{7}{12} = \frac{n}{100}\nonumber \]
Solve for n.
\[ \begin{aligned} 12n = 700 ~ & \textcolor{red}{ \text{ Cross multiply.}} \\ \frac{12n}{12} = \frac{700}{12} ~ & \textcolor{red}{ \text{ Divide both sides by 12.}} \\ n = \frac{175}{3} ~ & \textcolor{red}{ \text{ Reduce: Divide numerator and denominator by 4.}} \\ n = 58 \frac{1}{3} ~ & \textcolor{red}{ \text{ Change improper to mixed fraction.}} \end{aligned}\nonumber \]
Hence,
\[p = \frac{7}{12} = \frac{58 \frac{1}{3}}{100} = 58 \frac{1}{3} \%.\nonumber \]
Thus, \(58 \frac{1}{3} \%\) of the solution is hydrochloric acid.
Approximate Solution
If all that is needed is an approximate answer, say correct to the nearest tenth of a percent, then we would take a different approach starting with the line from above that has
\[\frac{35}{60} = p.\nonumber \]
We would divide 35 by 60 to get
\[p \approx 0.5833.\nonumber \]
Move the decimal two places to the right and append a percent symbol.

Round to the nearest tenth of a percent.
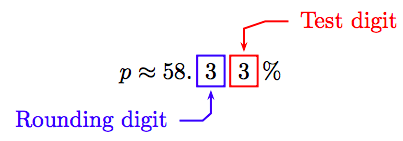
Because the test digit is less than 5, leave the rounding digit alone and truncate. Thus, correct to the nearest tenth of a percent,
\[p \approx 58.3 \%.\nonumber \]
Note that p ≈ 58.3% is approximate, but \(p = 58 \frac{1}{3} \%\) is exact.
Exercises
1. 31 millilitres of a 250 millilitre solution is sulphuric acid. What percent of the solution is sulphuric acid? Round your answer to the nearest tenth of a percent.
2. 34 millilitres of a 211 millilitre solution is phosphoric acid. What percent of the solution is phosphoric acid? Round your answer to the nearest tenth of a percent.
3. A family has completed 186 miles of a planned 346 mile trip. Find the percentage of the planned trip already traveled. Round your answer to the nearest percent.
4. A family has completed 153 miles of a planned 431 mile trip. Find the percentage of the planned trip already traveled. Round your answer to the nearest percent.
5. Erin takes roll in her fifth grade class and finds that 19 out of 34 total students on her roster are present. Find the percentage of the class that is present, correct to the nearest percent.
6. Barbara takes roll in her fifth grade class and finds that 15 out of 38 total students on her roster are present. Find the percentage of the class that is present, correct to the nearest percent.
7. Raven answered 135 of 150 possible questions on the meteorology examination correctly. What percent of the total number of questions did Raven mark correctly?
8. Liz answered 30 of 50 possible questions on the algebra examination correctly. What percent of the total number of questions did Liz mark correctly?
9. A family has traveled 114 miles of a planned trip. This is 37% of the total distance they must travel on the trip. Find, correct to the nearest mile, the total distance they will travel on their trip.
10. A family has traveled 102 miles of a planned trip. This is 23% of the total distance they must travel on the trip. Find, correct to the nearest mile, the total distance they will travel on their trip.
11. Trudy takes roll in her class at the university and finds that 65 students are present. If this is 50% of the total class enrollment, how many students are in the class?
12. Sandra takes roll in her class at the university and finds that 104 students are present. If this is 80% of the total class enrollment, how many students are in the class?
13. Bill earns a commission on all sales he makes. He sells a bed for $591 and earns a commission of $43. Find the percent commission, rounded to the nearest tenth of a percent.
14. Ira earns a commission on all sales he makes. He sells a sofa for $408 and earns a commission of $39. Find the percent commission, rounded to the nearest tenth of a percent.
15. Tami answered 70% of the questions on the physics examination correctly. If Tami had 98 correct answers, how many questions were on the exam?
16. Trinity answered 90% of the questions on the chemistry examination correctly. If Trinity had 99 correct answers, how many questions were on the exam?
17. A state charges 8% sales tax on all sales. If the sales tax on a computer is $20, find the sales price of the computer, correct to the nearest dollar.
18. A state charges 6.5% sales tax on all sales. If the sales tax on a bed is $33, find the sales price of the bed, correct to the nearest dollar.
19. Kenon earns 6% commission all his sales. If the sale of a computer earns him a $37 commission, find the sales price of the computer, correct to the nearest dollar.
20. Donald earns 4.5% commission all his sales. If the sale of a dryer earns him a $24 commission, find the sales price of the dryer, correct to the nearest dollar.
21. A 23% nitric acid solution contains 59 millilitres of nitric acid. How many total millilitres of solution are present? Round your answer to the nearest millilitre.
22. A 27% sulphuric acid solution contains 67 millilitres of sulphuric acid. How many total millilitres of solution are present? Round your answer to the nearest millilitre.
23. In a state, a television sold for $428 is assessed a sales tax of $45. Find the sales tax rate, rounded to the nearest tenth of a percent.
24. In a state, a refrigerator sold for $503 is assessed a sales tax of $44. Find the sales tax rate, rounded to the nearest tenth of a percent.
25. Mars gravity. The force of gravity on Mars is only 38% of the force of gravity on earth. If you weigh 150 pounds on earth, how much will you weigh on Mars?
26. Wiretaps. In 2008, there were a total of 1,891 applications to federal and state judges to authorize the interception of wire, oral, or electronic communications. If 94% of all wiretap applications were for a portable device such as a cell phone or pager, how many applications were made to tap mobile devices? Round-off to the nearest application. Associated Press Times-Standard 4/28/09
27. Seniors. 13% of Humboldt County’s population is age 65 and older, about 2% more than the state’s average. If the population of Humboldt County is approximately 130,000, how many people in Humboldt County are age 65 and older? Times-Standard 6/10/2009
28. Antibiotics. “The U.S. used about 35 million pounds of antibiotics last year. 70 percent of the drugs went to pigs, chickens, and cows.” How many million pounds of antibiotics went to the pigs, chickens, and cows? Associated Press-Times-Standard 12/29/09 Pressure rises to stop antibiotics in agriculture.
29. Grow faster. “Approximately 28 million pounds of antibiotics were fed to farm animals in the US during 2008. Thirteen percent of that was fed to healthy animals to make them grow faster.” How many pounds of antibiotics were fed to healthy animals? Associated Press-Times-Standard 12/29/09 Pressure rises to stop antibiotics in agriculture.
30. CO2 emissions. The accord agreed to by the US at the Copenhagen climate talks had greenhouse gas emissions held to 3.5% of 1990 levels. If 1990 levels were 5022 MMT (millions of metric tons), how many millions of metric tons might greenhouse emissions be held to? Round the result to the nearest MMT. Associated Press-Times-Standard 12/19/09 Elements of new Copenhagen accord.
31. Water supply. A new water desalination plant, the largest in the Western hemisphere, could come online by 2012 in Carlsbad, California, providing 50 million gallons of drinking water per day, or 10% of the supply for San Diego County. What is the total amount of drinking water supplied to San Diego County daily? Associated Press-Times-Standard
32. Earthquake damage. After the recent earthquake in Chile, an estimated 33 million gallons of Chilean wine, or 13% of annual production, was lost. Estimate the total annual production of Chilean wine rounded to the nearest millions of gallons. Associated Press-Times Standard 03/24/10 Hemorrhaging cabernet: Earthquake hits winemakers in Chile.
33. Snowpack. At a meadow near Echo Summit in the northern Sierra Nevada, water officials measured the snow at 65.7 inches. The water content was 25.9 inches, which is 92% of the average for this time of year. Determine the average water content for this time of year rounded to the nearest tenth of an inch. Associated Press-Times Standard 04/02/10 California’s Sierra snowpack slightly above normal.
34. Storefronts. According to the Times-Standard, as of April 2008 the Bayshore Mall had 55 occupied storefronts and 17 vacant storefronts. What percent of total storefronts are vacant? Round your answer to the nearest whole number. Times-Standard 4/19/09
35. Recovered. In Humboldt County, California, 427 of the 499 vehicles stolen between August 2008 and August 2009 were recovered. What percent of the stolen vehicles were recovered? Round your result to the nearest tenth of a percent. Times-Standard CHP offers tips on avoiding vehicle theft.
36. Freshman admissions. Stanford University sent acceptance letters to 2, 300 of 32, 022 freshman applicants. What percent of freshman applicants got acceptance letters, rounded to the nearest percent? Associated Press-Times-Standard 03/30/10 Stanford U. reports record-low admission rate.
37. Reduce. Each year, Americans throw out an average of about 1, 600 pounds of waste per person. Arcata, CA resident Michael Winkler only uses one trash bag every year – totaling at most 40 pounds. Find the percent of average annual waste per person Mr. Winkler throws out to a tenth of a percent. Times-Standard Allison White 12/26/09 Waste not...
38. Population decrease. The table below shows the population of Detroit, Michigan. Associated Press-Times-Standard 03/09/10 Detroit wants to save itself by shrinking.
\[ \begin{array}{c c} \text{ Year } & \text{ Population } \\ 1950 & 1,849,568 \\ 1990 & 1,027,974 \\ 2005 & 890,963 \end{array}\nonumber \]
What is the population of Detroit in 2005 as a percent of the population in 1950? Round your result to the nearest percent.




