8.5: Solving Nonlinear Systems
( \newcommand{\kernel}{\mathrm{null}\,}\)
Learning Objectives
- Identify nonlinear systems.
- Solve nonlinear systems using the substitution method.
Nonlinear Systems
A system of equations where at least one equation is not linear is called a nonlinear system32. In this section we will use the substitution method to solve nonlinear systems. Recall that solutions to a system with two variables are ordered pairs (x,y) that satisfy both equations.
Example 8.5.1:
Solve: {x+2y=0x2+y2=5.
Solution
In this case we begin by solving for x in the first equation.
{x+2y=0x2+y2=5⟹x=−2y
Substitute x=−2y into the second equation and then solve for y.
(−2y)2+y2=54y2+y2=55y2=5y2=1y=±1
Here there are two answers for y; use x=−2y to find the corresponding x-values.
| Using y=−1 | Using y=1 |
|---|---|
| x=−2y=−2(−1)=2 | x=−2y=−2(1)=−2 |
This gives us two ordered pair solutions, (2,−1) and (−2,1).
Answer:
(2,−1),(−2,1)
In the previous example, the given system consisted of a line and a circle. Graphing these equations on the same set of axes, we can see that the two ordered pair solutions correspond to the two points of intersection.
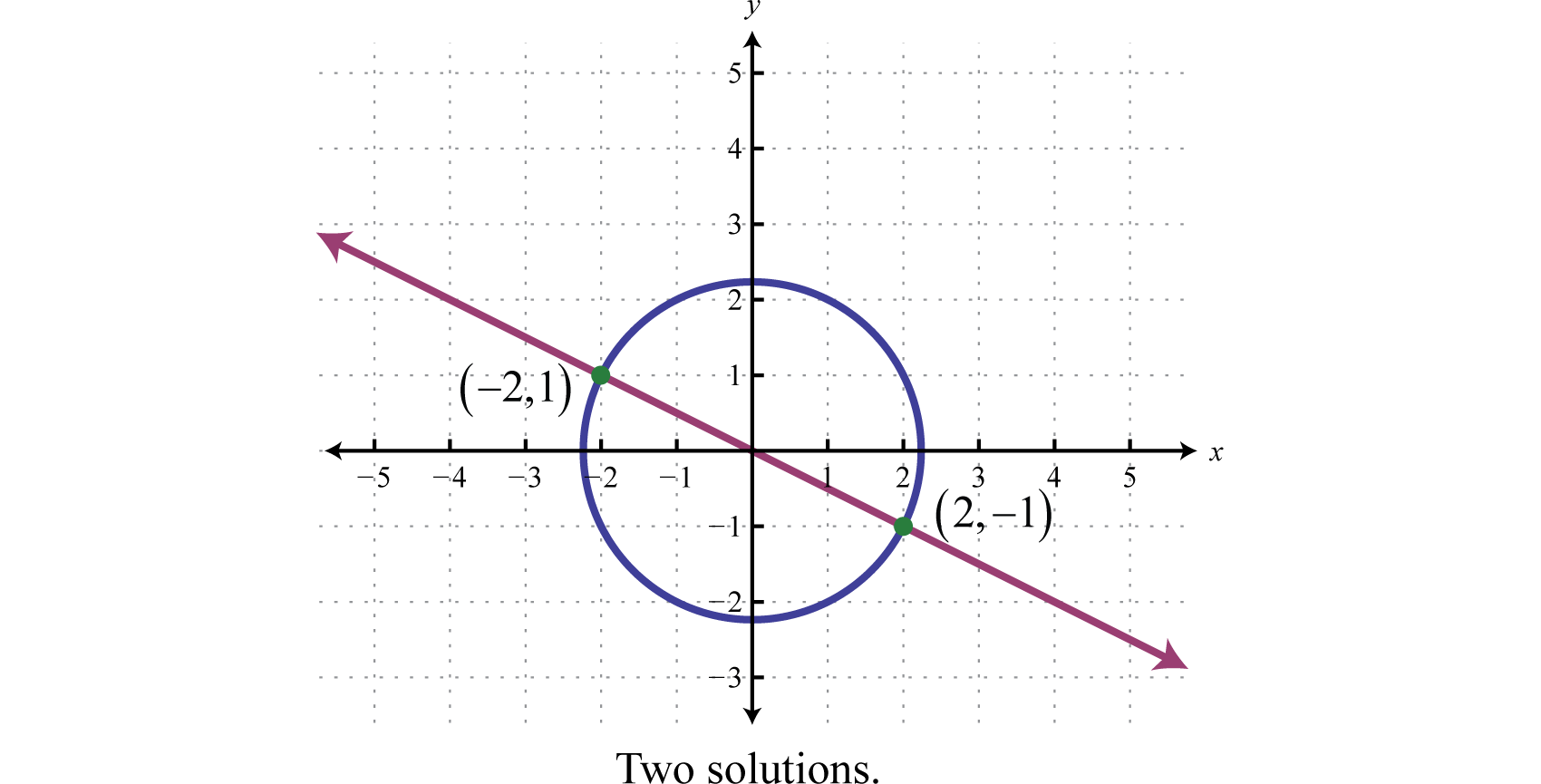
If we are given a system consisting of a circle and a line, then there are 3 possibilities for real solutions—two solutions as pictured above, one solution, or no solution.

Example 8.5.2
Solve: {x+y=3x2+y2=2.
Solution
Solve for y in the first equation.
{x+yx2+y2
Next, substitute y=3−x into the second equation and then solve for x.
x2+(3−x)2=2x2+9−6x+x2=22x2−6x+9=22x2−6x+7=0
The resulting equation does not factor. Furthermore, using a=2,b=−6, and c=7 we can see that the discriminant is negative:
b2−4ac=(−6)2−4(2)(7)=36−56=−20
We conclude that there are no real solutions to this equation and thus no solution to the system.
Answer:
Ø
Exercise 8.5.1
Solve: {x−y=5x2+(y+1)2=8
- Answer
-
(2,−3)
www.youtube.com/v/ToIrjw-8SNA
If given a circle and a parabola, then there are 5 possibilities for solutions.
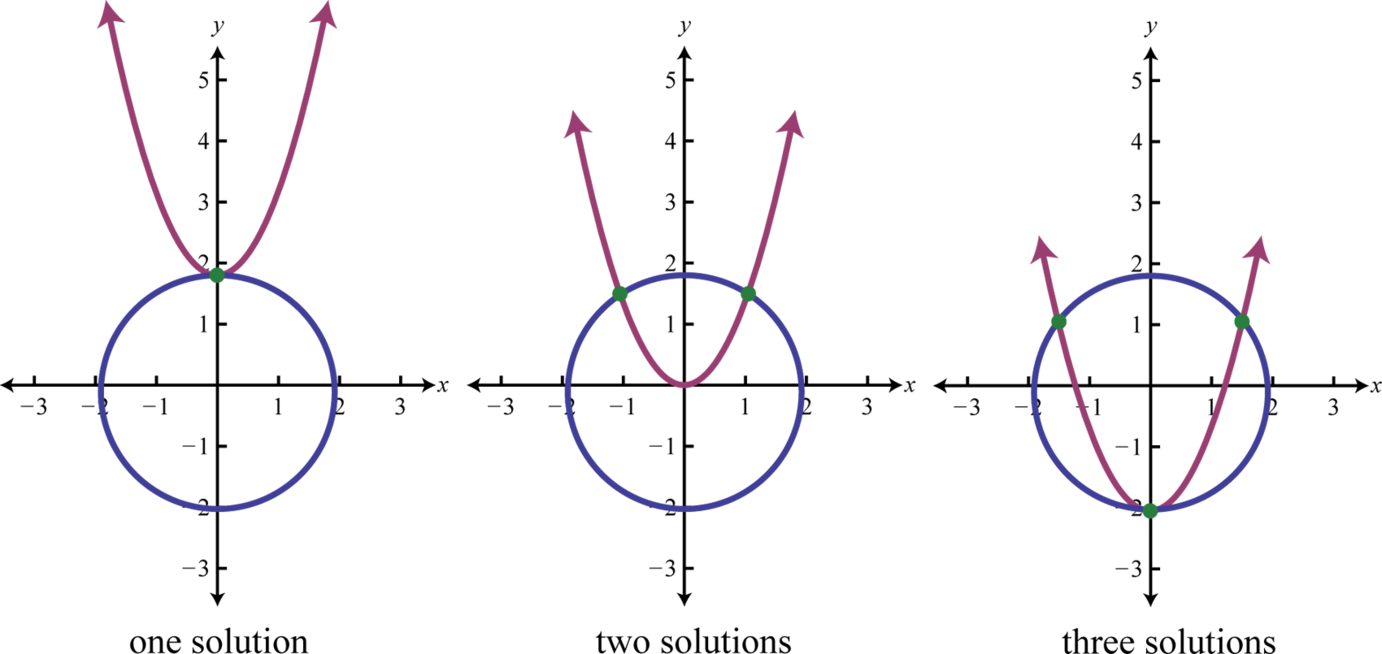
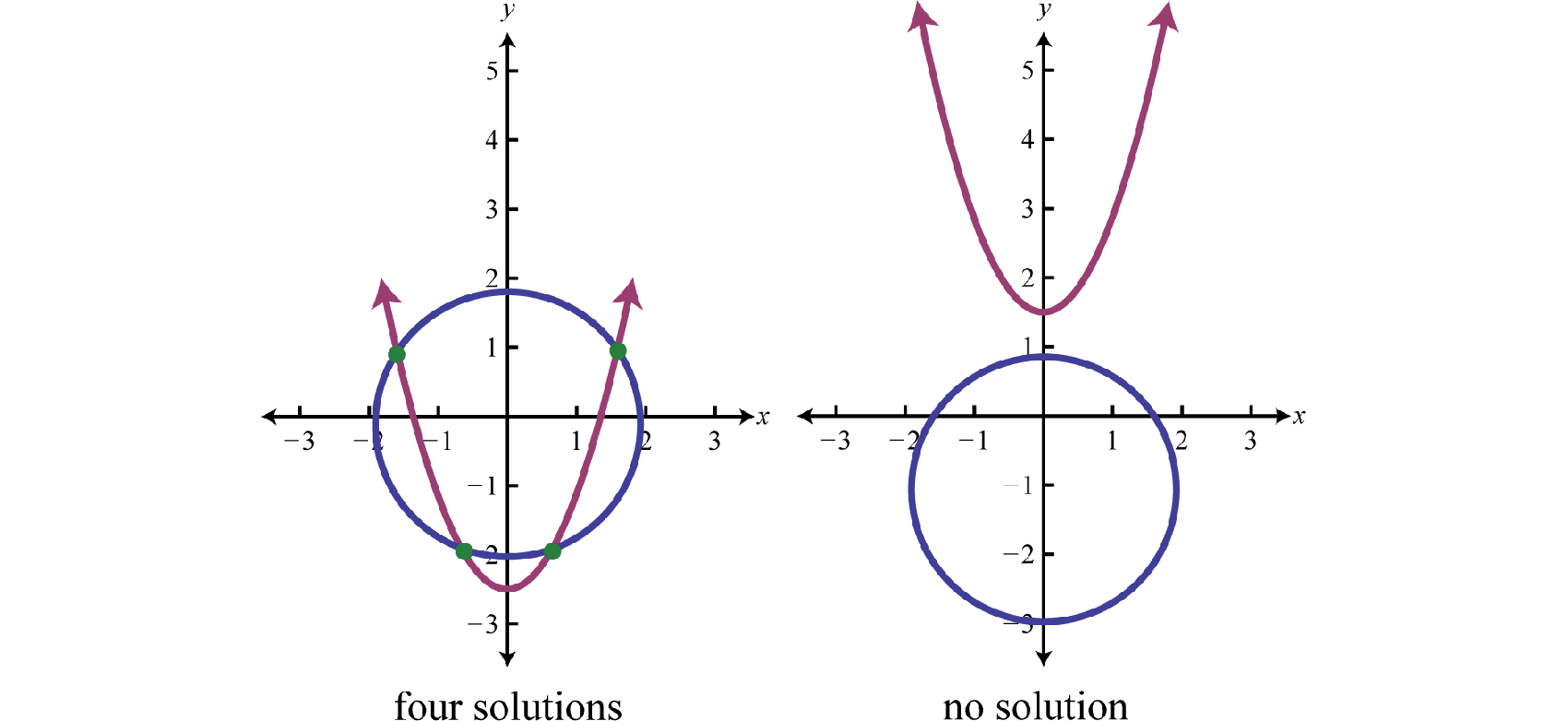
When using the substitution method, we can perform the substitution step using entire algebraic expressions. The goal is to produce a single equation in one variable that can be solved using the techniques learned up to this point in our study of algebra.
Example 8.5.3:
Solve: {x2+y2=2y−x2=−2.
Solution
We can solve for x2 in the second equation.
{x2+y2=2y−x2=−2⇒y+2=x2
Substitute x2=y+2 into the first equation and then solve for y.
y+2+y2=2y2+y=0y(y+1)=0y=0 or y=−1
Back substitute into x2=y+2 to find the corresponding x-values.
| Using y=−1 | Using y=0 |
|---|---|
| x2=y+2x2=−1+2x2=1x=±1 | x2=y+2x2=0+2x2=2x=±√2 |
This leads us to four solutions, (±1,−1) and (±√2,0).
Answer:
(±1,−1),(±√2,0)
Example 8.5.4
Solve: {(x−1)2−2y2=4x2+y2=9
Solution
We can solve for y2 in the second equation,
{(x−1)2−2y2=4x2+y2=9⟹y2=9−x2
Substitute y2=9−x2 into the first equation and then solve for x.
(x−1)2−2(9−x2)=4x2−2x+1−18+2x2=03x2−2x−21=0(3x+7)(x−3)=03x+7=0 or x−3=0x=−73x=3
Back substitute into y2=9−x2 to find the corresponding y-values.
| Using x=−73 | Using x=3 |
|---|---|
| y2=9−(−73)2y2=91−499y2=329y=±√323=±4√23 | y2=9−(3)2y2=0y=0 |
This leads to three solutions, (−73,±4√23) and (3,0).
Answer:
(3,0),(−73,±4√23)
Example 8.5.5
Solve: {x2+y2=2xy=1.
Solution
Solve for y in the second equation.
{x2+y2=2xy=1⟹y=1x
Substitute y=1x into the first equation and then solve for x.
x2+(1x)2=2
x2+1x2=2
This leaves us with a rational equation. Make a note that x≠0 and multiply both sides by x2.
x2(x2+1x2)=2⋅x2x4+1=2x2x4−2x2+1=0(x2−1)(x2−1)=0
At this point we can see that both factors are the same. Apply the zero product property.
x2−1=0x2=1x=±1
Back substitute into y=1x to find the corresponding y-values.
| Using x=−1 | Using x=1 |
|---|---|
| y=1x=1−1=−1 | y=1x=11=1 |
This leads to two solutions.
Answer:
(1,1),(−1,−1)
Exercise 8.5.2
Solve: {1x+1y=41x2+1y2=40
- Answer
-
(−12,16)(16,−12)
www.youtube.com/v/n8JJ_ybegkM
Key Takeaways
- Use the substitution method to solve nonlinear systems.
- Streamline the solving process by using entire algebraic expressions in the substitution step to obtain a single equation with one variable.
- Understanding the geometric interpretation of the system can help in finding real solutions.
Exercise 8.5.3
Solve.
- {x2+y2=10x+y=4
- {x2+y2=5x−y=−3
- {x2+y2=30x−3y=0
- {x2+y2=102x−y=0
- {x2+y2=182x−2y=−12
- {(x−4)2+y2=254x−3y=16
- {3x2+2y2=213x−y=0
- {x2+5y2=36x−2y=0
- {4x2+9y2=362x+3y=6
- {4x2+y2=42x+y=−2
- {2x2+y2=1x+y=1
- {4x2+3y2=122x−y=2
- {x2−2y2=35x−3y=0
- {5x2−7y2=392x+4y=0
- {9x2−4y2=363x+2y=0
- {x2+y2=25x−2y=−12
- {2x2+3y=98x−4y=12
- {2x−4y2=33x−12y=6
- {4x2+3y2=12x−32=0
- {5x2+4y2=40y−3=0
- The sum of the squares of two positive integers is 10. If the first integer is added to twice the second integer, the sum is 7. Find the integers.
- The diagonal of a rectangle measures √5 units and has a perimeter equal to 6 units. Find the dimensions of the rectangle.
- For what values of b will the following system have real solutions? {x2+y2=1y=x+b
- For what values of m will be the following system have real solutions? {x2−y2=1y=mx
- Answer
-
1. (1,3),(3,1)
3. (−3√3,−√3),(3√3,√3)
5. (−3,3)
7. (−1,−3),(1,3)
9. (0,2),(3,0)
11. (0,1),(23,13)
13. (−3√5,−√5),(3√5,√5)
15. ∅
17. (−3+3√52,−6+3√5),(−3−3√52,−6−3√5)
19. (32,−1),(32,1)
21. 1,3
23. b∈[−√2,√2]
Exercise 8.5.4
Solve.
- {x2+y2=4y−x2=2
- {x2+y2=4y−x2=−2
- {x2+y2=4y−x2=3
- {x2+y2=44y−x2=−4
- {x2+3y2=9y2−x=3
- {x2+3y2=9x+y2=−4
- {4x2−3y2=12x2+y2=1
- {x2+y2=1x2−y2=1
- {x2+y2=14y2−x2−4y=0
- {x2+y2=42x2−y2+4x=0
- {2(x−2)2+y2=6(x−3)2+y2=4
- {x2+y2−6y=04x2+5y2+20y=0
- {x2+4y2=254x2+y2=40
- {x2−2y2=−104x2+y2=10
- {2x2+y2=14x2−(y−1)2=6
- {3x2−(y−2)2=12x2+(y−2)2=1
- The difference of the squares of two positive integers is 12. The sum of the larger integer and the square of the smaller is equal to 8. Find the integers.
- The difference between the length and width of a rectangle is 4 units and the diagonal measures 8 units. Find the dimensions of the rectangle. Round off to the nearest tenth.
- The diagonal of a rectangle measures p units and has a perimeter equal to 2q units. Find the dimensions of the rectangle in terms of p and q.
- The area of a rectangle is p square units and its perimeter is 2q units. Find the dimensions of the rectangle in terms of p and q.
- Answer
-
1. (0,2)
3. ∅
5. (−3,0),(0,−√3),(0,√3)
7. ∅
9. (0,1),(−2√55,−15),(2√55,−15)
11. (3,−2),(3,2)
13. (−3,−2),(−3,2),(3,−2),(3,2)
15. (−√7,0),(√7,0),(−√553,43),(√553,43)
17. 2,4
19. q+√2p2−q22 units by q−√2p2−q22 units
Exercise 8.5.5
Solve.
- {x2+y2=26xy=5
- {x2+y2=10xy=3
- {2x2−3y2=5xy=1
- {3x2−4y2=−11xy=1
- {x2+y2=2xy−2=0
- {x2+y2=12xy−1=0
- {4x−y2=0xy=2
- {3y−x2=0xy−9=0
- {2y−x2=0xy−1=0
- {x−y2=0xy=3
- The diagonal of a rectangle measures 2√10 units. If the area of the rectangle is 12 square units, find its dimensions.
- The area of a rectangle is 48 square meters and the perimeter measures 32 meters. Find the dimensions of the rectangle.
- The product of two positive integers is 72 and their sum is 18. Find the integers.
- The sum of the squares of two positive integers is 52 and their product is 24. Find the integers.
- Answer
-
1. (−5,−1),(5,1),(−1,−5),(1,5)
3. (−√3,−√33),(√3,√33)
5. ∅
7. (1,2)
9. (3√2,3√42)
11. 2 units by 6 units
13. 6,12
Exercise 8.5.6
Solve.
- {1x+1y=41x−1y=2
- {2x−1y=51x+1y=2
- {1x+2y=13x−1y=2
- {1x+1y=61x2+1y2=20
- {1x+1y=21x2+1y2=34
- {xy−16=02x2−y=0
- {x+y2=4y=√x
- {y2−(x−1)2=1y=√x
- {y=2xy=22x−56
- {y=32x−72y−3x=0
- {y=e4xy=e2x+6
- {y−e2x=0y−ex=0
- Answer
-
1. (13,1)
3. (75,7)
5. (−13,15),(15,−13)
7. (2,√2)
9. (3,8)
11. (ln32,9)
Exercise 8.5.7
- How many real solutions can be obtained from a system that consists of a circle and a hyperbola? Explain.
- Make up your own nonlinear system, solve it, and provide the answer. Also, provide a graph and discuss the geometric interpretation of the solutions.
- Answer
-
1. Answer may vary
Footnotes
32A system of equations where at least one equation is not linear.


