7.3: Adding an algorithm
- Page ID
- 34213
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Up until now, we have been creating schedules by guess-and-check, which works well enough for small schedules, but would not work well with dozens or hundreds of tasks. To create a more procedural approach, we might begin by somehow creating a priority list. Once we have a priority list, we can begin scheduling using that list and the list processing algorithm.
A priority list is a list of tasks given in the order in which we desire them to be completed.
The List Processing Algorithm turns a priority list into a schedule.
- On the digraph or priority list, circle all tasks that are ready, meaning that all pre-requisite tasks have been completed.
- Assign to each available processor, in order, the first ready task. Mark the task as in progress, perhaps by putting a single line through the task.
- Move forward in time until a task is completed. Mark the task as completed, perhaps by crossing out the task. If any new tasks become ready, mark them as such.
- Repeat until all tasks have been scheduled.
Using our digraph from above, schedule it using the priority list below:
\(\mathrm{T}_{1}, \mathrm{T}_{3}, \mathrm{T}_{4}, \mathrm{T}_{5}, \mathrm{T}_{6}, \mathrm{T}_{7}, \mathrm{T}_{8}, \mathrm{T}_{2}, \mathrm{T}_{9}\)
Solution
Time 0: Mark ready tasks
Priority list: \(({\mathrm{T}_{1}}), (\mathrm{T}_{3}), (\mathrm{T}_{4}), (\mathrm{T}_{5}), \mathrm{T}_{6}, \mathrm{T}_{7}, \mathrm{T}_{8}, \mathrm{T}_{2}, \mathrm{T}_{9}\)
We assign the first task, \(\mathrm{T}_{1}\) to the first processor, \(\mathrm{P}_{1}\), and the second ready task, \(\mathrm{T}_{3}\), to the second processor. Making those assignments, we mark those tasks as in progress:
Priority list: \(\cancel{(\mathrm{T}_{1})}, \cancel{(\mathrm{T}_{3})}, (\mathrm{T}_{4}), (\mathrm{T}_{5}), \mathrm{T}_{6}, \mathrm{T}_{7}, \mathrm{T}_{8}, \mathrm{T}_{2}, \mathrm{T}_{9}\)
Schedule up to here:
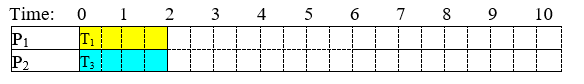
We jump to the time when the next task completes, which is at time 2.
Time 2: Both processors complete their tasks. We mark those tasks as complete. With Task 1 complete, Task 2 becomes ready:
Priority list: \(\xcancel{(\mathrm{T}_{1})}, \xcancel{(\mathrm{T}_{3})}, (\mathrm{T}_{4}), (\mathrm{T}_{5}), \mathrm{T}_{6}, \mathrm{T}_{7}, \mathrm{T}_{8}, (\mathrm{T}_{2}), \mathrm{T}_{9}\)
We assign the next ready task on the list, \(\mathrm{T}_{4}\) to \(\mathrm{P}_{1}\), and \(\mathrm{T}_{5}\) to \(\mathrm{P}_{2}\).
Priority list: \(\xcancel{(\mathrm{T}_{1})}, \xcancel{(\mathrm{T}_{3})}, \cancel{(\mathrm{T}_{4})}, \cancel{(\mathrm{T}_{5})}, \mathrm{T}_{6}, \mathrm{T}_{7}, \mathrm{T}_{8}, (\mathrm{T}_{2}), \mathrm{T}_{9}\)
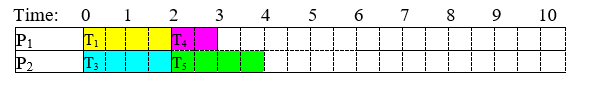
Time 3: Processor 1 has completed \(\mathrm{T}_{4}\). Completing \(\mathrm{T}_{4}\) does not make any other tasks ready (note that all the rest require that \(\mathrm{T}_{2}\) be completed first).
Priority list: \(\xcancel{(\mathrm{T}_{1})}, \xcancel{(\mathrm{T}_{3})}, \xcancel{(\mathrm{T}_{4})}, \cancel{(\mathrm{T}_{5})}, \mathrm{T}_{6}, \mathrm{T}_{7}, \mathrm{T}_{8}, (\mathrm{T}_{2}), \mathrm{T}_{9}\)
Since the next three tasks are not yet ready, we assign the next ready task, \(\mathrm{T}_{2}\) to \(\mathrm{P}_{1}\)
Priority list: \(\xcancel{(\mathrm{T}_{1})}, \xcancel{(\mathrm{T}_{3})}, \xcancel{(\mathrm{T}_{4})}, \xcancel{(\mathrm{T}_{5})}, \mathrm{T}_{6}, \mathrm{T}_{7}, \mathrm{T}_{8}, \cancel{(\mathrm{T}_{2})}, \mathrm{T}_{9}\)
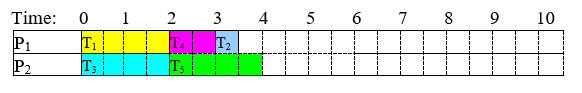
Time 3.5: Processor 1 has completed \(\mathrm{T}_{2}\). Completing \(\mathrm{T}_{2}\) causes \(\mathrm{T}_{6}\) and \(\mathrm{T}_{7}\) to become ready. We assign \(\mathrm{T}_{6}\) to \(\mathrm{P}_{1}\).
Priority list: \(\xcancel{(\mathrm{T}_{1})}, \xcancel{(\mathrm{T}_{3})}, \xcancel{(\mathrm{T}_{4})}, \xcancel{(\mathrm{T}_{5})}, \cancel{(\mathrm{T}_{6})}, (\mathrm{T}_{7}), \mathrm{T}_{8}, \xcancel{(\mathrm{T}_{2})}, \mathrm{T}_{9}\)
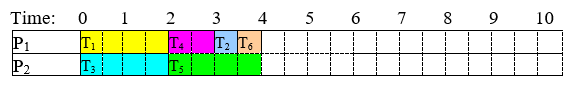
Time 4: Both processors complete their tasks. The completion of \(\mathrm{T}_{5}\) allows \(\mathrm{T}_{8}\) to become ready. We assign \(\mathrm{T}_{7}\) to \(\mathrm{P}_{1}\) and \(\mathrm{T}_{8}\) to \(\mathrm{P}_{2}\).
Priority list: \(\xcancel{(\mathrm{T}_{1})}, \xcancel{(\mathrm{T}_{3})}, \xcancel{(\mathrm{T}_{4})}, \xcancel{(\mathrm{T}_{5})}, \xcancel{(\mathrm{T}_{6})}, \cancel{(\mathrm{T}_{7})}, \cancel{(\mathrm{T}_{8})}, \xcancel{(\mathrm{T}_{2})}, \mathrm{T}_{9}\)
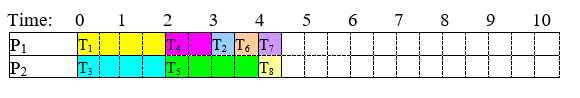
Time 4.5: Both processors complete their tasks. \(\mathrm{T}_{9}\) becomes ready, and is assigned to \(\mathrm{P}_{1}\). There is no ready task for \(\mathrm{P}_{2}\) to work on, so \(\mathrm{P}_{2}\) idles.
Priority list: \(\xcancel{(\mathrm{T}_{1})}, \xcancel{(\mathrm{T}_{3})}, \xcancel{(\mathrm{T}_{4})}, \xcancel{(\mathrm{T}_{5})}, \xcancel{(\mathrm{T}_{6})}, \xcancel{(\mathrm{T}_{7})}, \xcancel{(\mathrm{T}_{8})}, \xcancel{(\mathrm{T}_{2})}, \cancel{(\mathrm{T}_{9})}\)
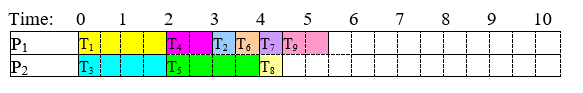
With the last task completed, we have a completed schedule, with finishing time 5.5 days.
Using the digraph below, create a schedule using the priority list:
\(\mathrm{T}_{1}, \mathrm{T}_{2}, \mathrm{T}_{3}, \mathrm{T}_{4}, \mathrm{T}_{5}, \mathrm{T}_{6}, \mathrm{T}_{7}, \mathrm{T}_{8}, \mathrm{T}_{9}\)
- Answer
-
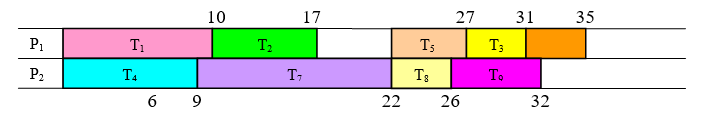
Time 0: Tasks 1, 4, and 7 are ready. Assign Tasks 1 and 4
Time 9: Task 4 completes. Only task 7 is ready; assign task 7
Time 10: Task 1 completes. Task 2 now ready. Assign task 2
Time 17: Task 2 completes. Nothing is ready. Processor 1 idles
Time 22: Task 7 completes. Tasks 5 and 8 are ready. Assign tasks 5 and 8
Time 26: Task 8 completes. Task 9 is ready. Assign task 9
Time 27: Task 5 completes. Tasks 3 and 6 are ready. Assign task 3
Time 31: Task 3 completes. Assign task 6
Time 35: Everything is done. Finishing time is 35 for this schedule.
It is important to note that the list processing algorithm itself does not influence the resulting schedule – the schedule is completely determined by the priority list followed. The list processing, while do-able by hand, could just as easily be executed by a computer. The interesting part of scheduling, then, is how to choose a priority list that will create the best possible schedule.


