1.26: Pyramids and Cones

Last updated
Save as PDF

Page ID: 153130

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

You may use a calculator throughout this module.

Pyramids

A pyramid is a geometric solid with a polygon base and triangular faces with a common vertex (called the apex of the pyramid). Pyramids are named according to the shape of their bases. The most common pyramids have a square or another regular polygon for a base, making all of the faces identical isosceles triangles. The height, $h$, is the distance from the apex straight down to the center of the base. Two other measures used with pyramids are the edge length $e$, the sides of the triangular faces, and the slant height $l$, the height of the triangular faces.

$Pyramid-variables.png$

Volume of a Pyramid

In general, the volume of a pyramid with base of area $B$ and height $h$ is

\[V=\dfrac{1}{3}Bh$ or $V=Bh\div3 \nonumber \]

If the base is a square with side length $s$, the volume is

\[V=\dfrac{1}{3}s^{2}h$ or $V=s^{2}h\div3 \nonumber \]

Interestingly, the volume of a pyramid is $\dfrac{1}{3}$ the volume of a prism with the same base and height.

Exercises

A pyramid has a square base with sides $16.0$ centimeters long, and a height of $15.0$ centimeters. Find the volume of the pyramid.
$Pyramid-template.gif$
The Great Pyramid at Giza has a height of $137$ meters and a square base with sides $23\overline{0}$ meters long.^[1] Find the volume of the pyramid.

The lateral surface area ($LSA$) of a pyramid is found by adding the area of each triangular face.

Lateral Surface Area of a Pyramid

If the base of a pyramid is a regular polygon with $n$ sides each of length $s$, and the slant height is $l$, then

\[LSA=\dfrac{1}{2}nsl$ or $LSA=nsl\div2 \nonumber \]

If the base is a square, then

\[LSA=2sl \nonumber \]

The total surface area ($TSA$) is of course found by adding the area of the base $B$ to the lateral surface area. If the base is a regular polygon, you will need to use the techniques we studied in Module 23.

Total Surface Area of a Pyramid

\[TSA=LSA+B \nonumber \]

If the base is a square, then

\[TSA=2sl+s^2 \nonumber \]

Exercises

A pyramid has a square base with sides $16.0$ centimeters long, and a slant height of $17.0$ centimeters. Find the lateral surface area and total surface area of the pyramid.
$Pyramid-template-slant-height.gif$
The Great Pyramid at Giza has a slant height of $179$ meters and a square base with sides $23\overline{0}$ meters long. Find the lateral surface area of the pyramid.

Cones

A cone is like a pyramid with a circular base. You may be able to determine the height $h$ of a cone (the altitude from the apex, perpendicular to the base), or the slant height $l$ (which is the length from the apex to the edge of the circular base). Note that the height, radius, and slant height form a right triangle with the slant height as the hypotenuse. We can use the Pythagorean theorem to determine the following equivalences.

$Cone-template-variables.png$

The slant height $l$, height $h$, and radius $r$ of a cone are related as follows:

\[l=\sqrt{r^2+h^2} \nonumber \]

\[h=\sqrt{l^2-r^2} \nonumber \]

\[r=\sqrt{l^2-h^2} \nonumber \]

Just as the volume of a pyramid is $\dfrac{1}{3}$ the volume of a prism with the same base and height, the volume of a cone is $\dfrac{1}{3}$ the volume of a cylinder with the same base and height.

Volume of a Cone

The volume of a cone with a base radius $r$ and height $h$ is

\[V=\dfrac{1}{3}\pi{r^2}h$ or $V=\pi{r^2}h\div3 \nonumber \]

Exercises

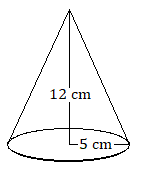
The base of a cone has a radius of $5.0$ centimeters, and the vertical height of the cone is $12.0$ centimeters. Find the volume of the cone.
$Cone-radius-5-12.gif$
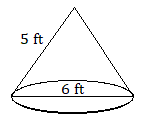
The base of a cone has a diameter of $6.0$ feet, and the slant height of the cone is $5.0$ feet. Find the volume of the cone.
$Cone-diameter-1.gif$

For the surface area of a cone, we have the following formulas.

Surface Area of a Cone

\[LSA=\pi{rl} \nonumber \]

\[TSA=LSA+\pi{r^2}=\pi{rl}+\pi{r^2} \nonumber \]

$Cone-LSA-net.png$ It’s hard to explain the $LSA$ formula in words, but here goes. The lateral surface of a cone, when flattened out, is a circle with radius $l$ that is missing a wedge. The circumference of this partial circle, because it matched the circumference of the circular base, is $2\pi{r}$. The circumference of the entire circle with radius $l$ would be $2\pi{l}$, so the part we have is just a fraction of the entire circle. To be precise, the fraction is $\dfrac{2\pi{r}}{2\pi{l}}$, which reduces to $\dfrac{r}{l}$. The area of the entire circle with radius $l$ would be $\pi{l^2}$. Because the partial circle is the fraction $\dfrac{r}{l}$ of the entire circle, the area of the partial circle is $\pi{l^2}\cdot\dfrac{r}{l}=\pi{rl}$.

Exercises

The base of a cone has a diameter of $6.0$ feet, and the slant height of the cone is $5.0$ feet. Find the lateral surface area and total surface area of the cone.
$Cone-diameter-1.gif$
The base of a cone has a radius of $5.0$ centimeters, and the vertical height of the cone is $12.0$ centimeters. Find the lateral surface area and total surface area of the cone.
$Cone-radius-5-12.gif$

en.Wikipedia.org/wiki/Great_Pyramid_of_Giza ↵

Search

Text Color

Text Size

Margin Size

Font Type

Exercises

Exercises

Exercises

Exercises