1.35: Exercise Answers

Last updated
Save as PDF

Page ID: 153140

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Module 1: Order of Operations

$7$
$13$
$7$
$13$
$2$
$8$
$18$
$6$
$25$
$49$
$80$
$31$
$28$
$67$
$22$
$4$
$160$
$19$
$2$
$12$
$40$
$200$
$2$
$14$
$9\cdot2+30=48$°F
$(72-30)\div2=21$°C

Module 2: Negative Numbers

$5$
$5$
$-15$
$-22$
$4$
$-4$
$-9$
$9$
$18$°F
$3$
$-200$
$3$
$-3$
$-7$
$-7$
$7$
$7$
$3$
$-3$
$55$
$55$
$11,123\text{ ft}$
$543\text{ ft}$
$-12$
$-40$
$18$
$21$
$4$
$-8$
$16$
$-32$
$-7$
$-4$
$9$
$0$
$0$
undefined
$19$
$-73$
$1$
$-6$
$-8$
$40$

Module 3: Decimals

If you’ve seen Modules 5 & 6, don’t worry about accuracy or precision on these exercises.

$90.23$
$7.056$
$16.55$
$184.015$
$8.28$
$15.756$
$4,147$
$414.7$
$41.47$
$4.147$
$65,625$
$65.625$
$6.5625$
$$656.25$
$243.5$
$2,435$
$243,500$
$24.35$
$6,000$
$6,380$
$0.71$
$0.715$
$$3.67$ per month
$7.5$ miles per hour

Module 4: Fractions

$\dfrac{11}{30}$
$\dfrac{19}{30}$
$\dfrac{12}{15}$
$\dfrac{8}{12}$
$7$
$1$
$0$
undefined
$\dfrac{3}{4}$
$\dfrac{5}{3}$
$2$
$\dfrac{1}{2}$
$\dfrac{5}{12}$
$1$
at least $45$ questions
$16$
$\dfrac{3}{5}$
$6$ scoops
A requires $\dfrac{1}{12}$ cup more than B
$\dfrac{1}{2}$ of the pizza
$\dfrac{2}{3}$ more
$\dfrac{5}{8}$ inches combined
$\dfrac{1}{8}$ inches difference
$\dfrac{7}{12}$ combined
$\dfrac{1}{12}$ more
$2.75$
$0.35$
$0.\overline5$ or $0.555…$
$1.\overline{63}$ or $1.636363…$
$11\dfrac{1}{2}$
$4\dfrac{2}{3}$
$\dfrac{11}{5}$
$\dfrac{20}{3}$
$10\dfrac{3}{8}$
$4\dfrac{7}{8}$
$8\dfrac{1}{6}$
$1\dfrac{5}{6}$ cup

Module 5: Accuracy and Significant Figures

exact value
approximation
exact value
approximation
exact value
approximation
three significant figures
four significant figures
five significant figures
two significant figures
three significant figures
four significant figures
two significant figures; the actual value could be anywhere between $28,500$ and $29,500$
three significant figures; the actual value could be anywhere between $28,950$ and $29,050$
four significant figures; the actual value could be anywhere between $28,995$ and $29,005$
five significant figures; the actual value could be anywhere between $28,999.5$ and $29,000.5$
$51,800$
$51,840$
$4.3$
$4.28$
$14,000$
$14,\overline{0}00$
$2.6$
$2.60$
$29,000\text{ ft}$
$29,\overline{0}00\text{ ft}$
$29,030\text{ ft}$
$29,032\text{ ft}$
$29,031.7\text{ ft}$
$107$
$640$
$14.4$
$12$
$$23$

Module 6: Precision and GPE

thousands
hundreds
tens
thousandths
ten thousandths
hundred thousandths
$82,000$
$82,\overline{0}00$
$82,0\overline{0}0$
$0.6$
$0.60$
$0.600$
$39.3$ lb
$39$ lb
$$9,800$
$$8\overline{0}0$
thousands place; the nearest $1,000$ people
$\pm 500$ people
hundred thousandths place; the nearest $0.00001$ in
$\pm 0.000005$ in
hundredths place; the nearest $0.01$ mil
$\pm 0.005$ mil
$30\overline{0}$ miles
ones place; the nearest $1$ mile
$\pm 0.5$ mi
ones place; the nearest $1$ minute
$\pm 0.5$ min
two sig figs
three sig figs
$55$ mi/hr
When dividing, we must round the result based on the accuracy; i.e., the number of significant figures.

Module 7: Formulas

$$2.46$
$$4.14$
$$17.80$
$$24.80$
$$3.80$
$6$ representatives
$10$ representatives
$52$ representatives
$8$ electoral votes
$12$ electoral votes
$54$ electoral votes
$\approx115$°F; the official record high in the city was 116°F.
$37$°C
$-0.4$°F
$200$°C
$90$ mm Hg
around $107$ mm Hg
$70$ in
$74$ in
yes
no; too large
no; too small
yes

Module 8: Perimeter and Circumference

$70$ ft
$58$ cm
$28$ cm
$104$ ft
$130$ ft
$56$ ft
$24$ in
$20$ cm
$28.3$ in
$18.8$ cm
$44.0$ ft
$53$ m

Module 9: Percents Part 1

$47\%$
$53\%$
$\dfrac{71}{100}$
$\dfrac{1.3}{100}=\dfrac{13}{1000}$
$\dfrac{0.04}{100}=\dfrac{1}{2500}$
$\dfrac{106}{100}=\dfrac{53}{50}$
$0.71$
$0.013$
$0.0004$
$1.06$
$23\%$
$7\%$
$8.5\%$
$250\%$
$28\%$
$12.5\%$
$31.5$
$22.5$
$67.5$
$100$
$38.6$
$2.25$
$$9.35$
$$119.32$

Module 10: Ratios, Rates, Proportions

$\dfrac{3}{8}$
$\dfrac{105\text{ mi}}{2\text{ hr}}$
$\dfrac{52.5\text{ mi}}{1\text{ hr}}$ or $52.5$ miles per hour
$\approx$0.335$/oz, or $33.5$¢/oz
$Carmella-Creeper-300x175.png$
Carmella Creeper parties like it’s 1995.
$\approx$0.281$/oz, or $28.1$¢/oz
$\approx$0.281$/oz, or $28.1$¢/oz
$\dfrac{3}{4}=\dfrac{3}{4}$; true
$\dfrac{2}{3} \neq \dfrac{4}{5}$; false
$168 = 168$; true
$200 \neq 240$; false
$70 \neq 60$; false
$20 = 20$; true
$x = 12$
$n = 5$
$k = 4$
$w = 10$
$x = 10.4$
$m = 2.0$
$256$ miles
$20$ hours
$\approx 50$ miles (rounding to one sig fig seems like a good idea here)
$190$ pixels wide

Module 11: Scientific Notation

Earth’s mass is larger because it’s a $25$-digit number and Mars’ mass is a $24$-digit number, but it might take a lot of work counting the zeros to be sure.
Earth’s mass is about ten times larger, because the power of $10$ is $1$ higher than that of Mars.
A chlorine atom’s radius is larger because it has $9$ zeros before the significant digits begin, but a hydrogen atom’s radius has $10$ zeros before the significant digits begin. As above, counting the zeros is a pain in the neck.
The chlorine atom has a larger radius because its power of $10$ is $1$ higher than that of the hydrogen atom. (Remember that $-10$ is larger than $-11$ because $-10$ is farther to the right on a number line.)
$$100,000$; $1.00000\times10^5$
$$999,999$; $9.99999\times10^5$
$1.234 \times 10^3$
$1.02 \times 10^7$
$8.70 \times 10^{-4}$
$7.32 \times 10^{-2}$
$35,000$
$90,120,000$
$0.00825$
$0.000014$
$8 \times 10^7$
$3.5 \times 10^{13}$
$6 \times 10^{-5}$
$4.8 \times 10^5$
$1,260$ people per square mile
$250$ people per square mile
the proton’s mass is roughly $1,830$ or $1.83 \times 10^3$ times larger
$335.9 \times 10^6$; $3.359 \times 10^8$
$8.020 \times 10^9$; $8.020 \times 10^9$
$33.9 \times 10^{12}$; $3.39 \times 10^{13}$
$\approx$101,000$ per person

Module 12: Percents Part 2 and Error Analysis

$93\%$ or $93.3\%$
$37.5\%$
$$2,500$
$720$
$93\%$ or $93.3\%$
$37.5\%$
$$2,500$
$720$
$44.8\%$ or $45\%$
$50$ grams of added sugars is the recommended daily intake for a $2,000$ calorie diet.
$56\%$ increase
$10.1\%$ sales tax
$36\%$ decrease
$2.7\%$ decrease
$0.1875\div25\approx0.75\%$
$0.13\div10.8\approx1.2\%$
$4.806$ g; $5.194$ g
$3.88\%$
$5.443$ g; $5.897$ g
$4.00\%$

Module 13: The US Measurement System

We generally won’t worry about significant figures in these answers; we’ll probably say “$2$ miles” even if “$2.000$ miles” is technically correct.

$54$ in
$54$ ft
$36$ in
$1,760$ yd
$14\dfrac{2}{3}$ ft or $14$ ft $8$ in
$15$ yd
$2$ mi
$30$ yd
$40$ oz
$2,400$ lb
18.75 lb
$32,000$ oz
$48$ fl oz
$7$ pt
$8$ pt
$5$ c
$1.25$ gal
$64$ fl oz
$3$ lb $7$ oz
$7$ c $3$ fl oz
$15$ ft $2$ in
$4$ t $500$ lb
$20$ lb or $20$ lb $0$ oz combined
$3$ lb $2$ oz heavier
$9$ ft $1$ in combined
$1$ ft $5$ in longer

Module 14: The Metric System

$5$ m
$28$ cm
$3.8$ km
$1.6$ m
$160$ cm
$3$ mm
$536$ cm
$5,360$ mm
$1,609$ m
$160,900$ cm
$0.297$ m
$29.7$ cm
$0.828$ km
$82.8$ dam
$100$ g
$80$ kg
$500$ mg
$2,000$ kg
$2,270$ g
$2,270,000$ mg
$6,500$ cg
$65,000$ mg
$0.065$ kg
$9.5$ cg
$0.095$ g
$50$ L
$30$ mL
$0.5$ L
$10.5$ dL
$1,750$ mL
$0.25$ L
they are equal in size
about $11$ to $1$
$4$ bottles; this is easier if you know that a 500-milliliter bottle of Mexican Coke is called a medio litro.
$Medio-Litro-300x225-1.jpg$
Doce litros de Coca-Cola

Module 15: Converting Between Systems

We may round some of these answers to three significant figures even if the given number has fewer than three sig figs. On the other hand, you’ll see that some of these answers include a critique of a manufacturer’s decision to round numbers a certain way.

$31\text{ mi}$
$183\text{ cm}$
$164.0\text{ ft}$
yes, $4\text{ in}=101.6\text{ mm}$ according to the conversion, but is it really $4.000\text{ in}$ to begin with? Rounding the result to $100\text{ mm}$ or $102\text{ mm}$ seems reasonable.
$20$ in converts to around $50.8$ cm, and $50.0$ cm converts to around $19.7$ in. It looks like somebody used the conversion $1\text{ in}=2.5\text{ cm}$, which is fine if you’re estimating but not if you’re going to report a number to three sig figs.
not exactly but they’re pretty close; the error is around $0.3\%$.
yes; using either conversion gives a result of $294.8$ kg, rounded to the nearest tenth. However, it doesn’t make sense for this to be accurate to four sig figs. It would be best to round to $295$ or perhaps even $3\overline{0}0$ kg.
not exactly; using 1 kg $\approx 2.205$ lb gives a result of $1,473.9$ kg, and using 1 lb $\approx 0.4536$ kg gives a result of $1,474.2$ kg. Again, the accuracy here doesn’t make sense, especially when you consider that the numbers on the box don’t agree with each other: $294.8\cdot5\neq1,474.1$.
$11\text{ lb}$
$\approx230\text{ g}$
$\approx110\text{ g}$
$\approx1.8\text{ oz}$
about $$1.09$
about $$0.99$
$\approx1.3\text{ gal}$
$355\text{ mL}$
$3.4\text{ fl oz}$
no matter which conversion you use, the result should round to $22.7$ liters.
a bit less than $600\text{ km}$
$11\text{ km/L}$

Module 16: Other Conversions

We will generally round these answers to three significant figures; your answer may be slightly different depending on which conversion ratio you used.

$525,600$ min; if you’re familiar with the musical Rent, then you already knew the answer.
this is roughly $31.7$ years, which is indeed possible
$37.6$ km/hr
$23.3$ mi/hr
$1,770$ mi/hr
$29.5$ mi in $1$ min
$20.3$ min
$0.17$ mi/gal
$5.8$ gal/mi
$171$ gal in $1$ min
the capacity increased by a factor of $14.4$
$4$ times greater
$1,200$ megawatts per home
$1$ watt per gallon
$2,500$ times more powerful
$0.4$ ms, $0.04$ ms, $0.004$ ms; $400$ μs, $40$ μs, $4$ μs
the ratio of the wavelengths of red and infrared is $7$ to $100$;
the ratio of the wavelengths of infrared and red is around $14$ to $1$
this is equivalent to $2,500$ chest x-rays

Module 17: Angles

right angle
obtuse angle
reflexive angle
straight angle
acute angle
$a=127^\circ$; $b=53^\circ$; $c=127^\circ$
$27^\circ$
$97^\circ$
$23^\circ$ each
$45^\circ$ each
$60^\circ$ each
$A=61^\circ$; $B=80^\circ$; $C=39^\circ$
$18.9111^\circ$
$155.6808^\circ$
$34.1924^\circ$
$29^\circ58’30”$
$31^\circ8’15”$
$76^\circ20’48.1”$

Module 18: Triangles

right isosceles triangle
obtuse scalene triangle
acute equilateral triangle (yes, an equilateral triangle will always be acute)
$w=35\text{ ft}$
$x=8\text{ cm}$; $y=10.5\text{ cm}$
$d=268\text{ ft}$
$n=55\text{ cm}$
this is a right triangle, because $5^2+12^2=13^2$.
this is not a right triangle, because $8^2+17^2\neq19^2$.
$7.07$
$17.20$
$30.71$
$10\text{ ft}$
$15\text{ ft}$
$12.3\text{ cm}$
$1.8\text{ cm}$

Module 19: Area of Polygons and Circles

We may occasionally include extra sig figs in these answers so you can be sure that your answer matches ours.

$20\text{ cm}^2$
$16\text{ cm}^2$
$4.86\text{ m}^2$
$12.25\text{ ft}^2$
$120\text{ in}^2$
$360\text{ m}^2$
$210\text{ ft}^2$
$126\text{ cm}^2$
$38.5\text{ cm}^2$
$204\text{ ft}^2$
$2,160\text{ in}^2$
$36\text{ m}^2$
$124\text{ cm}^2$
$192\text{ cm}^2$
$28.3\text{ cm}^2$
$220\text{ m}^2$
$154\text{ ft}^2$
$63.6\text{ in}^2$

Module 20: Composite Figures

$64\text{ ft}$
$189\text{ ft}^2$
$590\text{ cm}^2$; the area of the rectangle is $800\text{ cm}^2$ and the areas of the triangles are $70\text{ cm}^2$ and $140\text{ cm}^2$.
$590\text{ cm}^2$; hey, that’s what we got for #3!
$148\text{ m}$
$940\text{ m}^2$
Based on the stated measurements, the distance around the track will be 401 meters, which appears to be 1 meter too long. In real life, precision would be very important here, and you might ask for the measurements to be given to the nearest tenth of a meter.
around $9,620\text{ m}^2$
$1,960\text{ cm}^2$
$178.5\text{ cm}$
$29\text{ ft}^2$
$47\text{ ft}$
around $21.5\%$ (Hint: Make up an easy number for the side of the square, like 2 or 10.)
around $36.3\%$ (Hint: The diagonals of the square are equal to the circle’s diameter.)

Module 21: Converting Units of Area

We may occasionally include extra sig figs in these answers so that you can be sure that your answer matches ours.

$162\text{ ft}^2$
$162\text{ ft}^2$
$7\text{ ft}^2$
$7\text{ ft}^2$
$7,776\text{ in}^2$
$8.3\text{ ac}$
$180,000\text{ cm}^2$
$180,000\text{ cm}^2$
$623.7\text{ cm}^2$
$623.7\text{ cm}^2$
$6\text{ m}^2$
$4\text{ ha}$
$376\text{ km}^2$
$2,\overline{0}00\text{ ha}$, rounded to two sig figs
$603\text{ cm}^2$
$75,300\text{ ft}^2$, rounded to three sig figs
$154\text{ in}^2$
$38.5\text{ in}^2$
$4$ to $1$; if we double the linear measurement, we get four times the area.
$54\text{ cm}^2$
$6\text{ cm}^2$
$9$ to $1$; if we triple the linear measurement, we get nine times the area.

Module 22: Surface Area of Common Solids

$36\text{ cm}^2$
$76\text{ cm}^2$
$471\text{ cm}^2$
$628\text{ cm}^2$
$616\text{ cm}^2$
$380\text{ in}^2$

Module 23: Area of Regular Polygons

All answers have been given to two or three significant figures.

$6,900\text{ in}^2$
$94\text{ cm}^2$
$750\text{ in}^2$
$751\text{ mm}^2$
$480\text{ cm}^2$
$110\text{ m}^2$
$280\text{ mm}^2$ (the area of the circle $\approx1,660\text{ mm}^2$ and the area of the hexagon is $1,380\text{ mm}^2$)

Module 24: Volume of Common Solids

$40\text{ cm}^3$
$531\text{ cm}^3$
$45\text{ cm}^3$
$350\text{ cm}^3$
$520\text{ cm}^3$
$22,600\text{ ft}^3$
$3.7\text{ mm}^3$
$1,440\text{ cm}^3$
$697\text{ in}^3$ or $7\overline{0}0\text{ in}^3$
$22.7\text{ cm}^3$ (the cylinder’s volume $\approx14.1\text{ cm}^3$ and the hemisphere’s volume $\approx8.6\text{ cm}^3$.)
$37.6\text{ ft}^3$ (the cylinder’s volume $\approx29.45\text{ ft}^3$ and the two hemispheres’ combined volume $\approx8.18\text{ ft}^3$)
$37.6\text{ ft}^3\approx282\text{ gal}$, which is more than $250\text{ gal}$.

Module 25: Converting Units of Volume

the result is very close to $1$ cubic yard: $(112\text{ in}\cdot14\text{ in}\cdot10\text{ in})\cdot3\text{ crates}=47,040\text{ in}^3\approx1.01\text{ yd}^3$
this estimate is also $1$ cubic yard: $(9\text{ ft}\cdot1\text{ ft}\cdot1\text{ ft})\cdot3\text{ crates}=27\text{ ft}^3=1\text{ yd}^3$
around $60$ gallons
yes, the can is able to hold $12$ fluid ounces; the can’s volume is roughly $23.3\text{ in}^3\approx12.9\text{ fl oz}$.
$5$ gallons
around $2,300$ to $2,400$ liters; a calculator says $2,356$ liters which should technically be rounded up to $2,400$ liters, but it would be reasonable to round down to $2,300$ liters instead if you considered the volume of the benches and the fact that the sides might slope inwards near to bottom of the tub.
the rectangular section of the carton has a volume of $1.7$ liters, which is larger than the required $1.5\text{ L}$.
$\approx21\text{ cm}$ high
$POG-Juice-Height-300x300-1.jpg$
Independent verification from my kitchen.
$19.6\text{ yd}^3$
$53\overline{0}\text{ ft}^3$
$1.53\text{ m}^3$
$327\text{ in}^3$
$5,350\text{ cm}^3$
$5.35\text{ L}$
$0.5\text{ ft}^3\approx14.16\text{ L}$; assuming that it’s $0.50\text{ ft}^3$, these measures are equivalent to two sig figs.
$100\text{ lb/ft}^3$
$31\text{ lb}$; now you can laugh whenever you see someone in a heist movie load a cheap duffel bag with gold bars and carry it out of the vault.
$1.1\text{ kg}$
$268\text{ cm}^3$
$33.5\text{ cm}^3$
$8$ to $1$
$1,620\text{ in}^3$
$60\text{ in}^3$
$27$ to $1$

Module 26: Pyramids and Cones

$1,280\text{ cm}^3$
$2,420,000\text{ m}^3$
$544\text{ cm}^2$; $80\overline{0}\text{ cm}^2$
$82,300\text{ m}^2$
$310\text{ cm}^3$ (if we had greater accuracy, the result would be 314.16 because it’s 100 times $\pi$.)
$38\text{ ft}^3$
$47\text{ ft}^2$; $75\text{ ft}^2$
$2\overline{0}0\text{ cm}^2$; $280\text{ cm}^2$

Module 27: Percents Part 3

$$1,299.00$
$14,861$ students; notice that if the percent had fewer than five sig figs, we wouldn’t have been able to get an answer that was accurate to the nearest whole number.
Yes, you can! Each bottle cost $$3.59$.
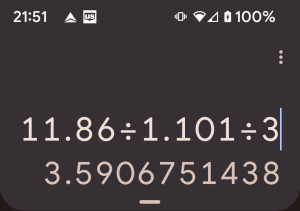
$Screenshot_20230901-215146-300x211-1.png$
Real time screenshot of my phone’s calculator.
$$3.20$ million
$873,900$ people
$$16$; the percent has only two sig figs, so it doesn’t make sense to assume that the price was $$16.13$. They probably rounded the percent from $68.75\%$ to make the numbers in the advertisement seems less complicated.

Module 28: Mean, Median, Mode

$10.3\text{ min}$
$$4.488$
$11.0\text{ min}$ (because it is the seventh value in the list of thirteen)
$$4.475$
$$256,000$
$$250,000$
$$338,000$
$$275,000$
the median is more representative because the mean is higher than five of the six home values.
$11.0\text{ min}$ (because it appears four times in the list)
no mode (there are no repeated values)
AT&T Mobility
Samoas and Thin Mints
$12.2$ games
$12$ games
$12$ games
they all represent the data fairly well; $12$ wins represents a typical Patriots season.
$6.8$ games
$7$ games
$6$ games
they all represent the data fairly well; $6$ or $7$ wins represents a typical Bills season.
$98.2$ grams; the mean doesn’t seem to represent a typical clementine because there is a group of smaller ones (from $82$ to $94$ grams) and a group of larger ones (from $102$ to $109$ grams) with none in the middle.
$94$ grams; for the same reason, the median doesn’t represent a typical clementine, but you could say it helps split the clementines into a lighter group and a heavier group.
no mode; too many values appear twice.
$98.3$ grams; this is a small increase over the previous mean.
$94$ grams; the median does not change when one of the highest numbers increases.
$109$ grams; you might say it represents the mass of a typical large clementine, but it doesn’t represent the entire group.

Module 29: Probability

$\dfrac{6}{36}=\dfrac{1}{6}$
$\dfrac{2}{36}=\dfrac{1}{18}$
$\dfrac{8}{36}=\dfrac{2}{9}$
$\dfrac{36}{36}=1$
$\dfrac{0}{36}=0$
$\dfrac{4}{36}=\dfrac{1}{9}$
$\dfrac{32}{36}=\dfrac{8}{9}$
$\dfrac{6}{36}=\dfrac{1}{6}$
$\dfrac{30}{36}=\dfrac{5}{6}$
$\dfrac{15}{60}=\dfrac{1}{4}=0.25=25\%$
$\dfrac{45}{60}=\dfrac{3}{4}=0.75=75\%$
$\dfrac{18}{60}=\dfrac{3}{10}=0.3=30\%$
$\dfrac{42}{60}=\dfrac{7}{10}=0.7=70\%$
$\dfrac{1}{4}=25\%$
$\dfrac{3}{4}=75\%$
$\dfrac{2}{4}=50\%$
$\dfrac{2}{4}=50\%$
$\dfrac{8}{250}=3.2\%$
$\dfrac{242}{250}=96.8\%$
we should expect $968$ copies to be acceptable
$\dfrac{44}{200}=22\%$
we should expect $220$ tax returns to have errors
$22\%=0.22$
$(0.22)^2\approx4.8\%$
$(0.22)^3\approx1.1\%$
$0.22\cdot0.78\approx17.2\%$
$0.78\cdot0.22\approx17.2\%$
$(0.78)^2\approx60.8\%$
$(0.78)^3\approx47.5\%$
$1-(0.78)^3\approx52.5\%$

Module 30: Standard Deviation

$60.5$; $66.5$
$57.5$; $69.5$
$54.5$; $72.5$
$66.5$; $72.5$
$63.5$; $75.5$
$60.5$; $78.5$
$68\%$ because $100\%-(16\%+16\%)=68\%$
$195$ lb because this is halfway between $160$ and $230$ lb
$35$ lb because $195-35$ lb and $195+35$ lb encompasses $68\%$ of the data
$125$; $265$
$8.8$; $15.6$
You would not have predicted this from the data because it is more than two standard deviations below the mean, so there would be a roughly $2.5\%$ chance of this happening randomly. In fact, $(12.2-7)\div1.7$ is slightly larger than $3$, so this is more than three standard deviations below the mean, making it even more unlikely. (You might have predicted that the Patriots would get worse when Tom Brady left them for Tampa Bay, but you wouldn’t have predicted only $7$ wins based on the previous nineteen years of data.)
$3.4$; $10.2$
You would not predict this from the data because it is more than two standard deviations above the mean, so there would be a roughly $2.5\%$ chance of this happening randomly. In fact, $(13-6.8)\div1.7\approx3.6$, so this is more than three standard deviations above the mean, making it even more unlikely. This increased win total is partly due to external forces (i.e., the Patriots becoming weaker and losing two games to the Bills) but even $11$ wins would have been a bold prediction, let alone $13$.
$3.9$; $14.3$
The trouble with making predictions about the Broncos is that their standard deviation is so large. You could choose any number between $4$ and $14$ wins and be within the $95\%$ interval. $(9.1-5)\div2.6\approx1.6$, so this is around $1.6$ standard deviations below the mean, which makes it not very unusual. Whereas the Patriots and Bills are more consistent, the Broncos’ win totals fluctuate quite a bit and are therefore more unpredictable.

Module 31: Right Triangle Trigonometry

the adjacent side is e, the opposite side is f, and the hypotenuse is d.
the adjacent side is x, the opposite side is y, and the hypotenuse is r.
$\dfrac{3}{5}=0.6$
$\dfrac{4}{5}=0.8$
$\dfrac{3}{4}=0.75$
$\dfrac{4}{5}=0.8$
$\dfrac{3}{5}=0.6$
$\dfrac{4}{3}\approx1.333$
$0.6000$
$0.8000$
$0.7500$
$0.8000$
$0.6000$
$1.333$
$z\approx4.6\text{ cm}$
$g\approx2.6\text{ cm}$
$b\approx5.706\text{ in}$
$p\approx75.51\text{ mm}$
$y\approx136.18\text{ mm}$
$d\approx296.87\text{ mm}$
the wire is approximately $27\text{ ft}$ long
$\approx17.85\text{ ft}$, which is roughly $17\text{ ft}, 10\text{ in}$
No; $64\cdot\text{tan }1^\circ\approx1.1\text{ ft}$, so the puck will hit the fabric over 1 foot away from the center of the hole. (The person in the photo was given a bunch of pucks and 30 seconds to score, but he scored on his first shot. Boston Bruins at Vancouver Canucks, February 24, 2024.)
$36.87^\circ$
$60^\circ$
$53.13^\circ$
$\angle A\approx36.47^\circ$
$\angle1\approx42.03^\circ$
$\angle1\approx30.76^\circ$
$\angle y\approx52.88^\circ$
$\angle1\approx55.28^\circ$
$\angle x\approx31.50^\circ$; $\angle y\approx58.50^\circ$
$\text{tan}^{-1}\left(\dfrac{4}{1}\right)\approx76^\circ$ angle of elevation
Yes; $\text{sin}^{-1}\left(\dfrac{2}{25}\right)\approx4.59^\circ$, which is less than $4.75^\circ$.
$\text{tan}^{-1}\left(\dfrac{17}{14}\right)\approx50.5^\circ$
$17\div\text{sin }51^\circ\approx22.0$
$14\div\text{cos }51^\circ\approx22.0$
$\sqrt{14^2+17^2}\approx22.0$
All three answers are the same rounded to three significant figures. This is true because we rounded $\angle A$ to the nearest tenth; if we had rounded it to $51^\circ$ instead of $50.5^\circ$, we would have decreased the accuracy of #36 & #37 to only two sig figs and the three results all would have been slightly different.
$\approx23^\circ$
$\approx8,900\text{ ft}$

Module 32: Slope

$0.36\text:12$, $0.03$, $3\%$
$0.60\text:12$, $0.05$, $5\%$
$1.00\text:12$, $0.0833$, $8.33\%$
$2.16\text:12$, $0.18$, $18\%$
$1.72^\circ$
$2.86^\circ$
$4.76^\circ$
$10.20^\circ$
$0.5\text{ ft}$
$1\text{ ft}$
$5\text{ ft}$
$\text{tan}^{-1}\left(20/12\right)\approx59^\circ$ from vertical. (this would be an angle of depression of $31^\circ$.)
$30\text{ ft}$; the proportion $\dfrac{1}{12}=\dfrac{2.5}{x}$ gives a result of exactly $30\text{ ft}$.
Using the result from #7, the equation $\text{tan}\left(4.76^\circ\right)=\dfrac{2.5}{x}$ gives a result very close to $30.0\text{ ft}$.

Module 33: Non-Right Triangle Trigonometry

$k\approx61\text{ in}$
$a=c=74^\circ$; $x\approx5.168\text{ in}$
$x\approx11.6\text{ cm}$; $y\approx12.3\text{ cm}$
the shorter wire is roughly $23.5$ feet; the longer wire is roughly $26$ feet.
$a photograph of the actual utility pole that is described in exercise 4$
Exercise 4 is based on actual events!
$n\approx72.4^\circ$
$n\approx107.6^\circ$
$d\approx65\text{ mm}$
$p\approx11.4\text{ cm}$
$w\approx14.12\text{ m}$
the distance across the pond is roughly $81.5$ meters.
$X\approx19.4^\circ$; $Y\approx133.5^\circ$; $Z\approx27.1^\circ$

Module 34: Radian Measure

$\dfrac{\pi}{6}$
$\dfrac{\pi}{4}$
$\dfrac{\pi}{3}$
$\dfrac{\pi}{2}$
$\dfrac{2\pi}{3}$
$\dfrac{5\pi}{4}$
$\dfrac{4\pi}{3}$
$\dfrac{3\pi}{2}$
$15^\circ$
$135^\circ$
$150^\circ$
$315^\circ$
$\approx57.3^\circ$

Search

Text Color

Text Size

Margin Size

Font Type