3.4: Subtraction

You will need: A Calculator, Base Blocks (Material Cards 4-15) C-Strips (Material Cards 16A-16L)

Exercise 1

How would you use manipulative to explain how to do subtraction to a young child?

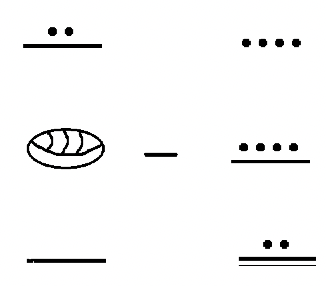
There are two distinct approaches to subtraction. The one most of us are familiar with is the Take-Away Method. A typical way someone might introduce the idea of subtraction is by saying "If I put five apples in the bowl and then take away two of the apples, how many are left in the bowl?" The illustration below shows this as a subtraction problem where after two apples are removed from the bowl, there are three apples remaining.

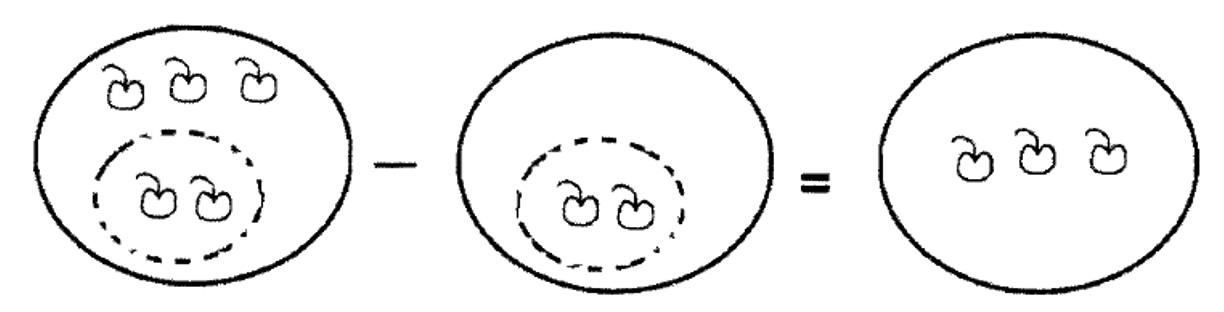
The above problem illustrates the Take-Away Approach to Subtraction.

Subtraction Vocabulary: For x – y = z, x is called the minuend, y is called the subtrahend, and z (the answer) is called the difference.

Exercise 2

For the subtraction problem 7 – 3, State the

Definition: Subtraction of Whole Numbers using Set Theory

If B is a subset of A, then n(A) – n(B) = n(A – B)

Exercise 3

In your own words, explain how to use the definition of subtraction. What are the steps involved?

Examples of how to do subtraction using the Set Theory Definition:

Example 1

Use the set theory definition of subtraction to show that 5 – 2 = 3.

Let A = {v, w, x, y, z} and B = {w, z}. Since n(A) = 5, n(B) = 2 and B \(\subseteq\) A,

\[\begin{aligned} 5 – 2 &= n(A) – n(B) && \text{ by substituting } n(A) \text{ for 5 and } n(B) \text{ for 2} \\ &= n(A – B) && \text{ by the set theory definition of subtraction} \\ &= n(\{v,x,y\}) && \text{ by computing }A – B \\ &= 3 && \text{ by counting the elements in } A – B \end{aligned} \nonumber \]

Therefore, 5 – 2 = 3.

Exercise 2

Use the set theory definition of subtraction to show that 6 – 1 = 5.

Let A {1, 2, 3, 4, 5, 6} and B = {4}. Since n(A) = 6, n(B) = 1 and B \(\subseteq\) A,
\[\begin{aligned} 6 – 1 &= n(A) – n(B) && \text{ by substituting } n(A) \text{ for 6 and } n(B) \text{ for 1} \\ &= n(A – B) && \text{ by the set theory definition of subtraction} \\ &= n(\{1, 2, \mathbf{3}, 5, 6\}) && \text{ by computing }A – B \\ &= 5 && \text{ by counting the elements in } A – B \end{aligned} \nonumber \]

Therefore, 6– 1 = 5.

Exercise 3

Use the set theory definition of subtraction to show that 3 – 0 = 3.

Let A = {x,y, z} and B= {}. Since n(A)=3, n(B)=0 and B \(\subseteq\) A,

\[\begin{aligned} 3 – 0 &= n(A) – n(B) && \text{ by substituting } n(A) \text{ for 3 and } n(B) \text{ for 0} \\ &= n(A – B) && \text{ by the set theory definition of subtraction} \\ &= n(\{x,y,z\}) && \text{ by computing }A – B \\ &= 3 && \text{ by counting the elements in } A – B \end{aligned} \nonumber \]

Therefore, 3 – 0 = 3.

Exercise 4

For each subtraction problem below, provide two sets that allow you to use the definition of subtraction to find the answer. Then, compute the answer using this definition.

1. 7 - 3
2. 6 - 0
3. 4 - 4

Parts b and c of Exercise 4 illustrate two familiar properties of subtraction.

The first property states that for any whole number \(m\), \(m – 0 = m\). Using our knowledge of Set Theory, choose the first set A to have m elements and then choose a second set B that has zero elements – there is only one set you can choose and that is the null or empty set, { }. Then, A – { } = A. Therefore, it is a fact that m – 0 = 0.

The second property states that for any whole number m, m – m = 0. This must be true because for any set A, whether it has m elements or any other number of elements, we know from Set Theory that A – A = { }.

We will use the Take-Away approach to perform subtraction problems in Egyptian now.

You are reminded of the symbols and their Hindu-Arabic equivalents below:

| (1)

(10)

(100)

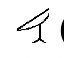(1,000)

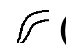(10,000)

(100,000)

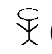(1,000,000)

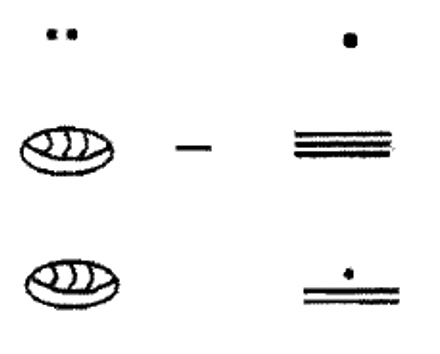
To use the Take-Away approach, we want to see the subtrahend as a subset of the minuend and then remove the subtrahend from the minuend. The symbols that remain in the minuend is the difference. In this first example, notice how the following subtraction is performed. In this case, you can see the subtrahend as a subset of the minuend. A box is put around what is to be taken away and the final answer is clear.

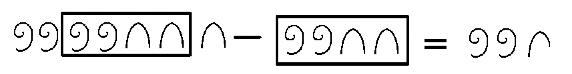
Sometimes, exchanges have to be made in the minuend before the subtraction can be done. For instance, consider the following subtraction:

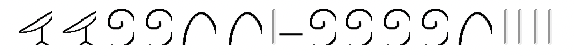
The first step would be to make some exchanges in the minuend. One lotus flower must be exchanged for ten scrolls and one heel bone must be exchanged for ten staffs. After doing that, the subtraction can be performed as shown below:

In the above example, put a box around the subset that is being removed from the minuend.

The next subtraction will be

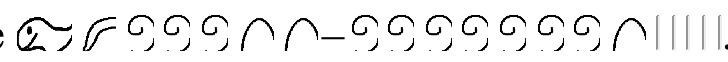
. This time the subtraction will be shown as a vertical problem with the minuend and subtrahend shown enclosed in a box. In order to do the subtraction, first the pointed finger in the minuend must be exchanged for ten lotus flowers. Then, one lotus flower must be exchanged for ten scrolls and one heel bone must be exchanged for ten strokes. Finally, the subset (the subtrahend) is taken away from the minuend. I've traced out what is being removed. The symbols remaining in the minuend is the answer (

) as shown in the illustration below.

Exercise 5

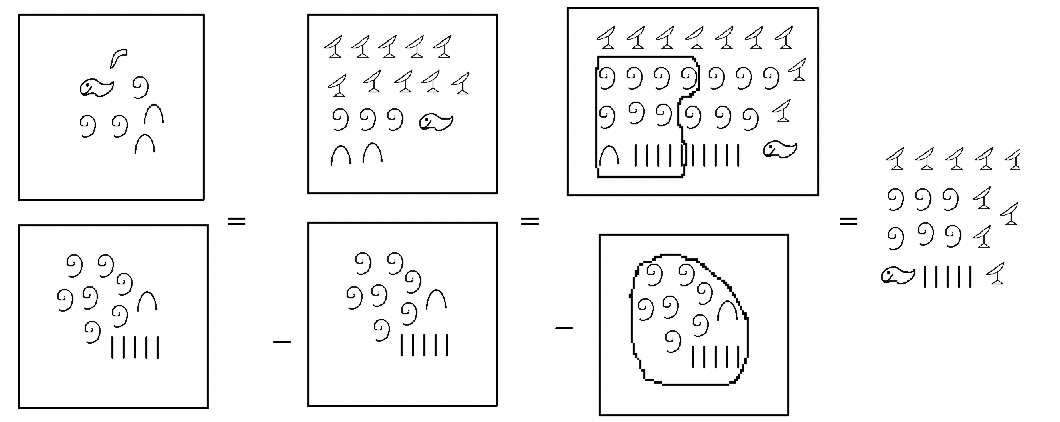
Perform the following subtraction problems in Egyptian, showing all steps. You can model the problem however you like as long as the steps are clear.

a.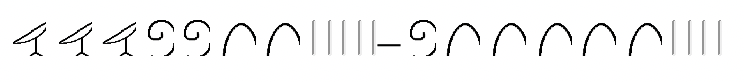

b.

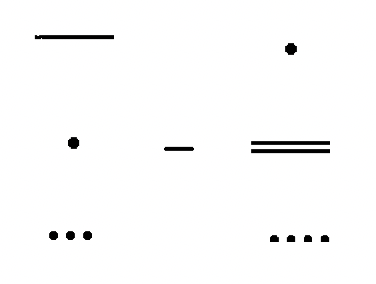
Let's use the take-away approach to subtract Mayan numerals. It's similar to addition in that you remove a subset at each level – exchanges or trades might have to be done before being able to take away at any given level. Pay close attention to which level you are on when making exchanges. A dot at one level can be traded in for a group of 20 at the next level down except from level three to level two – one dot at the third level is replaced by a group of 18 at the second level. Study the following examples. See if you can figure out which exchanges are being made. I will indicate exchanges which are being made from one level to the other with a downward arrow.

Example 1

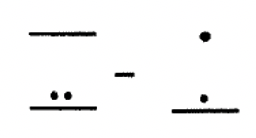
Solution

Example 2

Solution

In Examples 1 and 2, note that as exchanges are being made in the minuend (so that you can use the take-away approach), the subtrahend just keeps getting repeated. Alternately, you can first show the exchanges being made for the minuend, make a break (like a dotted line as shown below) and then write the subtraction problem out and subtract. Here is another way, you might show the steps for Example 2.

Exercise 6

Perform the following subtraction problem in Mayan and show all of the steps.

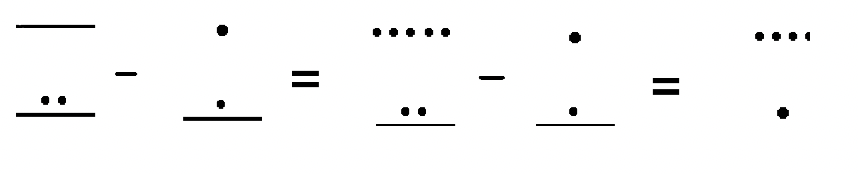
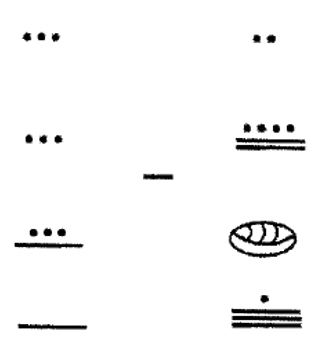
Here are some more examples.

Example 3

Solution

Example 4

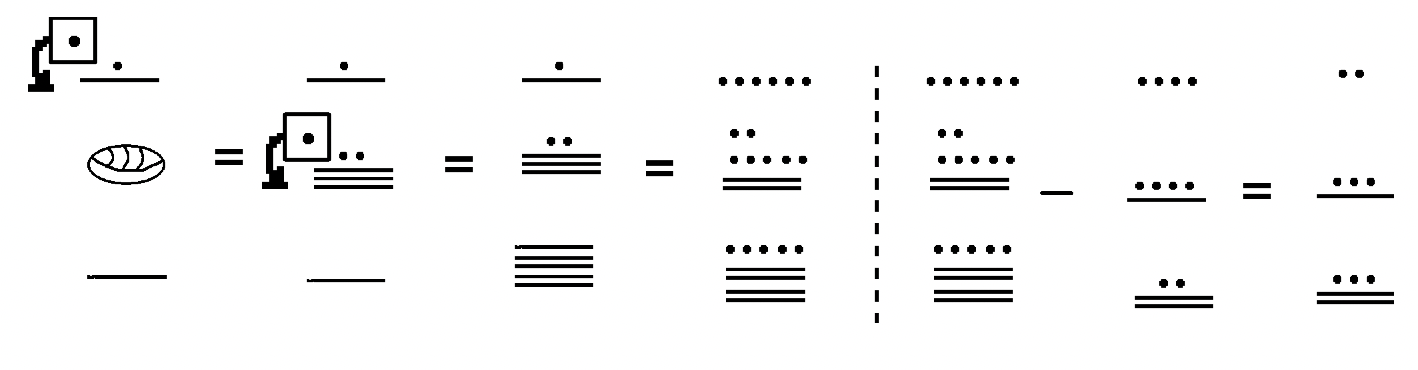
Solution

It takes a lot of concentration and effort to work these correctly. Perform each step carefully because it is easy to make mistakes. Each of the previous four examples can be checked by adding the answer to the subtrahend and seeing if the sum is the minuend. The other way to check is convert the minuend and subtrahend to Hindu-Arabic, subtract in Hindu-Arabic, then convert the difference to Mayan, making sure the answer is the same as the one you came up with when you did it in Mayan. Here is the check for Example 4: 46,084 –25,396 = 20,688. I made mistakes on this problem the first time through, but found my mistakes by checking and starting over. You should definitely check your answers. Try doing examples 3 and 4 above on your own before going on to the next exercise.

Exercise 7

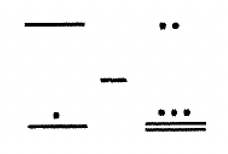
Perform the following subtraction problems in Mayan. Show all steps and check!

a.

b.

c.

d.

Take out a set of Base Four blocks. We are going to do the subtraction problem 17 – 7 in Base Four. \(101_{\text{four}}\) is the Base Four numeral for 17 and \(13_{\text{four}}\) is the Base Four numeral for 7. We will make a pile, called Pile A, using the Base Four blocks to represent 17 and another pile, called Pile B, to represent 7. Your piles should look something like what you see to the right.

Pile A

Pile B

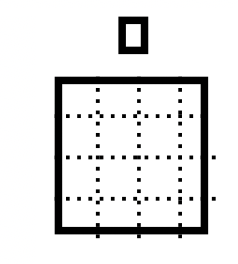Below is an illustration of the subtraction problem being performed. In order to use the take-away approach, Pile B must be a subset of Pile A. That exact subset must be removed from Pile A. First, the flat in Pile A must be exchanged for 3 longs and 4 units so you can actually see Pile B inside Pile A. There is a box around the subset (Pile B) to be removed from Pile A note how it matches Pile B. After taking those blocks away, 2 longs and 2 units remain. Therefore, the completed subtraction problem is \(101_{\text{four}} – 13_{\text{four}} = 22_{\text{four}}\).

Pile A

Pile B

Pile A - Pile B

Get out and use your base blocks to do the following exercises! Follow directions.

Exercise 8

Count out 17 unit blocks and make exchanges with base five blocks. Put them in a pile, called Pile A, and SAVE THIS PILE. Since you've made exchanges in base five, write 17 as a base five numeral on the space provided for Pile A below. Now, count out 9 more units and make exchanges with base five blocks. Put them in a pile, called Pile B. Since you've made exchanges in base five, write 9 as a base five numeral on the space provided for Pile B below. If necessary, make exchanges within Pile A so that an exact subset of Pile B is seen in Pile A. Take away those blocks in Pile A which represent the blocks in Pile B. The blocks left in Pile A are those that are left after subtracting the blocks that were in Pile B. If necessary, make any exchanges with base five blocks and then write this number as a base five numeral on the third blank provided below.

Below is the subtraction problem you have just performed in Base Five :

____________ + _____________ = _____________________

Pile A Pile B Difference of Pile A and B

Convert the difference (answer) to Base Ten. It should be 8 since 17 – 9 = 8. Is it?

Illustrate the subtraction problem using blocks below. Show the steps.

Exercise 9

Count out 17 unit blocks and make exchanges with base eight blocks. Put them in a pile, called Pile A, and SAVE THIS PILE. Since you've made exchanges in base eight , write 17 as a base eight numeral on the space provided for Pile A below. Now, count out 7 more units and make exchanges with base eight blocks. Put them in a pile, called Pile B. Since you've made exchanges in base eight , write 7 as a base eight numeral on the space provided for Pile B below. If necessary, make exchanges within Pile A so that an exact subset of Pile B is seen in Pile A. Take away those blocks in Pile A which represent the blocks in Pile B. The blocks left in Pile A are those that are left after subtracting the blocks that were in Pile B. If necessary, make any exchanges with base eight blocks and then write this number as a base eight numeral on the third blank provided below.

Below is the subtraction problem you have just performed in Base Eight:

____________ + _____________ = _____________________

Pile A Pile B Difference of Pile A and B

Convert the difference (answer) to Base Ten. It should be 10 since 17 – 7 = 10. Is it? Illustrate the subtraction problem using blocks below. Show the steps.

Exercise 10

Count out 21 unit blocks and make exchanges with base three blocks. Put them in a pile, called Pile A, and SAVE THIS PILE. Since you've made exchanges in base three , write 21 as a base three numeral on the space provided for Pile A below. Now, count out 7 more units and make exchanges with base three blocks. Put them in a pile, called Pile B. Since you've made exchanges in base three , write 7 as a base three numeral on the space provided for Pile B below. If necessary, make exchanges within Pile A so that an exact subset of Pile B is seen in Pile A. Take away those blocks in Pile A which represent the blocks in Pile B. The blocks left in Pile A are those that are left after subtracting the blocks that were in Pile B. If necessary, make any exchanges with base three blocks and then write this number as a base three numeral on the third blank provided below.

Below is the subtraction problem you have just performed in Base Three :

Pile A Pile B Difference of Pile A and B

Convert the difference (answer) to Base Ten. It should be 14 since 21 –7 = 14. Is it? Illustrate the subtraction problem using blocks below. Show the steps.

Exercise 11

Write the three subtraction problems from exercises 8, 9 and 10 in a vertical format like the one in Base Four (my example) shown to the right. Study this Base Four problem as well as the three problems you write down. Try to figure out a way to do the subtraction problems using paper and pencil instead of by using the Base Blocks. In other words, try to come up with your own algorithm (method) for doing subtraction in other bases. Explain your method and show a few examples. Later, you will be learning algorithms for subtraction.

\[ \begin{aligned} 101_{\text{ four}} \\ \underline{ - 13_{\text{ four}}} \\ 22_{\text{ four}} \end{aligned} \nonumber \]

Exercise 12

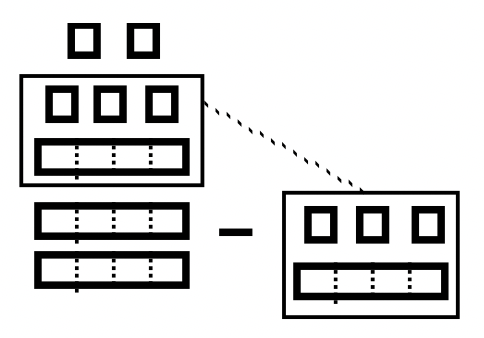
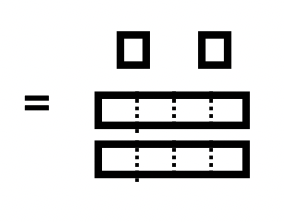
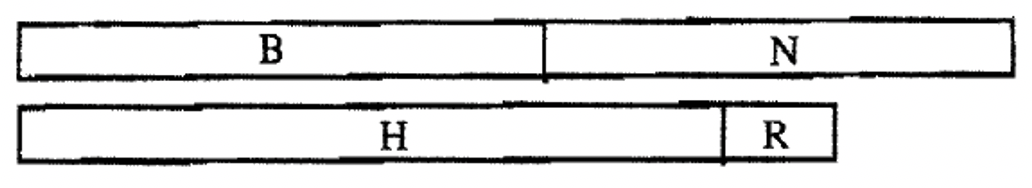
Using your C-Strips, make two trains as indicated. Let B + N be the first train, \(t_{1}\), and let H + R be the second train, \(t_{2}\). Place \(t_{1}\) adjacent to t 2 as shown below. Find a train (write it as a single C-strip) that, when added to t 2 , will form a train equal in length to \(t_{1}\). This train is called the difference of the two trains, \(t_{1}\) and \(t_{2}\) and is denoted by \(t_{1} –t_{2}\). What is \(t_{1} –t_{2}\) ?

Exercise 13

Use C-Strips to find the following differences. Draw a diagram of your work.

a. H – L	b. S – D	c. B – W
d. (B + K) – N	e. (O + Y) – P

Exercise 14

Use the C-Strips to verify and illustrate each of the following statements:

a. (S + Y) – Y = S	b. (H – P) + P = H	c. (B + R) – B = R
d. H – (L + D) = H – L –D	e. (B + Y) – (K + L) = (B – K) + (Y – L)

In Exercise 12, a new approach was used to defining difference. There are two distinct ways of defining subtraction – the take-away approach and the missing addend approach. We can use the model for how difference was defined using trains and now apply it to the definition of subtraction for whole numbers. Below is a definition of subtraction using the model for how difference was defined using trains in Exercise 12.

Definition of Subtraction (Missing Addend Approach): Let a and b be any two whole numbers. a – b is the whole number c such that a = b + c. In other words, if c is added to the subtrahend, b, the sum is the minuend, a. The answer, c, is called the missing addend.

Exercise 15

Is there a whole-number answer for every whole-number subtraction problem? In other words, is subtraction of whole numbers closed? Explain your answer and provide a counterexample if it is not closed

Exercise 16

Determine which sets, if any, are closed under subtraction. Provide a counterexample if a set is not closed.

a. {0}

b. {0, 2, 4, 6, ...}

If you think of subtraction in terms of the missing addend approach, then we say that the statements a – b = c and a = b + c are equivalent to each other. Consider the statement, 8 – 2 = 6. It is equivalent to the statement 8 = 2 + 6. We also know that 2 + 6 = 6 + 2 because of the commutative property of addition. Therefore, 8 = 6 + 2, which in turn is equivalent to the statement 8 – 6 = 2. This gives us four facts about how to relate the numbers 2, 6 and 8 using addition and subtraction

8 – 2 = 6

8 = 2 + 6

8 – 6 = 2

8 = 6 + 2

Some teachers relate subtraction and addition by using the idea of "fact families" such as the four facts above, which is one fact family.

It is important to note that each addition statement gives us two subtraction statements, which is how many people learn their subtraction facts. Because of this relationship between addition and subtraction, once a child learns basic addition facts, the subtraction facts naturally follow.

Exercise 17

Write down two subtraction statements that are equivalent to each addition statement.

You may wonder why I wrote the addition statements in Exercise 14 with the plus sign on the left of the equals sign, unlike the way I happened to write the two addition facts in the fact family relating 2, 6 and 8 at the top of the page. It's because 8 + 4 = 12 is the same fact as 12 = 8 + 4 due to the Symmetric Property of Equality which is defined below.

The Symmetric Property of Equality states that for any equation, if a = b, then it is also true that b = a

Therefore, it is perfectly okay to write the four facts above as

2 + 6 = 8

6 + 2 = 8

8 – 2 = 6

8 – 6 = 2

Use the symmetric property of equality to rewrite each equation.

Exercise 18

a. 7 – 3 = 4 : _____

b. 9 – 1 = 8 : _____

Exercise 19

Is there a symmetric property of inequality? Explain.

Note: Inequality refers to less than ( < ) and/or greater than ( > ).

Let's do a subtraction problem using the missing addend approach in Egyptian. The set-up is exactly the same as when employing the take-away approach, but the way in which you go about finding the answer is different. The first example we looked at in Egyptian was

We ask ourselves "What must be added to

to get

?" Another way to express this is to write:

+ ????? =

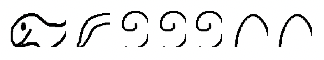
. We begin by converting in the minuend until it contains the subtrahend. We did these exact steps when using the take-away approach. But to get the final answer, we note what must be added to the subtrahend to get the minuend. The answer is

. The illustration is shown below.

To check, see if the missing addend (the answer) + the subtrahend = the minuend. In other words, is the following addition statement true?

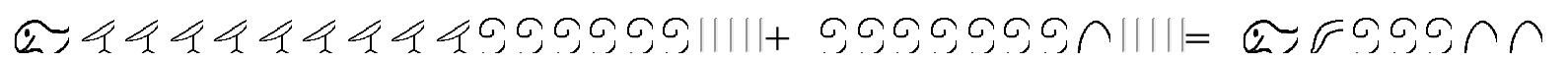
Another way to check is to convert to Hindu-Arabic.

The same approach could be used to subtract in Mayan. The steps used to get ready to find the missing addend are exactly the same as when employing the take-away approach. But to compute the answer, at each level you decide which symbols must be added to the subtrahend to get what is in the minuend.

Although it may be hard to distinguish between the two approaches to subtraction, it's the way that you think about the problem that makes it different. For instance, one child learning about subtraction might think about the problem 8 – 3 by thinking "If I have 8 pennies in one hand and take away 3 of them to put in my pocket, how many are left in my hand?" This is the take-away approach. The child might compute the answer by counting backwards –7, 6, 5 or by actually using pennies or manipulatives where 8 pennies are put in one hand, 3 are taken away and what's left is counted to get the answer. Another child might think of the same subtraction problem in this way –"If I have 3 pennies, how many more pennies do I need so that I'll have 8 pennies?" This is the missing addend approach.

Exercise 20

Make up two word problems that would require the subtraction problem 8 – 3 to be computed. The first should use the take-away approach and the second should use the missing addend approach. Explain and show how you would solve each word problem using the given approach.

a. Take-Away Approach

b. Missing Addend Approach

Without using manipulatives, each subtraction problem can be worded as a missing addend addition problem. In other words, to find the answer to 8 – 3, you figure out what goes in the blank for 3 + ____ = 8. Since 3 + 5 = 8, then 8 – 3 = 5 .

Exercise 21

Perform the following subtraction problems by employing the missing addend approach. Fill in the blanks

a. 9 – 7 = ____ because _____________

b. 13 – 6 = ____ because _____________

c. 88 – 8 = ____ because ______________

d. 70 – 14 = ____ because _____________

Use Base Blocks to compute the following subtraction problems using the missing addend approach. Begin by forming two piles, one for the minuend (Pile A) and one for the subtrahend (Pile B). Then figure out what must go in a third pile (Pile X) so that B + X = A. Show how you found the answer.

Exercise 22

\(231_{\text{five}} – 140_{\text{five}}\)

Exercise 23

\(100_{\text{two}} – 11_{\text{two}}\)

Exercise 24

\(135_{\text{twelve}} – T6_{\text{twelve}}\)

The missing addend approach is sometimes called the Additive (or Austrian) Algorithm.

This approach is very useful for doing subtraction on the number line.

Let's review the definition of subtraction using the missing addend approach. It says the answer to a – b = c where c is the number that must be added to b to get c, or b + c = a.

Think about this using a number line. How would we find the answer to 7 – 3? We need to find a number to add on to 3 that gives us an answer of 7. In other words, what would go in the blank 3 + ____ = 7? I know you know the answer, but how could we get the answer by using a number line?

First, we have to define a new term: vector. A vector is a directed line segment. Basically, a directed vector looks like an arrow. It has a certain length and points in a certain direction. Since we'll be using horizontal number lines, we'll be using horizontal vectors. An arrow pointing to the right will denote a positive number and an arrow pointing to the left will denote a negative number.

Below is a number line, with some vectors shown above. Vector a is 6 units long and the arrow points to the right. Therefore, it represents the number +6. Vector b is also 6 units long, but it points to the left. Therefore, it represents the number -6.

Exercise 25

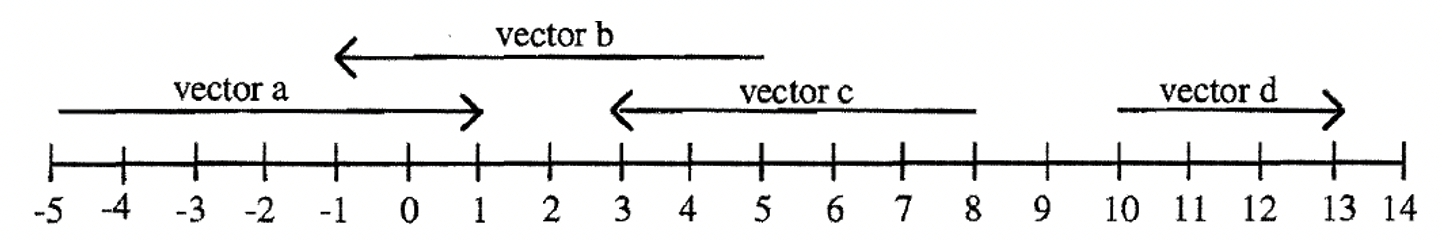
a. What number does vector c represent? _________

b. What number does vector d represent? _________

Now, we're ready to use vectors in conjunction with the missing addend approach to compute a subtraction problem. Let's go back to the problem 7 – 3. The missing addend approach states the answer to the subtraction problem is the number that when added to the number after the subtraction sign gives the number that is before the subtraction sign. Since 3 is the number after the subtraction sign, we have to find a number to add to 3 that will give an answer of 7. Using a number line, this means if you start at 3, how can you get to 7? In other words, draw a vector that starts at 3 and ends up at 7. Make sure the arrow points to 7. The number that the vector represents is the answer to the problem. The illustration is shown below. The vector has length 4 and points to the right, so the answer is 4, which is written above the vector to indicate the answer.

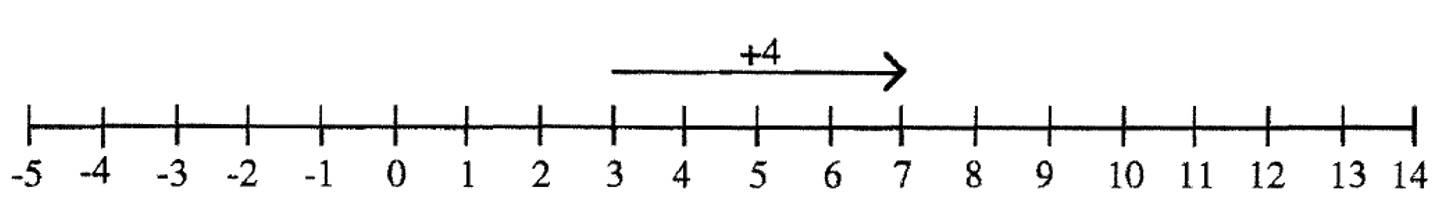
Let's try the problem 2 - 9. Then, 9 + ____ = 2. On the number line, draw a vector that starts at 9 and ends at 2. Then, see what number the vector represents to find the answer. The vector shown below gives the answer of -7.

Exercise 26

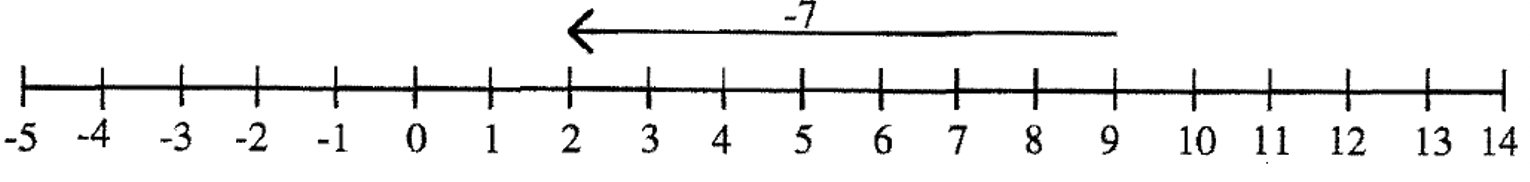
Find the answer to the following subtraction problems by using directed vectors on the number line in conjunction with the missing addend approach. Draw the vectors.

a. 10 - 4 =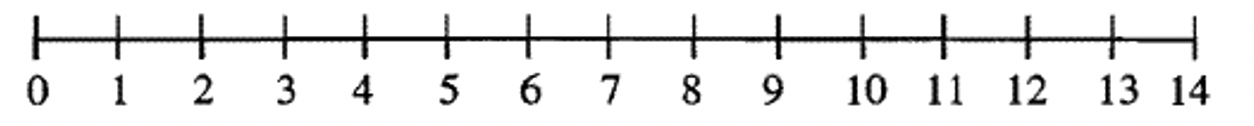

b. 12 - 5 =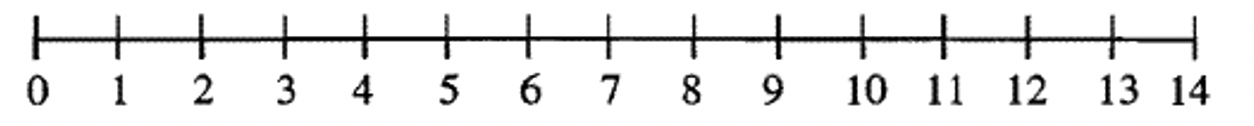

c. 8 - 3 =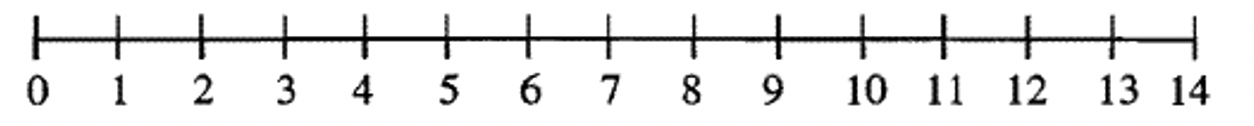

d. 9 - 7 =

e. 3 - 10 =

f. 5 - 8 =

g. 1 - 13 =

h. 9 - 9 =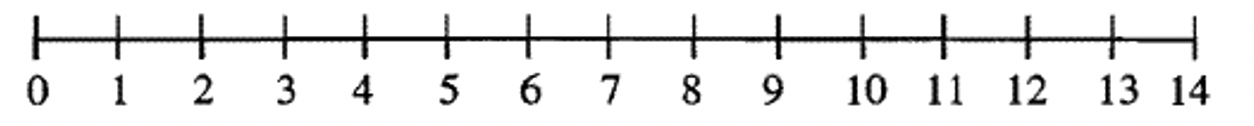

Exercise 27

Looking at the vectors a – d below, figure out what subtraction problem was being performed, and then state the answer. For instance, vector b came from doing the problem -1 – 5. So, -1 – 5 = -6 (since vector b is 6 units long and goes left).

Vector a: ____

Vector b: ____

Vector c: ____