31.2: Truth and Equations
- Page ID
- 40605
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Lesson
Let's use equations to represent stories and see what it means to solve equations.
Exercise \(\PageIndex{1}\): Three Letters
- The equation \(a+b=c\) could be true or false.
- If \(a\) is 3, \(b\) is 4, and \(c\) is 5, is the equation true or false?
- Find new values of \(a, b,\) and \(c\) that make the equation true.
- Find new values of \(a, b,\) and \(c\) that make the equation false.
- The equation \(x\cdot y=z\) could be true or false.
- If \(x\) is 3, \(y\) is 4, and \(z\) is 12, is the equation true or false?
- Find new values of \(x, y,\) and \(z\) that make the equation true.
- Find new values of \(x, y,\) and \(z\) that make the equation false.
Exercise \(\PageIndex{2}\): Storytime
Here are three situations and six equations. Which equation best represents each situation? If you get stuck, consider drawing a diagram.
\(\begin{array}{lllll}{x+5=20}&{\qquad}&{x=20+5}&{\qquad}&{5x=20}\\{x+20=5}&{\qquad}&{5\cdot 20=x}&{\qquad}&{20x=5}\end{array}\)
- After Elena ran 5 miles on Friday, she had run a total of 20 miles for the week. She ran \(x\) miles before Friday.
- Andre’s school has 20 clubs, which is five times as many as his cousin’s school. His cousin’s school has \(x\) clubs.
- Jada volunteers at the animal shelter. She divided 5 cups of cat food equally to feed 20 cats. Each cat received \(x\) cups of food.
Exercise \(\PageIndex{3}\): Using Structures to Find Solutions
Here are some equations that contain a variable and a list of values. Think about what each equation means and find a solution in the list of values. If you get stuck, consider drawing a diagram. Be prepared to explain why your solution is correct.
- \(1000-a=400\)
- \(12.6=b+4.1\)
- \(8c=8\)
- \(\frac{2}{3}\cdot d=\frac{10}{9}\)
- \(10e=1\)
- \(10=0.5f\)
- \(0.99=1-g\)
- \(h+\frac{3}{7}=1\)
List:
\(\begin{array}{ccccccccc}{\frac{1}{8}}&{\frac{3}{7}}&{\frac{4}{7}}&{\frac{3}{5}}&{\frac{5}{3}}&{\frac{7}{3}}&{0.01}&{0.1}&{0.5}\\{1}&{2}&{8.5}&{9.5}&{16.7}&{20}&{400}&{600}&{1400}\end{array}\)
Are you ready for more?
One solution to the equation \(a+b+c=10\) is \(a=2, b=5, c=3\).
How many different whole-number solutions are there to the equation \(a+b+c=10\)? Explain or show your reasoning.
Summary
An equation can be true or false. An example of a true equation is \(7+1=4\cdot 2\). An example of a false equation is \(7+1=9\).
An equation can have a letter in it, for example, \(u+1=8\). This equation is false if \(u\) is 3, because \(3+1\) does not equal 8. This equation is true if \(u\) is 7, because \(7+1=8\).
A letter in an equation is called a variable. In \(u+1=8\), the variable is \(u\). A number that can be used in place of the variable that makes the equation true is called a solution to the equation. In \(u+1=8\), the solution is 7.
When a number is written next to a variable, the number and the variable are being multiplied. For example, \(7x=21\) means the same thing as \(7\cdot x=21\). A number written next to a variable is called a coefficient. If no coefficient is written, the coefficient is 1. For example, in the equation \(p+3=5\), the coefficient of \(p\) is 1.
Glossary Entries
Definition: Coefficient
A coefficient is a number that is multiplied by a variable.
For example, in the expression \(3x+5\), the coefficient of \(x\) is \(3\). In the expression \(y+5\), the coefficient of \(y\) is \(1\), because \(y=1\cdot y\).
Definition: Solution to an Equation
A solution to an equation is a number that can be used in place of the variable to make the equation true.
For example, 7 is the solution to the equation \(m+1=8\), because it is true that \(7+1=8\). The solution to \(m+1=8\) is not \(9\), because \(9+1\neq 8\).
Definition: Variable
A variable is a letter that represents a number. You can choose different numbers for the value of the variable.
For example, in the expression \(10-x\), the variable is \(x\). If the value of \(x\) is 3, then \(10-x=7\), because \(10-3=7\). If the value of \(x\) is \(6\), then \(10-x=4\), because \(10-6=4\).
Practice
Exercise \(\PageIndex{4}\)
Select all the true equations.
- \(5+0=0\)
- \(15\cdot 0=0\)
- \(1.4+2.7=4.1\)
- \(\frac{2}{3}\cdot\frac{5}{9}=\frac{7}{12}\)
- \(4\frac{2}{3}=5-\frac{1}{3}\)
Exercise \(\PageIndex{5}\)
Mai's water bottle had 24 ounces in it. After she drank \(x\) ounces of water, there were 10 ounces left. Select all the equations that represent this situation.
- \(24\div 10=x\)
- \(24+10=x\)
- \(24-10=x\)
- \(x+10=24\)
- \(10x=24\)
Exercise \(\PageIndex{6}\)
Priya has 5 pencils, each \(x\) inches in length. When she lines up the pencils end to end, they measure 34.5 inches. Select all the equations that represent this situation.
- \(5+x=34.5\)
- \(5x=34.5\)
- \(34.5\div 5=x\)
- \(34.5-5=x\)
- \(x=(34.5)\cdot 5\)
Exercise \(\PageIndex{7}\)
Match each equation with a solution from the list of values.
- \(2a=4.6\)
- \(b+2=4.6\)
- \(c\div 2=4.6\)
- \(d-2=4.6\)
- \(e+\frac{3}{8}=2\)
- \(\frac{1}{8}f=3\)
- \(g\div\frac{8}{5}=1\)
- \(\frac{8}{5}\)
- \(1\frac{5}{8}\)
- \(2.3\)
- \(2.6\)
- \(6.6\)
- \(9.2\)
- \(24\)
Exercise \(\PageIndex{8}\)
The daily recommended allowance of vitamin C for a sixth grader is 45 mg. 1 orange has about 75% of the recommended daily allowance of vitamin C. How many milligrams are in 1 orange? If you get stuck, consider using the double number line.
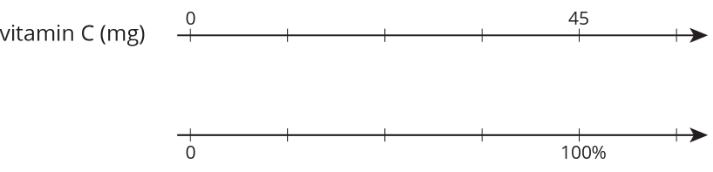
(From Unit 3.4.2)
Exercise \(\PageIndex{9}\)
There are 90 kids in the band. 20% of the kids own their own instruments, and the rest rent them.
- How many kids own their own instruments?
- How many kids rent instruments?
- What percentage of kids rent their instruments?
(From Unit 3.4.3)
Exercise \(\PageIndex{10}\)
Find each product.
- \((0.25)\cdot (1.4)\)
- \((0.061)\cdot (0.43)\)
- \((1.017)\cdot (0.072)\)
- \((5.226)\cdot (0.037)\)
(From Unit 5.3.4)