6.E: Applications of Integration (Exercises)

Last updated
Save as PDF

Page ID: 3438

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

These are homework exercises to accompany OpenStax's "Calculus" Textmap.

6.1: Areas between Curves

For exercises 1 - 2, determine the area of the region between the two curves in the given figure by integrating over the $x$-axis.

1) $y=x^2−3$ and $y=1$

$This figure is has two graphs. They are the functions f(x) = x^2-3and g(x)=1. In between these graphs is a shaded region, bounded above by g(x) and below by f(x). The shaded area is between x=-2 and x=2.$

Answer:: $\dfrac{32}{3} \, \text{units}^2$

2) $y=x^2$ and $y=3x+4$

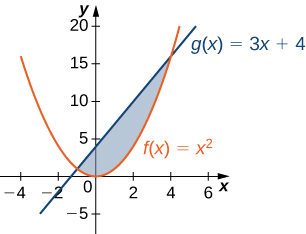
$This figure is has two graphs. They are the functions f(x) = x^2 and g(x)= 3x+4. In between these graphs is a shaded region, bounded above by g(x) and below by g(x).$

For exercises 3 - 4, split the region between the two curves into two smaller regions, then determine the area by integrating over the $x$-axis. Note that you will have two integrals to solve.

3) $y=x^3$ and $ y=x^2+x$

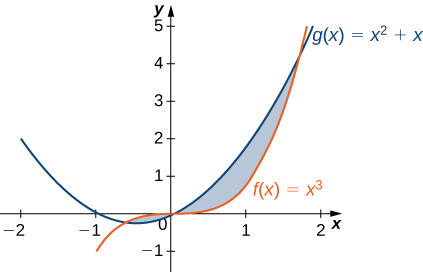
$This figure is has two graphs. They are the functions f(x) = x^3 and g(x)= x^2+x. These graphs intersect twice. The regions between the intersections are shaded. The first region is bounded above by f(x) and below by g(x). The second region is bounded above by g(x) and below by f(x).$

Answer:: $\dfrac{13}{12}\, \text{units}^2$

4) $y=\cos θ$ and $ y=0.5$, for $ 0≤θ≤π$

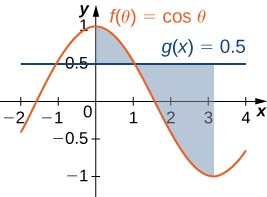
$This figure is has two graphs. They are the functions f(theta) = cos(theta) and g(x)= 0.5. These graphs intersect twice. The regions between the intersections are shaded. The first region is bounded above by f(x) and below by g(x). The second region is bounded above by g(x) and below by f(x).$

For exercises 5-6, determine the area of the region between the two curves by integrating over the $y$-axis.

5) $x=y^2$ and $x=9$

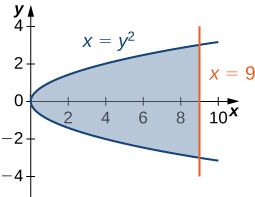
$This figure is has two graphs. They are the equations x=y^2 and x=9. The region between the graphs is shaded. It is horizontal, between the y-axis and the line x=9.$

Answer:: $36 \, \text{units}^2$

6) $y=x$ and $ x=y^2$

$This figure is has two graphs. They are the equations y=x and x=y^2. The region between the graphs is shaded, bounded above by x=y^2 and below by y=x.$

For exercises 7 - 13, graph the equations and shade the area of the region between the curves. Determine its area by integrating over the $x$-axis.

7) $y=x^2$ and $y=−x^2+18x$

Answer:

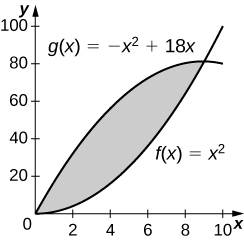
$This figure is has two graphs. They are the functions f(x)=x^2 and g(x)=-x^2+18x. The region between the graphs is shaded, bounded above by g(x) and below by f(x). It is in the first quadrant.$

243 square units

8) $y=\dfrac{1}{x}, \quad y=\dfrac{1}{x^2}$, and $x=3$

9) $y=\cos x$ and $y=\cos^2x$ on $x \in [−π,π]$

Answer:

$This figure is has two graphs. They are the functions y=cos(x) and y=cos^2(x). The graphs are periodic and resemble waves. There are four regions created by intersections of the curves. The areas are shaded.$

4 square units

10) $y=e^x,\quad y=e^{2x−1}$, and $x=0$

11) $y=e^x, \quad y=e^{−x}, \quad x=−1$ and $x=1$

Answer:

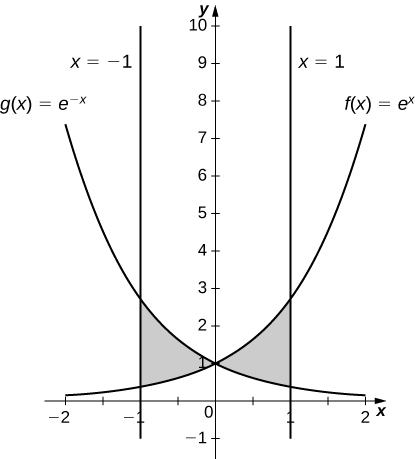
$This figure is has two graphs. They are the functions f(x)=e^x and g(x)=e^-x. There are two shaded regions. In the second quadrant the region is bounded by x=-1, g(x) above and f(x) below. The second region is in the first quadrant and is bounded by f(x) above, g(x) below, and x=1.$

$\dfrac{2(e−1)^2}{e}\, \text{units}^2$

12) $ y=e, \quad y=e^x,$ and $y=e^{−x}$

13) $y=|x|$ and $y=x^2$

Answer:

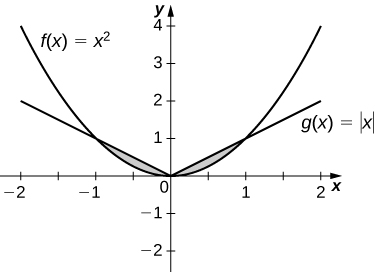
$This figure is has two graphs. They are the functions f(x)=x^2 and g(x)=absolute value of x. There are two shaded regions. The first region is in the second quadrant and is between g(x) above and f(x) below. The second region is in the first quadrant and is bounded above by g(x) and below by f(x).$

$\dfrac{1}{3}\, \text{units}^2$

For exercises 14 - 19, graph the equations and shade the area of the region between the curves. If necessary, break the region into sub-regions to determine its entire area.

14) $y=\sin(πx),\quad y=2x,$ and $x>0$

15) $y=12−x,\quad y=\sqrt{x},$ and $y=1$

Answer:

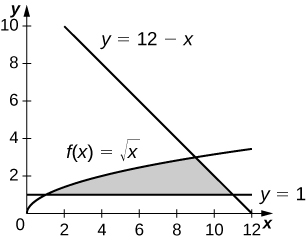
$This figure is has three graphs. They are the functions f(x)=squareroot of x, y=12-x, and y=1. The region between the graphs is shaded, bounded above and to the left by f(x), above and to the right by the line y=12-x, and below by the line y=1. It is in the first quadrant.$

$\dfrac{34}{3}\, \text{units}^2$

16) $y=\sin x$ and $y=\cos x$ over $x \in [−π,π]$

17) $y=x^3$ and $y=x^2−2x$ over $x \in [−1,1]$

Answer:

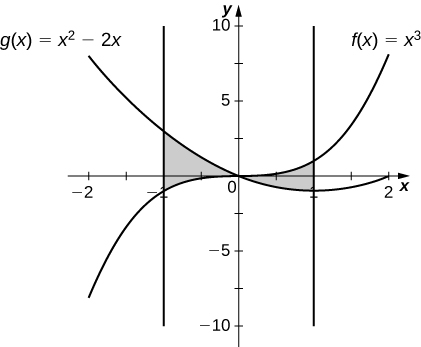
$This figure is has two graphs. They are the functions f(x)=x^3 and g(x)=x^2-2x. There are two shaded regions between the graphs. The first region is bounded to the left by the line x=-2, above by g(x) and below by f(x). The second region is bounded above by f(x), below by g(x) and to the right by the line x=2.$

$\dfrac{5}{2}\, \text{units}^2$

18) $y=x^2+9$ and $ y=10+2x$ over $x \in [−1,3]$

19) $y=x^3+3x$ and $y=4x$

Answer:

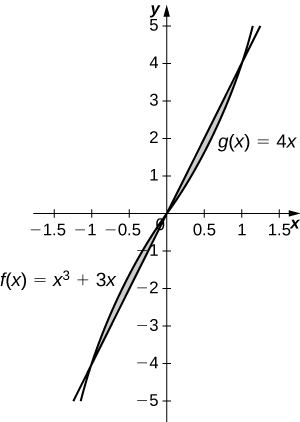
$This figure is has two graphs. They are the functions f(x)=x^3+3x and g(x)=4x. There are two shaded regions between the graphs. The first region is bounded above by f(x) and below by g(x). The second region is bounded above by g(x), below by f(x).$

$\dfrac{1}{2}\, \text{units}^2$

For exercises 20 -25, graph the equations and shade the area of the region between the curves. Determine its area by integrating over the $y$-axis.

20) $x=y^3$ and $ x = 3y−2$

21) $x=y$ and $ x=y^3−y$

Answer:

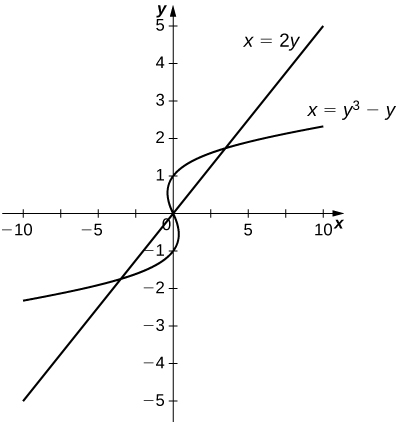
$This figure is has two graphs. They are the equations x=2y and x=y^3-y. The graphs intersect in the third quadrant and again in the first quadrant forming two closed regions in between them.$

$\dfrac{9}{2}\, \text{units}^2$

22) $x=−3+y^2$ and $ x=y−y^2$

23) $y^2=x$ and $x=y+2$

Answer:

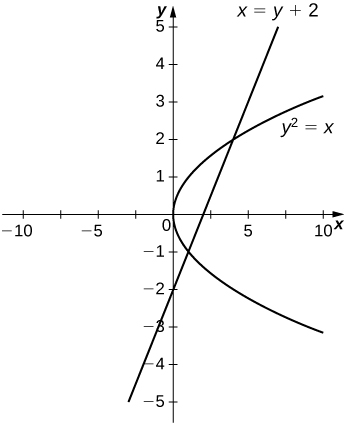
$This figure is has two graphs. They are the equations x=y+2 and y^2=x. The graphs intersect, forming a region in between them$

$\dfrac{9}{2}\, \text{units}^2$

24) $x=|y|$ and $2x=−y^2+2$

25) $x=\sin y,\quad x=\cos(2y),\quad y=π/2$, and $ y=−π/2$

Answer:

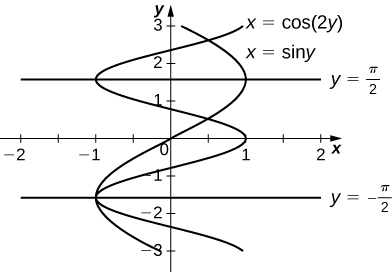
$This figure is has two graphs. They are the equations x=cos(y) and x=sin(y). The graphs intersect, forming two regions bounded above by the line y=pi/2 and below by the line y=-pi/2.$

$\dfrac{3\sqrt{3}}{2}\, \text{units}^2$

For exercises 26 - 37, graph the equations and shade the area of the region between the curves. Determine its area by integrating over the $x$-axis or $y$-axis, whichever seems more convenient.

26) $x=y^4$ and $x=y^5$

27) $y=xe^x,\quad y=e^x,\quad x=0$, and $x=1$.

Answer:

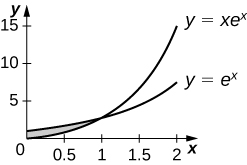
$This figure is has two graphs. They are the equations y=xe^x and y=e^x. The graphs intersect, forming a region in between them in the first quadrant.$

$e^{−2}\, \text{units}^2$

28) $y=x^6$ and $y=x^4$

29) $x=y^3+2y^2+1$ and $x=−y^2+1$

Answer:

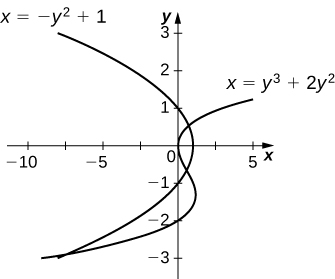
$This figure is has two graphs. They are the equations x=-y^2+1 and x=y^3+2y^2. The graphs intersect, forming two regions in between them.$

$\dfrac{27}{4}\, \text{units}^2$

30) $ y=|x|$ and $ y=x^2−1$

31) $y=4−3x$ and $y=\dfrac{1}{x}$

Answer:

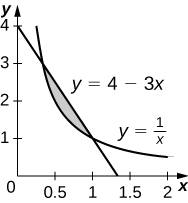
$This figure is has two graphs. They are the equations y=4-3x and y=1/x. The graphs intersect, having region between them shaded. The region is in the first quadrant.$

$\left(\dfrac{4}{3}−\ln(3)\right)\, \text{units}^2$

32) $y=\sin x,\quad x=−π/6,\quad x=π/6,$ and $y=\cos^3 x$

33) $y=x^2−3x+2$ and $ y=x^3−2x^2−x+2$

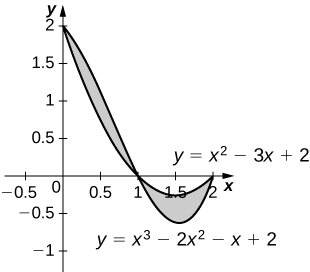
Answer:: $This figure is has two graphs. They are the equations y=x^2-3x+2 and y=x^3-2x^2-x+2. The graphs intersect, having region between them shaded.$
$\dfrac{1}{2}$ square units

34) $y=2\cos^3(3x),\quad y=−1,\quad x=\dfrac{π}{4},$ and $ x=−\dfrac{π}{4}$

35) $y+y^3=x$ and $2y=x$

Answer:

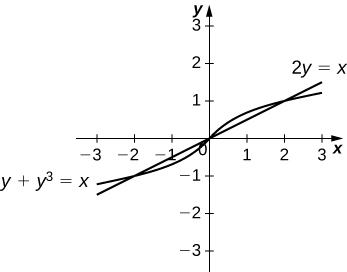
$This figure is has two graphs. They are the equations 2y=x and y+y^3=x. The graphs intersect, forming two regions. The regions are shaded.$

$\dfrac{1}{2}$ square units

36) $ y=\sqrt{1−x^2}$ and $y=x^2−1$

37) $y=\cos^{−1}x,\quad y=\sin^{−1}x,\quad x=−1,$ and $ x=1$

Answer:

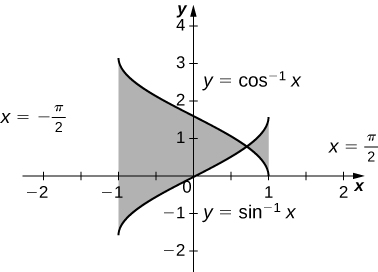
$This figure is has two graphs. They are the equations y=arccos(x) and y=arcsin (x). The graphs intersect, forming two regions. The first region is bounded to the left by x=-1. The second region is bounded to the right by x=1. Both regions are shaded.$

$−2(\sqrt{2}−π)$ square units

For exercises 38 - 47, find the exact area of the region bounded by the given equations if possible. If you are unable to determine the intersection points analytically, use a calculator to approximate the intersection points with three decimal places and determine the approximate area of the region.

38) [T] $x=e^y$ and $y=x−2$

39) [T] $y=x^2$ and $y=\sqrt{1−x^2}$

Answer:: $1.067$ square units

40) [T] $y=3x^2+8x+9$ and $3y=x+24$

41) [T] $x=\sqrt{4−y^2}$ and $ y^2=1+x^2$

Answer:: $0.852$ square units

42) [T] $x^2=y^3$ and $x=3y$

43) [T] $y=\sin^3x+2,\quad y=\tan x,\quad x=−1.5,$ and $x=1.5$

Answer:: $7.523$ square units

44) [T] $y=\sqrt{1−x^2}$ and $y^2=x^2$

45) [T] $y=\sqrt{1−x^2}$ and $y=x^2+2x+1$

Answer:: $\dfrac{3π−4}{12}$ square units

46) [T] $x=4−y^2$ and $ x=1+3y+y^2$

47) [T] $y=\cos x,\quad y=e^x,\quad x=−π,\quad$ and$\quad x=0$

Answer:: $1.429$ square units

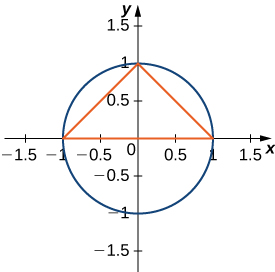
48) The largest triangle with a base on the $x$-axis that fits inside the upper half of the unit circle $y^2+x^2=1$ is given by $ y=1+x$ and $ y=1−x$. See the following figure. What is the area inside the semicircle but outside the triangle?

$This figure is has the graph of a circle with center at the origin and radius of 1. There is a triangle inscribed with base on the x-axis from -1 to 1 and the third corner at the point y=1.$

49) A factory selling cell phones has a marginal cost function $C(x)=0.01x^2−3x+229$, where $x$ represents the number of cell phones, and a marginal revenue function given by $R(x)=429−2x.$ Find the area between the graphs of these curves and $x=0.$ What does this area represent?

Answer:: $33,333.33 total profit for 200 cell phones sold

50) An amusement park has a marginal cost function $C(x)=1000e−x+5$, where $x$ represents the number of tickets sold, and a marginal revenue function given by $R(x)=60−0.1x$. Find the total profit generated when selling $550$ tickets. Use a calculator to determine intersection points, if necessary, to two decimal places.

51) The tortoise versus the hare: The speed of the hare is given by the sinusoidal function $H(t)=1−\cos((πt)/2)$ whereas the speed of the tortoise is $T(t)=(1/2)\tan^{−1}(t/4)$, where $t$ is time measured in hours and the speed is measured in miles per hour. Find the area between the curves from time $t=0$ to the first time after one hour when the tortoise and hare are traveling at the same speed. What does it represent? Use a calculator to determine the intersection points, if necessary, accurate to three decimal places.

Answer:: $3.263$ mi represents how far ahead the hare is from the tortoise

52) The tortoise versus the hare: The speed of the hare is given by the sinusoidal function $H(t)=(1/2)−(1/2)\cos(2πt)$ whereas the speed of the tortoise is $T(t)=\sqrt{t}$, where $t$ is time measured in hours and speed is measured in kilometers per hour. If the race is over in 1 hour, who won the race and by how much? Use a calculator to determine the intersection points, if necessary, accurate to three decimal places.

For exercises 53 - 55, find the area between the curves by integrating with respect to $x$ and then with respect to $y$. Is one method easier than the other? Do you obtain the same answer?

53) $y=x^2+2x+1$ and $y=−x^2−3x+4$

Answer:: $\dfrac{343}{24}$ square units

54) $y=x^4$ and $x=y^5$

55) $x=y^2−2$ and $x=2y$

Answer:: $4\sqrt{3}$ square units

For exercises 56 - 57, solve using calculus, then check your answer with geometry.

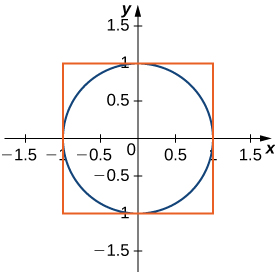
56) Determine the equations for the sides of the square that touches the unit circle on all four sides, as seen in the following figure. Find the area between the perimeter of this square and the unit circle. Is there another way to solve this without using calculus?

$This figure is the graph of a circle centered at the origin with radius of 1. There is a circumscribed square around the circle.$

57) Find the area between the perimeter of the unit circle and the triangle created from $y=2x+1,\,y=1−2x$ and $y=−\dfrac{3}{5}$, as seen in the following figure. Is there a way to solve this without using calculus?

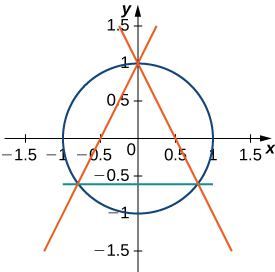
$This figure is the graph of a circle centered at the origin with radius of 1. There are three lines intersecting the circle. The lines intersect the circle at three points to form a triangle within the circle.$

Answer:: $ \left(π−\dfrac{32}{25}\right)$ square units

6.2: Determining Volumes by Slicing

1) Derive the formula for the volume of a sphere using the slicing method.

2) Use the slicing method to derive the formula for the volume of a cone.

3) Use the slicing method to derive the formula for the volume of a tetrahedron with side length $a.$

4) Use the disk method to derive the formula for the volume of a trapezoidal cylinder.

5) Explain when you would use the disk method versus the washer method. When are they interchangeable?

Volumes by Slicing

For exercises 6 - 10, draw a typical slice and find the volume using the slicing method for the given volume.

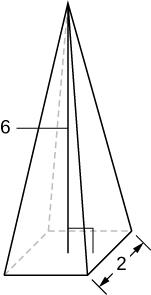
6) A pyramid with height 6 units and square base of side 2 units, as pictured here.

$This figure is a pyramid with base width of 2 and height of 6 units.$

Solution:: Here the cross-sections are squares taken perpendicular to the $y$-axis.
We use the vertical cross-section of the pyramid through its center to obtain an equation relating $x$ and $y$.
Here this would be the equation, $ y = 6 - 6x $. Since we need the dimensions of the square at each $y$-level, we solve this equation for $x$ to get, $x = 1 - \tfrac{y}{6}$.
This is half the distance across the square cross-section at the $y$-level, so the side length of the square cross-section is, $s = 2\left(1 - \tfrac{y}{6}\right).$
Thus, we have the area of a cross-section is,

$A(y) = \left[2\left(1 - \tfrac{y}{6}\right)\right]^2 = 4\left(1 - \tfrac{y}{6}\right)^2.$

$\begin{align*} \text{Then},\quad V &= \int_0^6 4\left(1 - \tfrac{y}{6}\right)^2 \, dy \\[5pt]
&= -24 \int_1^0 u^2 \, du, \quad \text{where} \, u = 1 - \tfrac{y}{6}, \, \text{so} \, du = -\tfrac{1}{6}\,dy, \quad \implies \quad -6\,du = dy \\[5pt]
&= 24 \int_0^1 u^2 \, du = 24\dfrac{u^3}{3}\bigg|_0^1 \\[5pt]
&= 8u^3\bigg|_0^1 \\[5pt]
&= 8\left( 1^3 - 0^3 \right) \quad= \quad 8\, \text{units}^3 \end{align*}$

7) A pyramid with height 4 units and a rectangular base with length 2 units and width 3 units, as pictured here.

$This figure is a pyramid with base width of 2, length of 3, and height of 4 units.$

8) A tetrahedron with a base side of 4 units,as seen here.

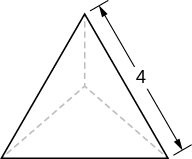
$This figure is an equilateral triangle with side length of 4 units.$

Answer:: $V = \frac{32}{3\sqrt{2}} = \frac{16\sqrt{2}}{3}$ units³

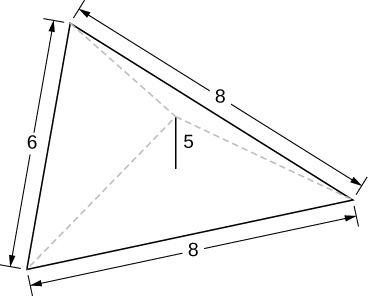
9) A pyramid with height 5 units, and an isosceles triangular base with lengths of 6 units and 8 units, as seen here.

$This figure is a pyramid with a triangular base. The view is of the base. The sides of the triangle measure 6 units, 8 units, and 8 units. The height of the pyramid is 5 units.$

10) A cone of radius $ r$ and height $ h$ has a smaller cone of radius $ r/2$ and height $ h/2$ removed from the top, as seen here. The resulting solid is called a frustum.

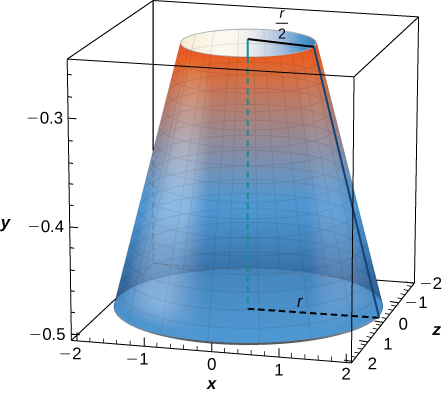
$This figure is a 3-dimensional graph of an upside down cone. The cone is inside of a rectangular prism that represents the xyz coordinate system. the radius of the bottom of the cone is “r” and the radius of the top of the cone is labeled “r/2”.$

Answer:: $V = \frac{7\pi}{12} hr^2$ units³

For exercises 11 - 16, draw an outline of the solid and find the volume using the slicing method.

11) The base is a circle of radius $ a$. The slices perpendicular to the base are squares.

12) The base is a triangle with vertices $ (0,0),(1,0),$ and $ (0,1)$. Slices perpendicular to the $xy$-plane are semicircles.

Answer:

$This figure shows the x-axis and the y-axis with a line starting on the x-axis at (1,0) and ending on the y-axis at (0,1). Perpendicular to the xy-plane are 4 shaded semi-circles with their diameters beginning on the x-axis and ending on the line, decreasing in size away from the origin.$

$\displaystyle V = \int_0^1 \frac{\pi(1-x)^2}{8}\, dx \quad = \quad \frac{π}{24}$ units³

13) The base is the region under the parabola $ y=1−x^2$ in the first quadrant. Slices perpendicular to the $xy$-plane are squares.

14) The base is the region under the parabola $ y=1−x^2$ and above the $x$-axis. Slices perpendicular to the $y$-axis are squares.

Answer:

$This figure shows the x-axis and the y-axis in 3-dimensional perspective. On the graph above the x-axis is a parabola, which has its vertex at y=1 and x-intercepts at (-1,0) and (1,0). There are 3 square shaded regions perpendicular to the x y plane, which touch the parabola on either side, decreasing in size away from the origin.$

$\displaystyle V = \int_0^1 4(1 - y)\,dy \quad = \quad 2$ units³

15) The base is the region enclosed by $ y=x^2)$ and $ y=9.$ Slices perpendicular to the $x$-axis are right isosceles triangles.

16) The base is the area between $ y=x$ and $ y=x^2$. Slices perpendicular to the $x$-axis are semicircles.

Answer:

$This figure is a graph with the x and y axes diagonal to show 3-dimensional perspective. On the first quadrant of the graph are the curves y=x, a line, and y=x^2, a parabola. They intersect at the origin and at (1,1). Several semicircular-shaped shaded regions are perpendicular to the x y plane, which go from the parabola to the line and perpendicular to the line.$

$\displaystyle V = \int_0^1 \frac{\pi}{8}\left( x - x^2 \right)^2 \, dx \quad=\quad \frac{π}{240}$ units³

Disk and Washer Method

For exercises 17 - 24, draw the region bounded by the curves. Then, use the disk or washer method to find the volume when the region is rotated around the $x$-axis.

17) $ x+y=8,\quad x=0$, and $ y=0$

18) $ y=2x^2,\quad x=0,\quad x=4,$ and $ y=0$

Answer:

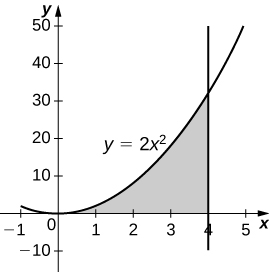
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the curve y=2x^2, below by the x-axis, and to the right by the vertical line x=4.$

$\displaystyle V = \int_0^4 4\pi x^4\, dx \quad=\quad \frac{4096π}{5}$ units³

19) $ y=e^x+1,\quad x=0,\quad x=1,$ and $ y=0$

20) $ y=x^4,\quad x=0$, and $ y=1$

Answer:

$This figure is a graph in the first quadrant. It is a shaded region bounded above by the line y=1, below by the curve y=x^4, and to the left by the y-axis.$

$\displaystyle V = \int_0^1 \pi\left( 1^2 - \left( x^4\right)^2\right)\, dx = \int_0^1 \pi\left( 1 - x^8\right)\, dx \quad = \quad \frac{8π}{9}$ units³

21) $ y=\sqrt{x},\quad x=0,\quad x=4,$ and $ y=0$

22) $ y=\sin x,\quad y=\cos x,$ and $ x=0$

Answer:

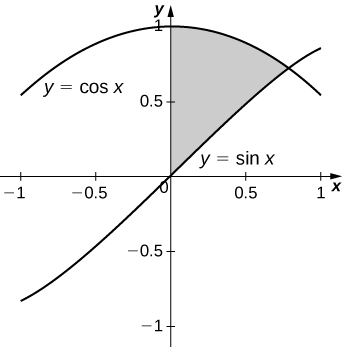
$This figure is a shaded region bounded above by the curve y=cos(x), below to the left by the y-axis and below to the right by y=sin(x). The shaded region is in the first quadrant.$

$\displaystyle V = \int_0^{\pi/4} \pi \left( \cos^2 x - \sin^2 x\right) \, dx = \int_0^{\pi/4} \pi \cos 2x \, dx \quad=\quad \frac{π}{2}$ units³

23) $ y=\dfrac{1}{x},\quad x=2$, and $ y=3$

24) $ x^2−y^2=9$ and $ x+y=9,\quad y=0$ and $ x=0$

Answer:

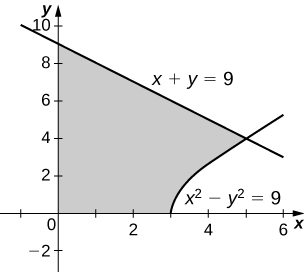
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the line x + y=9, below by the x-axis, to the left by the y-axis, and to the left by the curve x^2-y^2=9.$

$V = 207π$ units³

For exercises 25 - 32, draw the region bounded by the curves. Then, find the volume when the region is rotated around the $y$-axis.

25) $ y=4−\dfrac{1}{2}x,\quad x=0,$ and $ y=0$

26) $ y=2x^3,\quad x=0,\quad x=1,$ and $ y=0$

Answer:

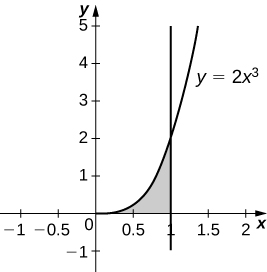
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the curve y=2x^3, below by the x-axis, and to the right by the line x=1.$

$V = \frac{4π}{5}$ units³

27) $ y=3x^2,\quad x=0,$ and $ y=3$

28) $ y=\sqrt{4−x^2},\quad y=0,$ and $ x=0$

Answer:

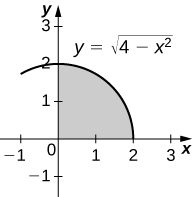
$This figure is a graph in the first quadrant. It is a quarter of a circle with center at the origin and radius of 2. It is shaded on the inside.$

$V = \frac{16π}{3}$ units³

29) $ y=\dfrac{1}{\sqrt{x+1}},\quad x=0$, and $ x=3$

30) $ x=\sec(y)$ and $ y=\dfrac{π}{4},\quad y=0$ and $ x=0$

Answer:

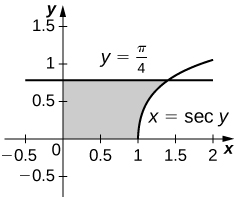
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the line y=pi/4, to the right by the curve x=sec(y), below by the x-axis, and to the left by the y-axis.$

$V = π$ units³

31) $ y=\dfrac{1}{x+1},\quad x=0$, and $ x=2$

32) $ y=4−x,\quad y=x,$ and $ x=0$

Answer:

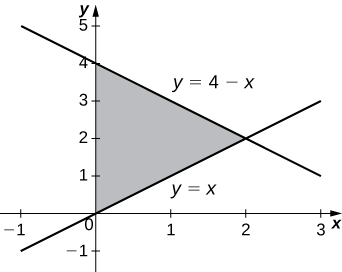
$This figure is a graph in the first quadrant. It is a shaded triangle bounded above by the line y=4-x, below by the line y=x, and to the left by the y-axis.$

$V = \frac{16π}{3}$ units³

For exercises 33 - 40, draw the region bounded by the curves. Then, find the volume when the region is rotated around the $x$-axis.

33) $ y=x+2,\quad y=x+6,\quad x=0$, and $ x=5$

34) $ y=x^2$ and $ y=x+2$

Answer:

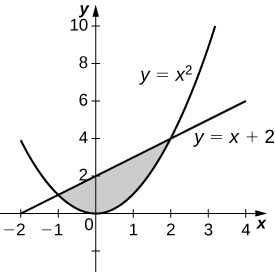
$This figure is a graph above the x-axis. It is a shaded region bounded above by the line y=x+2, and below by the parabola y=x^2.$

$V = \frac{72π}{5}$ units³

35) $ x^2=y^3$ and $ x^3=y^2$

36) $ y=4−x^2$ and $ y=2−x$

Answer:

$This figure is a shaded region bounded above by the curve y=4-x^2 and below by the line y=2-x.$

$V = \frac{108π}{5}$ units³

37) [T] $ y=\cos x,\quad y=e^{−x},\quad x=0$, and $ x=1.2927$

38) $ y=\sqrt{x}$ and $ y=x^2$

Answer:

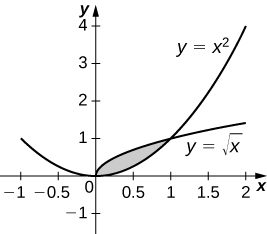
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the curve y=squareroot(x), below by the curve y=x^2.$

$V = \frac{3π}{10}$ units³

39) $ y=\sin x,\quad y=5\sin x,\quad x=0$ and $ x=π$

40) $ y=\sqrt{1+x^2}$ and $ y=\sqrt{4−x^2}$

Answer:

$This figure is a shaded region bounded above by the curve y=squareroot(4-x^2) and, below by the curve y=squareroot(1+x^2).$

$V = 2\sqrt{6}π$ units³

For exercises 41 - 45, draw the region bounded by the curves. Then, use the washer method to find the volume when the region is revolved around the $y$-axis.

41) $ y=\sqrt{x},\quad x=4$, and $ y=0$

42) $ y=x+2,\quad y=2x−1$, and $ x=0$

Answer:

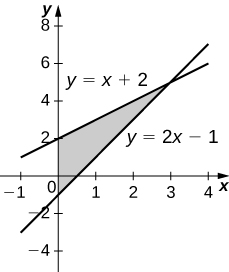
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the line y=x+2, below by the line y=2x-1, and to the left by the y-axis.$

$V = 9π$ units³

43) $ y=\dfrac{3}{x}$ and $ y=x^3$

44) $ x=e^{2y},\quad x=y^2,\quad y=0$, and $ y=\ln(2)$

Answer:

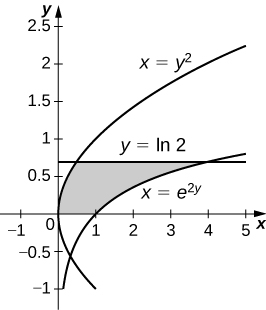
$This figure is a graph in the first quadrant. It is a shaded region bounded above by the curve y=ln(2), below by the x-axis, to the left by the curve x=y^2, and to the right by the curve x=e^(2y).$

$V = \dfrac{π}{20}(75−4\ln^5(2))$ units³

45) $ x=\sqrt{9−y^2},\quad x=e^{−y},\quad y=0$, and $ y=3$

46) Yogurt containers can be shaped like frustums. Rotate the line $ y=\left(\frac{1}{m}\right)x$ around the $y$-axis to find the volume between $ y=a$ and $ y=b$.

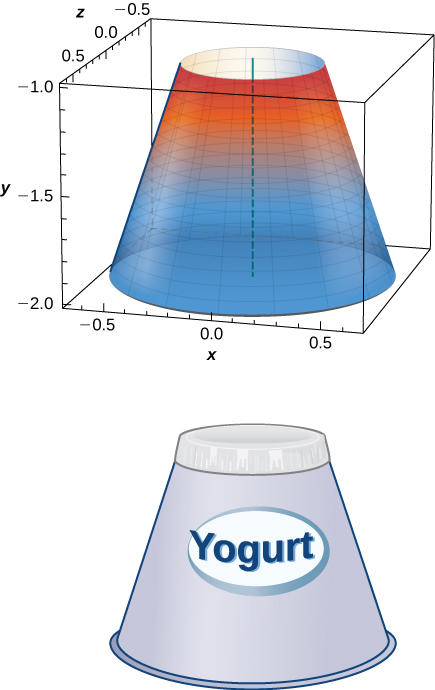
$This figure has two parts. The first part is a solid cone. The base of the cone is wider than the top. It is shown in a 3-dimensional box. Underneath the cone is an image of a yogurt container with the same shape as the figure.$

Answer:: $V = \dfrac{m^2π}{3}(b^3−a^3)$ units³

47) Rotate the ellipse $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1$ around the $x$-axis to approximate the volume of a football, as seen here.

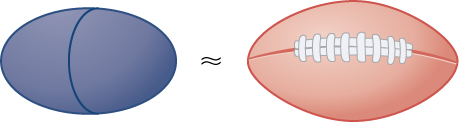
$This figure has an oval that is approximately equal to the image of a football.$

48) Rotate the ellipse $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1$ around the $y$-axis to approximate the volume of a football.

Answer:: $V = \frac{4a^2bπ}{3}$ units³

49) A better approximation of the volume of a football is given by the solid that comes from rotating $ y=\sin x) around the \(x$-axis from $ x=0$ to $ x=π$. What is the volume of this football approximation, as seen here?

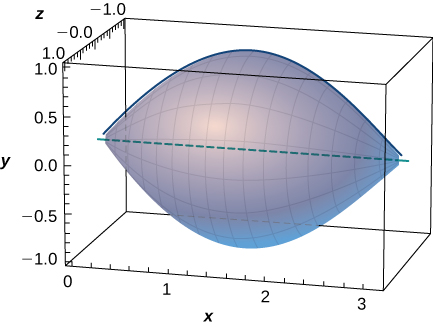
$This figure has a 3-dimensional oval shape. It is inside of a box parallel to the x axis on the bottom front edge of the box. The y-axis is vertical to the solid.$

For exercises 51 - 56, find the volume of the solid described.

51) The base is the region between $ y=x$ and $ y=x^2$. Slices perpendicular to the $x$-axis are semicircles.

52) The base is the region enclosed by the generic ellipse $ \dfrac{x^2}{a^2}+\dfrac{y^2}{b^2}=1.$ Slices perpendicular to the $x$-axis are semicircles.

Answer:: $V = \frac{2ab^2π}{3}$ units³

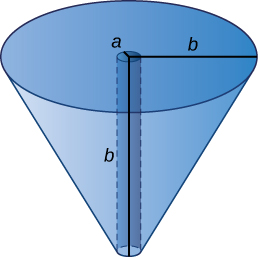
53) Bore a hole of radius a down the axis of $a$ right cone and through the base of radius $b$, as seen here.

$This figure is an upside down cone. It has a radius of the top as “b”, center at “a”, and height as “b”.$

54) Find the volume common to two spheres of radius $r$ with centers that are $2h$ apart, as shown here.

$This figure has two circles that intersect. Both circles have radius “r”. There is a line segment from one center to the other. In the middle of the intersection of the circles is point “h”. It is on the line segment.$

Answer:: $V = \frac{π}{12}(r+h)^2(6r−h)$ units³

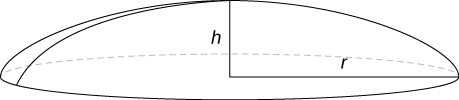
55) Find the volume of a spherical cap of height $h$ and radius $r$ where $h<r$, as seen here.

$This figure a portion of a sphere. This spherical cap has radius “r” and height “h”.$

56) Find the volume of a sphere of radius $R$ with a cap of height $h$ removed from the top, as seen here.

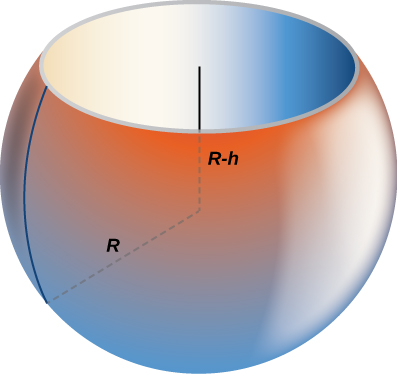
$This figure is a sphere with a top portion removed. The radius of the sphere is “R”. The distance from the center to where the top portion is removed is “R-h”.$

Answer:: $V = \dfrac{π}{3}(h+R)(h−2R)^2$ units³

6.3: Volumes of Revolution: Cylindrical Shells

For exercises 1 - 6, find the volume generated when the region between the two curves is rotated around the given axis. Use both the shell method and the washer method. Use technology to graph the functions and draw a typical slice by hand.

1) [T] Over the curve of $ y=3x,$ $x=0,$ and $ y=3$ rotated around the $y$-axis.

2) [T] Under the curve of $ y=3x,$ $x=0$, and $ x=3$ rotated around the $y$-axis.

Answer:

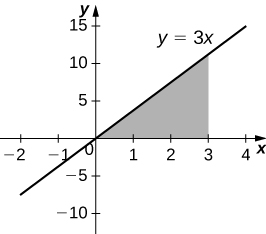
$This figure is a graph in the first quadrant. It is the line y=3x. Under the line and above the x-axis there is a shaded region. The region is bounded to the right at x=3.$

$V = 54π$ units³

3) [T] Over the curve of $ y=3x,$ $x=0$, and $ y=3$ rotated around the $x$-axis.

4) [T] Under the curve of $ y=3x,$ $x=0,$ and $ x=3$ rotated around the $x$-axis.

Answer:

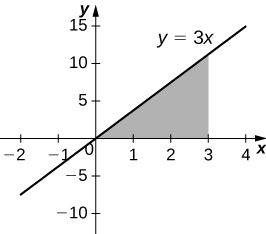
$This figure is a graph in the first quadrant. It is the line y=3x. Under the line and above the x-axis there is a shaded region. The region is bounded to the right at x=3.$

$V = 81π$ units³

5) [T] Under the curve of $ y=2x^3,\;x=0,$ and $ x=2$ rotated around the $y$-axis.

6) [T] Under the curve of $ y=2x^3,\;x=0,$ and $ x=2$ rotated around the $x$-axis.

Answer:

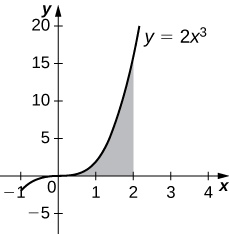
$This figure is a graph in the first quadrant. It is the increasing curve y=2x^3. Under the curve and above the x-axis there is a shaded region. The region is bounded to the right at x=2.$

$V = \frac{512π}{7}$ units³

For exercises 7 - 16, use shells to find the volumes of the given solids. Note that the rotated regions lie between the curve and the $x$-axis and are rotated around the $y$-axis.

7) $ y=1−x^2,$ $x=0,$ and $ x=1$

8) $ y=5x^3,$ $x=0$, and $ x=1$

Answer:: $V = 2π$ units³

9) $ y=\dfrac{1}{x},$ $x=1,$ and $ x=100$

10) $ y=\sqrt{1−x^2},$ $x=0$, and $ x=1$

Answer:: $V= \frac{2π}{3}$ units³

11) $ y=\dfrac{1}{1+x^2},$ $x=0$,and $ x=3$

12) $ y=\sin x^2,x=0$, and $ x=\sqrt{π}$

Answer:: $V= 2π$ units³

13) $ y=\dfrac{1}{\sqrt{1−x^2}},$ $x=0$, and $ x=\frac{1}{2}$

14) $ y=\sqrt{x},$ $x=0$, and $ x=1$

Answer:: $V = \frac{4π}{5}$ units³

15) $ y=(1+x^2)^3,$ $x=0$, and $ x=1$

16) $ y=5x^3−2x^4,$ $x=0$, and $ x=2$

Answer:: $V= \frac{64π}{3}$ units³

For exercises 17 - 26, use shells to find the volume generated by rotating the regions between the given curve and $ y=0$ around the $x$-axis.

17) $ y=\sqrt{1−x^2},$ $x=0$, and $ x=1$

18) $ y=x^2,$ $x=0$, and $ x=2$

Answer:: $V = \frac{32π}{5}$ units³

19) $ y=e^x,$ $x=0$, and $ x=1$

20) $ y=\ln(x),$ $x=1$, and $ x=e$

Answer:: $V= π(e−2)$ units³

21) $ x=\dfrac{1}{1+y^2},$ $y=1$, and $ y=4$

22) $ x=\dfrac{1+y^2}{y},$ $y=0$, and $ y=2$

Answer:: $V= \frac{28π}{3}$ units³

23) $ x=\cos y,$ $y=0$, and $ y=π$

24) $ x=y^3−4y^2,$ $x=−1$, and $ x=2$

Answer:: $V= \frac{84π}{5}$ units³

25) $ x=ye^y,$ $x=−1$, and $ x=2$

26) $ x=e^y\cos y,$ $x=0$, and $ x=π$

Answer:: $V = e^ππ^2$ units³

For exercises 27 - 36, find the volume generated when the region between the curves is rotated around the given axis.

27) $ y=3−x$, $y=0$, $x=0$, and $ x=2$ rotated around the $y$-axis.

28) $ y=x^3$, $y=0$, $x=0$, and $ y=8$ rotated around the $y$-axis.

Answer:: $ V=\frac{64π}{5}$ units³

29) $ y=x^2,$ $y=x,$ rotated around the $y$-axis.

30) $ y=\sqrt{x},$ $x=0$, and $ x=1$ rotated around the line $ x=2.$

Answer:: $V=\frac{28π}{15}$ units³

31) $ y=\dfrac{1}{4−x},$ $x=1,$ and $ x=2$ rotated around the line $ x=4$.

32) $ y=\sqrt{x}$ and $ y=x^2$ rotated around the $y$-axis.

Answer:: $V=\frac{3π}{10}$ units³

33) $ y=\sqrt{x}$ and $ y=x^2$ rotated around the line $ x=2$.

34) $ x=y^3,$ $y=\dfrac{1}{x},$ $x=1$, and $ y=2$ rotated around the $x$-axis.

Answer:: $ \frac{52π}{5}$ units³

35) $ x=y^2$ and $ y=x$ rotated around the line $ y=2$.

36) [T] Left of $ x=\sin(πy)$, right of $ y=x$, around the $y$-axis.

Answer:: $V \approx 0.9876$ units³

For exercises 37 - 44, use technology to graph the region. Determine which method you think would be easiest to use to calculate the volume generated when the function is rotated around the specified axis. Then, use your chosen method to find the volume.

37) [T] $ y=x^2$ and $ y=4x$ rotated around the $y$-axis.

38) [T] $ y=\cos(πx),y=\sin(πx),x=\frac{1}{4}$, and $ x=\frac{5}{4}$ rotated around the $y$-axis.

Answer:

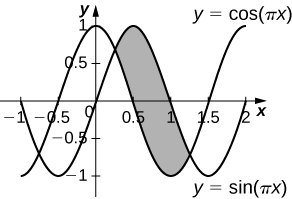
$This figure is a graph. On the graph are two curves, y=cos(pi times x) and y=sin(pi times x). They are periodic curves resembling waves. The curves intersect in the first quadrant and also the fourth quadrant. The region between the two points of intersection is shaded.$

$V = 3\sqrt{2}$ units³

39) [T] $ y=x^2−2x,\; x=2,$ and $ x=4$ rotated around the $y$-axis.

40) [T] $ y=x^2−2x,\; x=2,$ and $ x=4$ rotated around the $x$-axis.

Answer:

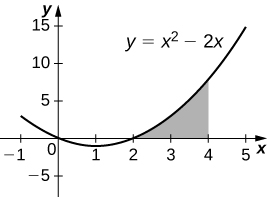
$This figure is a graph in the first quadrant. It is the parabola y=x^2-2x. . Under the curve and above the x-axis there is a shaded region. The region begins at x=2 and is bounded to the right at x=4.$

$V= \frac{496π}{15}$ units³

41) [T] $ y=3x^3−2,\; y=x$, and $ x=2$ rotated around the $x$-axis.

42) [T] $ y=3x^3−2,\; y=x$, and $ x=2$ rotated around the $y$-axis.

Answer:

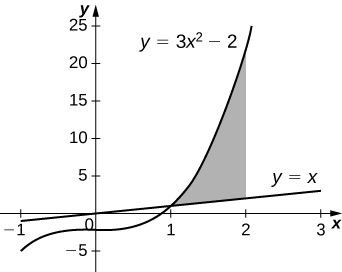
$This figure is a graph in the first quadrant. There are two curves on the graph. The first curve is y=3x^2-2 and the second curve is y=x. Between the curves there is a shaded region. The region begins at x=1 and is bounded to the right at x=2.$

$ V = \frac{398π}{15}$ units³

43) [T] $ x=\sin(πy^2)$ and $ x=\sqrt{2}y$ rotated around the $x$-axis.

44) [T] $ x=y^2,\; x=y^2−2y+1$, and $ x=2$ rotated around the $y$-axis.

Answer:

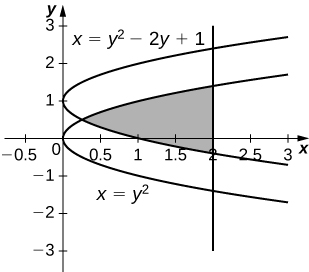
$This figure is a graph. There are two curves on the graph. The first curve is x=y^2-2y+1 and is a parabola opening to the right. The second curve is x=y^2 and is a parabola opening to the right. Between the curves there is a shaded region. The shaded region is bounded to the right at x=2.$

$ V =15.9074$ units³

For exercises 45 - 51, use the method of shells to approximate the volumes of some common objects, which are pictured in accompanying figures.

45) Use the method of shells to find the volume of a sphere of radius $ r$.

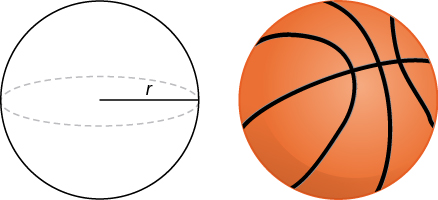
$This figure has two images. The first is a circle with radius r. The second is a basketball.$

46) Use the method of shells to find the volume of a cone with radius $ r$ and height $ h$.

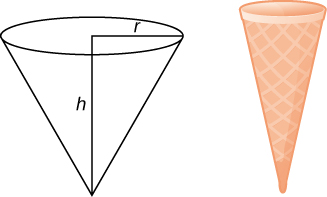
$This figure has two images. The first is an upside-down cone with radius r and height h. The second is an ice cream cone.$

Answer:: $V = \frac{1}{3}πr^2h$ units³

47) Use the method of shells to find the volume of an ellipse $ (x^2/a^2)+(y^2/b^2)=1$ rotated around the $x$-axis.

$This figure has two images. The first is an ellipse with a the horizontal distance from the center to the edge and b the vertical distance from the center to the top edge. The second is a watermelon.$

48) Use the method of shells to find the volume of a cylinder with radius $ r$ and height $ h$.

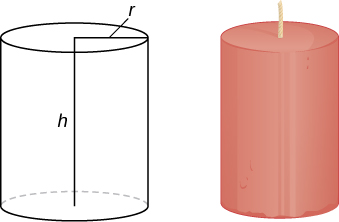
$This figure has two images. The first is a cylinder with radius r and height h. The second is a cylindrical candle.$

Answer:: $V= πr^2h$ units³

49) Use the method of shells to find the volume of the donut created when the circle $ x^2+y^2=4$ is rotated around the line $ x=4$.

$This figure has two images. The first has two ellipses, one inside of the other. The radius of the path between them is 2 units. The second is a doughnut.$

50) Consider the region enclosed by the graphs of $ y=f(x),\; y=1+f(x),\; x=0,\; y=0,$ and $ x=a>0$. What is the volume of the solid generated when this region is rotated around the $y$-axis? Assume that the function is defined over the interval $ [0,a]$.

Answer:: $ V=πa^2$ units³

51) Consider the function $ y=f(x)$, which decreases from $ f(0)=b$ to $ f(1)=0$. Set up the integrals for determining the volume, using both the shell method and the disk method, of the solid generated when this region, with $ x=0$ and $ y=0$, is rotated around the $y$-axis. Prove that both methods approximate the same volume. Which method is easier to apply? (Hint: Since $ f(x)$ is one-to-one, there exists an inverse $ f^{−1}(y)$.)

6.4: Arc Length of a Curve and Surface Area

For exercises 1 - 3, find the length of the functions over the given interval.

1) $ y=5x$ from $ x=0$ to $ x=2$

Answer:: $s = 2\sqrt{26}$ units

2) $ y=−\frac{1}{2}x+25$ from $ x=1$ to $ x=4$

3) $ x=4y$ from $ y=−1$ to $ y=1$

Answer:: $ s = 2\sqrt{17}$ units

4) Pick an arbitrary linear function $ x=g(y)$ over any interval of your choice $ (y_1,y_2).$ Determine the length of the function and then prove the length is correct by using geometry.

5) Find the surface area of the volume generated when the curve $ y=\sqrt{x}$ revolves around the $x$-axis from $ (1,1)$ to $ (4,2)$, as seen here.

Answer:: $A = \frac{π}{6}(17\sqrt{17}−5\sqrt{5})$ units²

6) Find the surface area of the volume generated when the curve $ y=x^2$ revolves around the $y$-axis from $ (1,1)$ to $ (3,9)$.

For exercises 7 - 16, find the lengths of the functions of $x$ over the given interval. If you cannot evaluate the integral exactly, use technology to approximate it.

7) $ y=x^{3/2}$ from $ (0,0)$ to $ (1,1)$

Answer:: $s= \frac{13\sqrt{13}−8}{27}$ units

8) $ y=x^{2/3}$ from $ (1,1)$ to $ (8,4)$

9) $ y=\frac{1}{3}(x^2+2)^{3/2}$ from $ x=0$ to $ x=1$

Answer:: $s= \frac{4}{3}$ units

10) $ y=\frac{1}{3}(x^2−2)^{3/2}$ from $ x=2$ to $ x=4$

11) [T] $ y=e^x$ on $ x=0$ to $ x=1$

Answer:: $s \approx 2.0035$ units

12) $ y=\dfrac{x^3}{3}+\dfrac{1}{4x}$ from $ x=1$ to $ x=3$

13) $ y=\dfrac{x^4}{4}+\dfrac{1}{8x^2}$ from $ x=1$ to $ x=2$

Answer:: $s= \frac{123}{32}$ units

14) $ y=\dfrac{2x^{3/2}}{3}−\dfrac{x^{1/2}}{2}$ from $ x=1$ to $ x=4$

15) $ y=\frac{1}{27}(9x^2+6)^{3/2}$ from $ x=0$ to $ x=2$

Answer:: $s=10$ units

16) [T] $ y=\sin x$ on $ x=0$ to $ x=π$

For exercises 17 - 26, find the lengths of the functions of $y$ over the given interval. If you cannot evaluate the integral exactly, use technology to approximate it.

17) $ y=\dfrac{5−3x}{4}$ from $ y=0$ to $ y=4$

Answer:: $s= \frac{20}{3}$ units

18) $ x=\frac{1}{2}(e^y+e^{−y})$ from $ y=−1$ to $ y=1$

19) $ x=5y^{3/2}$ from $ y=0$ to $ y=1$

Answer:: $s= \frac{1}{675}(229\sqrt{229}−8)$ units

20) [T] $ x=y^2$ from $ y=0$ to $ y=1$

21) $ x=\sqrt{y}$ from $ y=0$ to $ y=1$

Answer:: $s= \frac{1}{8}(4\sqrt{5}+\ln(9+4\sqrt{5}))$ units

22) $ x=\frac{2}{3}(y^2+1)^{3/2}$ from $ y=1$ to $ y=3$

23) [T] $ x=\tan y$ from $ y=0$ to $ y=\frac{3}{4}$

Answer:: $s \approx 1.201$ units

24) [T] $ x=\cos^2y$ from $ y=−\frac{π}{2}$ to $ y=\frac{π}{2}$

25) [T] $ x=4^y$ from $ y=0$ to $ y=2$

Answer:: $s \approx 15.2341$ units

26) [T] $ x=\ln(y)$ on $ y=\dfrac{1}{e}$ to $ y=e$

For exercises 27 - 34, find the surface area of the volume generated when the following curves revolve around the $x$-axis. If you cannot evaluate the integral exactly, use your calculator to approximate it.

27) $ y=\sqrt{x}$ from $ x=2$ to $ x=6$

Answer:: $A= \frac{49π}{3}$ units²

28) $ y=x^3$ from $ x=0$ to $ x=1$

29) $ y=7x$ from $ x=−1$ to $ x=1$

Answer:: $A = 70π\sqrt{2}$ units²

30) [T] $ y=\frac{1}{x^2}$ from $ x=1$ to $ x=3$

31) $ y=\sqrt{4−x^2}$ from $ x=0$ to $ x=2$

Answer:: $A = 8π$ units²

32) $ y=\sqrt{4−x^2}$ from $ x=−1$ to $ x=1$

33) $ y=5x$ from $ x=1$ to $ x=5$

Answer:: $A = 120π\sqrt{26}$ units²

34) [T] $ y=\tan x$ from $ x=−\frac{π}{4}$ to $ x=\frac{π}{4}$

For exercises 35 - 42, find the surface area of the volume generated when the following curves revolve around the $y$-axis. If you cannot evaluate the integral exactly, use your calculator to approximate it.

35) $ y=x^2$ from $ x=0$ to $ x=2$

Answer:: $A= \frac{π}{6}(17\sqrt{17}−1)$ units²

36) $ y=\frac{1}{2}x^2+\frac{1}{2}$ from $ x=0$ to $ x=1$

37) $ y=x+1$ from $ x=0$ to $ x=3$

Answer:: $A = 9\sqrt{2}π$ units²

38) [T] $ y=\dfrac{1}{x}$ from $ x=\dfrac{1}{2}$ to $ x=1$

39) $ y=\sqrt[3]{x}$ from $ x=1$ to $ x=27$

Answer:: $A = \frac{10\sqrt{10}π}{27}(73\sqrt{73}−1)$ units²

40) [T] $ y=3x^4$ from $ x=0$ to $ x=1$

41) [T] $ y=\dfrac{1}{\sqrt{x}}$ from $ x=1$ to $ x=3$

Answer:: $A \approx 25.645$ units²

42) [T] $ y=\cos x$ from $ x=0$ to $ x=\frac{π}{2}$

43) The base of a lamp is constructed by revolving a quarter circle $ y=\sqrt{2x−x^2}$ around the $y$-axis from $ x=1$ to $ x=2$, as seen here. Create an integral for the surface area of this curve and compute it.

Answer:: $A = 2π$ units²

44) A light bulb is a sphere with radius $1/2$ in. with the bottom sliced off to fit exactly onto a cylinder of radius $1/4$ in. and length $1/3$ in., as seen here. The sphere is cut off at the bottom to fit exactly onto the cylinder, so the radius of the cut is $1/4$ in. Find the surface area (not including the top or bottom of the cylinder).

45) [T] A lampshade is constructed by rotating $ y=1/x$ around the $x$-axis from $ y=1$ to $ y=2$, as seen here. Determine how much material you would need to construct this lampshade—that is, the surface area—accurate to four decimal places.

Answer:: $10.5017$ units²

46) [T] An anchor drags behind a boat according to the function $ y=24e^{−x/2}−24$, where $ y$ represents the depth beneath the boat and $ x$ is the horizontal distance of the anchor from the back of the boat. If the anchor is $ 23$ ft below the boat, how much rope do you have to pull to reach the anchor? Round your answer to three decimal places.

47) [T] You are building a bridge that will span $ 10$ ft. You intend to add decorative rope in the shape of $ y=5|\sin((xπ)/5)|$, where $ x$ is the distance in feet from one end of the bridge. Find out how much rope you need to buy, rounded to the nearest foot.

Answer:: $ 23$ ft

For exercise 48, find the exact arc length for the following problems over the given interval.

48) $ y=\ln(\sin x)$ from $ x=π/4$ to $ x=(3π)/4$. (Hint: Recall trigonometric identities.)

49) Draw graphs of $y=x^2, y=x^6$, and $y=x^{10}$. For $ y=x^n$, as $ n$ increases, formulate a prediction on the arc length from $ (0,0)$ to $ (1,1)$. Now, compute the lengths of these three functions and determine whether your prediction is correct.

Answer:: $2$

50) Compare the lengths of the parabola $x=y^2$ and the line $x=by$ from $(0,0)$ to $(b^2,b)$ as $b$ increases. What do you notice?

51) Solve for the length of $x=y^2$ from $(0,0)$ to $(1,1)$. Show that $ x=\dfrac{y^2}{2}$ from $(0,0)$ to $(2,2)$ is twice as long. Graph both functions and explain why this is so.

Answer:: Answers may vary

52) [T] Which is longer between $(1,1)$ and $\left(2,\frac{1}{2}\right)$: the hyperbola $y=\dfrac{1}{x}$ or the graph of $x+2y=3$?

53) Explain why the surface area is infinite when $y=1/x$ is rotated around the $x$-axis for $ 1≤x<∞,$ but the volume is finite.

Answer:: For more information, look up Gabriel’s Horn.

6.5: Physical Applications

Basic Work Problems

For exercises 1 - 6, find the work done.

1) Find the work done when a constant force $ F=12$ lb moves a chair from $ x=0.9$ to $ x=1.1$ ft.

2) How much work is done when a person lifts a $ 50$ lb box of comics onto a truck that is $ 3$ ft off the ground?

Answer:: $W = 150$ ft-lb

3) What is the work done lifting a $ 20$ kg child from the floor to a height of $ 2$ m? (Note that $ 1$ kg equates to $ 9.8$ N)

4) Find the work done when you push a box along the floor $ 2$ m, when you apply a constant force of $ F=100$ N.

Answer:: $W = 200$ J

5) Compute the work done for a force $ F=\dfrac{12}{x^2}$ N from $ x=1$ to $ x=2$ m.

6) What is the work done moving a particle from $ x=0$ to $ x=1$ m if the force acting on it is $ F=3x^2$ N?

Answer:: $W = 1$ J

Density Problems

In exercises 7 - 11, find the mass of the one-dimensional object.

7) A wire that is $2$ ft long (starting at $x=0$) and has a density function of $ρ(x)=x^2+2x$ lb/ft

8) A car antenna that is $3$ ft long (starting at $x=0)$ and has a density function of $ρ(x)=3x+2$ lb/ft

Answer:: $ \frac{39}{2}$

9) A metal rod that is $ 8$ in. long (starting at $ x=0$) and has a density function of $ ρ(x)=e^{1/2x}$ lb/in.

10) A pencil that is $ 4$ in. long (starting at $ x=2$) and has a density function of $ ρ(x)=\dfrac{5}{x}$ oz/in.

Answer:: $ \ln(243)$

11) A ruler that is $ 12$ in. long (starting at $ x=5$) and has a density function of $ ρ(x)=\ln(x)+(1/2)x^2$ oz/in.

In exercises 12 - 16, find the mass of the two-dimensional object that is centered at the origin.

12) An oversized hockey puck of radius $ 2$ in. with density function $ ρ(x)=x^3−2x+5$

Answer:: $ \frac{332π}{15}$

13) A frisbee of radius $ 6$ in. with density function $ ρ(x)=e^{−x}$

14) A plate of radius $ 10$ in. with density function $ ρ(x)=1+\cos(πx)$

Answer:: $ 100π$

15) A jar lid of radius $ 3$ in. with density function $ρ(x)=\ln(x+1)$

16) A disk of radius $5$ cm with density function $ρ(x)=\sqrt{3x}$

Answer:: $20π\sqrt{15}$

Spring Work Problems

17) A $ 12$-in. spring is stretched to $ 15$ in. by a force of $ 75$ lb. What is the spring constant?

18) A spring has a natural length of $ 10$ cm. It takes $ 2$ J to stretch the spring to $ 15$ cm. How much work would it take to stretch the spring from $ 15$ cm to $ 20$ cm?

Answer:: $W = 6$ J

19) A $ 1$-m spring requires $ 10$ J to stretch the spring to $ 1.1$ m. How much work would it take to stretch the spring from $ 1$ m to $ 1.2$ m?

20) A spring requires $ 5$ J to stretch the spring from $ 8$ cm to $ 12$ cm, and an additional $ 4$ J to stretch the spring from $ 12$ cm to $ 14$ cm. What is the natural length of the spring?

Answer:: The natural length is$ 5$ cm.

21) A shock absorber is compressed 1 in. by a weight of 1 ton. What is the spring constant?

22) A force of $ F=\left(20x−x^3\right)$ N stretches a nonlinear spring by $ x$ meters. What work is required to stretch the spring from $ x=0$ to $ x=2$ m?

Answer:: $W = 36$ J

Cable and Chain Work Problems

23) Find the work done by winding up a hanging cable of length $ 100$ ft and weight-density $ 5$ lb/ft.

24) For the cable in the preceding exercise, how much work is done to lift the cable $ 50$ ft?

Answer:: $W = 18,750$ ft-lb

25) For the cable in the preceding exercise, how much additional work is done by hanging a $ 200$ lb weight at the end of the cable?

Pyramid & Satellite/Rocket Work Problems

26) [T] A pyramid of height $ 500$ ft has a square base $ 800$ ft by $ 800$ ft. Find the area $ A$ at height $ h$. If the rock used to build the pyramid weighs approximately $ w=100\,\text{lb/ft}^3$, how much work did it take to lift all the rock?

Answer:: $W= \frac{32}{3}×10^9$ ft-lb

27) [T] For the pyramid in the preceding exercise, assume there were $ 1000$ workers each working $ 10$ hours a day, $ 5$ days a week, $ 50$ weeks a year. If each of the workers, on average, lifted ten 100-lb rocks $ 2$ ft/hr, how long did it take to build the pyramid?

28) [T] The force of gravity on a mass $ m$ is $ F=−((GMm)/x^2)$ newtons. For a rocket of mass $ m=1000$ kg, compute the work to lift the rocket from $ x=6400$ to $ x=6500$ km. (Note: $ G=6×10^{−17}\,\text{N m}^2/\text{kg}^2$ and $ M=6×10^{24}$ kg.)

Answer:: $W = 8.65×10^5$ J

29) [T] For the rocket in the preceding exercise, find the work to lift the rocket from $ x=6400$ to $ x=∞$.

Hydrostatic Force and Pressure

30) [T] A rectangular dam is $40$ ft high and $60$ ft wide. Compute the total force $F$ on the dam when

a. the surface of the water is at the top of the dam and

b. the surface of the water is halfway down the dam.

Answer:: a. $3,000,000$ lb,
b. $749,000$ lb

Pumping Work Problems

31) [T] Find the work required to pump all the water out of a cylinder that has a circular base of radius $ 5$ft and height $ 200$ ft. Use the fact that the density of water is $ 62$ lb/ft³.

32) [T] Find the work required to pump all the water out of the cylinder in the preceding exercise if the cylinder is only half full.

Answer:: $W = 23.25π$ million ft-lb

33) [T] How much work is required to pump out a swimming pool if the area of the base is $800 \, \text{ft}^2$, the water is $4$ ft deep, and the top is $1$ ft above the water level? Assume that the density of water is $ 62$ lb/ft³.

34) A cylinder of depth $H$ and cross-sectional area $A$ stands full of water at density $ρ$. Compute the work to pump all the water to the top.

Answer:: $W = \dfrac{AρH^2}{2}$

35) For the cylinder in the preceding exercise, compute the work to pump all the water to the top if the cylinder is only half full.

36) A cone-shaped tank has a cross-sectional area that increases with its depth: $ A=\dfrac{πr^2h^2}{H^3}$. Show that the work to empty it is half the work for a cylinder with the same height and base.

Answer:: Answers may vary.

6.6: Moments and Centers of Mass

In exercises 1 - 6, calculate the center of mass for the collection of masses given.

1) $m_1=2$ at $x_1=1$ and $m_2=4$ at $x_2=2$

2) $m_1=1$ at $x_1=−1$ and $m_2=3$ at $x_2=2$

Answer:: $x = \frac{5}{4}$

3) $m=3$ at $x=0,1,2,6$

4) Unit masses at $(x,y)=(1,0),(0,1),(1,1)$

Answer:: $\left(\frac{2}{3},\, \frac{2}{3}\right)$

5) $m_1=1$ at $(1,0)$ and $m_2=4$ at $(0,1)$

6) $m_1=1$ at $(1,0)$ and $m_2=3$ at $(2,2)$

Answer:: $\left(\frac{7}{4},\,\frac{3}{2}\right)$

In exercises 7 - 16, compute the center of mass $\bar x.$

7) $ρ=1$ for $x∈(−1,3)$

8) $ρ=x^2$ for $x∈(0,L)$

Answer:: $\dfrac{3L}{4}$

9) $ρ=1$ for $x∈(0,1)$ and $ρ=2$ for $x∈(1,2)$

10) $ρ=\sin x$ for $x∈(0,π)$

Answer:: $\frac{π}{2}$

11) $ρ=\cos x$ for $x∈\left(0,\frac{π}{2}\right)$

12) $ρ=e^x$ for $x∈(0,2)$

Answer:: $\dfrac{e^2+1}{e^2−1}$

13) $ρ=x^3+xe^{−x}$ for $x∈(0,1)$

14) $ρ=x\sin x$ for $x∈(0,π)$

Answer:: $\dfrac{π^2−4}{π}$

15) $ρ=\sqrt{x}$ for $x∈(1,4)$

16) $ρ=\ln x$ for $x∈(1,e)$

Answer:: $\frac{1}{4}(1+e^2)$

In exercises 17 - 19, compute the center of mass $(\bar{x},\bar{y}).$ Use symmetry to help locate the center of mass whenever possible.

17) $ρ=7$ in the square $0≤x≤1, \; 0≤y≤1$

18) $ρ=3$ in the triangle with vertices $(0,0), \, (a,0)$, and $(0,b)$

Answer:: $\left(\frac{a}{3},\, \frac{b}{3}\right)$

19) $ρ=2$ for the region bounded by $y=\cos(x), \; y=−\cos(x), \; x=−\frac{π}{2}$, and $x=\frac{π}{2}$

In exercises 20 - 26, use a calculator to draw the region, then compute the center of mass $(\bar{x},\bar{y}).$ Use symmetry to help locate the center of mass whenever possible.

20) [T] The region bounded by $y=\cos(2x), \; x=−\frac{π}{4}$, and $x=\frac{π}{4}$

Answer:: $\left(0,\frac{π}{8}\right)$

21) [T] The region between $y=2x^2, \; y=0, \; x=0,$ and $x=1$

22) [T] The region between $y=\frac{5}{4}x^2$ and $y=5$

Answer:: $(0,3)$

23) [T] Region between $y=\sqrt{x}, \; y=\ln x, \; x=1,$ and $x=4$

24) [T] The region bounded by $y=0$ and $\dfrac{x^2}{4}+\dfrac{y^2}{9}=1$

Answer:: $\left(0,\frac{4}{π}\right)$

25) [T] The region bounded by $y=0, \; x=0,$ and $\dfrac{x^2}{4}+\dfrac{y^2}{9}=1$

26) [T] The region bounded by $y=x^2$ and $y=x^4$ in the first quadrant

Answer:: $\left(\frac{5}{8},\, \frac{1}{3}\right)$

In exercises 27 - 31, use the theorem of Pappus to determine the volume of the shape.

27) Rotating $y=mx$ around the $x$-axis between $x=0$ and $x=1$

28) Rotating $y=mx$ around the $y$-axis between $x=0$ and $x=1$

Answer:: $V = \frac{mπ}{3}$ units³

29) A general cone created by rotating a triangle with vertices $(0,0), \, (a,0),$ and $(0,b)$ around the $y$-axis. Does your answer agree with the volume of a cone?

30) A general cylinder created by rotating a rectangle with vertices $(0,0), \, (a,0), \, (0,b),$ and $(a,b)$ around the $y$-axis. Does your answer agree with the volume of a cylinder?

Answer:: $V = πa^2b$ units³

31) A sphere created by rotating a semicircle with radius $a$ around the $y$-axis. Does your answer agree with the volume of a sphere?

In exercises 32 - 36, use a calculator to draw the region enclosed by the curve. Find the area $M$ and the centroid $(\bar{x},\bar{y})$ for the given shapes. Use symmetry to help locate the center of mass whenever possible.

32) [T] Quarter-circle: $y=\sqrt{1−x^2}, \; y=0$, and $x=0$

Answer:: $\left(\frac{4}{3π},\, \frac{4}{3π}\right)$

33) [T] Triangle: $y=x, \; y=2−x$, and $y=0$

34) [T] Lens: $y=x^2$ and $y=x$

Answer:: $\left(\frac{1}{2},\, \frac{2}{5}\right)$

35) [T] Ring: $y^2+x^2=1$ and $y^2+x^2=4$

36) [T] Half-ring: $y^2+x^2=1, \; y^2+x^2=4,$ and $y=0$

Answer:: $\left(0,\, \frac{28}{9π}\right)$

37) Find the generalized center of mass in the sliver between $y=x^a$ and $y=x^b$ with $a>b$. Then, use the Pappus theorem to find the volume of the solid generated when revolving around the $y$-axis.

38) Find the generalized center of mass between $y=a^2−x^2, \; x=0$, and $y=0$. Then, use the Pappus theorem to find the volume of the solid generated when revolving around the $y$-axis.

Answer:: Center of mass: $\left(\frac{a}{6},\,\frac{4a^2}{5}\right),$
Volume: $\dfrac{2πa^4}{9}$ units³

39) Find the generalized center of mass between $y=b\sin(ax),\; x=0,$ and $x=\dfrac{π}{a}.$ Then, use the Pappus theorem to find the volume of the solid generated when revolving around the $y$-axis.

40) Use the theorem of Pappus to find the volume of a torus (pictured here). Assume that a disk of radius $a$ is positioned with the left end of the circle at $x=b, \, b>0,$ and is rotated around the $y$-axis.

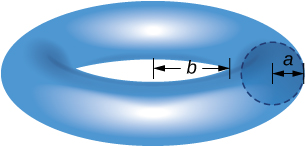
$This figure is a torus. It has inner radius of b. Inside of the torus is a cross section that is a circle. The circle has radius a.$

Answer:: Volume: $V = 2\pi^2a^2(b+a)$

41) Find the center of mass $(\bar{x},\bar{y})$ for a thin wire along the semicircle $y=\sqrt{1−x^2}$ with unit mass. (Hint: Use the theorem of Pappus.)

6.7: Integrals, Exponential Functions, and Logarithms

In exercises 1 - 3, find the derivative $\dfrac{dy}{dx}$.

1) $y=\ln(2x)$

Answer:: $\dfrac{dy}{dx} = \dfrac{1}{x}$

2) $y=\ln(2x+1)$

3) $y=\dfrac{1}{\ln x}$

Answer:: $\dfrac{dy}{dx} = −\dfrac{1}{x(\ln x)^2}$

In exercises 4 - 5, find the indefinite integral.

4) $\displaystyle ∫\frac{dt}{3t}$

5) $\displaystyle ∫\frac{dx}{1+x}$

Answer:: $\displaystyle ∫\frac{dx}{1+x} = \ln|x+1|+C$

In exercises 6 - 15, find the derivative $\dfrac{dy}{dx}.$ (You can use a calculator to plot the function and the derivative to confirm that it is correct.)

6) [T] $y=\dfrac{\ln x}{x}$

7) [T] $y=x\ln x$

Answer:: $\dfrac{dy}{dx} = \ln(x)+1$

8) [T] $y=\log_{10}x$

9) [T] $y=\ln(\sin x)$

Answer:: $\dfrac{dy}{dx} = \cot x$

10) [T] $y=\ln(\ln x)$

11) [T] $y=7\ln(4x)$

Answer:: $\dfrac{dy}{dx} = \frac{7}{x}$

12) [T] $y=\ln\big((4x)^7\big)$

13) [T] $y=\ln(\tan x)$

Answer:: $\dfrac{dy}{dx} = \csc x\sec x$

14) [T] $y=\ln(\tan 3x)$

15) [T] $y=\ln(\cos^2x)$

Answer:: $\dfrac{dy}{dx} = −2\tan x$

In exercises 16 - 25, find the definite or indefinite integral.

16) $\displaystyle ∫^1_0\frac{dx}{3+x}$

17) $\displaystyle ∫^1_0\frac{dt}{3+2t}$

Answer:: $\displaystyle ∫^1_0\frac{dt}{3+2t} = \tfrac{1}{2}\ln\left(\tfrac{5}{3}\right)$

18) $\displaystyle ∫^2_0\frac{x}{x^2+1}\, dx$

19) $\displaystyle ∫^2_0\frac{x^3}{x^2+1}\,dx$

Answer:: $\displaystyle ∫^2_0\frac{x^3}{x^2+1}\,dx = 2−\tfrac{1}{2}\ln(5)$

20) $\displaystyle ∫^e_2\frac{dx}{x\ln x}$

21) $\displaystyle ∫^e_2\frac{dx}{(x\ln x)^2}$

Answer:: $\displaystyle ∫^e_2\frac{dx}{(x\ln x)^2} = \frac{1}{\ln(2)}−1$

22) $\displaystyle ∫\frac{\cos x}{\sin x}\, dx$

23) $\displaystyle ∫^{π/4}_0\tan x\,dx$

Answer:: $\displaystyle ∫^{π/4}_0\tan x\,dx = \tfrac{1}{2}\ln(2)$

24) $\displaystyle ∫\cot(3x)\,dx$

25) $\displaystyle ∫\frac{(\ln x)^2}{x}\, dx$

Answer:: $\displaystyle ∫\frac{(\ln x)^2}{x}\, dx = \tfrac{1}{3}(\ln x)^3$

In exercises 26 - 35, compute $\dfrac{dy}{dx}$ by differentiating $\ln y$.

26) $y=\sqrt{x^2+1}$

27) $y=\sqrt{x^2+1}\sqrt{x^2−1}$

Answer:: $\dfrac{dy}{dx} = \dfrac{2x^3}{\sqrt{x^2+1}\sqrt{x^2−1}}$

28) $y=e^{\sin x}$

29) $y=x^{−1/x}$

Answer:: $\dfrac{dy}{dx} = x^{−2−(1/x)}(\ln x−1)$

30) $y=e^{ex}$

31) $y=x^e$

Answer:: $\dfrac{dy}{dx} = ex^{e−1}$

32) $y=x^{(ex)}$

33) $y=\sqrt{x}\sqrt[3]{x}\sqrt[6]{x}$

Answer:: $\dfrac{dy}{dx} = 1$

34) $y=x^{−1/\ln x}$

35) $y=e^{−\ln x}$

Answer:: $\dfrac{dy}{dx} = −\dfrac{1}{x^2}$

In exercises 36 - 40, evaluate by any method.

36) $\displaystyle ∫^{10}_5\dfrac{dt}{t}−∫^{10x}_{5x}\dfrac{dt}{t}$

37) $\displaystyle ∫^{e^π}_1\dfrac{dx}{x}+∫^{−1}_{−2}\dfrac{dx}{x}$

Answer:: $π−\ln(2)$

38) $\dfrac{d}{dx}\left[\displaystyle ∫^1_x\dfrac{dt}{t}\right]$

39) $\dfrac{d}{dx}\left[\displaystyle ∫^{x^2}_x\dfrac{dt}{t}\right]$

Answer:: $\dfrac{1}{x}$

40) $\dfrac{d}{dx}\Big[\ln(\sec x+\tan x)\Big]$

In exercises 41 - 44, use the function $\ln x$. If you are unable to find intersection points analytically, use a calculator.

41) Find the area of the region enclosed by $x=1$ and $y=5$ above $y=\ln x$.

Answer:: $(e^5−6)\text{ units}^2$

42) [T] Find the arc length of $\ln x$ from $x=1$ to $x=2$.

43) Find the area between $\ln x$ and the $x$-axis from $x=1$ to $x=2$.

Answer:: $\ln(4)−1) \text{ units}^2$

44) Find the volume of the shape created when rotating this curve from $x=1$ to $x=2$ around the $x$-axis, as pictured here.

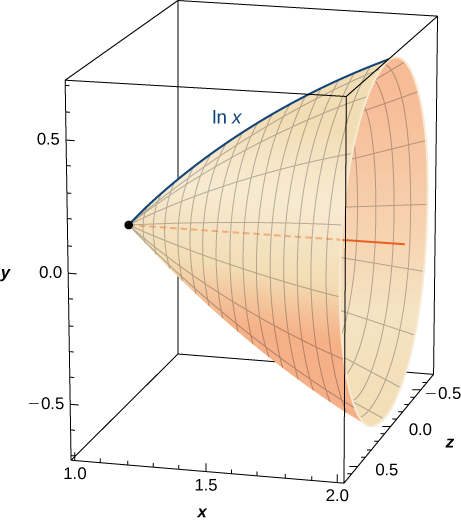
$This figure is a surface. It has been generated by revolving the curve ln x about the x-axis. The surface is inside of a cube showing it is 3-dimensinal.$

45) [T] Find the surface area of the shape created when rotating the curve in the previous exercise from $x=1$ to $x=2$ around the $x$-axis.

Answer:: $2.8656 \text{ units}^2$

If you are unable to find intersection points analytically in the following exercises, use a calculator.

46) Find the area of the hyperbolic quarter-circle enclosed by $x=2$ and $y=2$ above $y=1/x.$

47) [T] Find the arc length of $y=1/x$ from $x=1$ to $x=4$.

Answer:: $s = 3.1502$ units

48) Find the area under $y=1/x$ and above the $x$-axis from $x=1$ to $x=4$.

In exercises 49 - 53, verify the derivatives and antiderivatives.

49) $\dfrac{d}{dx}\Big[\ln(x+\sqrt{x^2+1})\Big]=\dfrac{1}{\sqrt{1+x^2}}$

50) $\dfrac{d}{dx}\Big[\ln\left(\frac{x−a}{x+a}\right)\Big]=\dfrac{2a}{(x^2−a^2)}$

51) $\dfrac{d}{dx}\Big[\ln\left(\frac{1+\sqrt{1−x^2}}{x}\right)\Big]=−\dfrac{1}{x\sqrt{1−x^2}}$

52) $\dfrac{d}{dx}\Big[\ln(x+\sqrt{x^2−a^2})\Big]=\dfrac{1}{\sqrt{x^2−a^2}}$

53) $\displaystyle ∫\frac{dx}{x\ln(x)\ln(\ln x)}=\ln|\ln(\ln x)|+C$

6.8: Exponential Growth and Decay

In exercises 1 - 2, answer True or False? If true, prove it. If false, find the true answer.

1) The doubling time for $y=e^{ct}$ is $\dfrac{\ln 2}{\ln c}$.

2) If you invest $$500$, an annual rate of interest of $3\%$ yields more money in the first year than a $2.5\%$ continuous rate of interest.

Answer:: True

3) If you leave a $100°C$ pot of tea at room temperature ($25°C$) and an identical pot in the refrigerator $(5°C)$, with $k=0.02$, the tea in the refrigerator reaches a drinkable temperature $(70°C)$ more than $5$ minutes before the tea at room temperature.

4) If given a half-life of t years, the constant $k$ for $y=e^{kt}$ is calculated by $k=\frac{\ln\left(\frac{1}{2}\right)}{t}$.

Answer:: False; $k=\dfrac{\ln 2}{t}$

In exercises 5 - 18, use $y=y_0e^{kt}.$

5) If a culture of bacteria doubles in $3$ hours, how many hours does it take to multiply by $10$?

6) If bacteria increase by a factor of $10$ in $10$ hours, how many hours does it take to increase by $100$?

Answer:: $20$ hours

7) How old is a skull that contains one-fifth as much radiocarbon as a modern skull? Note that the half-life of radiocarbon is $5730$ years.

8) If a relic contains $90\%$ as much radiocarbon as new material, can it have come from the time of Christ (approximately $2000$ years ago)? Note that the half-life of radiocarbon is $5730$ years.

Answer:: No. The relic is approximately $871$ years old.

9) The population of Cairo grew from $5$ million to $10$ million in $20$ years. Use an exponential model to find when the population was $8$ million.

10) The populations of New York and Los Angeles are growing at $1\%$ and $1.4\%$ a year, respectively. Starting from $8$ million (New York) and $6$ million (Los Angeles), when are the populations equal?

Answer:: $71.92$ years

11) Suppose the value of $$1$ in Japanese yen decreases at $2\%$ per year. Starting from $$1=¥250$, when will $$1=¥1$?

12) The effect of advertising decays exponentially. If $40\%$ of the population remembers a new product after $3$ days, how long will $20\%$remember it?

Answer:: $5$ days $6$ hours $27$minutes

13) If $y=1000$ at $t=3$ and $y=3000$ at $t=4$, what was $y_0$ at $t=0$?

14) If $y=100$ at $t=4$ and $y=10$ at $t=8$, when does $y=1$?

Answer:: At $t = 12$

15) If a bank offers annual interest of $7.5\%$ or continuous interest of $7.25\%,$ which has a better annual yield?

16) What continuous interest rate has the same yield as an annual rate of $9\%$?

Answer:: $s = 3.1502$ units

Solution: $8.618\%$

17) If you deposit $$5000$at $8\%$ annual interest, how many years can you withdraw $$500$ (starting after the first year) without running out of money?

18) You are trying to save $$50,000$ in $20$ years for college tuition for your child. If interest is a continuous $10\%,$ how much do you need to invest initially?

Answer:: $6766.76

19) You are cooling a turkey that was taken out of the oven with an internal temperature of $165°F$. After $10$ minutes of resting the turkey in a $70°F$ apartment, the temperature has reached $155°F$. What is the temperature of the turkey $20$ minutes after taking it out of the oven?

20) You are trying to thaw some vegetables that are at a temperature of $1°F$. To thaw vegetables safely, you must put them in the refrigerator, which has an ambient temperature of $44°F$. You check on your vegetables $2$ hours after putting them in the refrigerator to find that they are now $12°F$. Plot the resulting temperature curve and use it to determine when the vegetables reach $33°$.

Answer:: $9$hours $13$minutes

21) You are an archeologist and are given a bone that is claimed to be from a Tyrannosaurus Rex. You know these dinosaurs lived during the Cretaceous Era ($146$ million years to $65$ million years ago), and you find by radiocarbon dating that there is $0.000001\%$ the amount of radiocarbon. Is this bone from the Cretaceous?

22) The spent fuel of a nuclear reactor contains plutonium-239, which has a half-life of $24,000$ years. If $1$ barrel containing $10$ kg of plutonium-239 is sealed, how many years must pass until only $10$ g of plutonium-239 is left?

Answer:: $239,179$ years

For exercises 23 - 26, use the following table, which features the world population by decade.

Years since 1950	Population (millions)
0	2,556
10	3,039
20	3,706
30	4,453
40	5,279
50	6,083
60	6,849

Source: http:/www.factmonster.com/ipka/A0762181.html.

23) [T] The best-fit exponential curve to the data of the form $P(t)=ae^{bt}$ is given by $P(t)=2686e^{0.01604t}$. Use a graphing calculator to graph the data and the exponential curve together.

24) [T] Find and graph the derivative $y′$ of your equation. Where is it increasing and what is the meaning of this increase?

Answer:: $P'(t)=43e^{0.01604t}$. The population is always increasing.

25) [T] Find and graph the second derivative of your equation. Where is it increasing and what is the meaning of this increase?

26) [T] Find the predicted date when the population reaches $10$ billion. Using your previous answers about the first and second derivatives, explain why exponential growth is unsuccessful in predicting the future.

Answer:: The population reaches $10$ billion people in $2027$.

For exercises 27 - 29, use the following table, which shows the population of San Francisco during the 19th century.

Years since 1850	Population (thousands)
0	21.00
10	56.80
20	149.5
30	234.0

Source: http:/www.sfgenealogy.com/sf/history/hgpop.htm.

27) [T] The best-fit exponential curve to the data of the form $P(t)=ae^{bt}$ is given by $P(t)=35.26e^{0.06407t}$. Use a graphing calculator to graph the data and the exponential curve together.

28) [T] Find and graph the derivative $y′$ of your equation. Where is it increasing? What is the meaning of this increase? Is there a value where the increase is maximal?

Answer:: $P'(t)=2.259e^{0.06407t}$. The population is always increasing.

29) [T] Find and graph the second derivative of your equation. Where is it increasing? What is the meaning of this increase?

6.9: Calculus of the Hyperbolic Functions

1) [T] Find expressions for $\cosh x+\sinh x$ and $\cosh x−\sinh x.$ Use a calculator to graph these functions and ensure your expression is correct.

Answer: $e^x$ and $e^{−x}$

2) From the definitions of $\cosh(x)$ and $\sinh(x)$, find their antiderivatives.

3) Show that $\cosh(x)$ and $\sinh(x)$ satisfy $ y''=y$.

Answer: Answers may vary

4) Use the quotient rule to verify that $\dfrac{d}{dx}\big(\tanh(x)\big)=\text{sech}^2(x).$

5) Derive $\cosh^2(x)+\sinh^2(x)=\cosh(2x)$ from the definition.

Answer: Answers may vary

6) Take the derivative of the previous expression to find an expression for $\sinh(2x)$.

7) Prove $\sinh(x+y)=\sinh(x)\cosh(y)+\cosh(x)\sinh(y)$ by changing the expression to exponentials.

Answer: Answers may vary

8) Take the derivative of the previous expression to find an expression for $\cosh(x+y).$

In exercises 9 - 18, find the derivatives of the given functions and graph along with the function to ensure your answer is correct.

9) [T] $\cosh(3x+1)$

Answer: $3\sinh(3x+1)$

10) [T] $\sinh(x^2)$

11) [T] $\dfrac{1}{\cosh(x)}$

Answer: $−\tanh(x)\text{sech}(x)$

12) [T] $\sinh(\ln(x))$

13) [T] $\cosh^2(x)+\sinh^2(x)$

Answer: $4\cosh(x)\sinh(x)$

14) [T] $\cosh^2(x)−\sinh^2(x)$

15) [T] $\tanh(\sqrt{x^2+1})$

Answer: $\dfrac{x\text{sech}^2(\sqrt{x^2+1})}{\sqrt{x^2+1}}$

16) [T] $\dfrac{1+\tanh(x)}{1−\tanh(x)}$

17) [T] $\sinh^6(x)$

Answer: $6\sinh^5(x)\cosh(x)$

18) [T] $\ln(\text{sech}(x)+\tanh(x))$

In exercises 19 - 28, find the antiderivatives for the given functions.

19) $\cosh(2x+1)$

Answer: $\frac{1}{2}\sinh(2x+1)+C$

20) $\tanh(3x+2)$

21) $x\cosh(x^2)$

Answer: $\frac{1}{2}\sinh^2(x^2)+C$

22) $3x^3\tanh(x^4)$

23) $\cosh^2(x)\sinh(x)$

Answer: $\frac{1}{3}\cosh^3(x)+C$

24) $\tanh^2(x)\text{sech}^2(x)$

25) $\dfrac{\sinh(x)}{1+\cosh(x)}$

Answer: $\ln(1+\cosh(x))+C$

26) $\coth(x)$

27) $\cosh(x)+\sinh(x)$

Answer: $\cosh(x)+\sinh(x)+C$

28) $(\cosh(x)+\sinh(x))^n$

In exercises 29 - 35, find the derivatives for the functions.

29) $\tanh^{−1}(4x)$

Answer: $\dfrac{4}{1−16x^2}$

30) $\sinh^{−1}(x^2)$

31) $\sinh^{−1}(\cosh(x))$

Answer: $\dfrac{\sinh(x)}{\sqrt{\cosh^2(x)+1}}$

32) $\cosh^{−1}(x^3)$

33) $\tanh^{−1}(\cos(x))$

Answer: $−\csc(x)$

34) $e^{\sinh^{−1}(x)}$

35) $\ln(\tanh^{−1}(x))$

Answer: $−\dfrac{1}{(x^2−1)\tanh^{−1}(x)}$

In exercises 36 - 42, find the antiderivatives for the functions.

36) $\displaystyle ∫\frac{dx}{4−x^2}$

37) $\displaystyle ∫\frac{dx}{a^2−x^2}$

Answer: $\dfrac{1}{a}\tanh^{−1}\left(\dfrac{x}{a}\right)+C$

38) $\displaystyle ∫\frac{dx}{\sqrt{x^2+1}}$

39) $\displaystyle ∫\frac{xdx}{\sqrt{x^2+1}}$

Answer: $\sqrt{x^2+1}+C$

40) $\displaystyle ∫−\frac{dx}{x\sqrt{1−x^2}}$

41) $\displaystyle ∫\frac{e^x}{\sqrt{e^{2x}−1}}$

Answer: $\cosh^{−1}(e^x)+C$

42) $\displaystyle ∫−\frac{2x}{x^4−1}$

In exercises 43 - 45, use the fact that a falling body with friction equal to velocity squared obeys the equation $\dfrac{dv}{dt}=g−v^2$.

43) Show that $v(t)=\sqrt{g}\tanh(\sqrt{g}t)$ satisfies this equation.

Answer: Answers may vary

44) Derive the previous expression for $v(t)$ by integrating $\dfrac{dv}{g−v^2}=dt$.

45) [T] Estimate how far a body has fallen in $12$seconds by finding the area underneath the curve of $v(t)$.

Answer: $37.30$

In exercises 46 - 48, use this scenario: A cable hanging under its own weight has a slope $S=\dfrac{dy}{dx}$ that satisfies $\dfrac{dS}{dx}=c\sqrt{1+S^2}$. The constant $c$ is the ratio of cable density to tension.

46) Show that $S=\sinh(cx)$ satisfies this equation.

47) Integrate $\dfrac{dy}{dx}=\sinh(cx)$ to find the cable height $y(x)$ if $y(0)=1/c$.

Answer: $y=\frac{1}{c}\cosh(cx)$

48) Sketch the cable and determine how far down it sags at $x=0$.

In exercises 49 - 52, solve each problem.

49) [T] A chain hangs from two posts $2$m apart to form a catenary described by the equation $y=2\cosh(x/2)−1$. Find the slope of the catenary at the left fence post.

Answer: $−0.521095$

50) [T] A chain hangs from two posts four meters apart to form a catenary described by the equation $y=4\cosh(x/4)−3.$ Find the total length of the catenary (arc length).

51) [T] A high-voltage power line is a catenary described by $y=10\cosh(x/10)$. Find the ratio of the area under the catenary to its arc length. What do you notice?

Answer: $10$

52) A telephone line is a catenary described by $y=a\cosh(x/a).$ Find the ratio of the area under the catenary to its arc length. Does this confirm your answer for the previous question?

53) Prove the formula for the derivative of $y=\sinh^{−1}(x)$ by differentiating $x=\sinh(y).$

(Hint: Use hyperbolic trigonometric identities.)

54) Prove the formula for the derivative of $y=\cosh^{−1}(x)$ by differentiating $x=\cosh(y).$

(Hint: Use hyperbolic trigonometric identities.)

55) Prove the formula for the derivative of $y=\text{sech}^{−1}(x)$ by differentiating $x=\text{sech}(y).$

(Hint: Use hyperbolic trigonometric identities.)

56) Prove that $\cosh(x)+\sinh(x))^n=\cosh(nx)+\sinh(nx).$

57) Prove the expression for $\sinh^{−1}(x).$ Multiply $x=\sinh(y)=\dfrac{e^y−e^{−y}}{2}$ by $2e^y$ and solve for $y$. Does your expression match the textbook?

58) Prove the expression for $\cosh^{−1}(x).$ Multiply $x=\cosh(y)=\dfrac{e^y+e^{−y}}{2}$ by $2e^y$ and solve for $y$. Does your expression match the textbook?

Contributors

Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.

Chapter Review Exercises

True or False? Justify your answer with a proof or a counterexample.

1) The amount of work to pump the water out of a half-full cylinder is half the amount of work to pump the water out of the full cylinder.

Answer:: False

2) If the force is constant, the amount of work to move an object from $x=a$ to $x=b$ is $F(b−a)$.

3) The disk method can be used in any situation in which the washer method is successful at finding the volume of a solid of revolution.

Answer:: False

4) If the half-life of $seaborgium-266$ is $360$ ms, then $k=(\ln(2))/360.$

For exercises 5 - 8, use the requested method to determine the volume of the solid.

5) The volume that has a base of the ellipse $\dfrac{x^2}{4}+\dfrac{y^2}{9}=1$ and cross-sections of an equilateral triangle perpendicular to the $y$-axis. Use the method of slicing.

Answer:: $V = 32\sqrt{3}\, \text{units}^3$

6) $y=x^2−x$, from $x=1$ to $x=4$, rotated around the $y$-axis using the washer method

7) $x=y^2$ and $x=3y$ rotated around the $y$-axis using the washer method

Answer:: $V = \frac{162π}{5}\, \text{units}^3$

Solution:

8) $x=2y^2−y^3,\; x=0$,and $y=0$ rotated around the x-axis using cylindrical shells

For exercises 9 - 14, find

a. the area of the region,

b.the volume of the solid when rotated around the $x$-axis, and

c. the volume of the solid when rotated around the $y$-axis. Use whichever method seems most appropriate to you.

9) $y=x^3,x=0,y=0$, and $x=2$

Answer:: a. $A = 4$ units²
b. $V = \frac{128π}{7}$ units³
c. $V = \frac{64π}{5}$ units³

10) $y=x^2−x$ and $x=0$

11) [T] $y=\ln(x)+2$ and $y=x$

Answer:: a. $A \approx 1.949$ units²
b. $V \approx 21.952$ units³
c. $V = \approx 17.099$ units³

12) $y=x^2$ and $y=\sqrt{x}$

13) $y=5+x, y=x^2, x=0$, and $x=1$

Answer:: a. $A = \frac{31}{6}$ units²
b. $V = \frac{452π}{15}$ units³
c. $V = \frac{31π}{6}$ units³

14) Below $x^2+y^2=1$ and above $y=1−x$

15) Find the mass of $ρ=e^{−x}$ on a disk centered at the origin with radius $4$.

Answer:: $m \approx 245.282$

16) Find the center of mass for $ρ=\tan^2x$ on $x∈(−\frac{π}{4},\frac{π}{4})$.

17) Find the mass and the center of mass of $ρ=1$ on the region bounded by $y=x^5$ and $y=\sqrt{x}$.

Answer:: Mass: $\frac{1}{2},$
Center of mass: $(\frac{18}{35},\frac{9}{11})$

For exercises 18 - 19, find the requested arc lengths.

18) The length of $x$ for $y=\cosh(x)$ from $x=0$ to $x=2$.

19) The length of $y$ for $x=3−\sqrt{y}$ from $y=0$ to $y=4$

Answer:: $s = \big[\sqrt{17}+\frac{1}{8}\ln(33+8\sqrt{17})\big]$ units

For exercises 20 - 21, find the surface area and volume when the given curves are revolved around the specified axis.

20) The shape created by revolving the region between $y=4+x, \;y=3−x, \;x=0,$ and $x=2$ rotated around the $y$-axis.

21) The loudspeaker created by revolving $y=1/x$ from $x=1$ to $x=4$ around the $x$-axis.

Answer:: Volume: $V = \frac{3π}{4}$ units³
Surface area: $A = π\left(\sqrt{2}−\sinh^{−1}(1)+\sinh^{−1}(16)−\frac{\sqrt{257}}{16}\right)$ units²

For exercise 22, consider the Karun-3 dam in Iran. Its shape can be approximated as an isosceles triangle with height $205$ m and width $388$ m. Assume the current depth of the water is $180$ m. The density of water is $1000$ kg/m³.

22) Find the total force on the wall of the dam.

23) You are a crime scene investigator attempting to determine the time of death of a victim. It is noon and $45$ °F outside and the temperature of the body is $78$ °F. You know the cooling constant is $k=0.00824$ °F/min. When did the victim die, assuming that a human’s temperature is $98$ °F?

Answer:: 11:02 a.m.

For the following exercise, consider the stock market crash in 1929 in the United States. The table lists the Dow Jones industrial average per year leading up to the crash.

Year after 1920	Value ($)
1	63.90
3	100
5	110
7	160
9	381.17

Source: http:/stockcharts.com/freecharts/hi...a19201940.html

24) [T] The best-fit exponential curve to these data is given by $y=40.71+1.224^x$. Why do you think the gains of the market were unsustainable? Use first and second derivatives to help justify your answer. What would this model predict the Dow Jones industrial average to be in 2014 ?

For exercises 25 - 26, consider the catenoid, the only solid of revolution that has a minimal surface, or zero mean curvature. A catenoid in nature can be found when stretching soap between two rings.

25) Find the volume of the catenoid $y=\cosh(x)$ from $x=−1$ to $x=1$ that is created by rotating this curve around the $x$-axis, as shown here.

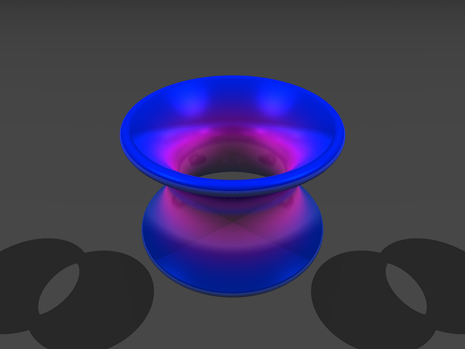
$This figure is an image of a catenoid. It has been formed by rotating a catenary curve about a vertical axis.$

Answer:: $V = π\big(1+\sinh(1)\cosh(1)\big)$ units³

26) Find surface area of the catenoid $y=\cosh(x)$ from $x=−1$ to $x=1$ that is created by rotating this curve around the $x$-axis.

Contributors and Attributions

Gilbert Strang (MIT) and Edwin “Jed” Herman (Harvey Mudd) with many contributing authors. This content by OpenStax is licensed with a CC-BY-SA-NC 4.0 license. Download for free at http://cnx.org.

Search

Text Color

Text Size

Margin Size

Font Type

Contributors