1.8: Surfaces

Last updated

Oct 27, 2024
Save as PDF
- 1.7: Tangent Planes and Normal Lines
- 1.9: Partial Derivatives

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Cylinders

Let C be a curve, then we define a cylinder to be the set of all lines through C and perpendicular to the plane that C lies in.

$alt$

We can tell that an equation is a cylinder is it is missing one of the variables.

Quadric Surfaces

Recall that the quadrics or conics are lines , hyperbolas, parabolas, circles, and ellipses. In three dimensions, we can combine any two of these and make a quadric surface. For example

$alt$

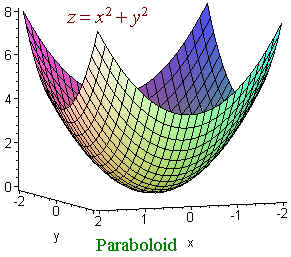
is a paraboloid since for constant z we get a circle and for constant x or y we get a parabola. We use the suffix -oid to mean ellipse or circle. We have:

$\begin{align*} \dfrac{x^2}{a^2} + \dfrac{y^2}{b^2 } + \dfrac{z^2}{c^2} &=1 \;\;\; \text{is an ellipsoid,} \\ -\dfrac{x^2}{a^2} - \dfrac{y^2}{b^2 } + \dfrac{z^2}{c^2} &=1 \;\;\; \text{is a hyperboloid of 2 sheets, while} \\ \dfrac{x^2}{a^2} + \dfrac{y^2}{b^2 } - \dfrac{z^2}{c^2} &=1 \;\;\; \text{is a hyperboloid of 1 sheet.} \end{align*} \nonumber$

Surface of Revolution

Let $y = f(x)$ be a curve, then the equation of the surface of revolution abut the x-axis is

$y^2 + z^2 = f^2(x). \nonumber$

$alt$

Example $\PageIndex{1}$

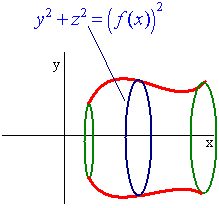
Find the equation of the surface that is formed when the curve

$y = \sin x\nonumber$

with

$0 \le x < \dfrac{\pi}{2} \nonumber$

is revolved around the y-axis.

Solution

This uses a different formula since this time the curve is revolved around the y-axis.

$alt$

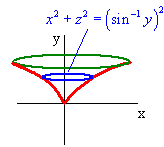
The circular cross section has radius $\sin^{-1}y$ and the circle is perpendicular to the y-axis. Hence the equation is

$x^2 + z^2 = (\sin^{-1} y)^2. \nonumber$

Cylindrical Coordinates

We can extend polar coordinates to three dimensions by

$x = r\, \cos\, q, \nonumber$

$y = r\, \sin\, q,\nonumber$

$z = z.\nonumber$

Example $\PageIndex{2}$

We can write $(1,1,3)$ in cylindrical coordinates. We find

$r = \sqrt{1^2 + 1^2} = sqrt{2} \nonumber$

and

$\theta = \tan^{-1} \left(\dfrac{1}{1} \right) = \dfrac{\pi}{4} \nonumber$

so that the cylindrical coordinates are

$( \sqrt{2}, \frac{\pi}{4},3).\nonumber$

Spherical Coordinates

An alternate coordinate system works on a distance and two angle method called spherical coordinates. We let $r$ denote the distance from the point to the origin, $\theta$ represent the same $\theta$ as in cylindrical coordinates, and $\phi$ denote the angle from the positive z-axis to the point.

$alt$

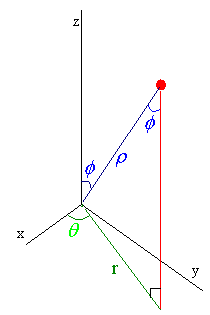
The picture tells us that

$r = r \sin \phi\nonumber$

and that

$z = r \cos \phi .\nonumber$

From this we can find

$x = r\cos \theta = r \sin \phi \cos \theta$ ,
$y = r \sin \theta = r \sin \phi \sin \theta$ ,
$z = r \cos \phi$ .

Immediately we see that

$x^2 + y^2 + z^2 = r^2. \nonumber$

We use spherical coordinates whenever the problem involves a distance from a source.

Example $\PageIndex{3}$

Convert the surface

$z = x^2 + y^2 \nonumber$

to an equation in spherical coordinates.

Solution

We add $z^2$ to both sides

$z + z^2 = x^2 + y^2 + z^2. \nonumber$

Now it is easier to convert

$r\, \cos \phi + r^2 \, \cos^2 \phi = r^2. \nonumber$

Divide by $r$ to get

$\cos \phi + r\, \cos^2 \phi = r .\nonumber$

Now solve for $r$ .

$r = \dfrac{\cos \phi }{1 - \cos^2 \phi } = \dfrac{\cos \phi }{\sin^2 \phi } = \csc \phi \; \cot \phi .\nonumber$

Search

Text Color

Text Size

Margin Size

Font Type

Example $\PageIndex{1}$

Solution

Example $\PageIndex{2}$

Example $\PageIndex{3}$

Solution

Cylinders

Quadric Surfaces

Surface of Revolution

Example 1.8.1\PageIndex{1}

Solution

Cylindrical Coordinates

Example 1.8.2\PageIndex{2}

Spherical Coordinates

Example 1.8.3\PageIndex{3}

Solution

Support Center

How can we help?

Example $\PageIndex{1}$

Example $\PageIndex{2}$

Example $\PageIndex{3}$