3.E: Multiple Integrals (Exercises)
( \newcommand{\kernel}{\mathrm{null}\,}\)
3.1: Double Integrals
A
For Exercises 1-4, find the volume under the surface z = f (x, y) over the rectangle R.
3.1.1. f (x, y) = 4x y,\, R = [0,1]×[0,1]
3.1.2. f (x, y) = e^{ x+y} ,\, R = [0,1]×[−1,1]
3.1.3. f (x, y) = x^ 3 + y^ 2 ,\, R = [0,1]×[0,1]
3.1.4. f (x, y) = x^ 4 + x y+ y^ 3 ,\, R = [1,2]×[0,2]
For Exercises 5-12, evaluate the given double integral.
3.1.5. \int_0^1 \int_1^2 (1− y)x^ 2 \,dx \,d y
3.1.6. \int_0^1 \int_0^2 x(x+ y)\,dx \,d y
3.1.7. \int_0^2 \int_0^1 (x+2)\,dx \,d y
3.1.8. \int_{−1}^2 \int_{−1}^1 x(x y+\sin x)\,dx\, d y
3.1.9. \int_0^{\pi /2} \int_0^1 x y\cos (x^ 2 y)\,dx\, d y
3.1.10. \int_0^{\pi} \int_0^{π/2} \sin x \cos (y−π) \,dx\, d y
3.1.11. \int_0^2 \int_1^4 x y \,dx\, d y
3.1.12. \int_{-1}^1 \int_{-1}^2 1\,dx\, d y
3.1.13. Let M be a constant. Show that \int_c^d \int_a^b M\, dx \,d y = M(d − c)(b − a).
3.2: Double Integrals Over a General Region
A
For Exercises 1-6, evaluate the given double integral.
3.2.1. \int_0^1 \int_{\sqrt{ x}}^1 24x^ 2 y \,d y\, dx
3.2.2. \int_0^π \int_0^y \sin x \,dx\, d y
3.2.3. \int_1^2 \int_0^{\ln x} 4x \,d y\, dx
3.2.4. \int_0^2 \int_0^{2y} e^ {y^ 2} \,dx \,d y
3.2.5. \int_0^{π/2} \int_0^y \cos x \sin y \,dx \,d y
3.2.6. \int_0^{∞} \int_0^{∞} x ye^{−(x^ 2+y^ 2 )}\, dx \,d y
3.2.7. \int_0^2 \int_0^y 1\,dx \,d y
3.2.8. \int_0^1 \int_0^{x^ 2} 2\,d y\, dx
3.2.9. Find the volume V of the solid bounded by the three coordinate planes and the plane x+ y+ z = 1.
3.2.10. Find the volume V of the solid bounded by the three coordinate planes and the plane 3x+2y+5z = 6.
B
3.2.11. Explain why the double integral \iint\limits_R 1\,d A gives the area of the region R. For simplicity, you can assume that R is a region of the type shown in Figure 3.2.1(a).
C
3.2.12. Prove that the volume of a tetrahedron with mutually perpendicular adjacent sides of lengths a,\, b, \text{ and }c, as in Figure 3.2.6, is \frac{abc}{ 6}. (Hint: Mimic Example 3.5, and recall from Section 1.5 how three noncollinear points determine a plane.)
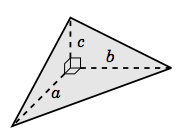
Figure 3.2.6
3.2.13. Show how Exercise 12 can be used to solve Exercise 10.
3.3: Triple Integrals
A
For Exercises 1-8, evaluate the given triple integral.
3.3.1. \int_0^3 \int_0^2 \int_0^1 x yz \,dx\, d y\, dz
3.3.2. \int_0^1 \int_0^x \int_0^y x yz \,dz\, d y\, dx
3.3.3. \int_0^π \int_0^x \int_0^{x y} x^ 2 \sin z \,dz\, d y\, dx
3.3.4. \int_0^1 \int_0^z \int_0^y ze^{ y^ 2} \,dx\, d y\, dz
3.3.5. \int_1^e \int_0^y \int_0^{1/y} x^ 2 z \,dx \,dz \,d y
3.3.6. \int_1^2 \int_0^{y^ 2} \int_0^{z^ 2} yz \,dx \,dz \,d y
3.3.7. \int_1^2 \int_2^4 \int_0^3 1\,dx \,d y\, dz
3.3.8. \int_0^1 \int_0^{1−x} \int_0^{1−x−y} 1\,dz\, d y\, dx
3.3.9. Let M be a constant. Show that \int_{z_1}^{z_2} \int_{y_1}^{y_2} \int_{x_1}^{x_2} M\, dx\, d y\, dz = M(z_2 − z_1)(y_2 − y_1)(x_2 − x_1).
B
3.3.10. Find the volume V of the solid S bounded by the three coordinate planes, bounded above by the plane x+ y+ z = 2, and bounded below by the plane z = x+ y.
C
3.3.11. Show that \int_a^b \int_a^z \int_a^y f (x)\,dx \,d y \,dz = \int_a^b \frac{(b−x)^ 2}{ 2} f (x)\,dx. (Hint: Think of how changing the order of integration in the triple integral changes the limits of integration.)
3.4: Numerical Approximation of Multiple Integrals
C
3.4.1. Write a program that uses the Monte Carlo method to approximate the double integral \iint\limits_R e^{ x y}\, d A, where R = [0,1]×[0,1]. Show the program output for N = 10,\, 100,\, 1000,\, 10000,\, 100000 \text{ and }1000000 random points.
3.4.2. Write a program that uses the Monte Carlo method to approximate the triple integral \iiint\limits_S e^{ x yz}\, dV\), where S = [0,1] × [0,1] × [0,1]. Show the program output for N = 10,\, 100,\, 1000,\, 10000,\, 100000 \text{ and }1000000 random points.
3.4.3. Repeat Exercise 1 with the region R = {(x, y) : −1 ≤ x ≤ 1,\, 0 ≤ y ≤ x^ 2 }.
3.4.4. Repeat Exercise 2 with the solid S = {(x, y, z) : 0 ≤ x ≤ 1,\, 0 ≤ y ≤ 1, \,0 ≤ z ≤ 1− x− y}.
3.4.5. Use the Monte Carlo method to approximate the volume of a sphere of radius 1.
3.4.6. Use the Monte Carlo method to approximate the volume of the ellipsoid \frac{x^ 2}{ 9} + \frac{y^ 2}{ 4} + \frac{z^ 2}{ 1} = 1.
3.5: Change of Variables in Multiple Integrals
A
3.5.1. Find the volume V inside the paraboloid z = x^ 2 + y^ 2 \text{ for }0 ≤ z ≤ 4.
3.5.2. Find the volume V inside the cone z = \sqrt{ x^ 2 + y^ 2} for 0 ≤ z ≤ 3.
B
3.5.3. Find the volume V of the solid inside both x^ 2 + y^ 2 + z^ 2 = 4 and x^ 2 + y^ 2 = 1.
3.5.4. Find the volume V inside both the sphere x^ 2 + y^ 2 + z^ 2 = 1 and the cone z = \sqrt{ x^ 2 + y^ 2}.
3.5.5. Prove Equation (3.25).
3.5.6. Prove Equation (3.26).
3.5.7. Evaluate \iiint\limits_R \sin \left ( \frac{x+y}{ 2} \right ) \cos \left ( \frac{x−y}{ 2} \right ) \,d A, where R is the triangle with vertices (0,0),\, (2,0) \text{ and }(1,1). (Hint: Use the change of variables u = (x+ y)/2,\, v = (x− y)/2.)
3.5.8. Find the volume of the solid bounded by z = x^ 2 + y^ 2 \text{ and }z^ 2 = 4(x^ 2 + y^ 2 ).
3.5.9. Find the volume inside the elliptic cylinder \frac{x^ 2}{ a^ 2} + \frac{y^ 2}{ b^ 2} = 1 \text{ for } 0 ≤ z ≤ 2.
C
3.5.10. Show that the volume inside the ellipsoid \frac{x^ 2}{ a^ 2} + \frac{y^ 2}{ b^ 2} + \frac{z^ 2}{ c^ 2} = 1 \text{ is }\frac{4πabc}{ 3}. (Hint: Use the change of variables x = au,\, y = bv,\, z = cw, then consider Example 3.12.)
3.5.11. Show that the Beta function, defined by
B(x, y) = \int_0^1 t^{ x−1} (1− t)^{ y−1} dt ,\text{ for }x > 0,\, y > 0,
satisfies the relation B(y, x) = B(x, y) \text{ for }x > 0,\, y > 0.
3.5.12. Using the substitution t = u/(u +1), show that the Beta function can be written as
B(x, y) = \int_0^∞ \frac{u^{ x−1}}{ (u +1)^{x+y}}\, du ,\text{ for }x > 0,\, y > 0.
3.6: Application: Center of Mass
A
For Exercises 1-5, find the center of mass of the region R with the given density function δ(x, y).
3.6.1. R = {(x, y) : 0 ≤ x ≤ 2,\, 0 ≤ y ≤ 4 },\, δ(x, y) = 2y
3.6.2. R = {(x, y) : 0 ≤ x ≤ 1,\, 0 ≤ y ≤ x 2 },\, δ(x, y) = x+ y
3.6.3. R = {(x, y) : y ≥ 0,\, x^ 2 + y^ 2 ≤ a^ 2 },\, δ(x, y) = 1
3.6.4. R = {(x, y) : y ≥ 0,\, x ≥ 0,\, 1 ≤ x^ 2 + y^ 2 ≤ 4 },\, δ(x, y) = \sqrt{ x^ 2 + y^ 2}
3.6.5. R = {(x, y) : y ≥ 0,\, x^ 2 + y^ 2 ≤ 1 },\, δ(x, y) = y
B
For Exercises 6-10, find the center of mass of the solid S with the given density function δ(x, y, z).
3.6.6. S = {(x, y, z) : 0 ≤ x ≤ 1,\, 0 ≤ y ≤ 1,\, 0 ≤ z ≤ 1 },\, δ(x, y, z) = x yz
3.6.7. S = {(x, y, z) : z ≥ 0,\, x^ 2 + y^ 2 + z^ 2 ≤ a^ 2 },\, δ(x, y, z) = x^ 2 + y^ 2 + z^ 2
3.6.8. S = {(x, y, z) : x ≥ 0,\, y ≥ 0,\, z ≥ 0,\, x^ 2 + y^ 2 + z^ 2 ≤ a^ 2 },\, δ(x, y, z) = 1
3.6.9. S = {(x, y, z) : 0 ≤ x ≤ 1,\, 0 ≤ y ≤ 1,\, 0 ≤ z ≤ 1 },\, δ(x, y, z) = x^ 2 + y^ 2 + z^ 2
3.6.10. S = {(x, y, z) : 0 ≤ x ≤ 1,\, 0 ≤ y ≤ 1,\, 0 ≤ z ≤ 1− x− y},\, δ(x, y, z) = 1
3.7: Application: Probability and Expected Value
B
3.7.1. Evaluate the integral \int_{−\infty}^{\infty} e^{ −x^ 2}\, dx using anything you have learned so far.
3.7.2. For σ > 0 \text{ and }µ > 0, evaluate \int_{\infty}^{−\infty} \frac{1}{ σ \sqrt{ 2π}} e^{ −(x−µ)^ 2 /2σ^ 2} dx.
3.7.3. Show that EY = \frac{n}{ n+1} in Example 3.18
C
3.7.4. Write a computer program (in the language of your choice) that verifies the results in Example 3.18 for the case n = 3 by taking large numbers of samples.
3.7.5. Repeat Exercise 4 for the case when n = 4.
3.7.6. For continuous random variables X, Y \text{ with joint p.d.f. }f (x, y), define the second moments E(X^ 2 ) \text{ and }E(Y^ 2 ) by
E(X^ 2 ) = \int_{-\infty}^{\infty} \int_{-\infty}^{\infty} x^ 2 f (x, y)\,dx\, d y \text{ and }E(Y^ 2 ) = \int_{-\infty}^{\infty} \int_{-\infty}^{\infty} y^ 2 f (x, y)\,dx \,d y ,
and the variances Var(X) and Var(Y) by
\text{Var}(X) = E(X^ 2 )−(EX)^ 2 \text{ and Var}(Y) = E(Y^ 2 )−(EY)^ 2 .
Find Var(X) and Var(Y) for X and Y as in Example 3.18.
3.7.7. Continuing Exercise 6, the correlation ρ \text{ between }X \text{ and }Y is defined as
ρ = \frac{E(XY)−(EX)(EY)}{ \sqrt{ \text{Var}(X)\text{Var}(Y)}} ,
where E(XY) = \int_{-\infty}^{\infty} \int_{-\infty}^{\infty} x y \,f (x, y)\,dx\, d y. Find ρ for X \text{ and }Y as in Example 3.18.
(Note: The quantity E(XY)−(EX)(EY) is called the covariance of X and Y.)
3.7.8. In Example 3.17 would the answer change if the interval (0,100) is used instead of (0,1)? Explain.



