2.3: Quadratic Functions

You may recall studying quadratic equations in Intermediate Algebra. In this section, we review those equations in the context of our next family of functions: the quadratic functions.

Definition 2.5.

A quadratic function is a function of the form

\(f(x) = ax^2 + bx + c,\)

where \(a\), \(b\) and \(c\) are real numbers with \(a \neq 0\). The domain of a quadratic function is \((-\infty, \infty)\).

The most basic quadratic function is \(f(x) = x^2\), whose graph appears below. Its shape should look familiar from Intermediate Algebra – it is called a parabola. The point \((0,0)\) is called the vertex of the parabola. In this case, the vertex is a relative minimum and is also the where the absolute minimum value of \(f\) can be found.

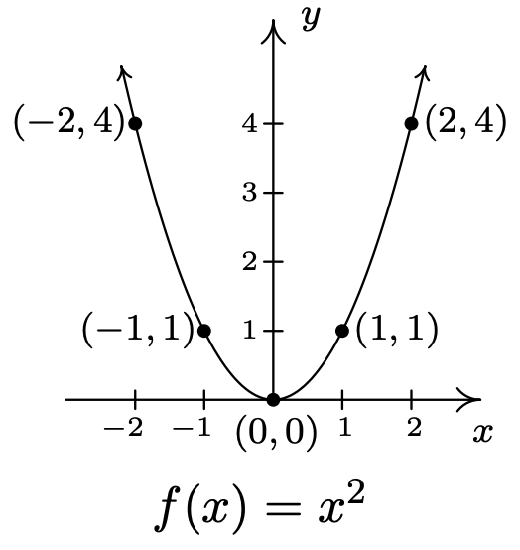
Much like many of the absolute value functions in Section 2.2, knowing the graph of \(f(x) = x^2\) enables us to graph an entire family of quadratic functions using transformations.

Example 2.3.1

Graph the following functions starting with the graph of \(f(x) = x^2\) and using transformations. Find the vertex, state the range and find the \(x\)- and \(y\)-intercepts, if any exist.

1. \(g(x) = (x+2)^2 - 3\)
2. \(h(x) = -2(x-3)^2+1\)

Solution.

1. Since \(g(x) = (x+2)^2 - 3 = f(x+2) - 3\), Theorem 1.7 instructs us to first subtract \(2\) from each of the \(x\)-values of the points on \(y=f(x)\). This shifts the graph of \(y = f(x)\) to the left \(2\) units and moves \((-2,4)\) to \((-4,4)\), \((-1,1)\) to \((-3,1)\), \((0,0)\) to \((-2,0)\), \((1,1)\) to \((-1,1)\) and \((2,4)\) to \((0,4)\). Next, we subtract \(3\) from each of the \(y\)-values of these new points. This moves the graph down \(3\) units and moves \((-4,4)\) to \((-4,1)\), \((-3,1)\) to \((-3,-2)\), \((-2,0)\) to \((-2,3)\), \((-1,1)\) to \((-1,-2)\) and \((0,4)\) to \((0,1)\). We connect the dots in parabolic fashion to get

   

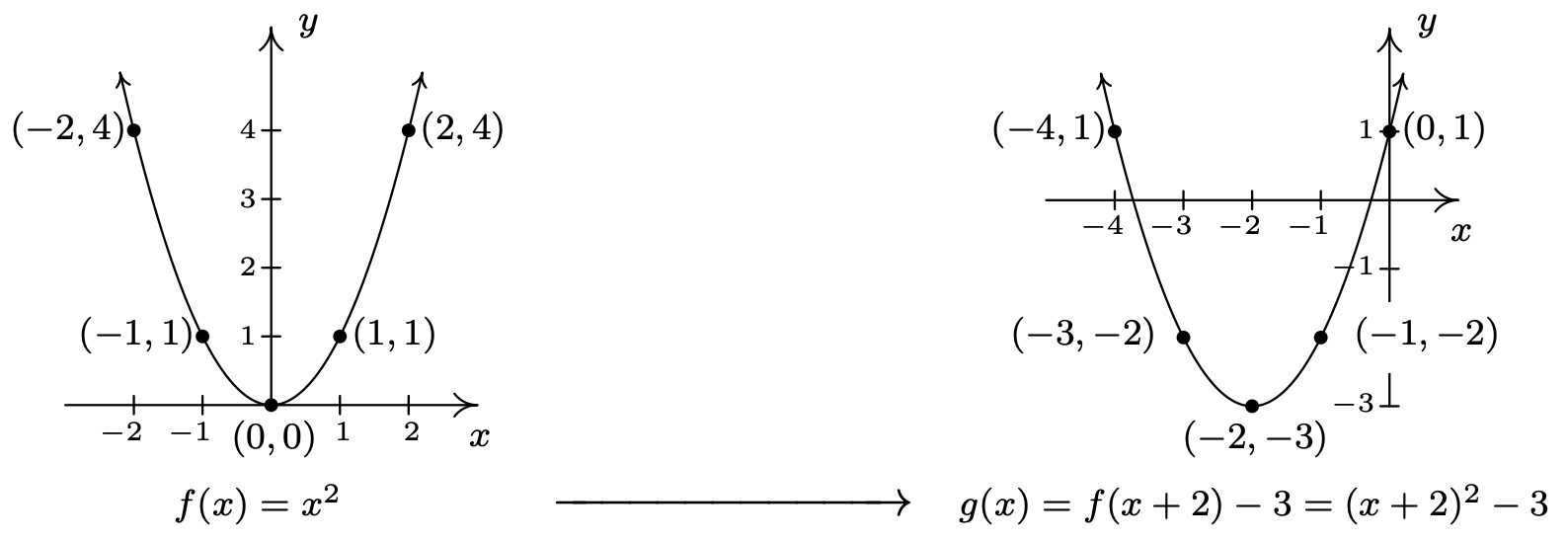
   From the graph, we see that the vertex has moved from \((0,0)\) on the graph of \(y = f(x)\) to \((-2,-3)\) on the graph of \(y = g(x)\). This sets \([-3, \infty)\) as the range of \(g\). We see that the graph of \(y=g(x)\) crosses the \(x\)-axis twice, so we expect two \(x\)-intercepts. To find these, we set \(y = g(x) = 0\) and solve. Doing so yields the equation \((x+2)^2 - 3 = 0\), or \((x+2)^2 = 3\). Extracting square roots gives \(x + 2 = \pm \sqrt{3}\), or \(x = -2 \pm \sqrt{3}\). Our \(x\)-intercepts are \((-2-\sqrt{3}, 0) \approx (-3.73, 0)\) and \((-2+\sqrt{3}, 0) \approx (-0.27, 0)\). The \(y\)-intercept of the graph, \((0,1)\) was one of the points we originally plotted, so we are done.

2. Following Theorem 1.7 once more, to graph \(h(x) = -2(x-3)^2+1 = -2f(x-3)+1\), we first start by adding \(3\) to each of the \(x\)-values of the points on the graph of \(y=f(x)\). This effects a horizontal shift right \(3\) units and moves \((-2,4)\) to \((1,4)\), \((-1,1)\) to \((2,1)\), \((0,0)\) to \((3,0)\), \((1,1)\) to \((4,1)\) and \((2,4)\) to \((5,4)\). Next, we multiply each of our \(y\)-values first by \(-2\) and then add \(1\) to that result. Geometrically, this is a vertical stretch by a factor of \(2\), followed by a reflection about the \(x\)-axis, followed by a vertical shift up \(1\) unit. This moves \((1,4)\) to \((1,-7)\), \((2,1)\) to \((2,-1)\), \((3,0)\) to \((3,1)\), \((4,1)\) to \((4,-1)\) and \((5,4)\) to \((5,-7)\).

   

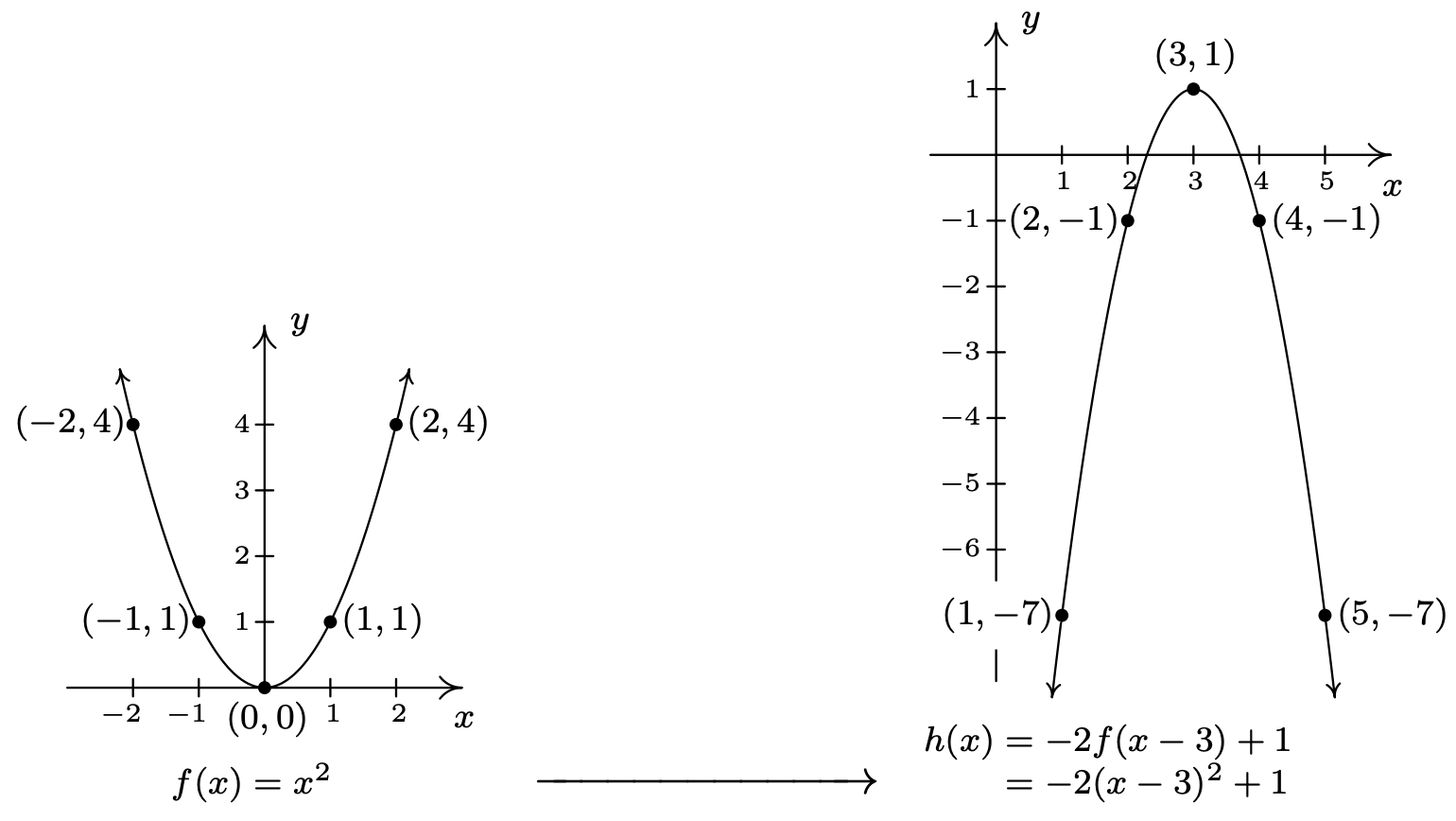
   The vertex is \((3,1)\) which makes the range of \(h\) \((-\infty, 1]\). From our graph, we know that there are two \(x\)-intercepts, so we set \(y = h(x) = 0\) and solve. We get \(-2(x-3)^2+1 = 0\) which gives \((x-3)^2 = \frac{1}{2}\). Extracting square roots¹ gives \(x - 3 = \pm \frac{\sqrt{2}}{2}\), so that when we add \(3\) to each side,² we get \(x = \frac{6 \pm \sqrt{2}}{2}\). Hence, our \(x\)-intercepts are \(\left(\frac{6 - \sqrt{2}}{2}, 0 \right) \approx (2.29, 0)\) and \(\left(\frac{6 + \sqrt{2}}{2}, 0 \right) \approx (3.71, 0)\). Although our graph doesn’t show it, there is a \(y\)-intercept which can be found by setting \(x=0\). With \(h(0) = -2(0-3)^2+1 = -17\), we have that our \(y\)-intercept is \((0,-17)\).

A few remarks about Example 2.3.1 are in order. First note that neither the formula given for \(g(x)\) nor the one given for \(h(x)\) match the form given in Definition 2.5. We could, of course, convert both \(g(x)\) and \(h(x)\) into that form by expanding and collecting like terms. Doing so, we find \(g(x) = (x+2)^2 - 3 = x^2 + 4x+1\) and \(h(x) = -2(x-3)^2+1 = -2x^2+12x-17\). While these ‘simplified’ formulas for \(g(x)\) and \(h(x)\) satisfy Definition 2.5, they do not lend themselves to graphing easily. For that reason, the form of \(g\) and \(h\) presented in Example 2.3.2 is given a special name, which we list below, along with the form presented in Definition 2.5.

Definition 2.6

Standard and General Form of Quadratic Functions: Suppose \(f\) is a quadratic function.

• The general form of the quadratic function \(f\) is \(f(x) = ax^2+bx+c\), where \(a\), \(b\) and \(c\) are real numbers with \(a \neq 0\).
• The standard form of the quadratic function \(f\) is \(f(x) = a(x-h)^2 + k\), where \(a\), \(h\) and \(k\) are real numbers with \(a\neq 0\).

It is important to note at this stage that we have no guarantees that every quadratic function can be written in standard form. This is actually true, and we prove this later in the exposition, but for now we celebrate the advantages of the standard form, starting with the following theorem.

Theorem 2.2

Vertex Formula for Quadratics in Standard Form: For the quadratic function \(f(x) = a(x-h)^2 + k\), where \(a\), \(h\) and \(k\) are real numbers with \(a\neq 0\), the vertex of the graph of \(y = f(x)\) is \((h,k)\).

We can readily verify the formula given Theorem 2.2 with the two functions given in Example 2.3.1. After a (slight) rewrite, \(g(x) = (x+2)^2 - 3 = (x-(-2))^2+(-3)\), and we identify \(h=-2\) and \(k=-3\). Sure enough, we found the vertex of the graph of \(y=g(x)\) to be \((-2,-3)\). For \(h(x) = -2(x-3)^{2}+1\), no rewrite is needed. We can directly identify \(h=3\) and \(k=1\) and, sure enough, we found the vertex of the graph of \(y=h(x)\) to be \((3,1)\).

To see why the formula in Theorem 2.2 produces the vertex, consider the graph of the equation \(y = a(x-h)^2 + k\). When we substitute \(x=h\), we get \(y=k\), so \((h,k)\) is on the graph. If \(x \neq h\), then \(x-h \neq 0\) so \((x-h)^2\) is a positive number. If \(a>0\), then \(a(x-h)^2\) is positive, thus \(y = a(x-h)^2 + k\) is always a number larger than \(k\). This means that when \(a>0\), \((h,k)\) is the lowest point on the graph and thus the parabola must open upwards, making \((h,k)\) the vertex. A similar argument shows that if \(a<0\), \((h,k)\) is the highest point on the graph, so the parabola opens downwards, and \((h,k)\) is also the vertex in this case.

Alternatively, we can apply the machinery in Section 1.7. Since the vertex of \(y = x^2\) is \((0,0)\), we can determine the vertex of \(y = a(x-h)^2+k\) by determining the final destination of \((0,0)\) as it is moved through each transformation. To obtain the formula \(f(x)= a(x-h)^2+k\), we start with \(g(x)=x^2\) and first define \(g_{1}(x) = a g(x) = ax^2\). This is results in a vertical scaling and/or reflection.³ Since we multiply the output by \(a\), we multiply the \(y\)-coordinates on the graph of \(g\) by \(a\), so the point \((0,0)\) remains \((0,0)\) and remains the vertex. Next, we define \(g_{2}(x) = g_{1}(x-h) = a(x-h)^2\). This induces a horizontal shift right or left \(h\) units⁴ moves the vertex, in either case, to \((h,0)\). Finally, \(f(x) = g_{2}(x)+k = a(x-h)^2+k\) which effects a vertical shift up or down \(k\) units⁵ resulting in the vertex moving from \((h,0)\) to \((h,k)\).

In addition to verifying Theorem 2.2, the arguments in the two preceding paragraphs have also shown us the role of the number \(a\) in the graphs of quadratic functions. The graph of \(y = a(x-h)^2 + k\) is a parabola ‘opening upwards’ if \(a > 0\), and ‘opening downwards’ if \(a < 0\). Moreover, the symmetry enjoyed by the graph of \(y = x^2\) about the \(y\)-axis is translated to a symmetry about the vertical line \(x=h\) which is the vertical line through the vertex.⁶ This line is called the axis of symmetry of the parabola and is dashed in the figures below.

Graphs of \(y=a(x-h)^2+k\).

Without a doubt, the standard form of a quadratic function, coupled with the machinery in Section 1.7, allows us to list the attributes of the graphs of such functions quickly and elegantly. What remains to be shown, however, is the fact that every quadratic function can be written in standard form. To convert a quadratic function given in general form into standard form, we employ the ancient rite of ‘Completing the Square’. We remind the reader how this is done in our next example.

Example 2.3.2

Convert the functions below from general form to standard form. Find the vertex, axis of symmetry and any \(x\)- or \(y\)-intercepts. Graph each function and determine its range.

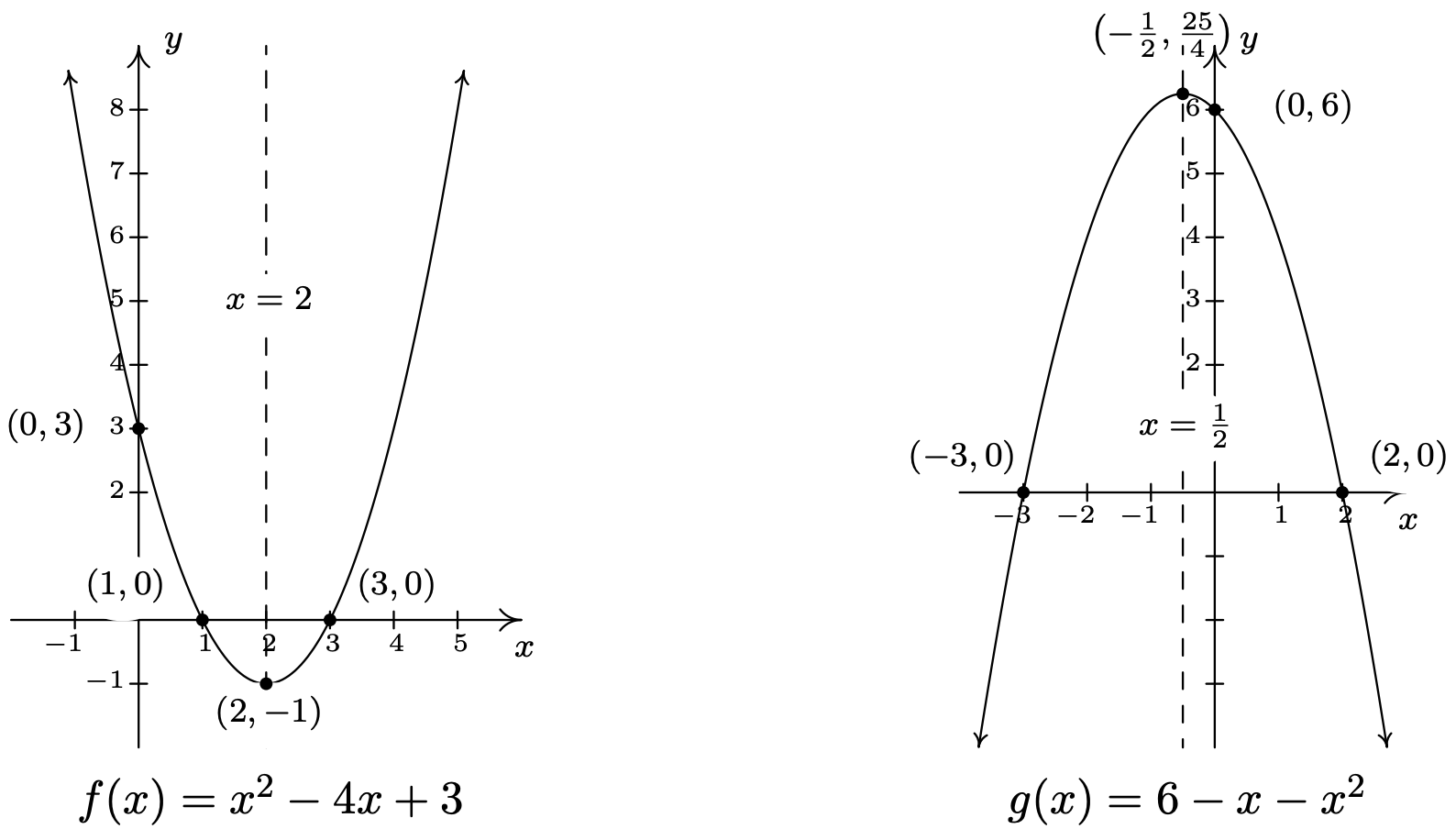
1. \(f(x) = x^2-4x+3\).
2. \(g(x) = 6-x-x^2\)

Solution.

1. To convert from general form to standard form, we complete the square.⁷ First, we verify that the coefficient of \(x^2\) is \(1\). Next, we find the coefficient of \(x\), in this case \(-4\), and take half of it to get \(\frac{1}{2}(-4) = -2\). This tells us that our target perfect square quantity is \((x-2)^2\). To get an expression equivalent to \((x-2)^2\), we need to add \((-2)^2 = 4\) to the \(x^2-4x\) to create a perfect square trinomial, but to keep the balance, we must also subtract it. We collect the terms which create the perfect square and gather the remaining constant terms. Putting it all together, we get

   \(\begin{array}{rclr} f(x) & = & x^2-4x+3 & \text{(Compute $\frac{1}{2} (-4) = -2$.)} \\[4pt] & = & \left(x^2 - 4x + \underline{4} - \underline{4}\right) + 3 & \text{(Add and subtract $(-2)^2 = 4$ to $(x^2+4x)$.)}\\[4pt] & = & \left(x^2 - 4x + 4\right) - 4 + 3 & \text{(Group the perfect square trinomial.)}\\[4pt] & = & (x-2)^2 - 1 & \text{(Factor the perfect square trinomial.)} \\ \end{array}\)

   Of course, we can always check our answer by multiplying out \(f(x) = (x-2)^2 -1\) to see that it simplifies to \(f(x) = x^2 - 4x - 1\). In the form \(f(x) = (x-2)^2-1\), we readily find the vertex to be \((2,-1)\) which makes the axis of symmetry \(x = 2\). To find the \(x\)-intercepts, we set \(y = f(x) = 0\). We are spoiled for choice, since we have two formulas for \(f(x)\). Since we recognize \(f(x) = x^2-4x+3\) to be easily factorable,⁸ we proceed to solve \(x^2-4x+3 = 0\). Factoring gives \((x-3)(x-1) = 0\) so that \(x = 3\) or \(x=1\). The \(x\)-intercepts are then \((1,0)\) and \((3,0)\). To find the \(y\)-intercept, we set \(x=0\). Once again, the general form \(f(x) = x^2-4x+3\) is easiest to work with here, and we find \(y = f(0) = 3\). Hence, the \(y\)-intercept is \((0,3)\). With the vertex, axis of symmetry and the intercepts, we get a pretty good graph without the need to plot additional points. We see that the range of \(f\) is \([-1,\infty)\) and we are done.

2. To get started, we rewrite \(g(x) = 6-x-x^2 = -x^2-x+6\) and note that the coefficient of \(x^2\) is \(-1\), not \(1\). This means our first step is to factor out the \((-1)\) from both the \(x^2\) and \(x\) terms. We then follow the completing the square recipe as above. \(\begin{array}{rclr} g(x) & = & -x^2-x+6 & \\ & = & (-1)\left(x^2 + x \right) + 6 & \text{(Factor the coefficient of $x^2$ from $x^2$ and $x$.)} \\[4pt] & = & (-1)\left(x^2 + x + \underline{\frac{1}{4}} - \underline{\frac{1}{4}} \right) + 6 & \\[4pt] & = & (-1)\left(x^2 + x + \frac{1}{4}\right) + (-1)\left(-\frac{1}{4}\right) + 6 & \text{(Group the perfect square trinomial.)}\\[4pt] & = & -\left(x +\frac{1}{2}\right)^2 + \frac{25}{4} & \\ \end{array}\)

From \(\ g(x)=-\left(x+\frac{1}{2}\right)^{2}+\frac{25}{4}\), we get the vertex to be \(\ \left(-\frac{1}{2}, \frac{25}{4}\right)\) and the axis of symmetry to be \(\ x=-\frac{1}{2}\). To get the x-intercepts, we opt to set the given formula \(\ g(x)=6-x-x^{2}=0\). Solving, we get \(\ x = −3\) and \(x = 2\), so the x-intercepts are (−3, 0) and (2, 0). Setting \(x = 0\), we find g(0) = 6, so the y-intercept is (0, 6). Plotting these points gives us the graph below. We see that the range of \(\ g\) is \(\ \left(-\infty, \frac{25}{4}\right]\).

With Example 2.3.2 fresh in our minds, we are now in a position to show that every quadratic function can be written in standard form. We begin with \(f(x) = ax^2+bx+c\), assume \(a \neq 0\), and complete the square in complete generality.

\(\ \begin{aligned} f(x) &=a x^{2}+b x+c \\ &\left.=a\left(x^{2}+\frac{b}{a} x\right)+c \quad \quad \text { (Factor out coefficient of } x^{2} \text { from } x^{2} \text { and } x .\right) \\ &=a\left(x^{2}+\frac{b}{a} x+\frac{b^{2}}{\frac{4 a^{2}}{2}}-\frac{b^{2}}{4 a^{2}}\right)+c \\ &=a\left(x^{2}+\frac{b}{a} x+\frac{b^{2}}{4 a^{2}}\right)-a\left(\frac{b^{2}}{4 a^{2}}\right)+c \quad \quad \text { (Group the perfect square trinomial.) } \\ &=a\left(x+\frac{b}{2 a}\right)^{2}+\frac{4 a c-b^{2}}{4 a} \quad \text { (Factor and get a common denominator.) } \end{aligned}\)

Comparing this last expression with the standard form, we identify \((x-h)\) with \(\left(x+\frac{b}{2a}\right)\) so that \(h = -\frac{b}{2a}\). Instead of memorizing the value \(k = \frac{4ac - b^2}{4a}\), we see that \(f\left(-\frac{b}{2a}\right) = \frac{4ac - b^2}{4a}\). As such, we have derived a vertex formula for the general form. We summarize both vertex formulas in the box at the top of the next page.

Equation 2.4

Vertex Formulas for Quadratic Functions: Suppose \(a\), \(b\), \(c\), \(h\) and \(k\) are real numbers with \(a \neq 0\). [vertexofquadraticfunctions]

• If \(f(x) = a(x-h)^2 + k\), the vertex of the graph of \(y=f(x)\) is the point \((h,k)\).
• If \(f(x) = ax^2+bx+c\), the vertex of the graph of \(y=f(x)\) is the point \(\left(-\dfrac{b}{2a}, f\left(-\dfrac{b}{2a}\right)\right)\).

There are two more results which can be gleaned from the completed-square form of the general form of a quadratic function,

\[f(x) = ax^2 + bx + c = a\left(x+\dfrac{b}{2a}\right)^2 + \dfrac{4ac - b^2}{4a}\]

We have seen that the number \(a\) in the standard form of a quadratic function determines whether the parabola opens upwards (if \(a>0\)) or downwards (if \(a < 0\)). We see here that this number \(a\) is none other than the coefficient of \(x^2\) in the general form of the quadratic function. In other words, it is the coefficient of \(x^2\) alone which determines this behavior – a result that is generalized in Section 3.1. The second treasure is a re-discovery of the quadratic formula.

Equation 2.5

The Quadratic Formula: If \(a\), \(b\) and \(c\) are real numbers with \(a \neq 0\), then the solutions to \(ax^2 + bx + c = 0\) are

\(x = \dfrac{-b \pm \sqrt{b^2-4ac}}{2a}.\)

Assuming the conditions of Equation 2.5, the solutions to \(ax^2+bx+c = 0\) are precisely the zeros of \(f(x) = ax^2 + bx + c\). Since

\(f(x) = ax^2+bx+c = a\left(x+\dfrac{b}{2a}\right)^2 + \dfrac{4ac - b^2}{4a}\)

the equation \(ax^2 + bx + c = 0\) is equivalent to

\(a\left(x+\dfrac{b}{2a}\right)^2 + \dfrac{4ac - b^2}{4a} = 0.\)

Solving gives

\(\begin{array}{rclr} a\left(x+\dfrac{b}{2a}\right)^2 + \dfrac{4ac - b^2}{4a} & = & 0 & \\[15pt] a\left(x+\dfrac{b}{2a}\right)^2 & = & - \dfrac{4ac - b^2}{4a} & \\[15pt] \dfrac{1}{a} \left[a\left(x+\dfrac{b}{2a}\right)^2\right] & = & \dfrac{1}{a} \left(\dfrac{b^2-4ac}{4a}\right) & \\[15pt] \left(x+\dfrac{b}{2a}\right)^2 & = & \dfrac{b^2-4ac}{4a^2} & \\[15pt] x+\dfrac{b}{2a} & = & \pm \sqrt{\dfrac{b^2-4ac}{4a^2}} & \mbox{extract square roots} \\[15pt] x+\dfrac{b}{2a} & = & \pm \dfrac{\sqrt{b^2-4ac}}{2a} & \\[15pt] x & = & -\dfrac{b}{2a} \pm \dfrac{\sqrt{b^2-4ac}}{2a} \\[15pt] x & = & \dfrac{-b \pm \sqrt{b^2-4ac}}{2a} & \end{array}\)

In our discussions of domain, we were warned against having negative numbers underneath the square root. Given that \(\sqrt{b^{2} - 4ac}\) is part of the Quadratic Formula, we will need to pay special attention to the radicand \(b^{2} - 4ac\). It turns out that the quantity \(b^2-4ac\) plays a critical role in determining the nature of the solutions to a quadratic equation. It is given a special name.

Definition 2.7

If \(\ a\), \(\ b\) and \(\ c\) are real numbers with \(\ a \neq 0\), then the discriminant of the quadratic equation \(\ a x^{2}+b x+c=0\) is the quantity \(\ b^{2}-4 a c\).

The discriminant ‘discriminates’ between the kinds of solutions we get from a quadratic equation. These cases, and their relation to the discriminant, are summarized below.

Theorem 2.3. Discriminant Trichotomy

Let \(a\), \(b\) and \(c\) be real numbers with \(a \neq 0\).

• If \(b^2 - 4ac < 0\), the equation \(ax^2 + bx + c = 0\) has no real solutions.
• If \(b^2 - 4ac = 0\), the equation \(ax^2 + bx + c = 0\) has exactly one real solution.
• If \(b^2 - 4ac > 0\), the equation \(ax^2 + bx + c = 0\) has exactly two real solutions.

The proof of Theorem 2.3 stems from the position of the discriminant in the quadratic equation, and is left as a good mental exercise for the reader. The next example exploits the fruits of all of our labor in this section thus far.

Example 2.3.3

Recall that the profit (defined on Summary of Common Economic Functions) for a product is defined by the equation \(\mbox{Profit} = \mbox{Revenue} - \mbox{Cost}\), or \(P(x) = R(x) - C(x)\). In Example 2.1.7 the weekly revenue, in dollars, made by selling \(x\) PortaBoy Game Systems was found to be \(R(x) = -1.5x^2+250x\) with the restriction (carried over from the price-demand function) that \(0 \leq x \leq 166\). The cost, in dollars, to produce \(x\) PortaBoy Game Systems is given in Example 2.1.5 as \(C(x) = 80x + 150\) for \(x \geq 0\).

1. Determine the weekly profit function \(P(x)\).
2. Graph \(y = P(x)\). Include the \(x\)- and \(y\)-intercepts as well as the vertex and axis of symmetry.
3. Interpret the zeros of \(P\).
4. Interpret the vertex of the graph of \(y = P(x)\).
5. Recall that the weekly price-demand equation for PortaBoys is \(p(x) = -1.5x+250\), where \(p(x)\) is the price per PortaBoy, in dollars, and \(x\) is the weekly sales. What should the price per system be in order to maximize profit?

Solution.

1. To find the profit function \(P(x)\), we subtract \(P(x) = R(x) - C(x) = \left(-1.5x^2+250x\right) - \left(80x + 150\right) = -1.5x^2+170x-150.\) Since the revenue function is valid when \(0 \leq x \leq 166\), \(P\) is also restricted to these values.
2. To find the \(x\)-intercepts, we set \(P(x) = 0\) and solve \(-1.5x^2+170x-150=0\). The mere thought of trying to factor the left hand side of this equation could do serious psychological damage, so we resort to the quadratic formula, Equation 2.5. Identifying \(a = -1.5\), \(b=170\), and \(c=-150\), we obtain

   \( \begin{array}{rclr} x & = & \dfrac{-b \pm \sqrt{b^2-4ac}}{2a} & \\ & = & \dfrac{-170 \pm \sqrt{170^2 - 4(-1.5)(-150)}}{2(-1.5)} & \\ & = & \dfrac{-170 \pm \sqrt{28000}}{-3} & \\ & = & \dfrac{170 \pm 20 \sqrt{70}}{3} & \\ \end{array}\)

   We get two \(x\)-intercepts: \(\left( \frac{170 - 20 \sqrt{70}}{3},0\right)\) and \(\left( \frac{170 + 20 \sqrt{70}}{3},0\right)\). To find the \(y\)-intercept, we set \(x=0\) and find \(y=P(0)=-150\) for a \(y\)-intercept of \((0,-150)\). To find the vertex, we use the fact that \(P(x)=-1.5x^2+170x-150\) is in the general form of a quadratic function and appeal to Equation 2.4. Substituting \(a = -1.5\) and \(b=170\), we get \(x = -\frac{170}{2(-1.5)} = \frac{170}{3}\). To find the \(y\)-coordinate of the vertex, we compute \(P\left( \frac{170}{3} \right) = \frac{14000}{3}\) and find that our vertex is \(\left(\frac{170}{3}, \frac{14000}{3}\right)\). The axis of symmetry is the vertical line passing through the vertex so it is the line \(x=\frac{170}{3}\). To sketch a reasonable graph, we approximate the \(x\)-intercepts, \((0.89,0)\) and \((112.44,0)\), and the vertex, \((56.67,4666.67)\). (Note that in order to get the \(x\)-intercepts and the vertex to show up in the same picture, we had to scale the \(x\)-axis differently than the \(y\)-axis. This results in the left-hand \(x\)-intercept and the \(y\)-intercept being uncomfortably close to each other and to the origin in the picture.)

   

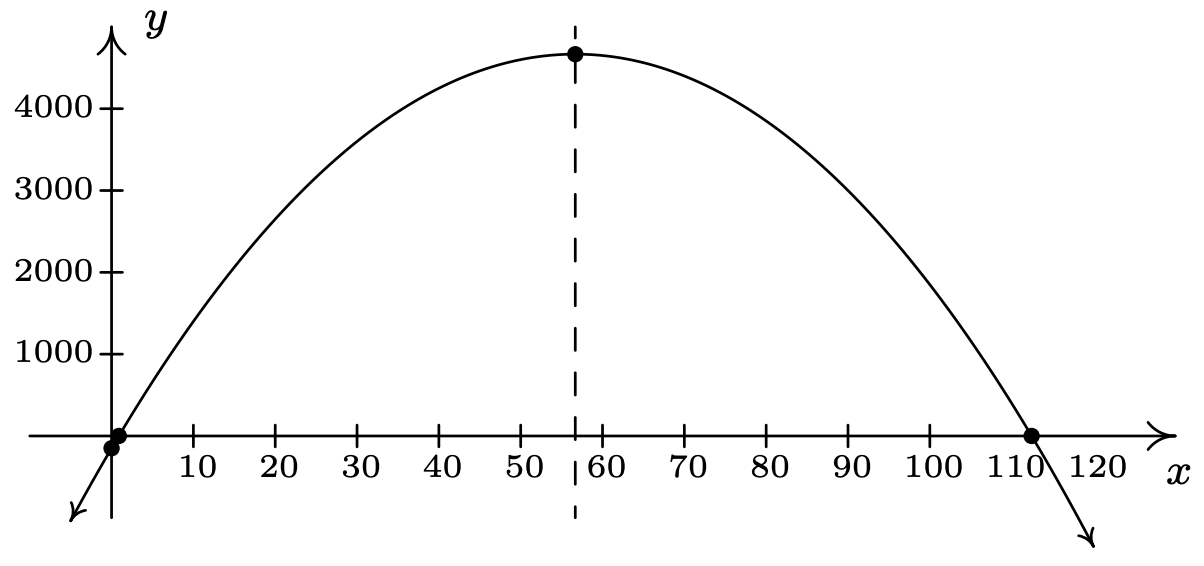
3. The zeros of \(P\) are the solutions to \(P(x)=0\), which we have found to be approximately \(0.89\) and \(112.44\). As we saw in Example 1.5.3, these are the ‘break-even’ points of the profit function, where enough product is sold to recover the cost spent to make the product. More importantly, we see from the graph that as long as \(x\) is between \(0.89\) and \(112.44\), the graph \(y=P(x)\) is above the \(x\)-axis, meaning \(y = P(x) > 0\) there. This means that for these values of \(x\), a profit is being made. Since \(x\) represents the weekly sales of PortaBoy Game Systems, we round the zeros to positive integers and have that as long as \(1\), but no more than \(112\) game systems are sold weekly, the retailer will make a profit.
4. From the graph, we see that the maximum value of \(P\) occurs at the vertex, which is approximately \((56.67,4666.67)\). As above, \(x\) represents the weekly sales of PortaBoy systems, so we can’t sell \(56.67\) game systems. Comparing \(P(56) = 4666\) and \(P(57)=4666.5\), we conclude that we will make a maximum profit of \(\$ 4666.50\) if we sell \(57\) game systems.
5. In the previous part, we found that we need to sell \(57\) PortaBoys per week to maximize profit. To find the price per PortaBoy, we substitute \(x=57\) into the price-demand function to get \(p(57) = -1.5(57)+250 = 164.5\). The price should be set at \(\$164.50\).

Our next example is another classic application of quadratic functions.

Example 2.3.4

Much to Donnie’s surprise and delight, he inherits a large parcel of land in Ashtabula County from one of his (e)strange(d) relatives. The time is finally right for him to pursue his dream of farming alpaca. He wishes to build a rectangular pasture, and estimates that he has enough money for 200 linear feet of fencing material. If he makes the pasture adjacent to a stream (so no fencing is required on that side), what are the dimensions of the pasture which maximize the area? What is the maximum area? If an average alpaca needs 25 square feet of grazing area, how many alpaca can Donnie keep in his pasture?

Solution. It is always helpful to sketch the problem situation, so we do so below.

We are tasked to find the dimensions of the pasture which would give a maximum area. We let \(w\) denote the width of the pasture and we let \(l\) denote the length of the pasture. Since the units given to us in the statement of the problem are feet, we assume \(w\) and \(l\) are measured in feet. The area of the pasture, which we’ll call \(A\), is related to \(w\) and \(l\) by the equation \(A = wl\). Since \(w\) and \(l\) are both measured in feet, \(A\) has units of \(\text{feet}^2\), or square feet. We are given the total amount of fencing available is \(200\) feet, which means \(w + l + w = 200\), or, \(l+2w = 200\). We now have two equations, \(A = wl\) and \(l+2w = 200\). In order to use the tools given to us in this section to maximize \(A\), we need to use the information given to write \(A\) as a function of just one variable, either \(w\) or \(l\). This is where we use the equation \(l+2w = 200\). Solving for \(l\), we find \(l = 200-2w\), and we substitute this into our equation for \(A\). We get \(A = wl = w(200-2w) = 200w-2w^2\). We now have \(A\) as a function of \(w\), \(A(w) = 200w-2w^2 = -2w^2+200w\).

Before we go any further, we need to find the applied domain of \(A\) so that we know what values of \(w\) make sense in this problem situation.⁹ Since \(w\) represents the width of the pasture, \(w > 0\). Likewise, \(l\) represents the length of the pasture, so \(l = 200-2w > 0\). Solving this latter inequality, we find \(w < 100\). Hence, the function we wish to maximize is \(A(w) = -2w^2 + 200w\) for \(0 < w < 100\). Since \(A\) is a quadratic function (of \(w\)), we know that the graph of \(y = A(w)\) is a parabola. Since the coefficient of \(w^2\) is \(-2\), we know that this parabola opens downwards. This means that there is a maximum value to be found, and we know it occurs at the vertex. Using the vertex formula, we find \(w = -\frac{200}{2(-2)} = 50\), and \(A(50) = -2(50)^2 + 200(50) = 5000\). Since \(w=50\) lies in the applied domain, \(0 < w < 100\), we have that the area of the pasture is maximized when the width is \(50\) feet. To find the length, we use \(l = 200-2w\) and find \(l = 200-2(50) = 100\), so the length of the pasture is \(100\) feet. The maximum area is \(A(50) = 5000\), or \(5000\) square feet. If an average alpaca requires 25 square feet of pasture, Donnie can raise \(\frac{5000}{25} = 200\) average alpaca.

We conclude this section with the graph of a more complicated absolute value function.

Example 2.3.5

Graph \(f(x) = |x^2 - x - 6|\).

Solution

Using the definition of absolute value, Definition 2.4, we have

\(f(x) = \left\{ \begin{array}{rcl} -\left(x^2 - x - 6\right), & \mbox{if} & x^2 - x - 6 < 0 \\ x^2 - x - 6, & \mbox{if} & x^2 - x - 6 \geq 0 \\ \end{array} \right.\)

The trouble is that we have yet to develop any analytic techniques to solve nonlinear inequalities such as \(x^2 - x - 6 < 0\). You won’t have to wait long; this is one of the main topics of Section 2.4. Nevertheless, we can attack this problem graphically. To that end, we graph \(y = g(x) = x^2 - x-6\) using the intercepts and the vertex. To find the \(x\)-intercepts, we solve \(x^2 - x - 6 = 0\). Factoring gives \((x-3)(x+2)=0\) so \(x=-2\) or \(x=3\). Hence, \((-2,0)\) and \((3,0)\) are \(x\)-intercepts. The \(y\)-intercept \((0,-6)\) is found by setting \(x=0\). To plot the vertex, we find \(x = -\frac{b}{2a} = -\frac{-1}{2(1)} = \frac{1}{2}\), and \(y = \left(\frac{1}{2}\right)^2 - \left(\frac{1}{2}\right)-6 = -\frac{25}{4} = -6.25\). Plotting, we get the parabola seen below on the left. To obtain points on the graph of \(y = f(x) = |x^2-x-6|\), we can take points on the graph of \(g(x) = x^2-x-6\) and apply the absolute value to each of the \(y\) values on the parabola. We see from the graph of \(g\) that for \(x \leq -2\) or \(x \geq 3\), the \(y\) values on the parabola are greater than or equal to zero (since the graph is on or above the \(x\)-axis), so the absolute value leaves these portions of the graph alone. For \(x\) between \(-2\) and \(3\), however, the \(y\) values on the parabola are negative. For example, the point \((0,-6)\) on \(y = x^2-x-6\) would result in the point \((0,|-6|) = (0,-(-6))= (0,6)\) on the graph of \(f(x) = |x^2-x-6|\). Proceeding in this manner for all points with \(x\)-coordinates between \(-2\) and \(3\) results in the graph seen below on the right.

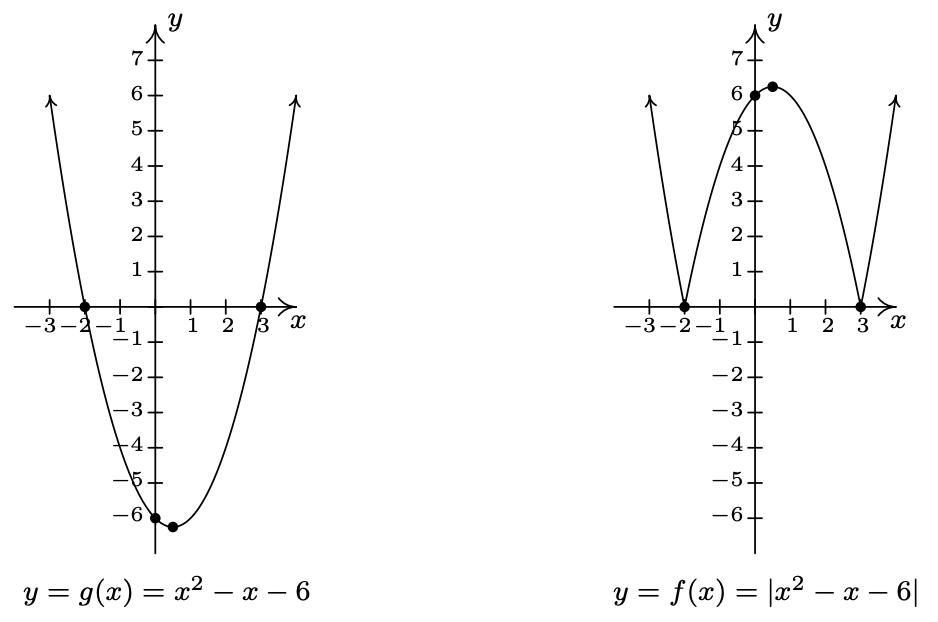
If we take a step back and look at the graphs of \(g\) and \(f\) in the last example, we notice that to obtain the graph of \(f\) from the graph of \(g\), we reflect a portion of the graph of \(g\) about the \(x\)-axis. We can see this analytically by substituting \(g(x) = x^2-x-6\) into the formula for \(f(x)\) and calling to mind Theorem 1.4 from Section 1.7.

\(f(x) = \left\{ \begin{array}{rcl} -g(x), & \mbox{if} & g(x) < 0 \\ g(x), & \mbox{if} & g(x) \geq 0 \\ \end{array} \right.\)

The function \(f\) is defined so that when \(g(x)\) is negative (i.e., when its graph is below the \(x\)-axis), the graph of \(f\) is its refection across the \(x\)-axis. This is a general template to graph functions of the form \(f(x) = |g(x)|\). From this perspective, the graph of \(f(x) = |x|\) can be obtained by reflecting the portion of the line \(g(x) =x\) which is below the \(x\)-axis back above the \(x\)-axis creating the characteristic ‘\(\vee\)’ shape.

2.3.1 Exercises

In Exercises 1-9, graph the quadratic function. Find the \(x\)- and \(y\)-intercepts of each graph, if any exist. If it is given in general form, convert it into standard form; if it is given in standard form, convert it into general form. Find the domain and range of the function and list the intervals on which the function is increasing or decreasing. Identify the vertex and the axis of symmetry and determine whether the vertex yields a relative and absolute maximum or minimum.

1. \(f(x) = x^{2} + 2\)
2. \(f(x) = -(x + 2)^{2}\)
3. \(f(x) = x^{2} - 2x - 8\)
4. \(f(x) = -2(x + 1)^{2} + 4\)
5. \(f(x) = 2x^2 - 4x - 1\)
6. \(f(x) = -3x^{2} + 4x - 7\)
7. \(f(x) = x^2 + x + 1\)
8. \(f(x) = -3x^2+5x+4\)
9. ¹⁰ \(f(x) = x^{2} - \dfrac{1}{100} x - 1\)

In Exercises 10-14, the cost and price-demand functions are given for different scenarios. For each scenario,

• Find the profit function \(P(x)\).
• Find the number of items which need to be sold in order to maximize profit.
• Find the maximum profit.
• Find the price to charge per item in order to maximize profit.
• Find and interpret break-even points.

10. The cost, in dollars, to produce \(x\) “I’d rather be a Sasquatch” T-Shirts is \(C(x) = 2x+26\), \(x \geq 0\) and the price-demand function, in dollars per shirt, is \(p(x) = 30 - 2x\), \(0 \leq x \leq 15\). [maxprofitfirst]
11. The cost, in dollars, to produce \(x\) bottles of \(100 \%\) All-Natural Certified Free-Trade Organic Sasquatch Tonic is \(C(x) = 10x+100\), \(x \geq 0\) and the price-demand function, in dollars per bottle, is \(p(x) = 35 - x\), \(0 \leq x \leq 35\).
12. The cost, in cents, to produce \(x\) cups of Mountain Thunder Lemonade at Junior’s Lemonade Stand is \(C(x) = 18x + 240\), \(x \geq 0\) and the price-demand function, in cents per cup, is \(p(x) = 90-3x\), \(0 \leq x \leq 30\).
13. The daily cost, in dollars, to produce \(x\) Sasquatch Berry Pies is \(C(x) = 3x + 36\), \(x \geq 0\) and the price-demand function, in dollars per pie, is \(p(x) = 12-0.5x\), \(0 \leq x \leq 24\).
14. The monthly cost, in hundreds of dollars, to produce \(x\) custom built electric scooters is \(C(x) = 20x + 1000\), \(x \geq 0\) and the price-demand function, in hundreds of dollars per scooter, is \(p(x) = 140-2x\), \(0 \leq x \leq 70\). [maxprofitlast]
15. The International Silver Strings Submarine Band holds a bake sale each year to fund their trip to the National Sasquatch Convention. It has been determined that the cost in dollars of baking \(x\) cookies is \(C(x) = 0.1x + 25\) and that the demand function for their cookies is \(p = 10 - .01x.\) How many cookies should they bake in order to maximize their profit?
16. Using data from Bureau of Transportation Statistics, the average fuel economy \(\ F\) in miles per gallon for passenger cars in the US can be modeled by \(\ F(t)=-0.0076 t^{2}+0.45 t+16\), \(\ 0 \leq t \leq 28\), where \(\ t\) is the number of years since 1980. Find and interpret the coordinates of the vertex of the graph of \(\ y = F(t)\).
17. The temperature \(T\), in degrees Fahrenheit, \(t\) hours after 6 AM is given by: \[T(t) = -\frac{1}{2} t^2 + 8t+32, \quad 0 \leq t \leq 12\]
18. Suppose \(C(x) = x^2-10x+27\) represents the costs, in hundreds, to produce \(x\) thousand pens. How many pens should be produced to minimize the cost? What is this minimum cost?
19. [fixedperimetermaxareagarden] Skippy wishes to plant a vegetable garden along one side of his house. In his garage, he found 32 linear feet of fencing. Since one side of the garden will border the house, Skippy doesn’t need fencing along that side. What are the dimensions of the garden which will maximize the area of the garden? What is the maximum area of the garden?
20. In the situation of Example 2.3.4, Donnie has a nightmare that one of his alpaca herd fell into the river and drowned. To avoid this, he wants to move his rectangular pasture away from the river. This means that all four sides of the pasture require fencing. If the total amount of fencing available is still 200 linear feet, what dimensions maximize the area of the pasture now? What is the maximum area? Assuming an average alpaca requires 25 square feet of pasture, how many alpaca can he raise now?
21. What is the largest rectangular area one can enclose with 14 inches of string?
22. The height of an object dropped from the roof of an eight story building is modeled by \(h(t) = -16t^2 + 64\), \(0 \leq t \leq 2\). Here, \(h\) is the height of the object off the ground, in feet, \(t\) seconds after the object is dropped. How long before the object hits the ground?
23. The height \(h\) in feet of a model rocket above the ground \(t\) seconds after lift-off is given by \(h(t) = -5t^2+100t\), for \(0 \leq t \leq 20\). When does the rocket reach its maximum height above the ground? What is its maximum height?
24. Carl’s friend Jason participates in the Highland Games. In one event, the hammer throw, the height \(h\) in feet of the hammer above the ground \(t\) seconds after Jason lets it go is modeled by \(h(t) = -16t^2 + 22.08t + 6\). What is the hammer’s maximum height? What is the hammer’s total time in the air? Round your answers to two decimal places.
25. Assuming no air resistance or forces other than the Earth’s gravity, the height above the ground at time \(t\) of a falling object is given by \(s(t) = -4.9t^{2} + v_0t + s_0\) where \(s\) is in meters, \(t\) is in seconds, \(v_0\) is the object’s initial velocity in meters per second and \(s_0\) is its initial position in meters. [whatgoesup]
    1. What is the applied domain of this function?
    2. Discuss with your classmates what each of \(v_0 > 0, \; v_0 = 0\) and \(v_0 < 0\) would mean.
    3. Come up with a scenario in which \(s_0 < 0\).
    4. Let’s say a slingshot is used to shoot a marble straight up from the ground \((s_0 = 0)\) with an initial velocity of 15 meters per second. What is the marble’s maximum height above the ground? At what time will it hit the ground?
    5. Now shoot the marble from the top of a tower which is 25 meters tall. When does it hit the ground?
    6. What would the height function be if instead of shooting the marble up off of the tower, you were to shoot it straight DOWN from the top of the tower?
26. The two towers of a suspension bridge are 400 feet apart. The parabolic cable¹¹ attached to the tops of the towers is 10 feet above the point on the bridge deck that is midway between the towers. If the towers are 100 feet tall, find the height of the cable directly above a point of the bridge deck that is 50 feet to the right of the left-hand tower.
27. Graph \(f(x) = |1 - x^{2}|\)
28. Find all of the points on the line \(y=1-x\) which are \(2\) units from \((1,-1)\).
29. Let \(L\) be the line \(y = 2x+1\). Find a function \(D(x)\) which measures the distance squared from a point on \(L\) to \((0,0)\). Use this to find the point on \(L\) closest to \((0,0)\).
30. With the help of your classmates, show that if a quadratic function \(f(x) = ax^{2} + bx + c\) has two real zeros then the \(x\)-coordinate of the vertex is the midpoint of the zeros.

In Exercises 31-36, solve the quadratic equation for the indicated variable.

31. \(x^{2} - 10y^{2} = 0\) for \(x\)
32. \(y^{2} - 4y = x^{2} - 4\) for \(x\)
33. \(x^{2} - mx = 1\) for \(x\)
34. \(y^{2} - 3y = 4x\) for \(y\)
35. \(y^{2} - 4y = x^{2} - 4\) for \(y\)
36. \(-gt^{2} + v_0t + s_0 = 0\) for \(t\) (Assume \(g \neq 0\).)

2.3.2 Answers

1. \(f(x) = x^{2} + 2\) (this is both forms!)
   No \(x\)-intercepts
   \(y\)-intercept \((0, 2)\)
   Domain: \((-\infty, \infty)\)
   Range: \([2, \infty)\)
   Decreasing on \((-\infty, 0]\)
   Increasing on \([0, \infty)\)
   Vertex \((0, 2)\) is a minimum
   Axis of symmetry \(x = 0\)

   

2. \(f(x) = -(x + 2)^{2} = -x^2-4x-4\)
   \(x\)-intercept \((-2, 0)\)
   \(y\)-intercept \((0, -4)\)
   Domain: \((-\infty, \infty)\)
   Range: \((-\infty, 0]\)
   Increasing on \((-\infty, -2]\)
   Decreasing on \([-2, \infty)\)
   Vertex \((-2, 0)\) is a maximum
   Axis of symmetry \(x = -2\)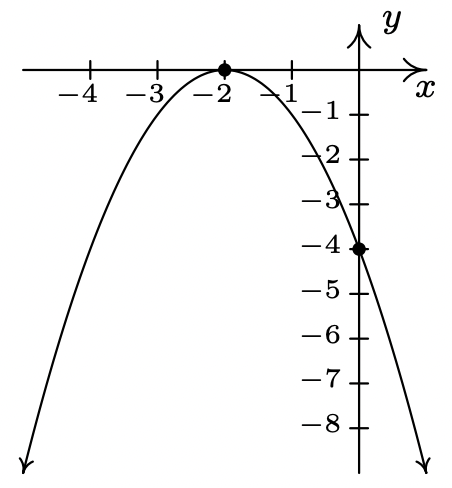

   

3. \(f(x) = x^{2} - 2x - 8 = (x - 1)^{2} - 9\)
   \(x\)-intercepts \((-2, 0)\) and \((4, 0)\)
   \(y\)-intercept \((0, -8)\)
   Domain: \((-\infty, \infty)\)
   Range: \([-9, \infty)\)
   Decreasing on \((-\infty, 1]\)
   Increasing on \([1, \infty)\)
   Vertex \((1, -9)\) is a minimum
   Axis of symmetry \(x = 1\)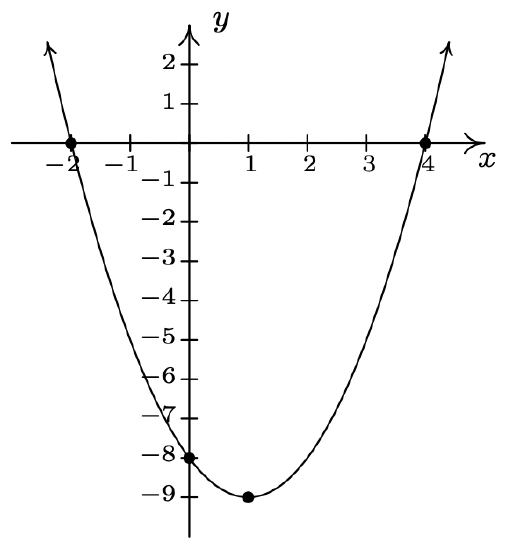

   

4. \(f(x) = -2(x + 1)^{2} + 4 = -2x^2-4x+2\)
   \(x\)-intercepts \((-1 - \sqrt{2}, 0)\) and \((-1 + \sqrt{2}, 0)\)
   \(y\)-intercept \((0, 2)\)
   Domain: \((-\infty, \infty)\)
   Range: \((-\infty, 4]\)
   Increasing on \((-\infty, -1]\)
   Decreasing on \([-1, \infty)\)
   Vertex \((-1, 4)\) is a maximum
   Axis of symmetry \(x = -1\)

   

5. \(f(x) = 2x^2-4x-1 = 2(x-1)^2-3\)
   \(x\)-intercepts \(\left(\frac{2-\sqrt{6}}{2}, 0\right)\) and \(\left(\frac{2+\sqrt{6}}{2}, 0\right)\)
   \(y\)-intercept \((0, -1)\)
   Domain: \((-\infty, \infty)\)
   Range: \([-3, \infty)\)
   Increasing on \([1,\infty)\)
   Decreasing on \((-\infty,1]\)
   Vertex \((1, -3)\) is a minimum
   Axis of symmetry \(x = 1\)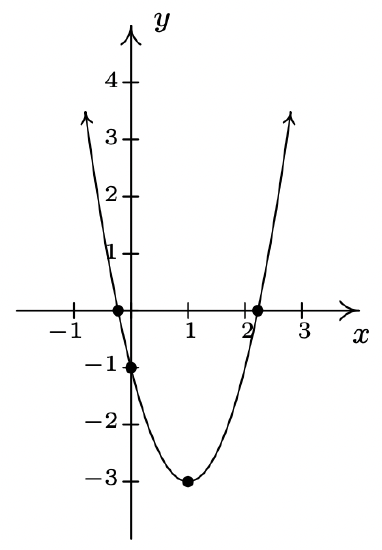

   

6. \(f(x) = -3x^{2} + 4x - 7 = -3\left(x - \frac{2}{3} \right)^{2} - \frac{17}{3}\)
   No \(x\)-intercepts
   \(y\)-intercept \((0, -7)\)
   Domain: \((-\infty, \infty)\)
   Range: \(\left(-\infty, -\frac{17}{3}\right]\)
   Increasing on \(\left(-\infty, \frac{2}{3}\right]\)
   Decreasing on \(\left[\frac{2}{3}, \infty\right)\)
   Vertex \(\left(\frac{2}{3}, -\frac{17}{3}\right)\) is a maximum
   Axis of symmetry \(x = \frac{2}{3}\)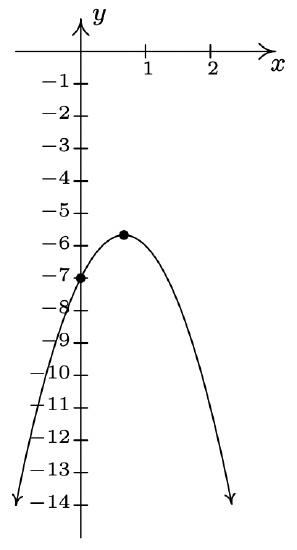

   

7. \(f(x) = x^2+x+1 = \left(x + \frac{1}{2}\right)^{2} + \frac{3}{4}\)
   No \(x\)-intercepts
   \(y\)-intercept \((0, 1)\)
   Domain: \((-\infty, \infty)\)
   Range: \(\left[ \frac{3}{4}, \infty\right)\)
   Increasing on \(\left[-\frac{1}{2}, \infty\right)\)
   Decreasing on \(\left(-\infty, -\frac{1}{2}\right]\)
   Vertex \(\left(-\frac{1}{2}, \frac{3}{4}\right)\) is a minimum
   Axis of symmetry \(x = -\frac{1}{2}\)

   

8. \(f(x) = -3x^2+5x+4 = -3\left(x-\frac{5}{6}\right)^2 + \frac{73}{12}\)
   \(x\)-intercepts \(\left(\frac{5 - \sqrt{73}}{6}, 0\right)\) and \(\left(\frac{5+\sqrt{73}}{6}, 0\right)\)
   \(y\)-intercept \((0, 4)\)
   Domain: \((-\infty, \infty)\)
   Range: \(\left(-\infty, \frac{73}{12} \right]\)
   Increasing on \(\left(-\infty, \frac{5}{6}\right]\)
   Decreasing on \(\left[ \frac{5}{6}, \infty\right)\)
   Vertex \(\left(\frac{5}{6}, \frac{73}{12} \right)\) is a maximum
   Axis of symmetry \(x = \frac{5}{6}\)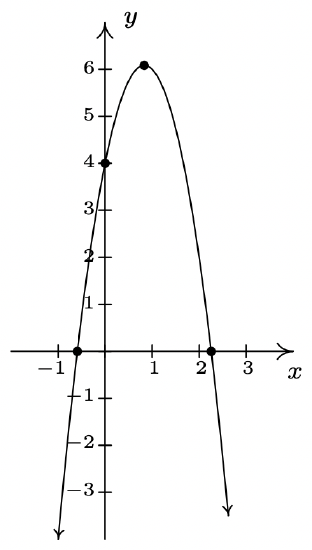

   

9. \(f(x) = x^{2} - \frac{1}{100} x - 1 = \left(x - \frac{1}{200}\right)^{2} - \frac{40001}{40000}\)
   \(x\)-intercepts \(\left(\frac{1 + \sqrt{40001}}{200}\right)\) and \(\left(\frac{1 - \sqrt{40001}}{200}\right)\)
   \(y\)-intercept \((0, -1)\)
   Domain: \((-\infty, \infty)\)
   Range: \(\left[-\frac{40001}{40000}, \infty \right)\)
   Decreasing on \(\left(-\infty, \frac{1}{200}\right]\)
   Increasing on \(\left[\frac{1}{200}, \infty \right)\)
   Vertex \(\left(\frac{1}{200}, -\frac{40001}{40000}\right)\) is a minimum¹²
   Axis of symmetry \(x = \frac{1}{200}\)

   

10. • \(P(x) = -2x^2+28x-26\), for \(0 \leq x \leq 15\).
    • \(7\) T-shirts should be made and sold to maximize profit.
    • The maximum profit is \(\$72\).
    • The price per T-shirt should be set at \(\$16\) to maximize profit.
    • The break even points are \(x=1\) and \(x=13\), so to make a profit, between 1 and 13 T-shirts need to be made and sold.
11. • \(P(x) = -x^2+25x-100\), for \(0 \leq x \leq 35\)
    • Since the vertex occurs at \(x=12.5\), and it is impossible to make or sell \(12.5\) bottles of tonic, maximum profit occurs when either \(12\) or \(13\) bottles of tonic are made and sold.
    • The maximum profit is \(\$56\).
    • The price per bottle can be either \(\$23\) (to sell 12 bottles) or \(\$22\) (to sell 13 bottles.) Both will result in the maximum profit.
    • The break even points are \(x=5\) and \(x=20\), so to make a profit, between 5 and 20 bottles of tonic need to be made and sold.
12. • \(P(x) = -3x^2+72x-240\), for \(0 \leq x \leq 30\)
    • \(12\) cups of lemonade need to be made and sold to maximize profit.
    • The maximum profit is \(192\)  or \(\$1.92\).
    • The price per cup should be set at \(54\)  per cup to maximize profit.
    • The break even points are \(x=4\) and \(x=20\), so to make a profit, between 4 and 20 cups of lemonade need to be made and sold.
13. • \(P(x) = -0.5 x^2+9x-36\), for \(0 \leq x \leq 24\)
    • \(9\) pies should be made and sold to maximize the daily profit.
    • The maximum daily profit is \(\$4.50\).
    • The price per pie should be set at \(\$7.50\) to maximize profit.
    • The break even points are \(x=6\) and \(x=12\), so to make a profit, between 6 and 12 pies need to be made and sold daily.
14. • \(P(x) = -2x^2+120x-1000\), for \(0 \leq x \leq 70\)
    • \(30\) scooters need to be made and sold to maximize profit.
    • The maximum monthly profit is \(800\) hundred dollars, or \(\$80,\!000\).
    • The price per scooter should be set at \(80\) hundred dollars, or \(\$8000\) per scooter.
    • The break even points are \(x=10\) and \(x=50\), so to make a profit, between 10 and 50 scooters need to be made and sold monthly.
15. 495 cookies
16. The vertex is (approximately) \((29.60, 22.66)\), which corresponds to a maximum fuel economy of 22.66 miles per gallon, reached sometime between 2009 and 2010 (29 – 30 years after 1980.) Unfortunately, the model is only valid up until 2008 (28 years after 1908.) So, at this point, we are using the model to predict the maximum fuel economy.
17. \(64^{\circ}\) at 2 PM (8 hours after 6 AM.)
18. 5000 pens should be produced for a cost of \(\$200\).
19. 8 feet by 16 feet; maximum area is 128 square feet.
20. 50 feet by 50 feet; maximum area is 2500 feet; he can raise 100 average alpacas.
21. The largest rectangle has area \(12.25\) square inches.
22. \(2\) seconds.
23. The rocket reaches its maximum height of \(500\) feet \(10\) seconds after lift-off.
24. The hammer reaches a maximum height of approximately \(13.62\) feet. The hammer is in the air approximately \(1.61\) seconds.
25. 1. The applied domain is \([0, \infty)\).
    2. The height function is this case is \(s(t) = -4.9t^{2} + 15t\). The vertex of this parabola is approximately \((1.53, 11.48)\) so the maximum height reached by the marble is \(11.48\) meters. It hits the ground again when \(t \approx 3.06\) seconds.
    3. The revised height function is \(s(t) = -4.9t^{2} + 15t + 25\) which has zeros at \(t \approx -1.20\) and \(t \approx 4.26\). We ignore the negative value and claim that the marble will hit the ground after \(4.26\) seconds.
    4. Shooting down means the initial velocity is negative so the height functions becomes \(s(t) = -4.9t^{2} - 15t + 25\).
26. Make the vertex of the parabola \((0, 10)\) so that the point on the top of the left-hand tower where the cable connects is \((-200, 100)\) and the point on the top of the right-hand tower is \((200, 100)\). Then the parabola is given by \(p(x) = \frac{9}{4000}x^{2} + 10\). Standing \(50\) feet to the right of the left-hand tower means you’re standing at \(x= -150\) and \(p(-150) = 60.625\). So the cable is 60.625 feet above the bridge deck there.
27. \(y = |1 -x^{2}|\)

    

28. \(\left(\dfrac{3 - \sqrt{7}}{2}, \dfrac{-1 + \sqrt{7}}{2} \right)\), \(\left(\dfrac{3 + \sqrt{7}}{2}, \dfrac{-1 - \sqrt{7}}{2} \right)\)
29. \(D(x) = x^2 + (2x+1)^2 = 5x^2+4x+1\), \(D\) is minimized when \(x=-\frac{2}{5}\), so the point on \(y=2x+1\) closest to \((0,0)\) is \(\left(-\frac{2}{5}, \frac{1}{5}\right)\)

31. \(x = \pm y\sqrt{10}\)
32. \(x = \pm (y - 2)\)
33. \(x = \dfrac{m \pm \sqrt{m^{2} + 4}}{2}\)
34. \(y = \dfrac{3 \pm \sqrt{16x + 9}}{2}\)
35. \(y = 2 \pm x\)
36. \(t = \dfrac{v_0 \pm \sqrt{v_0^{2} + 4gs_0}}{2g}\)

---

Reference

¹ and rationalizing denominators!

² and get common denominators!

³ Just a scaling if \(\ a>0\). If \(\ a < 0\), there is a reflection involved.

⁴ Right if \(\ h > 0\), left if \(\ h < 0\).

⁵ Up if \(\ k > 0\), down if \(\ k < 0\).

⁶ You should use transformations to verify this!

⁷ If you forget why we do what we do to complete the square, star \(\ a(x-h)^{2}+k\), multiply it out, step by step, and then reverse the process.

⁸ Experience pays off, here!

⁹ Donnie would be very upset if, for example, we told him the width of the pasture needs to be −50 feet

¹⁰ We have already seen the graph of this function. It was used as an example in Section 1.6 to show how the graphing calculator can be misleading.

¹² You’ll need to use your calculator to zoom in far enough to see that the vertex is not the y-interception.