4.6: Evolution of Virulence

Microorganisms continuously evolve due to selection pressures in their environments. Antibiotics are a common source of selection pressure on pathogenic bacteria, and the development of antibiotic-resistant strains presents a major health challenge to medical science. Bacteria and viruses also compete directly with each other for reproductive success resulting in the evolution of virulence. Here, using the SIR endemic disease model, we study how virulence may evolve.

For the sake of argument, we will assume that a population is initially in equilibrium with an endemic disease caused by a wildtype virus; that is, \(S, I\) and \(R\) are assumed to be nonzero and at equilibrium values. Now suppose that some virus particles mutate by a random, undirected process that occurs naturally. We want to determine the conditions under which the mutant virus will replace the wildtype virus in the population. In mathematical terms, we want to determine the linear stability of the endemic disease equilibrium to the introduction of a mutant viral strain.

We assume that the original wildtype virus has infection rate \(\beta\), removal rate \(\gamma\), and disease-related death rate \(c\), and that the mutant virus has corresponding rates \(\beta^{\prime}, \gamma^{\prime}\) and \(c^{\prime} .\) We further assume that an individual infected with either a wildtype or mutant virus gains immunity to subsequent infection from both wildtype and mutant viral forms. Our model thus has a single susceptible class \(S\), two distinct infective classes \(I\) and \(I^{\prime}\) depending on which virus causes the infection, and a single recovered class \(R\). The appropriate diagram is

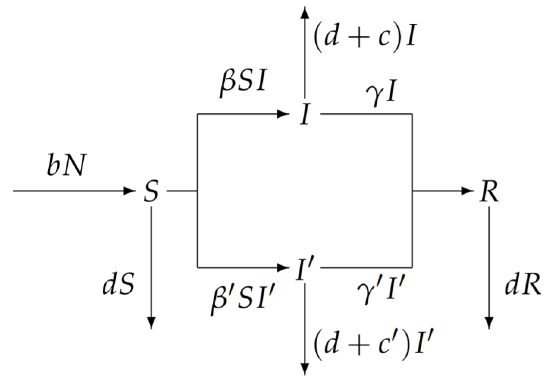
with corresponding differential equations

\[\begin{align} \frac{d S}{d t} &=b N-d S-S\left(\beta I+\beta^{\prime} I^{\prime}\right) \\[4pt] \frac{d I}{d t} &=\beta S I-(d+c+\gamma) I \\[4pt] \frac{d I^{\prime}}{d t} &=\beta^{\prime} S I^{\prime}-\left(d+c^{\prime}+\gamma^{\prime}\right) I^{\prime} \\[4pt] \frac{d R}{d t} &=\gamma I+\gamma^{\prime} I^{\prime}-d R \end{align} \nonumber \]

If the population is initially in equilibrium with the wildtype virus, then we have \(\dot{I}=0\) with \(I \neq 0\), and the equilibrium value for \(S\) is determined from (4.6.2) to be

\[S_{*}=\frac{d+c+\gamma}{\beta}, \nonumber \]

which corresponds to a basic reproductive ratio \(\beta S_{*} /(d+c+\gamma)\) of unity.

We perturb this endemic disease equilibrium by introducing a small number of infectives carrying the mutated virus, that is, by letting \(I^{\prime}\) be small. Rather than solve the stability problem by means of a Jacobian analysis, we can directly examine the equation for \(d I^{\prime} / d t\) given by (4.6.3). Here, with \(S=S_{*}\) given by (4.6.5), we have

\[\frac{d I^{\prime}}{d t}=\left[\frac{\beta^{\prime}(d+c+\gamma)}{\beta}-\left(d+c^{\prime}+\gamma^{\prime}\right)\right] I^{\prime} \nonumber \]

and \(I^{\prime}\) increases exponentially if

\[\frac{\beta^{\prime}(d+c+\gamma)}{\beta}-\left(d+c^{\prime}+\gamma^{\prime}\right)>0 \nonumber \]

or after some elementary algebra,

\[\frac{\beta^{\prime}}{d+c^{\prime}+\gamma^{\prime}}>\frac{\beta}{d+c+\gamma} \nonumber \]

Our result (4.6.8) suggests that endemic viruses (or other microorganisms) will tend to evolve (i) to be more easily transmitted between people \(\left(\beta^{\prime}>\beta\right) ;\) (ii) to make people sick longer \(\left(\gamma^{\prime}<\gamma\right)\), and; (iii) to be less deadly \(c^{\prime}<c\). In other words, viruses evolve to increase their basic reproductive ratios. For instance, our model suggests that viruses evolve to be less deadly because the dead do not spread disease. Our result would not be applicable, however, if the dead in fact did spread disease, a possibility if disposal of the dead was not done with sufficient care, perhaps because of certain cultural traditions such as family washing of the dead body.