1.6: Triangle CIassifications
( \newcommand{\kernel}{\mathrm{null}\,}\)
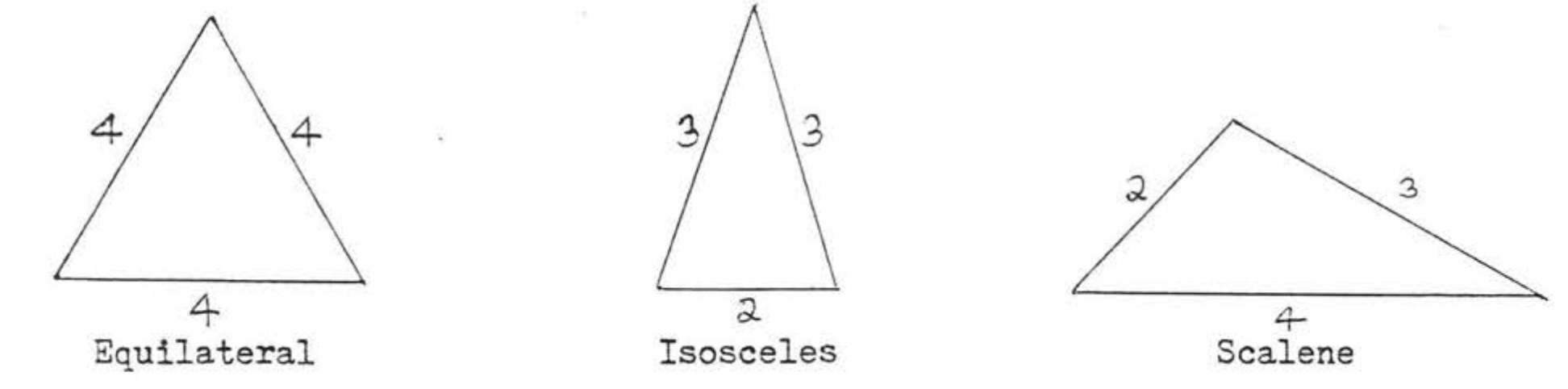
Triangles may be classified according to the relative lengths of their sides:
- An equilateral triangle has three equal sides,
- An isosceles triangle has two equal sides.
- A scalene triangle has no equal sides.
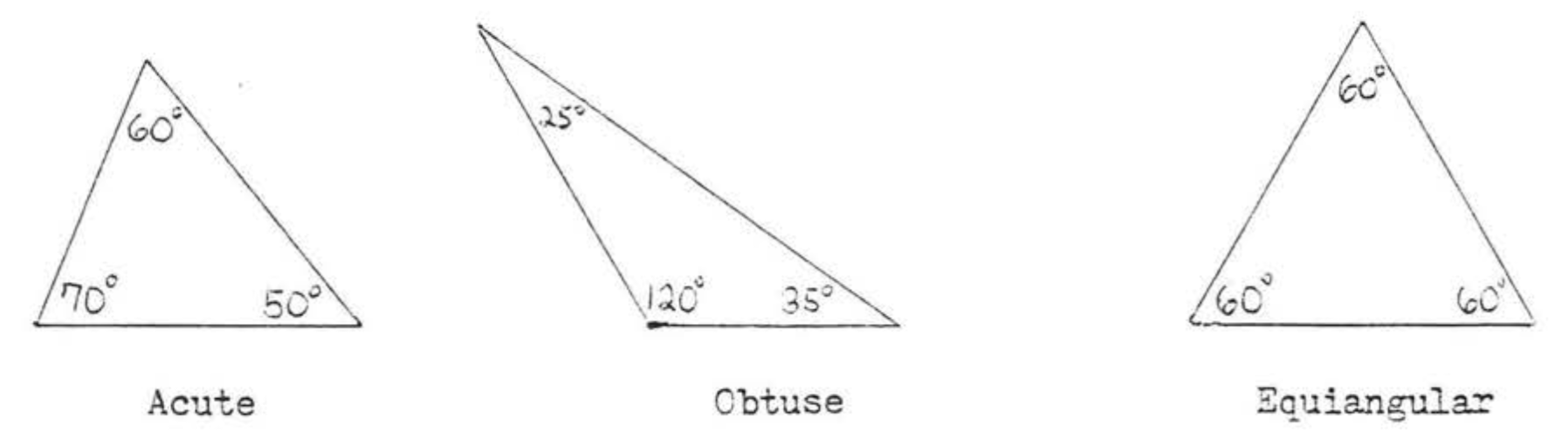
Triangles may also be classified according to the measure of their angles:
- An acute triangle is a triangle with three acute angles.
- An obtuse triangle is a triangle with one obtuse angle.
- An equiangular triangle is a triangle with three equal angles,
Each angle of an equiangular triangle must be
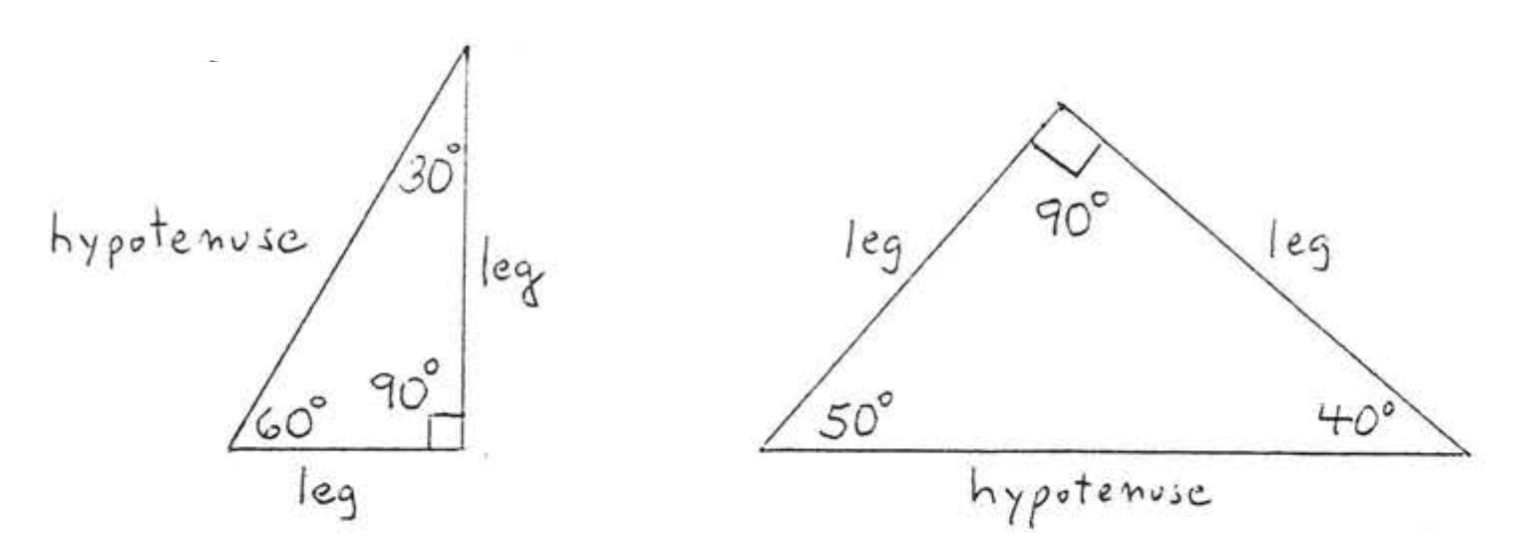
A right triangle is a triangle with one right angle, The sides of the right angle are called the legs of the triangle and the remaining side is called the hypotenuse,
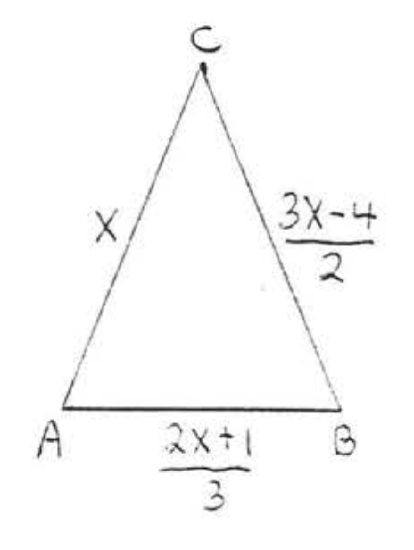
Find
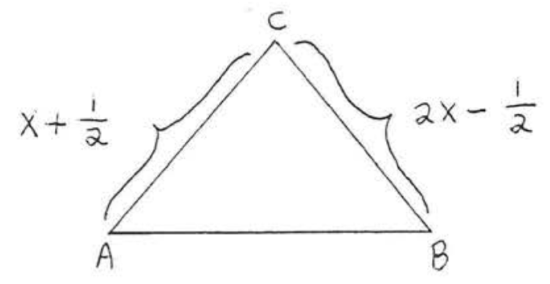
Solution
Check:
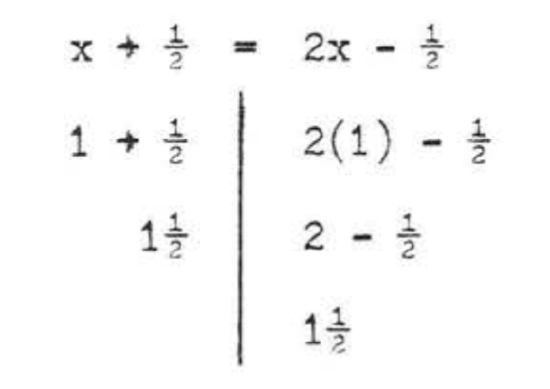
Answer:
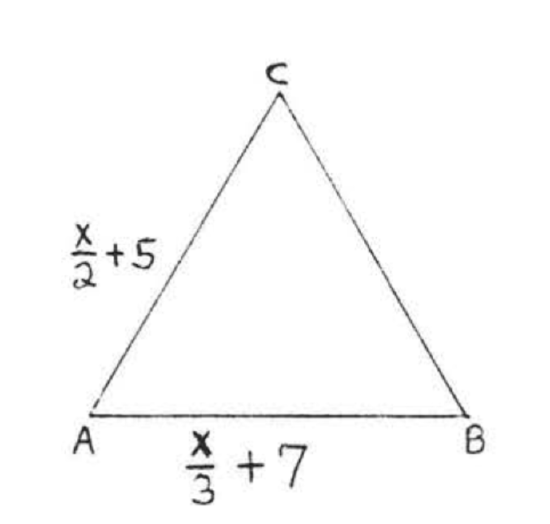
Solution
Check:
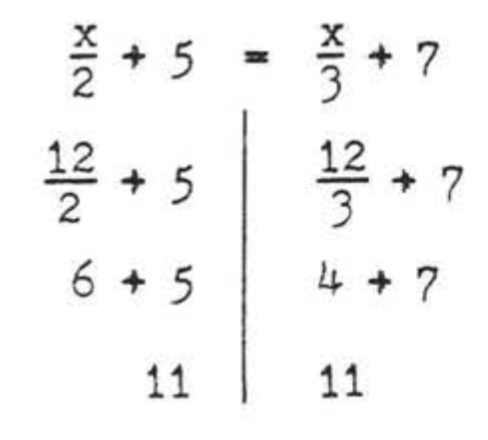
Answer:
An altitude of a triangle is a line segment from a vertex perpendicular to the opposite·side, In Figure 4,
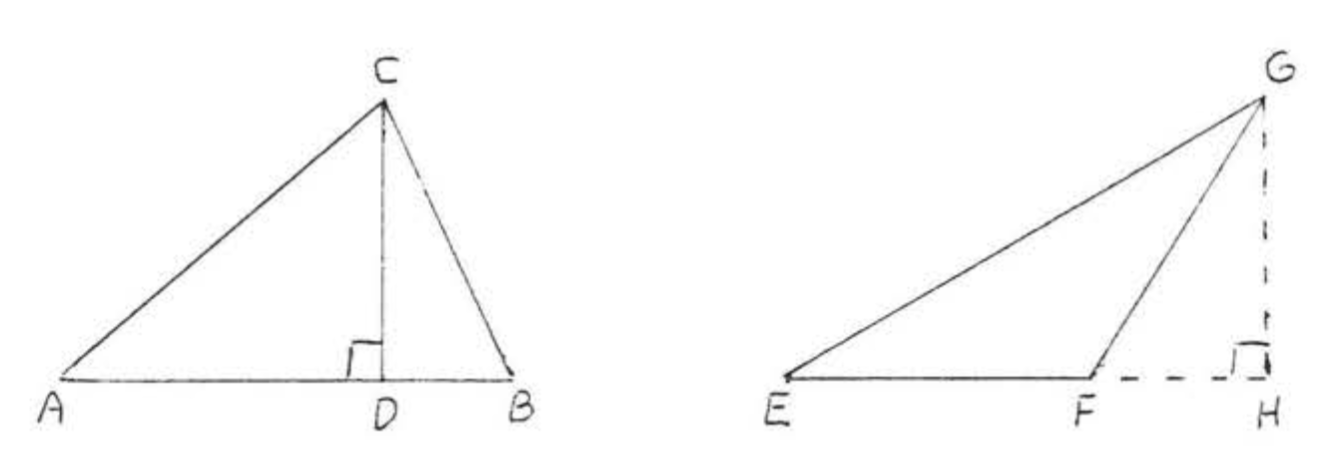
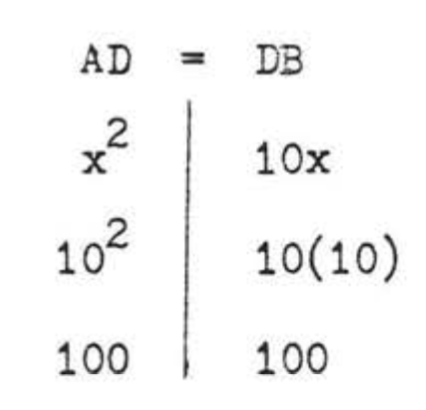
A median of a triangle is a line segment from a vertex to the midpoint of the opposite side, In Figure 5, CD is a median,
An angle bisector is a ray which divides an angle into two eaual angles. In Figure

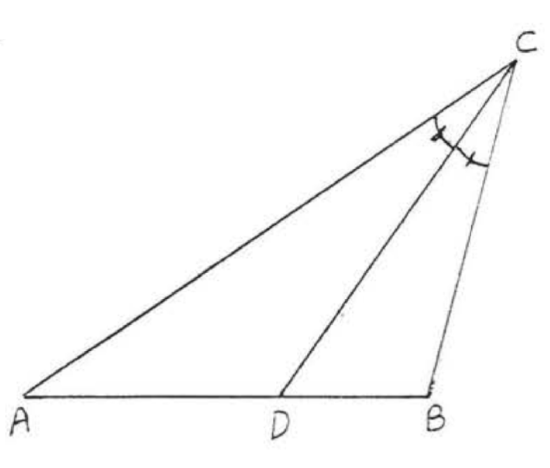
Find
Solution
Check,
Check,
We reject the answer
Answer:
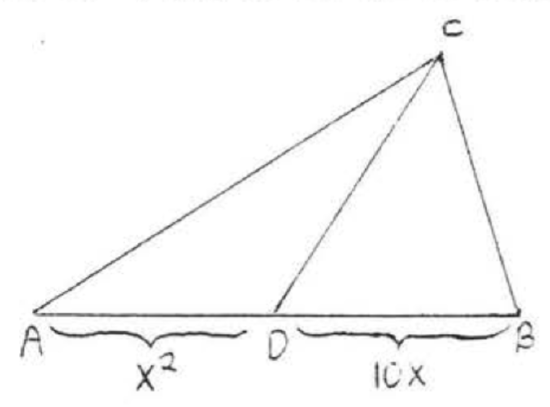
Find
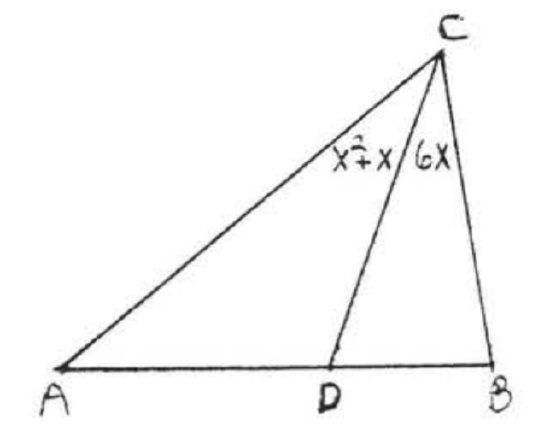
Solution
Check,
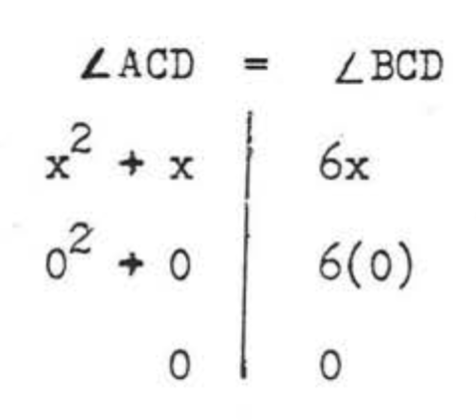
Check,
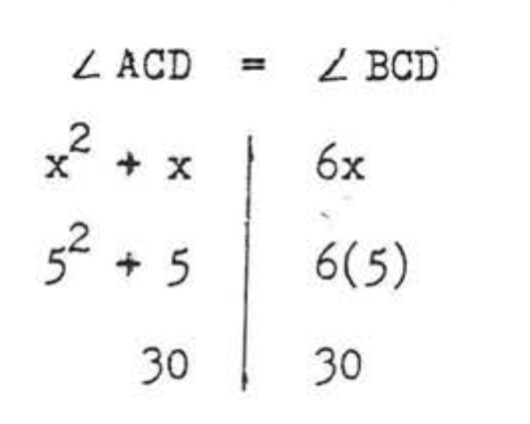
We reject the answer
Answer:
The perimeter of a triangle is the sum of the lengths of the sides. The perimeter of

The sum of any two sides of a triangle is greater than the remaining side.
For example, in Figure
- Proof
-
This follows from the postulate that the shortest distance between two noints is along a straight line, For example, in Figure
Find the perimeter of the triangle in terms of
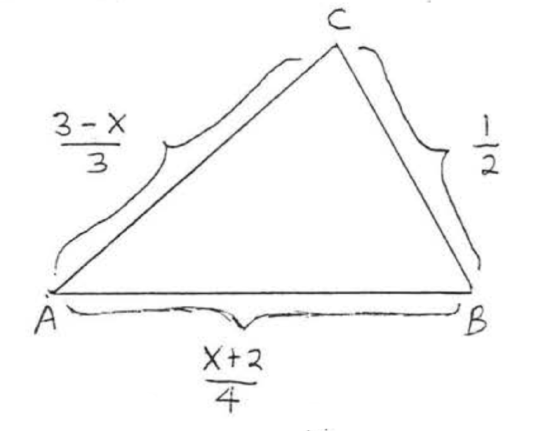
Solution
If
Check:
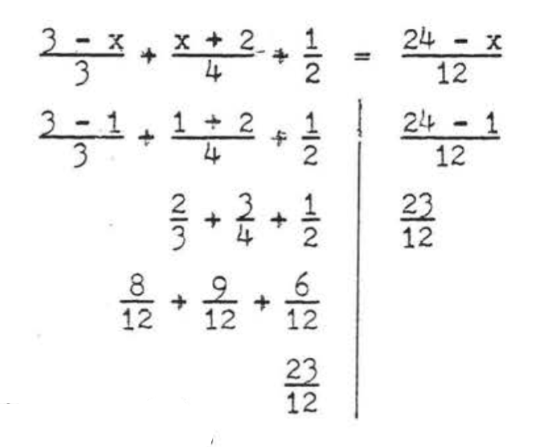
Answer:
Problems
1 - 2. Find
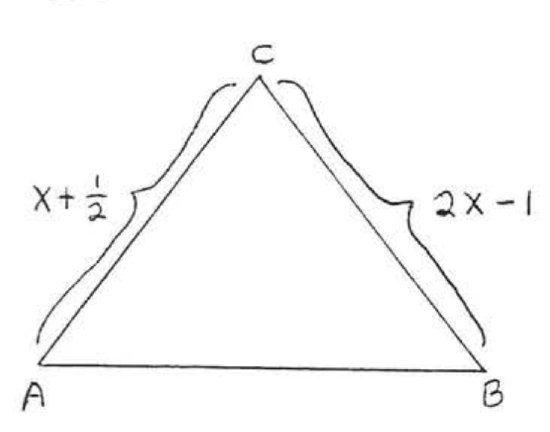
1. 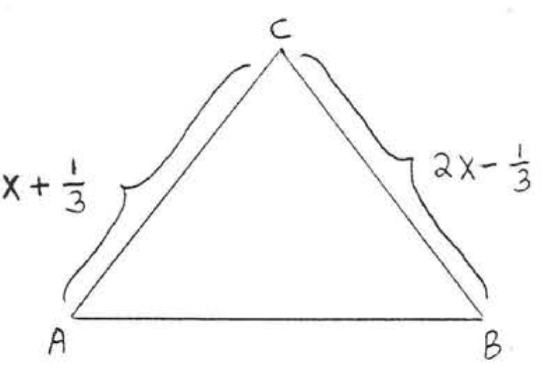 2.
2. 
3 - 4. Find
3. 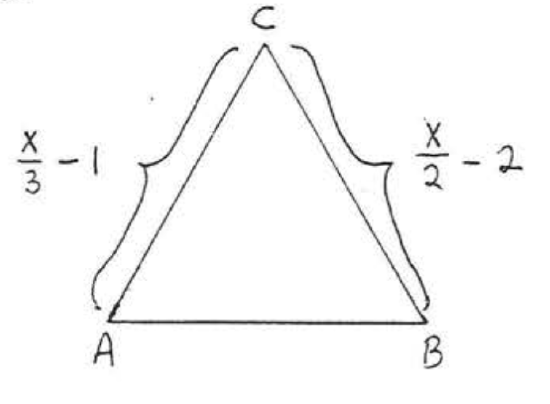 4.
4. 
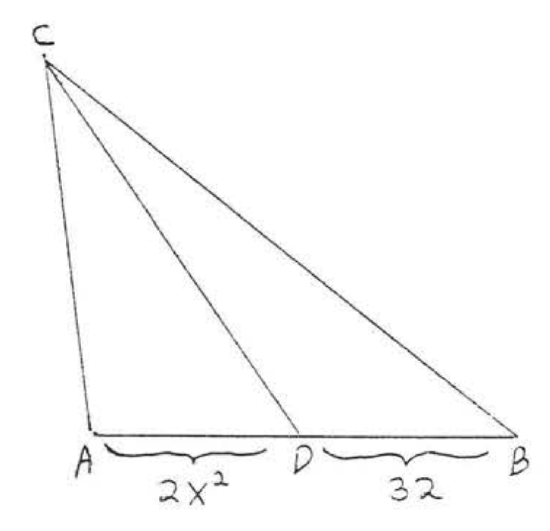
5 - 6. Find
5.  6.
6. 
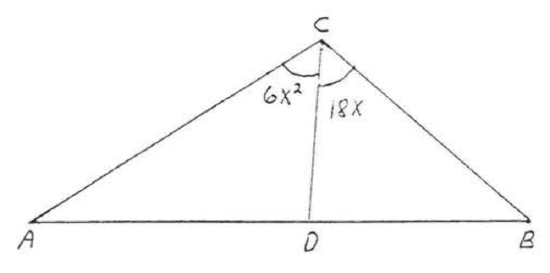
7 - 8. Find
7.  8.
8. 
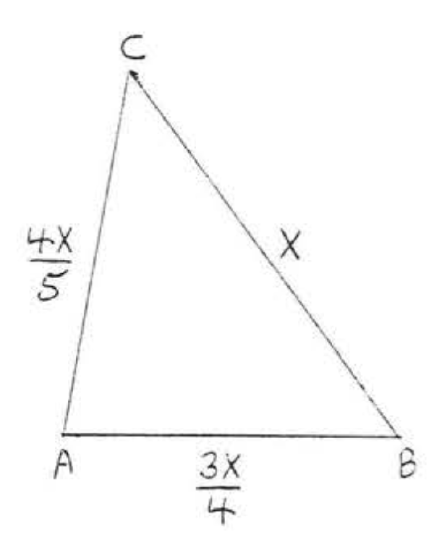
9 - 10. Find the perimeter of the triangle in terms of
9. 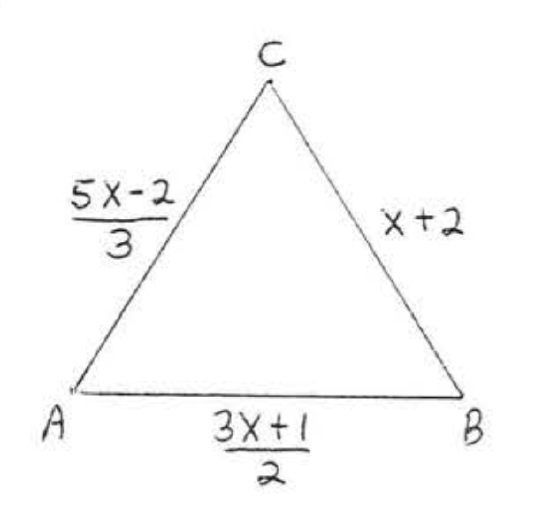 10.
10. 
11. Find

12. Find