4.3: Equivalent Fractions, Reducing Fractions to Lowest Terms, and Raising Fractions to Higher Terms

Learning Objectives

• be able to recognize equivalent fractions
• be able to reduce a fraction to lowest terms
• be able to raise a fraction to higher terms

Equivalent Fractions

Let's examine the following two diagrams.

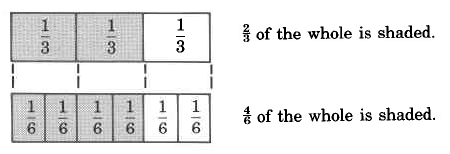
Notice that both $\dfrac{2}{3}$ and $\dfrac{4}{6}$ represent the same part of the whole, that is, they represent the same number.

Definition: Equivalent Fractions

Fractions that have the same value are called equivalent fractions. Equiva­lent fractions may look different, but they are still the same point on the number line.

There is an interesting property that equivalent fractions satisfy.

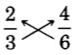
A Test for Equivalent Fractions Using the Cross Product

Definition: cross products

These pairs of products are called cross products.

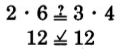
If the cross products are equal, the fractions are equivalent. If the cross products are not equal, the fractions are not equivalent.

Thus, $\dfrac{2}{3}$ and $\dfrac{4}{6}$ are equivalent, that is, $\dfrac{2}{3} = \dfrac{4}{6}$.

Sample Set A

Determine if the following pairs of fractions are equivalent.

$\dfrac{3}{4}$ and $\dfrac{6}{8}$. Test for equality of the cross products.

Solution

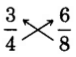
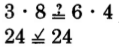
The cross products are equals.

The fractions $\dfrac{3}{4}$ and $\dfrac{6}{8}$ are equivalent, so $\dfrac{3}{4} = \dfrac{6}{8}$.

Sample Set A

$\dfrac{3}{8}$ and $\dfrac{9}{16}$. Test for equality of the cross products.

Solution

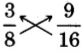
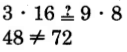
The cross products are equals.

The fractions $\dfrac{3}{8}$ and $\dfrac{9}{16}$ are not equivalent.

Practice Set A

Determine if the pairs of fractions are equivalent.

$\dfrac{1}{2}$, $\dfrac{3}{6}$

Answer

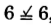
, yes

Practice Set A

$\dfrac{4}{5}$, $\dfrac{12}{15}$

Answer

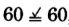
, yes

Practice Set A

$\dfrac{2}{3}$, $\dfrac{8}{15}$

Answer

$30 \ne 24$, no

Practice Set A

$\dfrac{1}{8}$, $\dfrac{4}{50}$

Answer

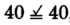
, yes

Practice Set A

$\dfrac{3}{12}$, $\dfrac{1}{4}$

Answer

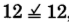
, yes

Reducing Fractions to Lowest Terms

It is often very useful to convert one fraction to an equivalent fraction that has reduced values in the numerator and denominator. We can suggest a method for doing so by considering the equivalent fractions $\dfrac{9}{15}$ and $\dfrac{3}{5}$. First, divide both the numerator and denominator of $\dfrac{9}{15}$ by 3. The fraction $\dfrac{9}{15}$ and $\dfrac{3}{5}$ are equivalent.

(Can you prove this?) So, $\dfrac{9}{15} = \dfrac{3}{5}$. We wish to convert $\dfrac{9}{15}$ to $\dfrac{3}{5}$. Now divide the numerator and denominator of $\dfrac{9}{15}$ by 3, and see what happens.

$\dfrac{9 \div 3}{15 \div 3} = \dfrac{3}{5}$

The fraction $\dfrac{9}{15}$ is converted to $\dfrac{3}{5}$.

A natural question is "Why did we choose to divide by 3?" Notice that

$\dfrac{9}{15} = \dfrac{3 \cdot 3}{5 \cdot 3}$

We can see that the factor 3 is common to both the numerator and denominator.

Definition: Reducing a Fraction

From these observations we can suggest the following method for converting one fraction to an equivalent fraction that has reduced values in the numerator and denominator. The method is called reducing a fraction.

A fraction can be reduced by dividing both the numerator and denominator by the same nonzero whole number.

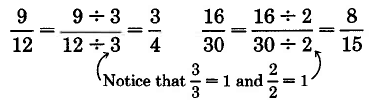
Consider the collection of equivalent fractions

$\dfrac{5}{20}, \dfrac{4}{16}, \dfrac{3}{12}, \dfrac{2}{8}, \dfrac{1}{4}$

Reduced to Lowest Terms

Notice that each of the first four fractions can be reduced to the last fraction, $\dfrac{1}{4}$, by dividing both the numerator and denominator by, respectively, 5, 4, 3, and 2. When a fraction is converted to the fraction that has the smallest numerator and denomi­nator in its collection of equivalent fractions, it is said to be reduced to lowest terms. The fractions $\dfrac{1}{4}$, $\dfrac{3}{8}$, $\dfrac{2}{5}$, and $\dfrac{7}{10}$ are all reduced to lowest terms.

Observe a very important property of a fraction that has been reduced to lowest terms. The only whole number that divides both the numerator and denominator without a remainder is the number 1. When 1 is the only whole number that divides two whole numbers, the two whole numbers are said to be relatively prime.

Relatively Prime
A fraction is reduced to lowest terms if its numerator and denominator are relatively prime.

Methods of Reducing Fractions to Lowest Terms

Method 1: Dividing Out Common Primes

1. Write the numerator and denominator as a product of primes.
2. Divide the numerator and denominator by each of the common prime factors. We often indicate this division by drawing a slanted line through each divided out factor. This process is also called cancelling common factors.
3. The product of the remaining factors in the numerator and the product of remaining factors of the denominator are relatively prime, and this fraction is reduced to lowest terms.

Sample Set B

$\dfrac{6}{18} = \dfrac{\begin{array} {c} {^1} \\ {\cancel{2}} \end{array} \cdot \begin{array} {c} {^1} \\ {\cancel{3}} \end{array}}{\begin{array} {c} {\cancel{2}} \\ {^1} \end{array} \cdot \begin{array} {c} {\cancel{3}} \\ {^1} \end{array} \cdot 3} = \dfrac{1}{3}$ 1 and 3 are relatively prime.

Sample Set B

$\dfrac{16}{20} = \dfrac{\begin{array} {c} {^1} \\ {\cancel{2}} \end{array} \cdot \begin{array} {c} {^1} \\ {\cancel{2}} \end{array} \cdot 2 \cdot 2}{\begin{array} {c} {\cancel{2}} \\ {^1} \end{array} \cdot \begin{array} {c} {\cancel{2}} \\ {^1} \end{array} \cdot 5} = \dfrac{4}{5}$ 4 and 5 are relatively prime.

Sample Set B

$\dfrac{56}{104} = \dfrac{\begin{array} {c} {^1} \\ {\cancel{2}} \end{array} \cdot \begin{array} {c} {^1} \\ {\cancel{3}} \end{array} \begin{array} {c} {^1} \\ {\cancel{2}} \end{array} \cdot 7}{\begin{array} {c} {\cancel{2}} \\ {^1} \end{array} \cdot \begin{array} {c} {\cancel{2}} \\ {^1} \end{array} \cdot \begin{array} {c} {\cancel{2}} \\ {^1} \end{array} \cdot 13} = \dfrac{7}{13}$ 7 and 13 are relatively prime (and also truly prime)

Sample Set B

$\dfrac{315}{336} = \dfrac{\begin{array} {c} {^1} \\ {\cancel{3}} \end{array} \cdot 3 \cdot 5 \cdot \begin{array} {c} {^1} \\ {\cancel{7}} \end{array}}{2 \cdot 2 \cdot 2 \cdot 2 \cdot \begin{array} {c} {\cancel{3}} \\ {^1} \end{array} \cdot \begin{array} {c} {\cancel{7}} \\ {^1} \end{array}} = \dfrac{15}{16}$ 15 and 16 are relatively prime.

Sample Set B

$\dfrac{8}{15} = \dfrac{2 \cdot 2 \cdot 2}{3 \cdot 5}$ No common prime factors, so 8 and 15 are relatively prime.

The fraction $\dfrac{8}{15}$ is reduced to lowest terms.

Practice Set B

Reduce each fraction to lowest terms.

$\dfrac{4}{8}$

Answer

$\dfrac{1}{2}$

Practice Set B

$\dfrac{6}{15}$

Answer

$\dfrac{2}{5}$

Practice Set B

$\dfrac{6}{48}$

Answer

$\dfrac{1}{8}$

Practice Set B

$\dfrac{21}{48}$

Answer

$\dfrac{7}{16}$

Practice Set B

$\dfrac{72}{42}$

Answer

$\dfrac{12}{7}$

Practice Set B

$\dfrac{135}{243}$

Answer

$\dfrac{5}{9}$

Method 2: Dividing Out Common Factors

1. Mentally divide the numerator and the denominator by a factor that is com­mon to each. Write the quotient above the original number.
2. Continue this process until the numerator and denominator are relatively prime.

Sample Set C

Reduce each fraction to lowest terms.

$\dfrac{25}{30}$. 5 divides into both 25 and 30.

$\dfrac{\begin{array} {c} {^5} \\ {\cancel{25}} \end{array}}{\begin{array} {c} {\cancel{30}} \\ {^6} \end{array}} = \dfrac{5}{6}$ 5 and 6 are relatively prime.

Sample Set C

$\dfrac{18}{24}$. Both numbers are even so we can divide by 2.

$\dfrac{\begin{array} {c} {^9} \\ {\cancel{18}} \end{array}}{\begin{array} {c} {\cancel{24}} \\ {^{12}} \end{array}}$ Now, both 9 and 12 are divisible by 3.

$\dfrac{\begin{array} {c} {^{^3}} \\ {^{\cancel{9}}} \\ {\cancel{18}} \end{array}}{\begin{array} {c} {\cancel{24}} \\ {^{\cancel{12}}} \\ {^{^4}} \end{array}} = \dfrac{3}{4}$ 3 and 4 are relatively prime.

Sample Set C

$\dfrac{\begin{array} {c} {^{^7}} \\ {^{\cancel{21}}} \\ {\cancel{210}} \end{array}}{\begin{array} {c} {\cancel{150}} \\ {^{\cancel{15}}} \\ {^{^5}} \end{array}} = \dfrac{7}{5}$ 7 and 5 are relatively prime.

Sample Set C

$\dfrac{36}{96} = \dfrac{18}{48} = \dfrac{9}{24} = \dfrac{3}{8}$. 3 and 8 are relatively prime.

Practice Set C

Reduce each fraction to lowest terms.

$\dfrac{12}{16}$

Answer

$\dfrac{3}{4}$

Practice Set C

$\dfrac{9}{24}$

Answer

$\dfrac{3}{8}$

Practice Set C

$\dfrac{21}{84}$

Answer

$\dfrac{1}{4}$

Practice Set C

$\dfrac{48}{64}$

Answer

$\dfrac{3}{4}$

Practice Set C

$\dfrac{63}{81}$

Answer

$\dfrac{7}{9}$

Practice Set C

$\dfrac{150}{240}$

Answer

$\dfrac{5}{8}$

Raising Fractions to Higher Terms

Equally as important as reducing fractions is raising fractions to higher terms. Raising a fraction to higher terms is the process of constructing an equivalent fraction that has higher values in the numerator and denominator than the original fraction.

The fractions $\dfrac{3}{5}$ and $\dfrac{9}{15}$ are equivalent, that is, $\dfrac{3}{5} = \dfrac{9}{15}$. Notice also,

$\dfrac{3 \cdot 3}{5 \cdot 3} = \dfrac{9}{15}$

Notice that $\dfrac{3}{3} = 1$ and that $\dfrac{3}{5} \cdot 1 = \dfrac{3}{5}$. We are not changing the value of $\dfrac{3}{5}$.

From these observations we can suggest the following method for converting one fraction to an equivalent fraction that has higher values in the numerator and denominator. This method is called raising a fraction to higher terms.

Raising a Fraction to Higher Terms
A fraction can be raised to an equivalent fraction that has higher terms in the numerator and denominator by multiplying both the numerator and denominator by the same nonzero whole number.

The fraction $\dfrac{3}{4}$ can be raised to $\dfrac{24}{32}$ by multiplying both the numerator and denominator by 8.

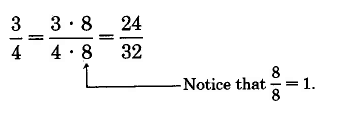
Most often, we will want to convert a given fraction to an equivalent fraction with a higher specified denominator. For example, we may wish to convert $\dfrac{5}{8}$ to an equivalent fraction that has denominator 32, that is,

$\dfrac{5}{8} = \dfrac{?}{32}$

This is possible to do because we know the process. We must multiply both the numerator and denominator of $\dfrac{5}{8}$ by the samenonzero whole number in order to 8 obtain an equivalent fraction.

We have some information. The denominator 8 was raised to 32 by multiplying it by some nonzero whole number. Division will give us the proper factor. Divide the original denominator into the new denominator.

$32 \div 8 = 4$

Now, multiply the numerator 5 by 4.

$5 \cdot 4 = 20$

Thus,

$\dfrac{5}{8} = \dfrac{5 \cdot 4}{8 \cdot 4} = \dfrac{20}{32}$

So,

$\dfrac{5}{8} = \dfrac{20}{32}$

Sample Set D

Determine the missing numerator or denominator.

$\dfrac{3}{7} = \dfrac{?}{35}$. Divide the original denominator into the new denominator.

$35 \div 7 = 5$. The quotient is 5. Multiply the original numerator by 5.

$\dfrac{3}{7} = \dfrac{3 \cdot 5}{7 \cdot 5} = \dfrac{15}{35}$ The missing numerator is 15.

Sample Set D

$\dfrac{5}{6} = \dfrac{45}{?}$. Divide the original numerator into the new numerator.

$45 \div 5 = 9$. The quotient is 9. Multiply the original denominator by 9.

$\dfrac{5}{6} = \dfrac{5 \cdot 9}{6 \cdot 9} = \dfrac{45}{54}$ The missing denominator is 45.

Practice Set D

Determine the missing numerator or denominator.

$\dfrac{4}{5} = \dfrac{?}{40}$

Answer

32

Practice Set D

$\dfrac{3}{7} = \dfrac{?}{28}$

Answer

12

Practice Set D

$\dfrac{1}{6} = \dfrac{?}{24}$

Answer

4

Practice Set D

$\dfrac{3}{10} = \dfrac{45}{?}$

Answer

150

Practice Set D

$\dfrac{8}{15} = \dfrac{?}{165}$

Answer

88

Exercises

For the following problems, determine if the pairs of fractions are equivalent.

Exercise $\PageIndex{1}$

$\dfrac{1}{2}, \dfrac{5}{10}$

Answer

equivalent

Exercise $\PageIndex{2}$

$\dfrac{2}{3}, \dfrac{8}{12}$

Exercise $\PageIndex{3}$

$\dfrac{5}{12}, \dfrac{10}{24}$

Answer

equivalent

Exercise $\PageIndex{4}$

$\dfrac{1}{2}, \dfrac{3}{6}$

Exercise $\PageIndex{5}$

$\dfrac{3}{5}, \dfrac{12}{15}$

Answer

not equivalent

Exercise $\PageIndex{6}$

$\dfrac{1}{6}, \dfrac{7}{42}$

Exercise $\PageIndex{7}$

$\dfrac{16}{25}, \dfrac{49}{75}$

Answer

not equivalent

Exercise $\PageIndex{8}$

$\dfrac{5}{28}, \dfrac{20}{112}$

Exercise $\PageIndex{9}$

$\dfrac{3}{10}, \dfrac{36}{110}$

Answer

not equivalent

Exercise $\PageIndex{10}$

$\dfrac{6}{10}, \dfrac{18}{32}$

Exercise $\PageIndex{11}$

$\dfrac{5}{8}, \dfrac{15}{24}$

Answer

equivalent

Exercise $\PageIndex{12}$

$\dfrac{10}{16}, \dfrac{15}{24}$

Exercise $\PageIndex{13}$

$\dfrac{4}{5}, \dfrac{3}{4}$

Answer

not equivalent

Exercise $\PageIndex{14}$

$\dfrac{5}{7}, \dfrac{15}{21}$

Exercise $\PageIndex{15}$

$\dfrac{9}{11}, \dfrac{11}{9}$

Answer

not equivalent

For the following problems, determine the missing numerator or denominator.

Exercise $\PageIndex{16}$

$\dfrac{1}{3} = \dfrac{?}{12}$

Exercise $\PageIndex{17}$

$\dfrac{1}{5} = \dfrac{?}{30}$

Answer

6

Exercise $\PageIndex{18}$

$\dfrac{2}{3} = \dfrac{?}{9}$

Exercise $\PageIndex{19}$

$\dfrac{3}{4} = \dfrac{?}{16}$

Answer

12

Exercise $\PageIndex{20}$

$\dfrac{5}{6} = \dfrac{?}{18}$

Exercise $\PageIndex{21}$

$\dfrac{4}{5} = \dfrac{?}{25}$

Answer

20

Exercise $\PageIndex{22}$

$\dfrac{1}{2} = \dfrac{4}{?}$

Exercise $\PageIndex{23}$

$\dfrac{9}{25} = \dfrac{27}{?}$

Answer

75

Exercise $\PageIndex{24}$

$\dfrac{3}{2} = \dfrac{18}{?}$

Exercise $\PageIndex{25}$

$\dfrac{5}{3} = \dfrac{80}{?}$

Answer

48

Exercise $\PageIndex{26}$

$\dfrac{1}{8} = \dfrac{3}{?}$

Exercise $\PageIndex{27}$

$\dfrac{4}{5} = \dfrac{?}{100}$

Answer

80

Exercise $\PageIndex{28}$

$\dfrac{1}{2} = \dfrac{25}{?}$

Exercise $\PageIndex{29}$

$\dfrac{3}{16} = \dfrac{?}{96}$

Answer

18

Exercise $\PageIndex{30}$

$\dfrac{15}{16} = \dfrac{225}{?}$

Exercise $\PageIndex{31}$

$\dfrac{11}{12} = \dfrac{?}{168}$

Answer

154

Exercise $\PageIndex{32}$

$\dfrac{9}{13} = \dfrac{?}{286}$

Exercise $\PageIndex{33}$

$\dfrac{32}{33} = \dfrac{?}{1518}$

Answer

1,472

Exercise $\PageIndex{34}$

$\dfrac{19}{20} = \dfrac{1045}{?}$

Exercise $\PageIndex{35}$

$\dfrac{37}{50} = \dfrac{1369}{?}$

Answer

1,850

For the following problems, reduce, if possible, each of the fractions to lowest terms.

Exercise $\PageIndex{36}$

$\dfrac{6}{8}$

Exercise $\PageIndex{37}$

$\dfrac{8}{10}$

Answer

$\dfrac{4}{5}$

Exercise $\PageIndex{38}$

$\dfrac{5}{10}$

Exercise $\PageIndex{39}$

$\dfrac{6}{14}$

Answer

$\dfrac{3}{7}$

Exercise $\PageIndex{40}$

$\dfrac{3}{12}$

Exercise $\PageIndex{41}$

$\dfrac{4}{14}$

Answer

$\dfrac{2}{7}$

Exercise $\PageIndex{42}$

$\dfrac{1}{6}$

Exercise $\PageIndex{43}$

$\dfrac{4}{6}$

Answer

$\dfrac{2}{3}$

Exercise $\PageIndex{44}$

$\dfrac{18}{14}$

Exercise $\PageIndex{45}$

$\dfrac{20}{8}$

Answer

$\dfrac{5}{2}$

Exercise $\PageIndex{46}$

$\dfrac{4}{6}$

Exercise $\PageIndex{47}$

$\dfrac{10}{6}$

Answer

$\dfrac{5}{3}$

Exercise $\PageIndex{48}$

$\dfrac{6}{14}$

Exercise $\PageIndex{49}$

$\dfrac{14}{6}$

Answer

$\dfrac{7}{3}$

Exercise $\PageIndex{50}$

$\dfrac{10}{12}$

Exercise $\PageIndex{51}$

$\dfrac{16}{70}$

Answer

$\dfrac{8}{35}$

Exercise $\PageIndex{52}$

$\dfrac{40}{60}$

Exercise $\PageIndex{53}$

$\dfrac{20}{12}$

Answer

$\dfrac{5}{3}$

Exercise $\PageIndex{54}$

$\dfrac{32}{28}$

Exercise $\PageIndex{55}$

$\dfrac{36}{10}$

Answer

$\dfrac{18}{5}$

Exercise $\PageIndex{56}$

$\dfrac{36}{60}$

Exercise $\PageIndex{57}$

$\dfrac{12}{18}$

Answer

$\dfrac{2}{3}$

Exercise $\PageIndex{58}$

$\dfrac{18}{27}$

Exercise $\PageIndex{59}$

$\dfrac{18}{24}$

Answer

$\dfrac{3}{4}$

Exercise $\PageIndex{60}$

$\dfrac{32}{40}$

Exercise $\PageIndex{61}$

$\dfrac{11}{22}$

Answer

$\dfrac{1}{2}$

Exercise $\PageIndex{62}$

$\dfrac{27}{81}$

Exercise $\PageIndex{63}$

$\dfrac{17}{51}$

Answer

$\dfrac{1}{3}$

Exercise $\PageIndex{64}$

$\dfrac{16}{42}$

Exercise $\PageIndex{65}$

$\dfrac{39}{13}$

Answer

3

Exercise $\PageIndex{66}$

$\dfrac{44}{11}$

Exercise $\PageIndex{67}$

$\dfrac{66}{33}$

Answer

2

Exercise $\PageIndex{68}$

$\dfrac{15}{1}$

Exercise $\PageIndex{69}$

$\dfrac{15}{16}$

Answer

already reduced

Exercise $\PageIndex{70}$

$\dfrac{15}{40}$

Exercise $\PageIndex{71}$

$\dfrac{36}{100}$

Answer

$\dfrac{9}{25}$

Exercise $\PageIndex{72}$

$\dfrac{45}{32}$

Exercise $\PageIndex{73}$

$\dfrac{30}{75}$

Answer

$\dfrac{2}{5}$

Exercise $\PageIndex{74}$

$\dfrac{121}{132}$

Exercise $\PageIndex{75}$

$\dfrac{72}{64}$

Answer

$\dfrac{9}{8}$

Exercise $\PageIndex{76}$

$\dfrac{30}{105}$

Exercise $\PageIndex{77}$

$\dfrac{46}{60}$

Answer

$\dfrac{23}{30}$

Exercise $\PageIndex{78}$

$\dfrac{75}{45}$

Exercise $\PageIndex{79}$

$\dfrac{40}{18}$

Answer

$\dfrac{20}{9}$

Exercise $\PageIndex{80}$

$\dfrac{108}{76}$

Exercise $\PageIndex{81}$

$\dfrac{7}{21}$

Answer

$\dfrac{1}{3}$

Exercise $\PageIndex{82}$

$\dfrac{6}{51}$

Exercise $\PageIndex{83}$

$\dfrac{51}{12}$

Answer

$\dfrac{17}{4}$

Exercise $\PageIndex{84}$

$\dfrac{8}{100}$

Exercise $\PageIndex{85}$

$\dfrac{51}{54}$

Answer

$\dfrac{17}{18}$

Exercise $\PageIndex{86}$

A ream of paper contains 500 sheets. What frac­tion of a ream of paper is 200 sheets? Be sure to reduce.

Exercise $\PageIndex{87}$

There are 24 hours in a day. What fraction of a day is 14 hours?

Answer

$\dfrac{7}{12}$

Exercise $\PageIndex{88}$

A full box contains 80 calculators. How many calculators are in $\dfrac{1}{4}$ of a box?

Exercise $\PageIndex{89}$

There are 48 plants per flat. How many plants are there in $\dfrac{1}{3}$ of a flat?

Answer

16

Exercise $\PageIndex{90}$

A person making $18,000 per year must pay $3,960 in income tax. What fraction of this per­son's yearly salary goes to the IRS?

For the following problems, find the mistake.

Exercise $\PageIndex{91}$

$\dfrac{3}{24} = \dfrac{\cancel{3}}{\cancel{3} \cdot 8} = \dfrac{0}{8} = 0$

Answer

Should be $\dfrac{1}{8}$; the cancellation is division, so the numerator should be 1.

Exercise $\PageIndex{92}$

$\dfrac{8}{10} = \dfrac{\cancel{2} + 6}{\cancel{2} + 8} = \dfrac{6}{8} = \dfrac{3}{4}$

Exercise $\PageIndex{93}$

$\dfrac{7}{15} = \dfrac{\cancel{7}}{\cancel{7} + 8} = \dfrac{1}{8}$

Answer

Cancel factors only, not addends; $\dfrac{7}{15}$ is already reduced.

Exercise $\PageIndex{94}$

$\dfrac{6}{7} = \dfrac{\cancel{5} + 1}{\cancel{5} + 2} = \dfrac{1}{2}$

Exercise $\PageIndex{95}$

$\dfrac{\cancel{9}}{\cancel{9}} = \dfrac{0}{0} = 0$

Answer

1

Exercises for Review

Exercise $\PageIndex{96}$

Round 816 to the nearest thousand.

Exercise $\PageIndex{97}$

Perform the division: $0 \div 6$.

Answer

0

Exercise $\PageIndex{98}$

Find all the factors of 24.

Exercise $\PageIndex{99}$

Find the greatest common factor of 12 and 18.

Answer

6

Exercise $\PageIndex{100}$

Convert $\dfrac{15}{8}$ to a mixed number.