Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

6.5: Multiplication of Decimals

Last updated

Jun 8, 2024
Save as PDF
- 6.4: Addition and Subtraction of Decimals
- 6.6: Division of Decimals

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Learning Objectives

understand the method used for multiplying decimals
be able to multiply decimals
be able to simplify a multiplication of a decimal by a power of 10
understand how to use the word "of" in multiplication

The Logic Behind the Method

Consider the product of 3.2 and 1.46. Changing each decimal to a fraction, we have

$\begin{array} {rcl} {(3.2)(1.46)} & = & {3 \dfrac{2}{10} \cdot 1 \dfrac{46}{100}} \\ {} & = & {\dfrac{32}{10} \cdot \dfrac{146}{100}} \\ {} & = & {\dfrac{32 \cdot 146}{10 \cdot 100}} \\ {} & = & {\dfrac{4672}{1000}} \\ {} & = & {4 \dfrac{672}{1000}} \\ {} & = & {\text{four and six hundred seventy-two thousandths}} \\ {} & = & {4.672} \end{array}$

Thus, $(3.2)(1.46) = 4.672$

Notice that the factor

$\left \{ \begin{array} {c} {\text{3.2 has 1 decimal place,}} \\ {\text{1.46 has 2 decimal places,}} \\ {\text{and the product}} \\ {\text{4.672 has 3 decimal places.}} \end{array} \right \} 1 + 2 = 3$

Using this observation, we can suggest that the sum of the number of decimal places in the factors equals the number of decimal places in the product.

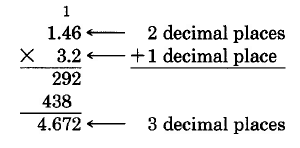
$Vertical multiplication. 1.46 times 3.2. The first round of multiplication yields a first partial product of 292. The second round yields a second partial product of 438, aligned in the tens column. Take note that 2 decimal places in the first factor and 1 decimal place in the second factor sums to a total of three decimal places in the product. The final product is 4.672.$

The Method of Multiplying Decimals

Method of Multiplying Decimals

To multiply decimals:

Multiply the numbers as if they were whole numbers.
Find the sum of the number of decimal places in the factors.
The number of decimal places in the product is the sum found in step 2.

Sample Set A

Find the following products.

$6.5 \cdot 4.3$

Solution

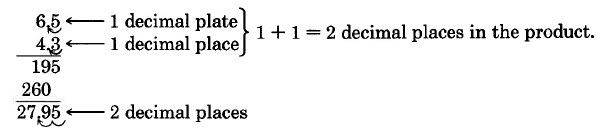
$Vertical multiplication. 6.5 times 4.3. The first round of multiplication yields a first partial product of 195. The second round yields a second partial product of 260, aligned in the tens column. Take note that 1 decimal place in the first factor and 1 decimal place in the second factor sums to a total of two decimal places in the product. The final product is 27.95.$

Thus, $6.5 \cdot 4.3 = 27.95.$

Sample Set A

$23.4 \cdot 1.96$

Solution

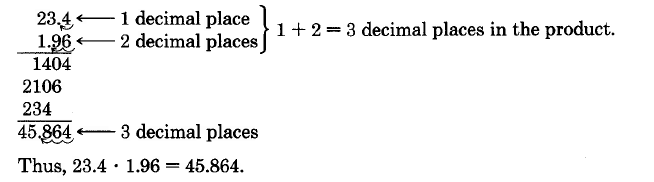
$Vertical multiplication. 23.4 times 1.96. The first round of multiplication yields a first partial product of 1404. The second round yields a second partial product of 2106, aligned in the tens column. The third round yields a third partial product of 234, aligned in the hundred column. Take note that 1 decimal place in the first factor and 2 decimal places in the second factor sums to a total of three decimal places in the product. The final product is 45.864.$

Thus, $23.4 \cdot 1.96 = 45.864.$

Sample Set A

Find the product of 0.251 and 0.00113 and round to three decimal places.

Solution

$Vertical multiplication. 0.251 times 0.00113. The first round of multiplication yields a first partial product of 753. The second round yields a second partial product of 251, aligned in the tens column. The third round yields a third partial product of 251, aligned in the hundred column. Take note that 3 decimal places in the first factor and 5 decimal places in the second factor sums to a total of eight decimal places in the product. The final product is 0.00028363.$

Now, rounding to three decimal places, we get

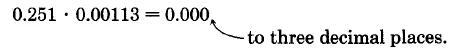
$0.251 times 0.00113 = 0.000, if the product is rounded to three decimal places.$

Practice Set A

Find the following products.

$5.3 \cdot 8.6$

Answer: 45.58

Practice Set A

$2.12 \cdot 4.9$

Answer: 10.388

Practice Set A

$1.054 \cdot 0.16$

Answer: 0.16864

Practice Set A

$0.00031 \cdot 0.002$

Answer: 0.00000062

Practice Set A

Find the product of 2.33 and 4.01 and round to one decimal place.

Answer: 9.3

Practice Set A

$10 \cdot 5.394$

Answer: 53.94

Practice Set A

$100 \cdot 5.394$

Answer: 539.4

Practice Set A

$1000 \cdot 5.394$

Answer: 5,394

Practice Set A

$10,000 \cdot 5.394$

Answer: 59,340

Calculators

Calculators can be used to find products of decimal numbers. However, a calculator that has only an eight-digit display may not be able to handle numbers or products that result in more than eight digits. But there are plenty of inexpensive ($50 - $75) calculators with more than eight-digit displays.

Sample Set B

Find the following products, if possible, using a calculator.

$2.58 \cdot 8.61$

Solution

		Display Reads
Type	2.58	2.58
Press	$\times$	2.58
Type	8.61	8.61
Press	=	22.2138

The product is 22.2138.

Sample Set B

$0.006 \cdot 0.0042$

Solution

		Display Reads
Type	.006	.006
Press	$\times$	.006
Type	.0042	0.0042
Press	=	0.0000252

We know that there will be seven decimal places in the product (since $3 + 4 = 7$ ). Since the display shows 7 decimal places, we can assume the product is correct. Thus, the product is 0.0000252.

Sample Set B

$0.0026 \cdot 0.11976$

Solution

Since we expect $4 + 5 = 9$ decimal places in the product, we know that an eight-digit display calculator will not be able to provide us with the exact value. To obtain the exact value, we must use "hand technology." Suppose, however, that we agree to round off this product to three decimal places. We then need only four decimal places on the display.

		Display Reads
Type	.0026	.0026
Press	$\times$	.0026
Type	.11976	0.11976
Press	=	0.0003114

Rounding 0.0003114 to three decimal places we get 0.000. Thus, $0.0026 \cdot 0.11976 = 0.000$ to three decimal places.

Practice Set B

Use a calculator to find each product. If the calculator will not provide the exact product, round the result to four decimal places.

$5.126 \cdot 4.08$

Answer: 20.91408

Practice Set B

$0.00165 \cdot 0.04$

Answer: 0.000066

Practice Set B

$0.5598 \cdot 0.4281$

Answer: 0.2397

Practice Set B

$0.000002 \cdot 0.06$

Answer: 0.0000

Multiplying Decimals by Powers of 10

There is an interesting feature of multiplying decimals by powers of 10. Consider the following multiplications.

Multiplication	Number of Zeros in the Power of 10	Number of Positions the Decimal Point Has Been Moved to the Right
$10 \cdot 8.315274 = 83.15274$	1	1
$100 \cdot 8.315274 = 831.5274$	2	2
$1,000 \cdot 8.315274 = 8,315.274$	3	3
$10,000 \cdot 8.315274 = 83,152.74$	4	4

Multiplying a Decimal by a Power of 10
To multiply a decimal by a power of 10, move the decimal place to the right of its current position as many places as there are zeros in the power of 10. Add zeros if necessary.

Sample Set C

Find the following products.

$100 \cdot 34.876$ . Since there are 2 zeros in 100, Move the decimal point in 34.876 two places to the right.

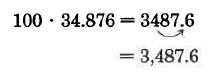
$100 times 34.876 equals 3487.6. An arrows shows how the decimal in 34.876 is moved two digits to the right to make 3,487.6$

Sample Set C

$1,000 \cdot 4.8058$ . Since there are 3 zeros in 1,000, move the decimal point in 4.8058 three places to the right.

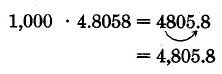
$1,000 times 4.8058 equals 4805.8. An arrows shows how the decimal in 4.8058 is moved three digits to the right to make 4,805.8$

Sample Set C

$10,000 \cdot 56.82$ . Since there are 4 zeros in 10,000, move the decimal point in 56.82 four places to the right. We will have to add two zeros in order to obtain the four places.

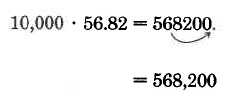
$10,000 times 56.82 equals 568200. An arrows shows how the decimal in 56.82 is moved four digits to the right to make 568,200.$

Since there is no fractional part, we can drop the decimal point.

Sample Set C

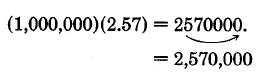
$1,000,000 times 2.57 equals 2570000. An arrows shows how the decimal in 2.57 is moved six digits to the right to make 2,570,000.$

Sample Set C

$1,000 times 0.0000029 equals 0.0029. An arrows shows how the decimal in 0.0000029 is moved six digits to the right to make 0.0029.$

Practice Set C

Find the following products.

$100 \cdot 4.27$ .

Answer: 427

Practice Set C

$10,000 \cdot 16.52187$ .

Answer: 165,218.7

Practice Set C

$(10)(0.0188)$ .

Answer: 0.188

Practice Set C

$(10,000,000,000)(52.7)$ .

Answer: 527,000,000,000

Multiplication in Terms of “Of”

Recalling that the word "of" translates to the arithmetic operation of multiplication, let's observe the following multiplications.

Sample Set D

Find 4.1 of 3.8.

Solution

Translating "of" to " $\times$ ", we get

$\begin{array} {r} {4.1} \\ {\underline{\times 3.8}} \\ {328} \\ {\underline{123\ \ }} \\ {15.58} \end{array}$

Sample Set D

Find 0.95 of the sum of 2.6 and 0.8.

Solution

We first find the sum of 2.6 and 0.8.

$\begin{array} {r} {2.6} \\ {\underline{+0.8}} \\ {3.4} \end{array}$

Now find 0.95 of 3.4

$\begin{array} {r} {3.4} \\ {\underline{\times 0.95}} \\ {170} \\ {\underline{306\ \ }} \\ {3.230} \end{array}$

Thus, 0.95 of $(2.6 + 0.8)$ is 3.230.

Practice Set D

Find 2.8 of 6.4.

Answer: 17.92

Practice Set D

Find 0.1 of 1.3.

Answer: 0.13

Practice Set D

Find 1.01 of 3.6.

Answer: 3.636

Practice Set D

Find 0.004 of 0.0009.

Answer: 0.0000036

Practice Set D

Find 0.83 of 12.

Answer: 9.96

Practice Set D

Find 1.1 of the sum of 8.6 and 4.2.

Answer: 14.08

Exercises

For the following 30 problems, find each product and check each result with a calculator.

Exercise $\PageIndex{1}$

$3.4 \cdot 9.2$

Answer: 31.28

Exercise $\PageIndex{2}$

$4.5 \cdot 6.1$

Exercise $\PageIndex{3}$

$8.0 \cdot 5.9$

Answer: 47.20

Exercise $\PageIndex{4}$

$6.1 \cdot 7$

Exercise $\PageIndex{5}$

$(0.1)(1.52)$

Answer: 0.152

Exercise $\PageIndex{6}$

$(1.99)(0.05)$

Exercise $\PageIndex{7}$

$(12.52)(0.37)$

Answer: 4.6324

Exercise $\PageIndex{8}$

$(5.116)(1.21)$

Exercise $\PageIndex{9}$

$(31.82)(0.1)$

Answer: 3.182

Exercise $\PageIndex{10}$

$(16.527)(9.16)$

Exercise $\PageIndex{11}$

$0.0021 \cdot 0.013$

Answer: 0.0000273

Exercise $\PageIndex{12}$

$1.0037 \cdot 1.00037$

Exercise $\PageIndex{13}$

$(1.6)(1.6)$

Answer: 2.56

Exercise $\PageIndex{14}$

$(4.2)(4.2)$

Exercise $\PageIndex{15}$

$0.9 \cdot 0.9$

Answer: 0.81

Exercise $\PageIndex{16}$

$1.11 \cdot 1.11$

Exercise $\PageIndex{17}$

$6.815 \cdot 4.3$

Answer: 29.3045

Exercise $\PageIndex{18}$

$9.0168 \cdot 1.2$

Exercise $\PageIndex{19}$

$(3.5162)(0.0000003)$

Answer: 0.00000105486

Exercise $\PageIndex{20}$

$(0.000001)(0.01)$

Exercise $\PageIndex{21}$

$(10)(4.96)$

Answer: 49.6

Exercise $\PageIndex{22}$

$(10)(36.17)$

Exercise $\PageIndex{23}$

$10 \cdot 421.8842$

Answer: 4,218.842

Exercise $\PageIndex{24}$

$10 \cdot 8.0107$

Exercise $\PageIndex{25}$

$100 \cdot 0.19621$

Answer: 19.621

Exercise $\PageIndex{26}$

$100 \cdot 0.779$

Exercise $\PageIndex{27}$

$1000 \cdot 3.596168$

Answer: 3,596.168

Exercise $\PageIndex{28}$

$1000 \cdot 42.7125571$

Exercise $\PageIndex{29}$

$1000 \cdot 25.01$

Answer: 25,010

Exercise $\PageIndex{30}$

$100,000 \cdot 9.923$

Exercise $\PageIndex{31}$

$(4.6)(6.17)$

Actual product	Tenths	Hundreds	Thousandths

Answer

Actual product	Tenths	Hundreds	Thousandths
28.382	28.4	28.38	28.382

Exercise $\PageIndex{32}$

$(8.09)(7.1)$

Actual product	Tenths	Hundreds	Thousandths

Exercise $\PageIndex{33}$

$(11.1106)(12.08)$

Actual product	Tenths	Hundreds	Thousandths

Answer

Actual product	Tenths	Hundreds	Thousandths
134.216048	134.2	134.22	134.216

Exercise $\PageIndex{34}$

$0.0083 \cdot 1.090901$

Actual product	Tenths	Hundreds	Thousandths

Exercise $\PageIndex{35}$

$7 \cdot 26.518$

Actual product	Tenths	Hundreds	Thousandths

Answer

Actual product	Tenths	Hundreds	Thousandths
185.626	185.6	185.63	185.626

For the following 15 problems, perform the indicated operations

Exercise $\PageIndex{36}$

Find 5.2 of 3.7.

Exercise $\PageIndex{37}$

Find 12.03 of 10.1

Answer: 121.503

Exercise $\PageIndex{38}$

Find 16 of 1.04

Exercise $\PageIndex{39}$

Find 12 of 0.1

Answer: 1.2

Exercise $\PageIndex{40}$

Find 0.09 of 0.003

Exercise $\PageIndex{41}$

Find 1.02 of 0.9801

Answer: 0.999702

Exercise $\PageIndex{42}$

Find 0.01 of the sum of 3.6 and 12.18

Exercise $\PageIndex{43}$

Find 0.2 of the sum of 0.194 and 1.07

Answer: 0.2528

Exercise $\PageIndex{44}$

Find the difference of 6.1 of 2.7 and 2.7 of 4.03

Exercise $\PageIndex{45}$

Find the difference of 0.071 of 42 and 0.003 of 9.2

Answer: 2.9544

Exercise $\PageIndex{46}$

If a person earns $8.55 an hour, how much does he earn in twenty-five hundredths of an hour?

Exercise $\PageIndex{47}$

A man buys 14 items at $1.16 each. What is the total cost?

Answer: $16.24

Exercise $\PageIndex{48}$

In the problem above, how much is the total cost if 0.065 sales tax is added?

Exercise $\PageIndex{49}$

A river rafting trip is supposed to last for 10 days and each day 6 miles is to be rafted. On the third day a person falls out of the raft after only $\dfrac{2}{5}$ of that day’s mileage. If this person gets discouraged and quits, what fraction of the entire trip did he complete?

Answer: 0.24

Exercise $\PageIndex{50}$

A woman starts the day with $42.28. She buys one item for $8.95 and another for $6.68. She then buys another item for sixty two-hundredths of the remaining amount. How much money does she have left?

Calculator Problems
For the following 10 problems, use a calculator to determine each product. If the calculator will not provide the exact product, round the results to five decimal places.

Exercise $\PageIndex{51}$

$0.019 \cdot 0.321$

Answer: 0.006099

Exercise $\PageIndex{52}$

$0.261 \cdot 1.96$

Exercise $\PageIndex{53}$

$4.826 \cdot 4.827$

Answer: 23.295102

Exercise $\PageIndex{54}$

$(9.46)^2$

Exercise $\PageIndex{55}$

$(0.012)^2$

Answer: 0.000144

Exercise $\PageIndex{56}$

$0.00037 \cdot 0.0065$

Exercise $\PageIndex{57}$

$0.002 \cdot 0.0009$

Answer: 0.0000018

Exercise $\PageIndex{58}$

$0.1286 \cdot 0.7699$

Exercise $\PageIndex{59}$

$0.01 \cdot 0.00000471$

Answer: 0.0000000471

Exercise $\PageIndex{60}$

$0.00198709 \cdot 0.03$

Exercises for Review

Exercise $\PageIndex{61}$

Find the value, if it exists, of $0 \div 15$ .

Answer: 0

Exercise $\PageIndex{62}$

Find the greatest common factor of 210, 231, and 357.

Exercise $\PageIndex{63}$

Reduce $\dfrac{280}{2,156}$ to lowest terms.

Answer: $\dfrac{10}{77}$

Exercise $\PageIndex{64}$

Write "fourteen and one hundred twenty-one ten-thousandths, using digits."

Exercise $\PageIndex{65}$

Subtract 6.882 from 8.661 and round the result to two decimal places.

Answer: 1.78

Learning Objectives

The Logic Behind the Method

The Method of Multiplying Decimals

Method of Multiplying Decimals

Sample Set A

Sample Set A

Sample Set A

Calculators

Sample Set B

Sample Set B

Sample Set B

Multiplying Decimals by Powers of 10

Sample Set C

Sample Set C

Sample Set C

Sample Set C

Sample Set C

Multiplication in Terms of “Of”

Sample Set D

Sample Set D

Exercises

Exercises for Review

Support Center

How can we help?