Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

1.E: Whole Numbers (Exercises)

Last updated

May 2, 2022
Save as PDF
- 1.9: Divide Whole Numbers (Part 1)
- 1.S: Whole Numbers (Summary)

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

1.1 - Introduction to Whole Numbers

Identify Counting Numbers and Whole Numbers

In the following exercises, determine which of the following numbers are (a) counting numbers (b) whole numbers.

0, 2, 99
0, 3, 25
0, 4, 90
0, 1, 75

Model Whole Numbers

In the following exercises, model each number using base-10 blocks and then show its value using place value notation.

Identify the Place Value of a Digit

In the following exercises, find the place value of the given digits.

472,981

(a) 8 (b) 4 (c) 1 (d) 7 (e) 2

12,403,295

(a) 4 (b) 0 (c) 1 (d) 9 (e) 3

Use Place Value to Name Whole Numbers

In the following exercises, name each number in words.

5,280
204,614
5,012,582
31,640,976

Use Place Value to Write Whole Numbers

In the following exercises, write each number as a whole number using digits.

six hundred two
fifteen thousand, two hundred fifty-three
three hundred forty million, nine hundred twelve thousand, sixty-one
two billion, four hundred ninety-two million, seven hundred eleven thousand, two

Round Whole Numbers

In the following exercises, round to the nearest ten.

412
648
3,556
2,734

In the following exercises, round to the nearest hundred.

38,975
26,849
81,486
75,992

1.2 - Add Whole Numbers

Use Addition Notation

In the following exercises, translate the following from math notation to words.

4 + 3
25 + 18
571 + 629
10,085 + 3,492

Model Addition of Whole Numbers

In the following exercises, model the addition.

6 + 7
38 + 14

Add Whole Numbers

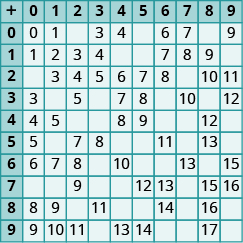
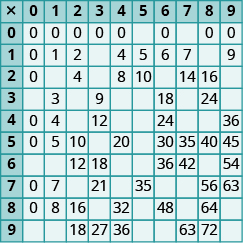
In the following exercises, fill in the missing values in each chart.

$A table with 11 rows down and 11 rows across. The first row and first column are headers and include the numbers 0 through 9 both across and down, with a plus sign in the first cell. The numbers across in the second row down appear as follows: 0,0, 1, null, 3, 4, null, 6, 7, null, 9. The numbers across in the third row down appear as follows: 1, 1, 2, 3, 4, null, null, 7, 8, 9, null. The numbers in the fourth row down appear across as follows: 2, null, 3,4,5,6,7,8, null, 10, 11. The numbers across in the fifth row down appear as follows: 3, 3, null, 5, null, 7, 8, null, 10, null 12. The numbers across in the sixth row down appear as follows: 4, 4, 5, null, null, 8, 9,null, null, 12, null. The numbers across in the seventh row down appear as follows: 5, 5, null, 7, 8, null null, 11, null, 13, null. The numbers across in the eighth row down appear as follows:6, 6, 7, 8, null, 10, null, null, 13, null, 15. The numbers across in the ninth row down appear as follows: null, null, 9, null, null, 12, 13, null, 15, 16. The numbers across in the tenth row down appear as follows: 8,8,9,null, 11, null, null, 14, null, eleventh row down appear as follows: 9, 9, 10, 11, null, 13, 14, null, null, 17, null.$
$This table is 5 rows and 8 columns. The top row is a header row and includes the numbers 3 through 9, one number to each cell. The rows down include 6, 7, 8, and 9. There is a plus sign in the first cell. All cells are null.$

In the following exercises, add.

(a) 0 + 19 (b) 19 + 0
(a) 0 + 480 (b) 480 + 0
(a) 7 + 6 (b) 6 + 7
(a) 23 + 18 (b) 18 + 23
44 + 35
63 + 29
96 + 58
375 + 591
7,281 + 12,546
5,280 + 16,324 + 9,731

Translate Word Phrases to Math Notation

In the following exercises, translate each phrase into math notation and then simplify.

the sum of 30 and 12
11 increased by 8
25 more than 39
total of 15 and 50

Add Whole Numbers in Applications

In the following exercises, solve.

Shopping for an interview Nathan bought a new shirt, tie, and slacks to wear to a job interview. The shirt cost $24, the tie cost $14, and the slacks cost $38. What was Nathan’s total cost?
Running Jackson ran 4 miles on Monday, 12 miles on Tuesday, 1 mile on Wednesday, 8 miles on Thursday, and 5 miles on Friday. What was the total number of miles Jackson ran?

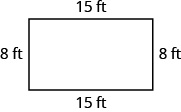
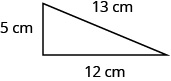
In the following exercises, find the perimeter of each figure.

$An image of a rectangle that is 8 feet tall and 15 feet wide.$
$An image of a right triangle that has a base of 12 centimeters, height of 5 centimeters, and diagonal hypotenuse of 13 centimeters.$

1.3 - Subtract Whole Numbers

Use Subtraction Notation

In the following exercises, translate the following from math notation to words.

14 − 5
40 − 15
351 − 249
5,724 − 2,918

Model Subtraction of Whole Numbers

In the following exercises, model the subtraction.

18 − 4
41 − 29

Subtract Whole Numbers

In the following exercises, subtract and then check by adding.

8 − 5
12 − 7
23 − 9
. 46 − 21
82 − 59
110 − 87
539 − 217
415 − 296
1,020 − 640
8,355 − 3,947
10,000 − 15
54,925 − 35,647

Translate Word Phrases to Math Notation

In the following exercises, translate and simplify.

the difference of nineteen and thirteen
subtract sixty-five from one hundred
seventy-four decreased by eight
twenty-three less than forty-one

Subtract Whole Numbers in Applications

In the following exercises, solve.

Temperature The high temperature in Peoria one day was 86 degrees Fahrenheit and the low temperature was 28 degrees Fahrenheit. What was the difference between the high and low temperatures?
Savings Lynn wants to go on a cruise that costs $2,485. She has $948 in her vacation savings account. How much more does she need to save in order to pay for the cruise?

1.4 - Multiply Whole Numbers

Use Multiplication Notation

In the following exercises, translate from math notation to words.

8 × 5
6 • 14
(10)(95)
54(72)

Model Multiplication of Whole Numbers

In the following exercises, model the multiplication.

2 × 4
3 × 8

Multiply Whole Numbers

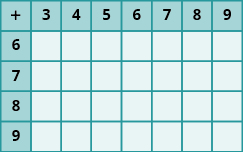
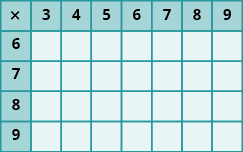
In the following exercises, fill in the missing values in each chart.

$A table with 10 rows down and 10 rows across. The first row and first column are headers and include the numbers 0 through 9 both across and down, with a plus sign in the first cell. The numbers across in the second row down appear as follows: 0, 0, 0, 0, 0, 0, null, 0, null, 0,0. The numbers across in the third row down appear as follows: 1, 0, 1, 2, null, 4, 5, 6, 7, null, 9. The numbers across in the fourth row down appear as follows: 2, 0, null, 4, null, 8, 10, null, 14, 16, null. The numbers across in the fifth row down appear as follows: 3, null, 3, null, 9, null, null, 18, null, 24, null. The numbers across in the sixth row down appear as follows: 4, 0, 4, 0, 12, null, null, 24, null, null, 36. The numbers across in the seventh row down appear as follows:5, 0, 5, 10, null, 20, null, 30, 35, 40, 45. The numbers across in the eighth row down appear as follows: 6, null, null, 12, 18, null, null, 36, 42, null, 54. The numbers across in the ninth row down appear as follows: 7, 0, 7, null, 21, null, 35, null, null, 56, 63. The numbers in the tenth row down appear as follows: 8, 0, 8, 16, null, 32, null, 48, null, 64, null. The numbers in the eleventh row down appear across as follows: 9, null, null, 18, 27, 36, null, null, 63, 72, null.$
$An image of a table with 8 columns and 5 rows. The cells in the first row and first column are shaded darker than the other cells. The first row has the values “x; 3; 4; 5; 6; 7; 8; 9”. The first column has the values “x; 6; 7; 8; 9”. All other cells are null.$

In the following exercises, multiply.

0 • 14
(256)0
1 • 99
(4,789)1
(a) 7 • 4 (b) 4 • 7
(25)(6)
9,261 × 3
48 • 76
64 • 10
1,000(22)
162 × 493
(601)(943)
3,624 × 517
10,538 • 22

Translate Word Phrases to Math Notation

In the following exercises, translate and simplify.

the product of 15 and 28
ninety-four times thirty-three
twice 575
ten times two hundred sixty-four

Multiply Whole Numbers in Applications

In the following exercises, solve.

Gardening Geniece bought 8 packs of marigolds to plant in her yard. Each pack has 6 flowers. How many marigolds did Geniece buy?
Cooking Ratika is making rice for a dinner party. The number of cups of water is twice the number of cups of rice. If Ratika plans to use 4 cups of rice, how many cups of water does she need?
Multiplex There are twelve theaters at the multiplex and each theater has 150 seats. What is the total number of seats at the multiplex?
Roofing Lewis needs to put new shingles on his roof. The roof is a rectangle, 30 feet by 24 feet. What is the area of the roof?

1.5 - Divide Whole Numbers

Use Division Notation

Translate from math notation to words.

54 ÷ 9
42 / 7
$\dfrac{72}{8}$
$6 \overline{\smash{)}48}$

Model Division of Whole Numbers

In the following exercises, model.

8 ÷ 2
$3 \overline{\smash{)}12}$

Divide Whole Numbers

In the following exercises, divide. Then check by multiplying.

14 ÷ 2
$\dfrac{32}{8}$
52 ÷ 4
$26 \overline{\smash{)}26}$
$\dfrac{97}{1}$
0 ÷ 52
100 ÷ 0
$\dfrac{355}{5}$
3828 ÷ 6
$31 \overline{\smash{)}1,519}$
$\dfrac{7505}{25}$
5,166 ÷ 42

Translate Word Phrases to Math Notation

In the following exercises, translate and simplify.

the quotient of 64 and 16
the quotient of 572 and 52

Divide Whole Numbers in Applications

In the following exercises, solve.

Ribbon One spool of ribbon is 27 feet. Lizbeth uses 3 feet of ribbon for each gift basket that she wraps. How many gift baskets can Lizbeth wrap from one spool of ribbon?
Juice One carton of fruit juice is 128 ounces. How many 4 ounce cups can Shayla fill from one carton of juice?

PRACTICE TEST

Determine which of the following numbers are (a) counting numbers (b) whole numbers. $0, 4, 87$
Find the place value of the given digits in the number 549,362.

(a) 9 (b) 6 (c) 2 (d) 5

Write each number as a whole number using digits.

(a) six hundred thirteen (b) fifty-five thousand two hundred eight

Round 25,849 to the nearest hundred.

Simplify.

45 + 23
65 − 42
85 ÷ 5
1,000 × 8
90 − 58
73 + 89
(0)(12,675)
634 + 255
$\dfrac{0}{9}$
$8 \overline{\smash{)}128}$
145 − 79
299 + 836
7 • 475
8,528 + 704
35(14)
$\dfrac{26}{0}$
733 − 291
4,916 − 1,538
495 ÷ 45
52 × 983

Translate each phrase to math notation and then simplify.

The sum of 16 and 58
The product of 9 and 15
The difference of 32 and 18
The quotient of 63 and 21
Twice 524
29 more than 32
50 less than 300

In the following exercises, solve.

LaVelle buys a jumbo bag of 84 candies to make favor bags for her son’s party. If she wants to make 12 bags, how many candies should she put in each bag?
Last month, Stan’s take-home pay was $3,816 and his expenses were $3,472. How much of his take-home pay did Stan have left after he paid his expenses?
Each class at Greenville School has 22 children enrolled. The school has 24 classes. How many children are enrolled at Greenville School?
Clayton walked 12 blocks to his mother’s house, 6 blocks to the gym, and 9 blocks to the grocery store before walking the last 3 blocks home. What was the total number of blocks that Clayton walked?

1.1 - Introduction to Whole Numbers

Identify Counting Numbers and Whole Numbers

Model Whole Numbers

Identify the Place Value of a Digit

Use Place Value to Name Whole Numbers

Use Place Value to Write Whole Numbers

Round Whole Numbers

1.2 - Add Whole Numbers

Use Addition Notation

Model Addition of Whole Numbers

Add Whole Numbers

Translate Word Phrases to Math Notation

1.3 - Subtract Whole Numbers

Use Subtraction Notation

Model Subtraction of Whole Numbers

Subtract Whole Numbers

Translate Word Phrases to Math Notation

Subtract Whole Numbers in Applications

1.4 - Multiply Whole Numbers

Use Multiplication Notation

Model Multiplication of Whole Numbers

Multiply Whole Numbers

Translate Word Phrases to Math Notation

Multiply Whole Numbers in Applications

1.5 - Divide Whole Numbers

Use Division Notation

Model Division of Whole Numbers

Divide Whole Numbers

Translate Word Phrases to Math Notation

Divide Whole Numbers in Applications

PRACTICE TEST

Contributors and Attributions

Support Center

How can we help?