11.0: Triangles and Circles
- Page ID
- 122906
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)1.1 Angles and Triangles
Homework 1.1
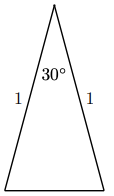
1. 
3. 
5. 
7. \(\theta = 108.8^{\circ}\)
9. \(\alpha = 29^{\circ}\)
11. \(\beta = 77^{\circ}\)
13. \(\alpha = 12^{\circ}\)
15. \(\theta = 65^{\circ}\)
17. \(\theta = 22^{\circ}\)
19. \(\psi = 73^{\circ}\)
21. \(\phi = 88^{\circ}\)
23.
a \(\phi = 120^{\circ}\)
b \(\phi=160^{\circ}\)
c \(\phi=\alpha+\beta\)
d An exterior angle is equal to the sum of the opposite interior angles.
25. \(\theta=72^{\circ}, \phi=54^{\circ}\)
27. \(\theta=100^{\circ}, \phi=30^{\circ}\)
29.
a \(180^{\circ}\)
b \(90^{\circ}\)
c a right triangle
31.
a \(180^{\circ}\)
b \(90^{\circ}\)
c a right triangle
33. \(\alpha=30^{\circ}, \beta=60^{\circ}\)
35. \(x=47^{\circ}, y=133^{\circ}\)
37. \(x=60^{\circ}, y=15^{\circ}\)
39. \(x=25^{\circ}, y=16^{\circ}\)
41. \(x=90^{\circ}, y=55^{\circ}\)
43. \(x=50^{\circ}, y=80^{\circ}\)
45.
a \(\angle 1=\angle 4, \angle 3=\angle 5\)
b \(180^{\circ}\)
c In the equation \(\angle 4+\angle 2+\angle 5=180^{\circ}\), substitute \(\angle 1\) for \(\angle 4\), and substitute \(\angle 3\) for \(\angle 5\) to conclude that the sum of the angles in the triangle is \(180^{\circ}\).
47. \(\angle 1=130^{\circ}\) because vertical angles are equal. \(\angle 2=50^{\circ}\) because it makes a straight angle with a \(130^{\circ}\) angle. \(\angle 3=65^{\circ}\) because it is a base angle of an isosceles triangle whose vertex angle is \(50^{\circ}\). \(\angle 41=65^{\circ}\) for the same reason. \(\angle 5=25^{\circ}\) because it is complementary to \(\angle 4\).
1.2 Similar Triangles
Homework 1.2
1. \(\triangle P Q T \simeq \triangle S R T, x=7, y=3, \alpha=18^{\circ}\)
3. \(\triangle P R E \simeq \triangle U R N, z=12, \theta=10^{\circ}, \phi=70^{\circ}\)
5. 
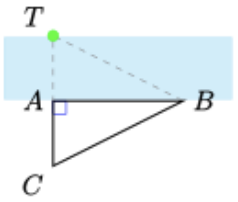\(\triangle ABT \simeq \triangle ABC, \text{ so } AT = AC\)
7. Similar. Corresponding sides are proportional.
9. Similar. Corresponding angles are equal.
11. \(\angle A=37^{\circ}, \angle B=37^{\circ}\)
13. \(h=12\)
15. \(p = 35\)
17. \(g = 84\)
19. \(h = 30\)
21. 154 feet
23. 1 mile
25. 17.1 square feet
27. \(y=\dfrac{12}{17} x\)
29. \(h = 7.5\)
31. \(c = 15\)
33. \(s = 6\)
35. \(y=\dfrac{3}{5} x\)
37. \(y=5+\dfrac{3}{4} x\)
39.
a \(\angle B=70^{\circ}, \angle C A D=70^{\circ}, \angle D A B=20^{\circ}\)
b \(\triangle D B A\) and \(\triangle D B C\). The hypotenuse is \(B C\) in \(\triangle A B C, B A\) in \(\triangle D B A\), and \(A C\) in \(\triangle D A C\). The short leg is \(A B\) in \(\triangle A B C, D B\) in \(\triangle D B A\), and \(D A\) in \(\triangle D A C\). The longer leg is \(A C\) in \(\triangle A B C, D A\) in \(\triangle D B A\), and \(D C\) in \(\triangle D A C\).
1.3 Circles
Homework 1.3
1. 13 miles
3. 10, 10.0
5. \(4 \sqrt{5} \approx 8.94\)
7. 5
9. \(2 \sqrt{5}\)
13. 
24.7
15.
a \(\sqrt{(x+3)^2+(y-4)^2}\)
b \(\sqrt{(x+3)^2+(y-4)^2}=5\)
17. The distance between the points \((x, y)\) and (4,-1) is 3 units.
19.
a \(6 \sqrt{2} \mathrm{~cm}\)
b \(8.49 \mathrm{~cm}\)
21.
a \(25 \pi \mathrm{sq}\) in
b \(78.54 \mathrm{sq}\) in
23.
a approximation
b approximation
c approximation
d exact
25.
a
| \(x\) | -5 | -4 | -3 | -2 | -1 | 0 | 1 | 2 | 3 | 4 | 5 |
| \(y\) | 0 | \(\pm 3\) | \(\pm 4\) | \(\pm \sqrt{21}\) | \(\pm 2\sqrt{6}\) | \(\pm 5\) | \(\pm 2 \sqrt{6}\) | \(\pm \sqrt{21}\) | \(\pm 4\) | \(\pm 3\) | 0 |
b 
27.
a 
b \(x^2+y^2 = 36\)
29.
a 
b \(x^2+y^2 < 9\)
31.
a No real value of \(y\) can satisfy \(x^2+y^2 = 16\) unless \(-4 \leq x \leq 4\)
b The graph has no points where \(x > 4\) and no points where \(x < -4\)
33. \(\sqrt{10}\)
35.
a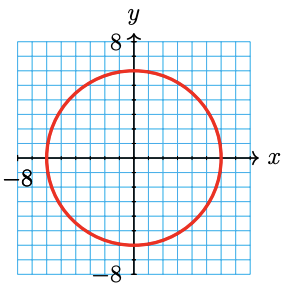 
b \(12 \pi\)
37.
a 
b \(4\pi\)
39. \( (-2\sqrt{5}, -4), (2\sqrt{5}, -4)\)
41. \(P\left(\dfrac{1}{2}, \dfrac{\sqrt{3}}{2}\right), Q\left(\dfrac{1}{2}, \dfrac{-\sqrt{3}}{2}\right), R\left(\dfrac{-3}{4}, \frac{\sqrt{7}}{4}\right), S\left(\dfrac{-3}{4}, \dfrac{-\sqrt{7}}{4}\right)\)
43.
a \(45^{\circ}\)
b \(5 \pi \mathrm{ft}\)
c \(50 \pi \mathrm{sq} \mathrm{ft}\)
45.
a \(\dfrac{2}{5}\)
b \(40 \pi \mathrm{sq} \mathrm{ft}\)
c \(8 \pi \mathrm{ft}\)
46.
a \(\dfrac{1}{10}\)
b \(\dfrac{\pi}{10} \mathrm{sq} \mathrm{km}\)
c \(\dfrac{\pi}{5} \mathrm{~km}\)
47.
a \(\dfrac{5}{6}\)
b \(\dfrac{15 \pi}{2}\) sq m
c \(5 \pi \mathrm{m}\)
51. 2070 miles
53.
a 54,000 miles
b 2240 mph
55.
a \((x-3)^2+(y+2)^2=36\)
b \((x-h)^2+(y-k)^2=r^2\)
1.4 Chapter 1 Summary and Review
Chapter 1 Review Problems
1. 
3. 
5. \(\alpha = \beta = \gamma = 60^{\circ}\)
7. \(\phi = \omega = 79^{\circ}\)
9. \(\theta = 65^{\circ}, \phi = 25^{\circ}\)
11. \(\delta = 30^{\circ}, \gamma = 60^{\circ}\)
13. \(\sigma = 39^{\circ}, \omega = 79^{\circ}\)
15. \(\alpha = 51 \dfrac{3}{7}^{\circ}, \beta = 64 \dfrac{2}{7}^{\circ}\)
17. \(\triangle A B C \cong \triangle E D C, \alpha=40^{\circ}, \beta=130^{\circ}, x=32\)
19. Yes, three pairs of equal angles
21. Yes, three pairs of equal angles
23. 13
25. 18
27. \(y = \dfrac{5x}{2}\)
29. \(y=\dfrac{7x}{3}\)
31. \(y = \dfrac{x}{3}\)
33. \(x = \dfrac{25}{13}, y = \dfrac{60}{13}\)
35. \(\alpha = 70^{\circ}\)
37. 14 ft
39. \(3\dfrac{3}{4}\)in
41. All side have length \(\sqrt{61}\), opposite sides have slopes \(\frac{5}{6}\) and \(\frac{-6}{5}\)
43. \(AC = BC = 18\)
45.
a \(\sqrt{(x-2)^2+(y-5)^2}=3\)
b \((x-2)^2+(y-5)^2=9\)
47. \(4 \sqrt{5} \approx 8.944 \mathrm{~cm}\)
49. \(\left(\dfrac{-1}{3}, \dfrac{2 \sqrt{2}}{3}\right),\left(\dfrac{-1}{3}, \dfrac{-2 \sqrt{2}}{3}\right)\)
51.
a \(4 \pi \mathrm{ft}\)
b \(20 \pi \mathrm{ft}^2\)
53.
a \(45^{\circ}, 60^{\circ}\)
b \(\dfrac{49 \pi}{8}\) in \(^2, 6 \pi\) in \(^2\) Delbert
c \(\dfrac{79 \pi}{4}\) in, \(2 \pi\) in, Francine