11.3: Trigonometric Functions

4.1 Angles and Rotation

Homework 4.1

1.

a $216^{\circ}$

b $108^{\circ}$

c $480^{\circ}$

d $960^{\circ}$

3.

a $\dfrac{1}{8}$

b $\dfrac{5}{6}$

c $\dfrac{3}{2}$

d $\dfrac{7}{6}$

5.

a $\dfrac{2}{3}$

b $\dfrac{5}{3}$

7. $60^{\circ}$

9. $60^{\circ}$

11. $14^{\circ}$

13. $400^{\circ} \text{ and } -320^{\circ}$ (Answers vary.)

15. $575^{\circ} \text{ and } -145^{\circ}$ (Answers vary.)

17. $665^{\circ} \text{ and } -55^{\circ}$ (Answers vary.)

19. $295^{\circ}$

21. $70^{\circ}$

23. $315^{\circ}$

25. $80^{\circ}$

27. $36^{\circ}$

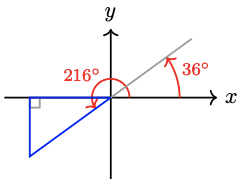
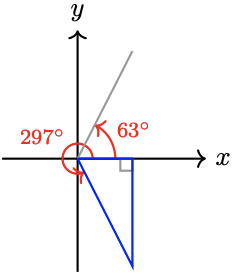
29. $63^{\circ}$

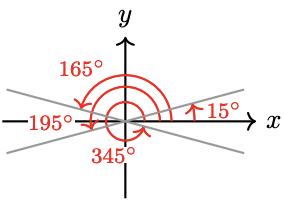
31. $165^{\circ}, 95^{\circ}, 345^{\circ}$

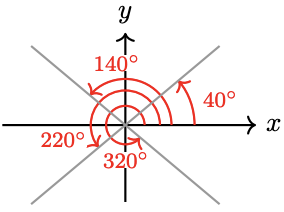
33. $140^{\circ}, 220^{\circ}, 320^{\circ}$

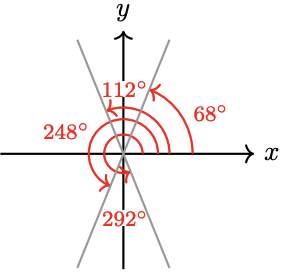
35. $112^{\circ}, 248^{\circ}, 292^{\circ}$

37. −0.9205

39. −0.7193

41. 4.705

43. −0.7193

45.

a $120^{\circ}$

b $135^{\circ}$

c $150^{\circ}$

d $210^{\circ}$

e $225^{\circ}$

f $240^{\circ}$

g $300^{\circ}$

h $315^{\circ}$

i $330^{\circ}$

47.

a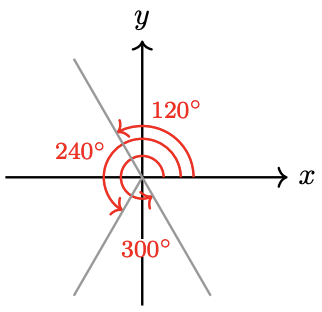

b

$$\begin{aligned} \mathrm{b} \sin 120^{\circ} & =\dfrac{\sqrt{3}}{2}, \cos 120^{\circ}=\dfrac{-1}{2}, \tan 120^{\circ}=-\sqrt{3}, \\ \sin 240^{\circ} & =\dfrac{-\sqrt{3}}{2}, \cos 240^{\circ}=\dfrac{-1}{2}, \tan 240^{\circ}=\sqrt{3}, \\ \sin 300^{\circ} & =\dfrac{-\sqrt{3}}{2}, \cos 300^{\circ}=\dfrac{1}{2}, \tan 300^{\circ}=-\sqrt{3} \end{aligned}$$

49.

a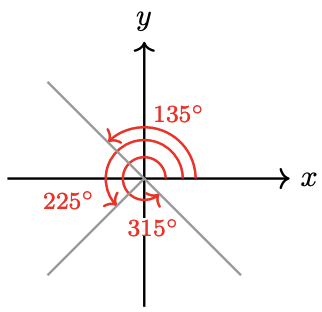

b

$$\begin{aligned} \text { b } \sin 135^{\circ} & =\dfrac{1}{\sqrt{2}}, \quad \cos 135^{\circ}=\dfrac{-1}{\sqrt{2}}, \quad \tan 135^{\circ}=-1, \\ \sin 225^{\circ} & =\dfrac{-1}{\sqrt{2}}, \quad \cos 225^{\circ}=\dfrac{-1}{\sqrt{2}}, \quad \tan 225^{\circ}=1, \\ \sin 315^{\circ} & =\dfrac{-1}{\sqrt{2}}, \quad \cos 315^{\circ}=\dfrac{1}{\sqrt{2}}, \quad \tan 315^{\circ}=-1 \end{aligned}$$

51.

a III and IV

b II and III

c I and III

53.

a $0^{\circ}$ and $180^{\circ}$

b $90^{\circ}$ and $270^{\circ}$

55. $105^{\circ}$

57. $264^{\circ}$

59. $313^{\circ}$

61. $83^{\circ}, 263^{\circ}$

63. $23^{\circ}, 337^{\circ}$

65. $265^{\circ}, 275^{\circ}$

67. $156^{\circ}, 204^{\circ}$

69. $246^{\circ}, 294^{\circ}$

71. $149^{\circ}, 329^{\circ}$

73. $(-2\sqrt{2}, 2\sqrt{2})$

75. $(\dfrac{3}{2}, \dfrac{3\sqrt{3}}{2})$

77. $(\dfrac{-\sqrt{3}}{2}, \dfrac{-1}{2})$

79.

a (−0.9, −0.3)

b (−0.940, −0.342)

c (−1.9, −0.7)

81.

a (−0.9, 0.3)

b (−0.940, 0.342)

c (−1.9, 0.7)

83. Sides of similar triangles are proportional.

4.2 Graphs of Trigonometric Functions

Homework 4.2

1. $(4 \sqrt{2},-4 \sqrt{2})$

3. $(-10,-10 \sqrt{3})$

5. $\left(\dfrac{-15 \sqrt{3}}{2}, \dfrac{15}{2}\right)$

7. $(-1.25,-5.87)$

9. $(5.70,-11.86)$

11. $(9.46,-3.26)$

13.

a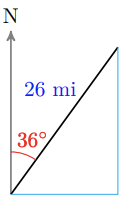

b 15.3 mi east, 21 mi north

15.

a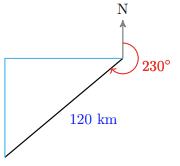

b 91.9 km west, 77.1 km south

17.

a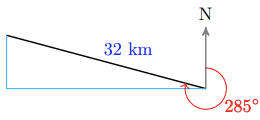

b 30.9 km west, 8.3 km north

19.

Angle	$0^{\circ}$	$10^{\circ}$	$20^{\circ}$	$30^{\circ}$	$40^{\circ}$	$50^{\circ}$	$60^{\circ}$	$70^{\circ}$	$80^{\circ}$	$90^{\circ}$
$x$-coordinate	1	0.98	0.94	0.97	0.77	0.64	0.5	0.34	0.17	0

Angle	$100^{\circ}$	$110^{\circ}$	$120^{\circ}$	$130^{\circ}$	$140^{\circ}$	$150^{\circ}$	$160^{\circ}$	$170^{\circ}$	$180^{\circ}$
$x$-coordinate	-0.17	-0.34	-0.5	-0.64	-0.77	-0.87	-0.94	-0.98	-1

Angle	$190^{\circ}$	$200^{\circ}$	$210^{\circ}$	$220^{\circ}$	$230^{\circ}$	$240^{\circ}$	$250^{\circ}$	$260^{\circ}$	$270^{\circ}$
$x$-coordinate	-0.98	-0.94	-0.87	-0.77	-0.64	-0.5	-0.34	-0.17	0

Angle	$280^{\circ}$	$290^{\circ}$	$300^{\circ}$	$310^{\circ}$	$320^{\circ}$	$330^{\circ}$	$340^{\circ}$	$350^{\circ}$	$360^{\circ}$
$x$-coordinate	0.17	0.34	0.5	0.64	0.77	0.87	0.94	0.98	1

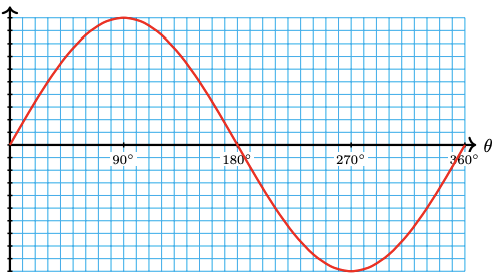
21.

23.

25.

27.

a $\left(-225^{\circ}, \dfrac{1}{\sqrt{2}}\right)$

b $\left(-135^{\circ}, \dfrac{-1}{\sqrt{2}}\right)$

c $\left(-90^{\circ},-1\right)$

d $\left(45^{\circ}, \dfrac{1}{\sqrt{2}}\right)$

e $\left(180^{\circ}, 0\right)$

f $\left(315^{\circ}, \dfrac{-1}{\sqrt{2}}\right)$

29.

a $\left(-240^{\circ}, \dfrac{-1}{2}\right)$

b $\left(-210^{\circ}, \dfrac{-\sqrt{3}}{2}\right)$

c $\left(-60^{\circ}, \dfrac{-1}{2}\right)$

d $\left(30^{\circ}, \dfrac{\sqrt{3}}{2}\right)$

e $\left(120^{\circ}, \dfrac{-1}{2}\right)$

f $\left(270^{\circ}, 0\right)$

30.

a

$\theta$	$0^{\circ}$	$90^{\circ}$	$180^{\circ}$	$270^{\circ}$	$360^{\circ}$
$f(\theta)$	0	1	0	-1	0

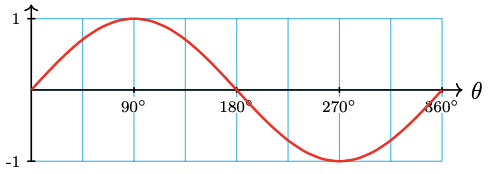
b

$\theta$	$0^{\circ}$	$90^{\circ}$	$180^{\circ}$	$270^{\circ}$	$360^{\circ}$
$f(\theta)$	1	0	-1	0	1

33. $\dfrac{7}{2}$

35. $-2\sqrt{2} - 1$

37. 2

39. $\dfrac{21}{2}$

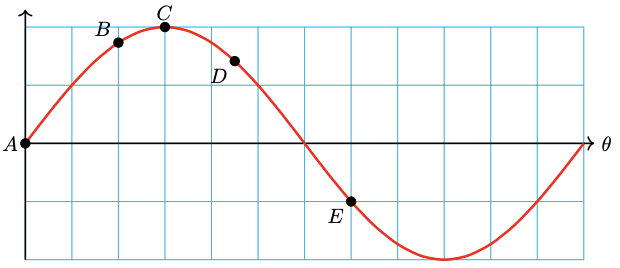
41.

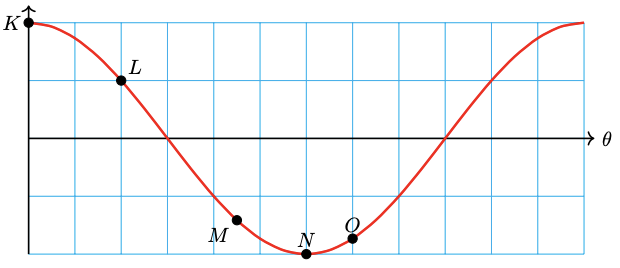
43.

45.

a $36.9^{\circ}, 143.1^{\circ}$

b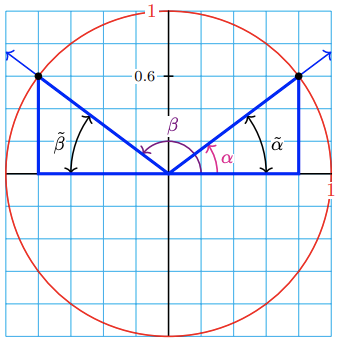

47.

a $72.5^{\circ}, 287.5^{\circ}$

b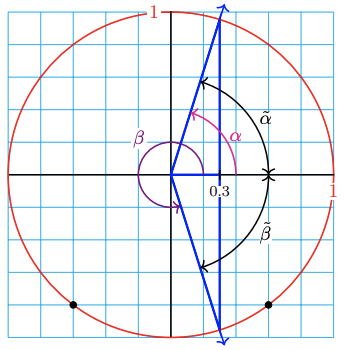

49. $36.9^{\circ}, 143.1^{\circ}$

51. $72.5^{\circ}, 287.5^{\circ}$

53. $191.5^{\circ}, 348.5^{\circ}$

55. $154.2^{\circ}, 205.8^{\circ}$

57.

a

$\theta$	$81^{\circ}$	$82^{\circ}$	$83^{\circ}$	$84^{\circ}$	$85^{\circ}$	$86^{\circ}$	$87^{\circ}$	$88^{\circ}$	$89^{\circ}$
$\tan \theta$	6.314	7.115	8.114	9.514	11.43	14.301	19.081	28.636	57.29

b $\tan \theta$ approaches $\infty$

c

$\theta$	$99^{\circ}$	$98^{\circ}$	$97^{\circ}$	$96^{\circ}$	$95^{\circ}$	$94^{\circ}$	$93^{\circ}$	$92^{\circ}$	$91^{\circ}$
$\tan \theta$	-6.314	-7.115	-8.144	-9.514	-11.43	-14.301	-19.081	-28.636	-57.29

d $\tan \theta$ approaches $-\infty$

e The calculator gives an error message because $\tan 90^{\circ}$ is undefined.

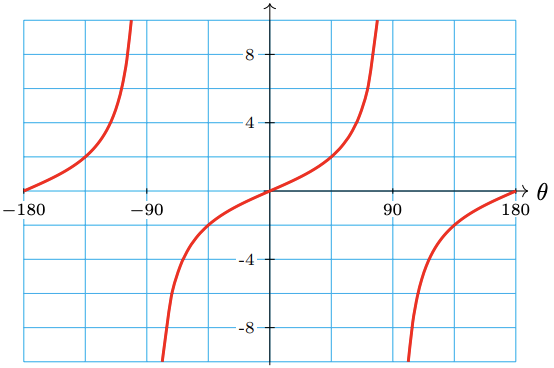
59.

61. $51.34^{\circ}$

63. $159.44^{\circ}$

65. $y+5=\left(\tan 28^{\circ}\right)(x-3)$ or $y+5=0.532(x-3)$

67. $y-12=\left(\tan 112^{\circ}\right)(x+8)$ or $y-12=-2.475(x+8)$

69.

$\alpha$	$0^{\circ}$	$15^{\circ}$	$30^{\circ}$	$45^{\circ}$	$60^{\circ}$	$75^{\circ}$	$90^{\circ}$	$105^{\circ}$	$120^{\circ}$	$135^{\circ}$	$150^{\circ}$	$165^{\circ}$	$180^{\circ}$
$m$	0	0.268	0.577	1	1.732	3.732	--	-3.732	-1.732	-1	-0.577	-0.268	0

a The slope increases toward $\infty$.

b The slope decreases toward $-\infty$.

4.3 Periodic Functions

Homework 4.3

1.

a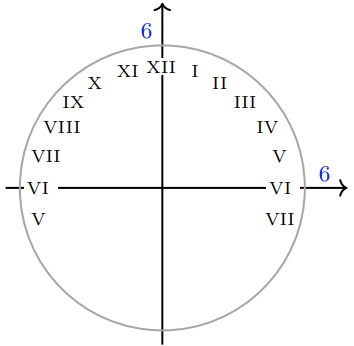

b

$t$	0	2	4	6	8	10	12	14	16	18	20	22	24
$\theta$	$90^{\circ}$	$60^{\circ}$	$30^{\circ}$	$0^{\circ}$	$330^{\circ}$	$300^{\circ}$	$270^{\circ}$	$240^{\circ}$	$210^{\circ}$	$180^{\circ}$	$150^{\circ}$	$120^{\circ}$	$90^{\circ}$
$y = f(t)$	6	$3 \sqrt{3}$	3	0	-3	$-3\sqrt{3}$	-6	$-3\sqrt{3}$	-3	0	3	$3\sqrt{3}$	6

c

d The graph from $t=24$ to $t=48$ will be exactly the same shape as the graph from $t=0$ to $t=24$. $f(t+24) = f(t)$ says that the ant's $y$-coordinate 24 seconds after a time $t$ is the same as its $y$-coordinate at time $t$.

3.

a 2, 5, 5

b 5

c

$d$	0	2	5	8	10	12	15	18	20	22	25	28	30	32	35	38	40
$y$	0	2	5	5	5	5	5	2	0	-2	-5	-5	-5	-5	-5	-2	0

d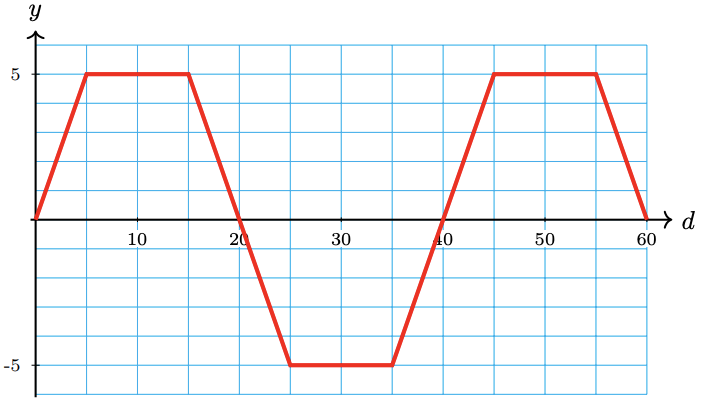

5.

a He will be back in the same position.

b $f(d+40) = f(d)$

c The graph for $0 \leq d \leq 40$ will be exactly the same shape as the graph for $40 \leq d \leq 80$.

d Every 40 unit wide piece of the graph will be identical to the previous 40 units.

7. $y = 6 \sin \theta$

9. $y = \cos \theta - 5$

11. $y = \sin (4\theta)$

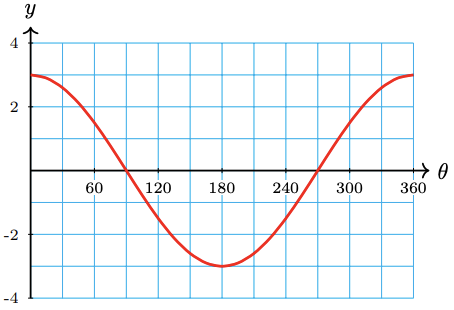
13.

15.

17.

19. amp = 4, period = $360^{\circ}$, midline: $y = 3$

21. amp = 5, period = $180^{\circ}$, midline: $y = 0$

23. amp = 3, period = $120^{\circ}$, midline: $y = -4$

25.

a amp = 1, period = $90^{\circ}$, midline: $y = 0$

b $y = \sin 4 \theta$

27.

a amp = 1, period = $360^{\circ}$, midline: $y = 3$

b $y = 3 + \cos \theta$

29.

a amp = 4, period = $360^{\circ}$, midline: $y = -2$

b $y = -2 + 4\sin \theta$

31.

a amp = 2, period = $120^{\circ}$, midline: $y = 2$

b $y = 2 + 2\cos 3 \theta$

33. $y = 2 + 5 \cos \theta$

35. $y = -4 \sin \theta$

37. $y = -4 + 6\sin 3 \theta$ (Answers vary)

39. $y = 3 + 2 \cos \theta$ (Answers vary)

41. $y = 12 \cos 2 \theta$

43. $A\left(0^{\circ},-3\right), B\left(135^{\circ}, \dfrac{3}{\sqrt{2}}\right), C\left(300^{\circ}, \dfrac{-3}{2}\right)$

45. $P\left(112.5^{\circ}, 1\right), Q\left(180^{\circ}, 0\right), R\left(337.5^{\circ},-1\right)$

47. $X\left(45^{\circ},-3+\dfrac{1}{\sqrt{2}}\right), Y\left(90^{\circ},-3\right) Z\left(300^{\circ},-2\right)$

49. not periodic

51. Periodic with period 4

53.

a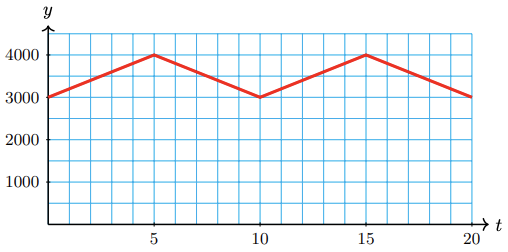

b 10 minutes

55.

a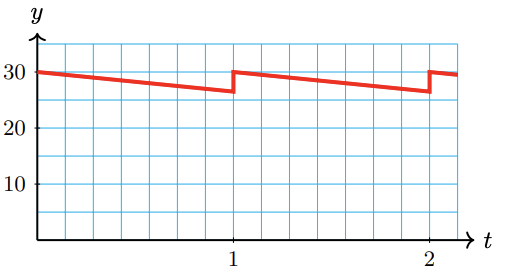

b 1 week

57.

a

b period 1 sec, midline $y = 12$, amp 10 inches

59.

a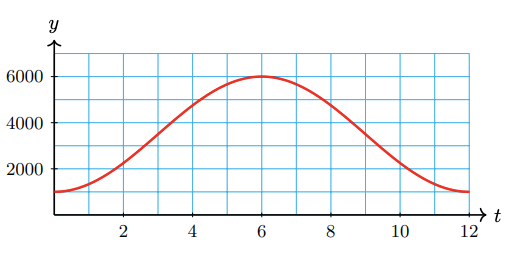

b period 1 year, midline $y = 3500$, amp 2500

61.

a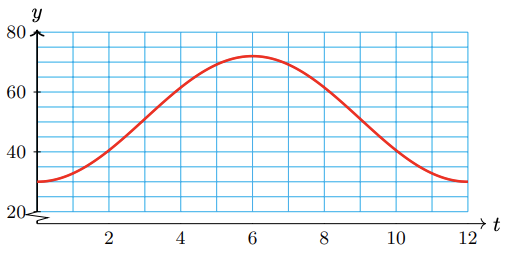

b period 1 year, midline $y = 51$, amp 21

63.

a. IV

b. III

c. II

d. I

65.

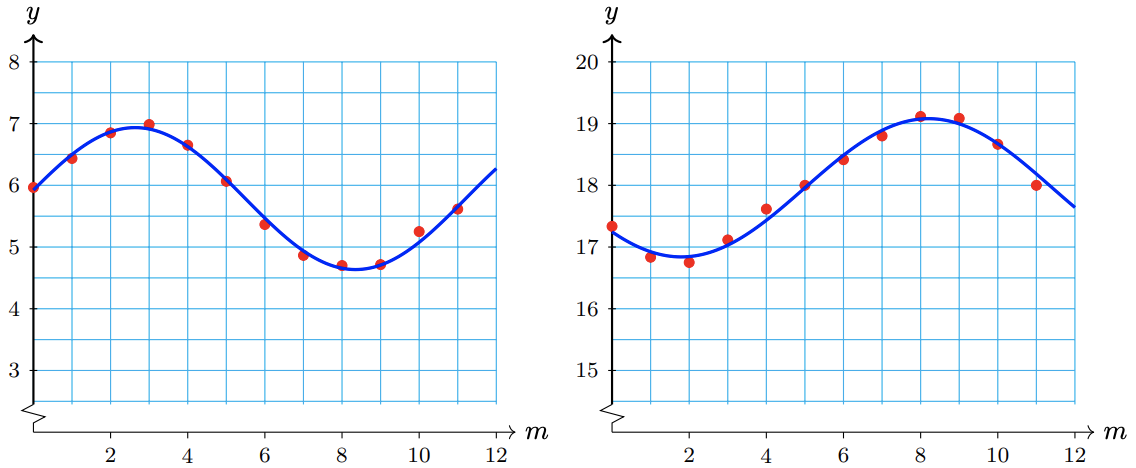
67.

a Emotional high: Oct 5 and Nov 3, low: Oct 19; Physical high: Sep 30 and Oct 23, low: Oct 12 and Nov 4; Intellectual high: Oct 10, low: Oct 26

b Emotional: 28 days, physical: 23 days, intellectual: 32 days

c 5152 days

69.

a periodic, period 8

b 4, midline: $y = 3$

c $k = 8$

d $a = 3, b = 7$

71.

a systolic 120 mm Hg, diastolic 80 mm Hg, pulse pressure 40 mm Hg.

b $99 \dfrac{1}{3}$

c 72 beats per minute

73.

a 69 hours.

b 2.2 to 3.5

c The larger dip corresponds to when the brighter star is eclipsed, the smaller dip corresponds to when the dimmer star is eclipsed.

4.4 Chapter 4 Summary and Review

Chapter 4 Review Problems

1. $12^{\circ}$

3.

a $150^{\circ}, -210^{\circ}$

b $240^{\circ},-120^{\circ}$

c $160^{\circ},-560^{\circ}$

d $20^{\circ},-340^{\circ}$

5.

a $I, 60^{\circ} ; 120^{\circ}, 240^{\circ}, 300^{\circ}$

b $I V, 25^{\circ} ; 155^{\circ}, 205^{\circ}, 335^{\circ}$

c $I I, 80^{\circ} ; 80^{\circ}, 260^{\circ}, 280^{\circ}$

d $I I I, 70^{\circ} ; 70^{\circ}, 110^{\circ}, 290^{\circ}$

7.

a

$\theta$	$30^{\circ}$	$60^{\circ}$	$90^{\circ}$	$120^{\circ}$	$150^{\circ}$	$180^{\circ}$	$210^{\circ}$	$240^{\circ}$	$270^{\circ}$	$300^{\circ}$	$330^{\circ}$	$360^{\circ}$
$f(\theta)$	30	60	90	60	30	0	30	60	90	60	30	0

b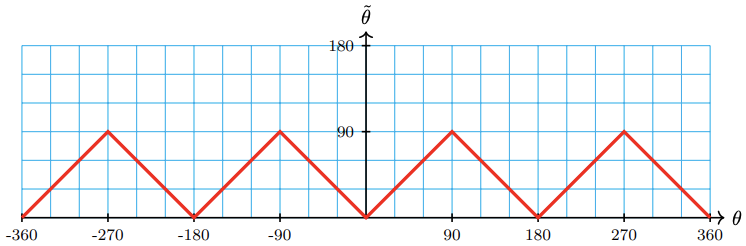

9. $210^{\circ}, 330^{\circ}$

11. $120^{\circ}, 240^{\circ}$

13. $45^{\circ}, 225^{\circ}$

15. $23^{\circ}, 337^{\circ}$

17. $72^{\circ}, 252^{\circ}$

19. $163^{\circ}, 277^{\circ}$

21. $221.81^{\circ}, 318.19^{\circ}$

23. $123.69^{\circ}, 303.69^{\circ}$

25. $128.68^{\circ}, 231.32^{\circ}$

27. (−9.74, −2.25)

29. (−0.28, 8.00)

31. (2.84, 0.98)

33. south: 1.74 mi, west: 9.85 mi

35. $y=4+7 \sin (180 \theta)$

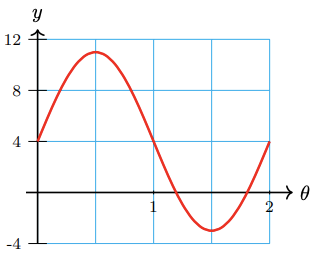
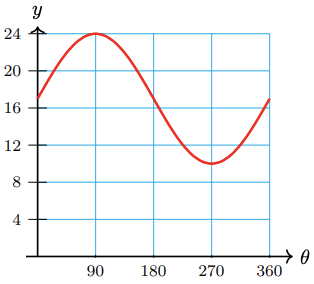
37. $y=17+7 \sin \theta$

39. $\dfrac{\sqrt{3}}{2}$

41. 0

43. $y=1.5 \cos \left(\dfrac{\theta}{3}\right), M\left(-90^{\circ}, \dfrac{3 \sqrt{3}}{4}\right), N\left(180^{\circ}, \dfrac{3}{4}\right)$

45. $y=3+3 \sin 2 \theta, A\left(-45^{\circ}, 6\right), B\left(120^{\circ}, 3-\dfrac{3 \sqrt{3}}{2}\right)$

47.

a

b 24 hours

49.

a

b 20 sec

51.

a

b amp: 2, period: $360^{\circ}$, midline: $y = 4$

53.

a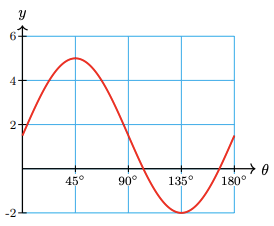

b amp: 3.5, period: $180^{\circ}$, midline: $y = 1.5$

55. $30^{\circ}$

57. $92.05^{\circ}$

59. $y = x + 2$

61. $y = - \sqrt{3}x + 3 \sqrt{3} - 4$

63.

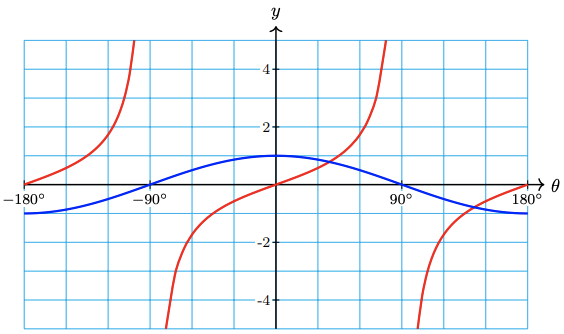
The $\theta$-intercepts of $\cos \theta$ occur at the vertical asymptotes of $\tan \theta$.