9.1: Lines and Angles
( \newcommand{\kernel}{\mathrm{null}\,}\)
You use geometric terms in everyday language, often without thinking about it. For example, any time you say “walk along this line” or “watch out, this road quickly angles to the left” you are using geometric terms to make sense of the environment around you. You use these terms flexibly, and people generally know what you are talking about. In the world of mathematics, each of these geometric terms has a specific definition. It is important to know these definitions—as well as how different figures are constructed—to become familiar with the language of geometry. Let’s start with a basic geometric figure: the plane.
A plane is a flat surface that continues forever (or, in mathematical terms, infinitely) in every direction. It has two dimensions: length and width.
You can visualize a plane by placing a piece of paper on a table. Now imagine that the piece of paper stays perfectly flat and extends as far as you can see in two directions, left-to-right and front-to-back. This gigantic piece of paper gives you a sense of what a geometric plane is like: it continues infinitely in two directions. (Unlike the piece of paper example, though, a geometric plane has no height.) A plane can contain a number of geometric figures.
The most basic geometric idea is a point, which has no dimensions. A point is simply a location on the plane. It is represented by a dot. Three points that don’t lie in a straight line will determine a plane.
The image below shows four points, labeled A, B, C, and D.
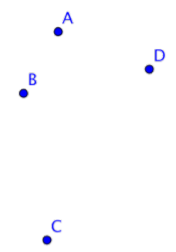
Two points on a plane determine a line. A line is a one-dimensional figure that is made up of an infinite number of individual points placed side by side. In geometry, all lines are assumed to be straight; if they bend they are called a curve. A line continues infinitely in two directions.
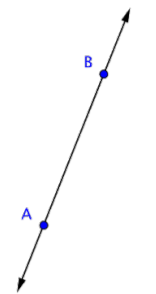
Below is an image of line AB or, in geometric notation, ↔AB. The arrows indicate that the line keeps going forever in the two directions. This line could also be called line BA. While the order of the points does not matter for a line, it is customary to name the two points in alphabetical order.
Name the line shown in red.
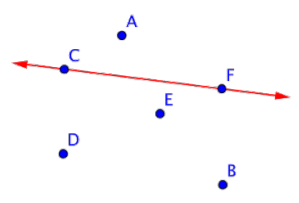
Solution
The red line goes through the points C and F, so the line is ↔CF.
The section between any two points on a line is called a line segment.
A line segment can be very long, very short, or somewhere in between. The difference between a line and a line segment is that the line segment has two endpoints and a line goes on forever. A line segment is denoted by its two endpoints, as in ¯CD in the image below.

A ray has one endpoint and goes on forever in one direction.
Mathematicians name a ray with notation like →EF, where point E is the endpoint and F is a point on the ray. When naming a ray, we always say the endpoint first. Note that →FE would have the endpoint at F, and continue through E, which is a different ray than →EF, which would have an endpoint at E, and continue through F.
The term “ray” may be familiar because it is a common word in English. “Ray” is often used when talking about light. While a ray of light resembles the geometric term “ray,” it does not go on forever, and it has some width. A geometric ray has no width; only length.
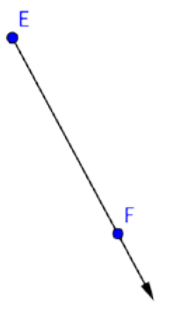
Below is an image of ray EF or →EF. Notice that the endpoint is E.
Identify each line and line segment in the picture below.

Solution
Two points define a line, and a line is denoted with arrows. There are two lines in this picture: ↔CE and ↔BG.
A line segment is a section between two points. ¯DF is a line segment. But there are also two more line segments on the lines themselves: ¯CE and ¯BG.
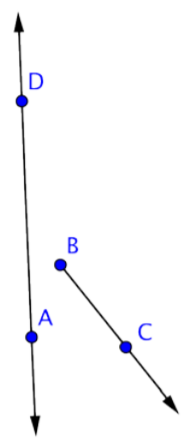
Identify each point and ray in the picture below.
Solution
There are four points: A, B, C, and D. There are also three rays, though only one may be obvious.
Ray →BC begins at point B and goes through C. Two more rays exist on line ↔AD: they are →DA and →AD.
Two rays with a common endpoint make up an angle. The common endpoint of the angle is called the vertex.
The angle ABC is shown below. This angle can also be called ∠ABC, ∠CBA or simply ∠B. When you are naming angles, be careful to include the vertex (here, point B) as the middle letter.
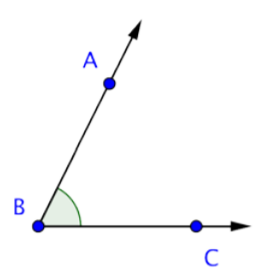
The image below shows a few angles on a plane. Notice that the label of each angle is written “point-vertex-point,” and the geometric notation is in the form ∠ABC.
Sometimes angles are very narrow; sometimes they are very wide. When people talk about the “size” of an angle, they are referring to the arc between the two rays. The length of the rays has nothing to do with the size of the angle itself. Drawings of angles will often include an arc (as shown above) to help the reader identify the correct ‘side’ of the angle.
Think about an analog clock face. The minute and hour hands are both fixed at a point in the middle of the clock. As time passes, the hands rotate around the fixed point, making larger and smaller angles as they go. The length of the hands does not impact the angle that is made by the hands.
An angle is measured in degrees, represented by the symbol º. A circle is defined as having 360º. (In skateboarding and basketball, “doing a 360” refers to jumping and doing one complete body rotation.
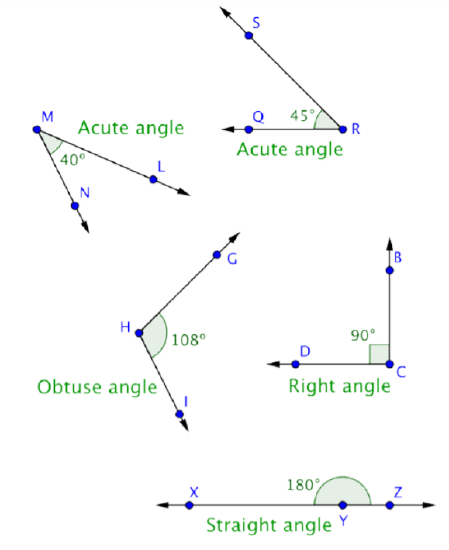
A right angle is any degree that measures exactly 90º. This represents exactly one-quarter of the way around a circle. Rectangles contain exactly four right angles. A corner mark is often used to denote a right angle, as shown in right angle DCB below.
Angles that are between 0º and 90º (smaller than right angles) are called acute angles.
Angles that are between 90º and 180º (larger than right angles and less than 180º) are called obtuse angles.
And an angle that measures exactly 180º is called a straight angle because it forms a straight line.
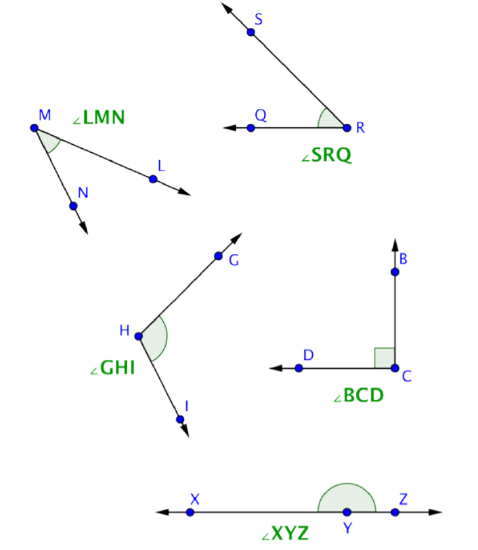
Label each angle below as acute, right, or obtuse.

Solution
You can start by identifying any right angles.
∠GFI is a right angle, as indicated by the corner mark at vertex F.
Acute angles will be smaller than ∠GFI (or less than 90º). This means that ∠DAB and ∠MLN are acute.
∠TQS is larger than ∠GFI, so it is an obtuse angle.
Identify each point, ray, and angle in the picture below.
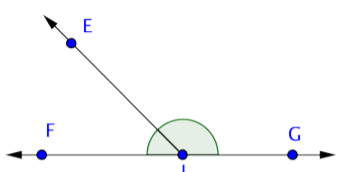
Solution
Begin by identifying each point in the figure. There are 4: E, F, G, and J.
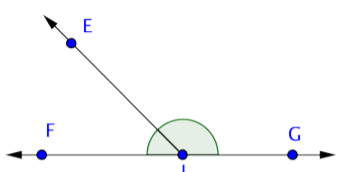
Now find rays. A ray begins at one point, and then continues through another point towards infinity (indicated by an arrow). Three rays start at point J: →JE, →JF, and →JG. But also notice that a ray could start at point F and go through J and G, and another could start at point G and go through J and F. These rays can be represented by →GF and →FG.
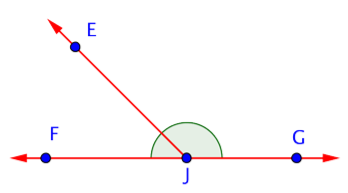
Finally, look for angles. ∠EJG is obtuse, ∠EJF is acute, and ∠FJG is straight. (Don’t forget those straight angles!)
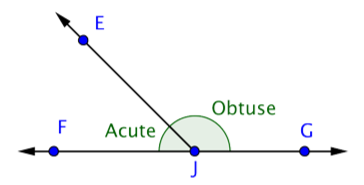
Imagine two separate and distinct lines on a plane. There are two possibilities for these lines: they will either intersect at one point, or they will never intersect. When two lines intersect, four angles are formed. Understanding how these angles relate to each other can help you figure out how to measure them, even if you only have information about the size of one angle.
Parallel lines are two or more lines that never intersect.
Perpendicular lines are two lines that intersect at a 90º (right) angle.
The image below shows some parallel and perpendicular lines. The geometric symbol for parallel is ||, so you can show that AB || CD. Parallel lines are also often indicated by the marking >> on each line (or just a single > on each line). Perpendicular lines are indicated by the symbol ⊥, so you can write ↔WX⊥↔YZ.
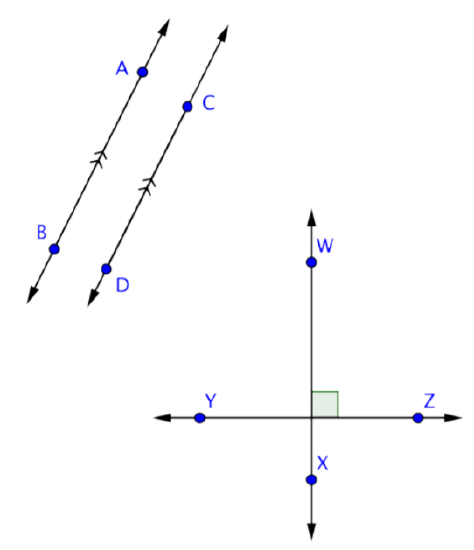
If two lines are parallel, then any line that is perpendicular to one line will also be perpendicular to the other line. Similarly, if two lines are both perpendicular to the same line, then those two lines are parallel to each other. Let’s take a look at one example and identify some of these types of lines.
Identify a set of parallel lines and a set of perpendicular lines in the image below.
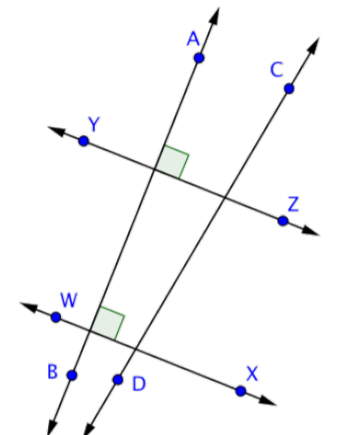
Solution
Parallel lines never meet, and perpendicular lines intersect at a right angle. ↔AB and ↔CD do not intersect in this image, but if you imagine extending both lines, they will intersect soon. So, they are neither parallel nor perpendicular.
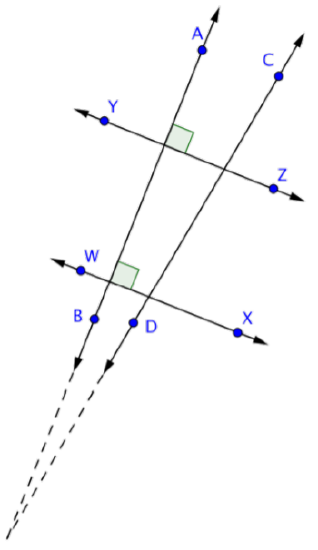
↔AB is perpendicular to both ↔WX and ↔YZ, as indicated by the right-angle marks at the intersection of those lines.
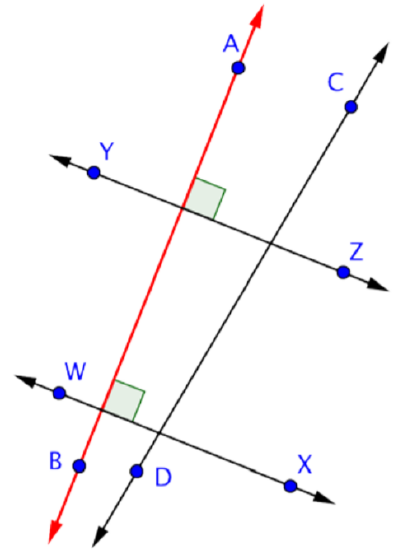
Since ↔AB is perpendicular to both lines, then ↔WX and ↔YZ are parallel.
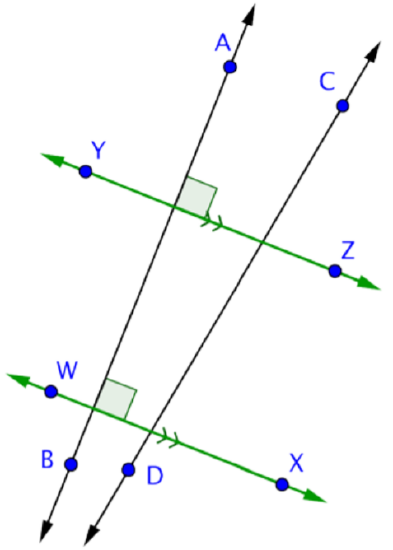
↔WX || ↔YZ
↔AB ⊥ ↔WX, ↔AB ⊥ ↔YZ
Understanding how parallel and perpendicular lines relate can help you figure out the measurements of some unknown angles. To start, all you need to remember is that perpendicular lines intersect at a 90º angle and that a straight angle measures 180º.
The measure of an angle such as ∠A is written as m∠A. Look at the example below. How can you find the measurements of the unmarked angles?
Find the measurement of ∠IJF.
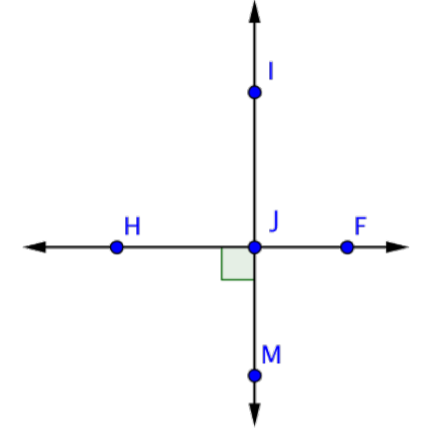
Solution
Only one angle, ∠HJM, is marked in the image. Notice that it is a right angle, so it measures 90º. ∠HJM is formed by the intersection of lines ↔IM and ↔HF. Since ↔IM is a line, ∠IJM is a straight angle measuring 180º.
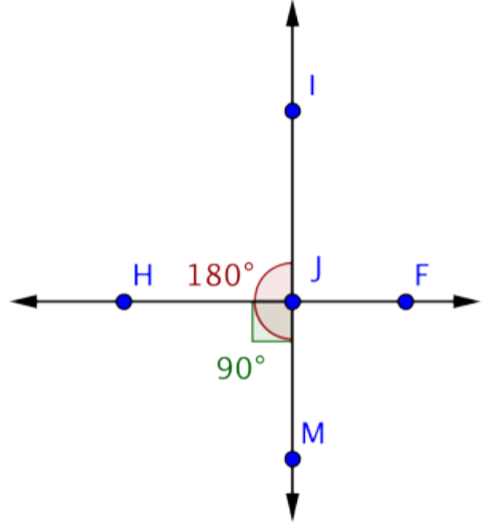
You can use this information to find the measurement of ∠HJI :
m∠HJM + m∠HJI = m∠IJM
90º + m∠HJI = 180º
m∠HJI = 90º
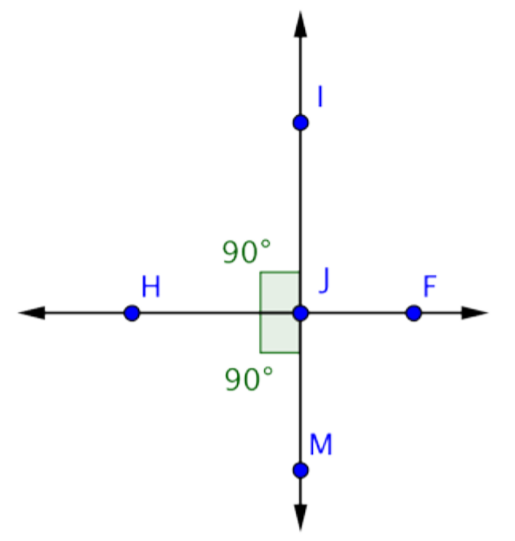
Now use the same logic to find the measurement of ∠IJF. ∠IJF is formed by the intersection of lines ↔IM and ↔HF. Since ↔HF is a line, ∠HJF will be a straight angle measuring 180º.
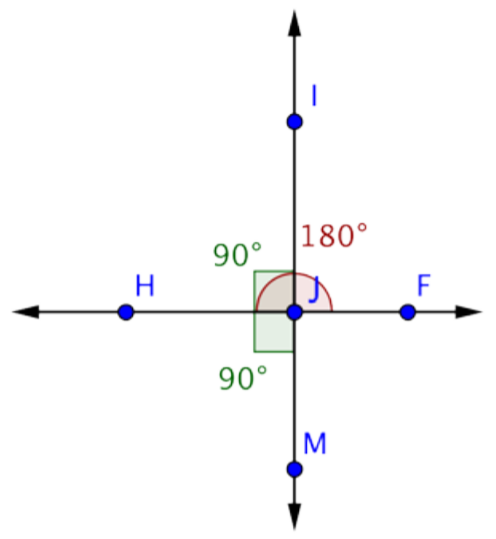
You know that ∠HJI measures 90º. Use this information to find the measurement of ∠IJF:
m∠HJM + m∠IJF = m∠HJF
90º + m∠IJF = 180º
m∠IJF = 90º
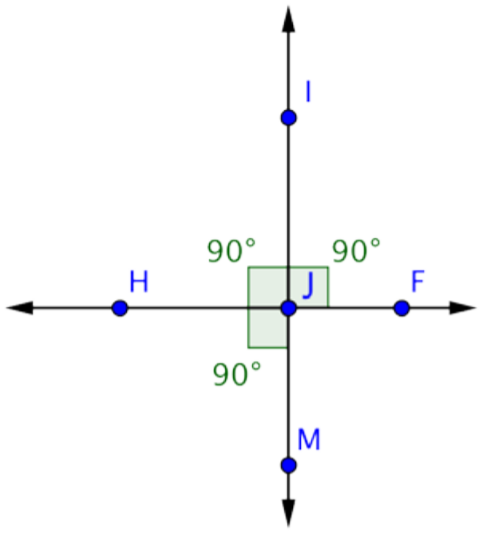
Thus, m∠IJF = 90º
In this example, you may have noticed that angles ∠HJI, ∠IJF, and ∠HJM are all right angles. (If you were asked to find the measurement of ∠FJM, you would find that angle to be 90º, too.) This is what happens when two lines are perpendicular—the four angles created by the intersection are all right angles.
Not all intersections happen at right angles, though. In the example below, notice how you can use the same technique as shown above (using straight angles) to find the measurement of a missing angle.
Find the measurement of ∠DAC.
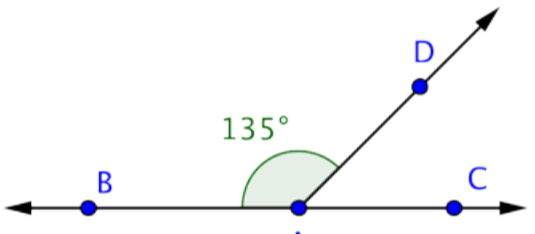
Solution
This image shows the line ↔BC and the ray →AD intersecting at point A. The measurement of ∠BAD is 135º. You can use straight angles to find the measurement of ∠DAC.
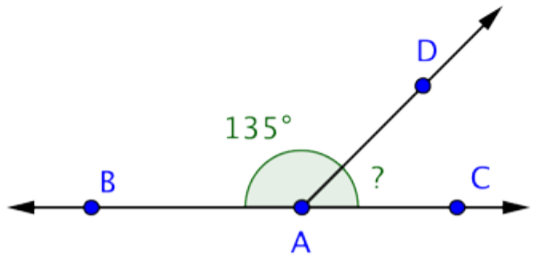
∠BAC is a straight angle, so it measures 180º.
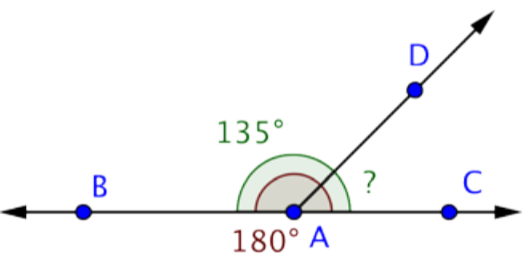
Use this information to find the measurement of ∠DAC.
m∠BAD + m∠DAC = m∠BAC
135º + m∠DAC = 180º
m∠DAC = 45º
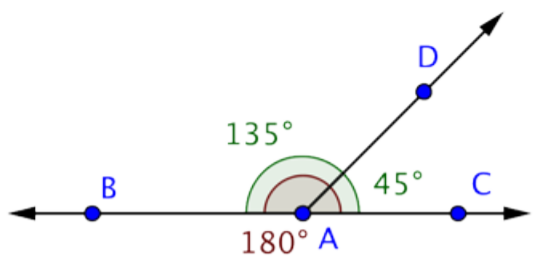
Thus, m∠DAC = 45º
Find the measurement of ∠CAD.
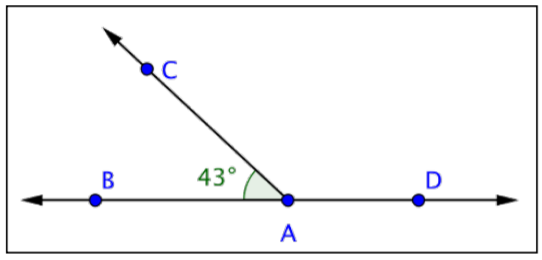
- Answer
-
m∠CAD = 137º
Two angles whose measures add up to 180º are called supplementary angles.
Two angles whose measurements add up to 90º, they are called complementary angles.
Hint: One way to remember the difference between the two terms is that “corner” and “complementary” each begin with c (a 90º angle looks like a corner), while straight and “supplementary” each begin with s (a straight angle measures 180º).
If you can identify supplementary or complementary angles within a problem, finding missing angle measurements is often simply a matter of adding or subtracting.
Two angles are supplementary. If one of the angles measures 48º, what is the measurement of the other angle?
Solution
Two supplementary angles make up a straight angle, so the measurements of the two angles will be 180º.
m∠A + m∠B = 180º
You know the measurement of one angle. To find the measurement of the second angle, subtract 48º from 180º.
48º+ m∠B = 180º
m∠B = 180º - 48º
m∠B = 132º
Find the measurement of ∠AXZ.
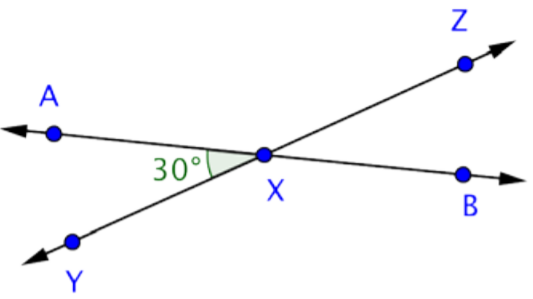
Solution
This image shows two intersecting lines, ↔AB and ↔YZ. They intersect at point X, forming four angles. Angles ∠AXY and ∠AXZ are supplementary because together they make up the straight angle ∠YXZ.
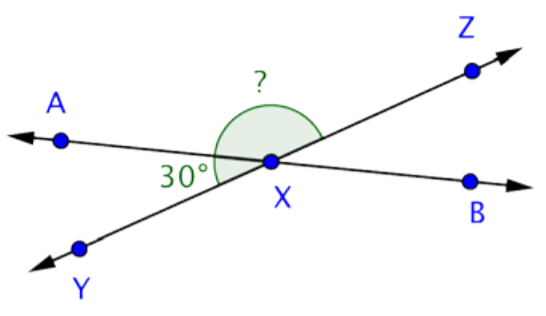
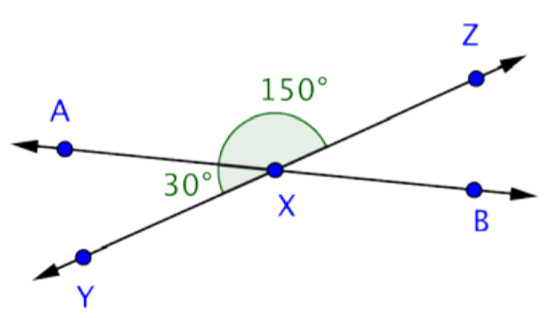
Use this information to find the measurement of ∠AXZ.
m∠AXY + m∠AXZ = m∠YXZ
30º + m∠AXZ = 180º
m∠AXZ = 150º
Find the measurement of ∠BAC.
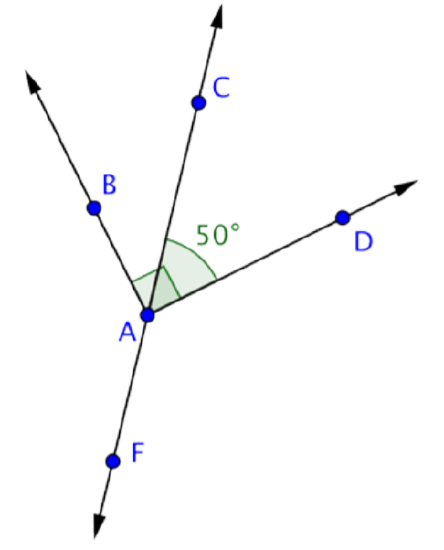
Solution
This image shows the line ↔CF and the rays ↔AB and ↔AD, all intersecting at point A. Angle ∠BAD is a right angle. Angles ∠BAC and ∠CAD are complementary because together they create ∠BAD.
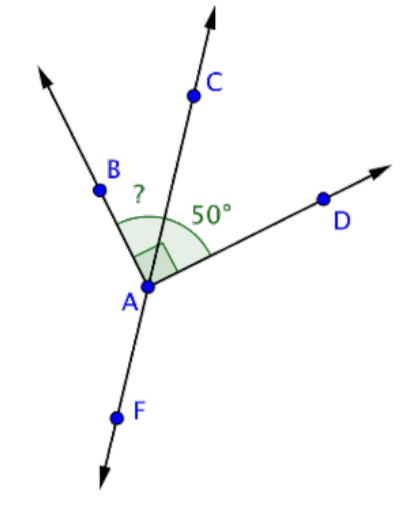
Use this information to find the measurement of ∠BAC .
m∠BAC + m∠CAD = m∠BAD
m∠BAC + 50º = 90º
m∠BAC = 40º
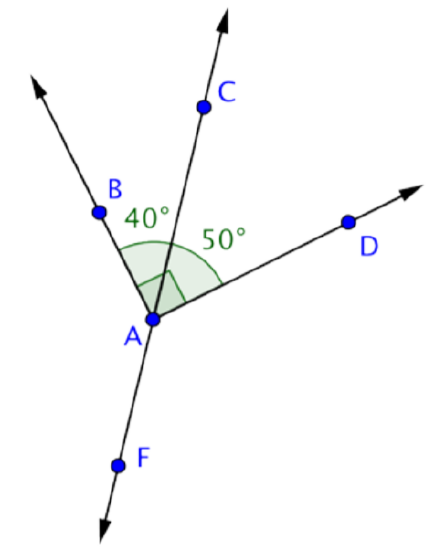
Find the measurement of ∠CAD.
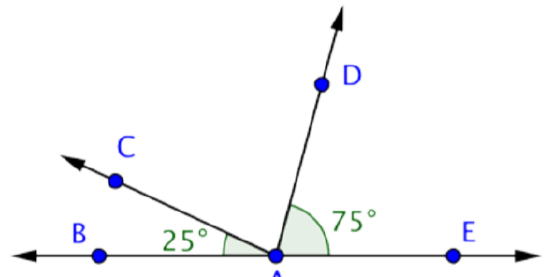
Solution
You know the measurements of two angles here: ∠CAB and ∠DAE. You also know that m∠BAE = 180º.
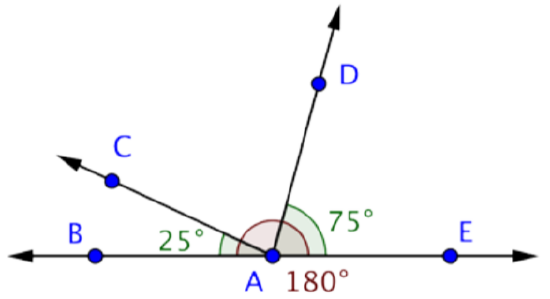
Use this information to find the measurement of ∠CAD.
m∠BAC + m∠CAD + m∠DAE = m∠BAE
25º + m∠CAD + 75º = 180º
m∠CAD + 100º = 180º
m∠CAD = 80º
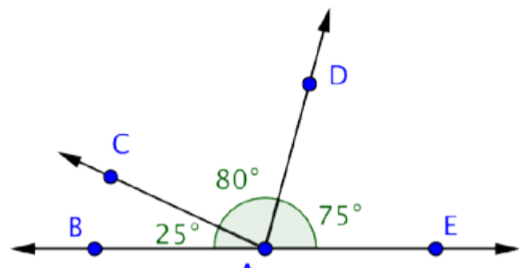
Which pair of angles is complementary?

A) ∠PKO and ∠MKN
B) ∠PKO and ∠PKM
C) ∠LKP and ∠LKN
D) ∠LKM and ∠MKN
- Answer
-
D) ∠LKM and ∠MKN


