10.5: Perpendicular circles

Assume two circles $\Gamma$ and $\Omega$ intersect at two points $X$ and $Y$. Let $\ell$ and $m$ be the tangent lines at $X$ to $\Gamma$ and $\Omega$ respectively. Analogously, $\ell'$ and $m'$ be the tangent lines at $Y$ to $\Gamma$ and $\Omega$.

From Exercise 9.6.3, we get that $\ell \perp m$ if and only if $\ell' \perp m'$.

We say that the circle $\Gamma$ is perpendicular to the circle $\Omega$ (briefly $\Gamma \perp \Omega$) if they intersect and the lines tangent to the circles at one point (and therefore, both points) of intersection are perpendicular.

Similarly, we say that the circle $\Gamma$ is perpendicular to the line $\ell$ (briefly $\Gamma \perp \ell$) if $\Gamma \cap \ell \ne \emptyset$ and $\ell$ perpendicular to the tangent lines to $\Gamma$ at one point (and therefore, both points) of intersection. According to Lemma 5.6.2, it happens only if the line l passes thru the center of $\Gamma$.

Now we can talk about perpendicular circlines.

Theorem $\PageIndex{1}$

Assume $\Gamma$ and $Omega$ are distinct circles. Then $\Omega \perp \Gamma$ if and only if the circle $\Gamma$ coincides with its inversion in $\Omega$.

Proof

Suppose that $\Gamma'$ denotes the inverse of $\Gamma$.

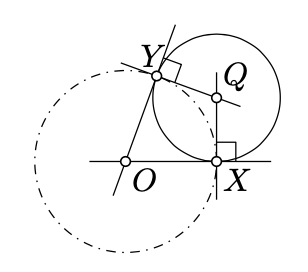
"Only if" part. Let $O$ be the center of $\Omega$ and $Q$ be the center of $\Gamma$. Let $X$ and $Y$ denote the points of intersections of $\Gamma$ and $Omega$. According to Lemma 5.6.2, $\Omega \perp \Gamma$ if and only if $(OX)$ and $(OY)$ are tangent to $\Gamma$.

Note that $\Gamma'$ is also tangent to $(OX)$ and $(OY)$ and $X$ and $Y$ respectively. It follows that $X$ and $Y$ are the foot points of the center of $\Gamma'$ on $(OX)$ and $(OY)$. Therefore, both $\Gamma'$ and $\Gamma$ have the center $Q$. Finally, $\Gamma' = \Gamma$, since both circles pass thru $X$.

"If" part. Assume $\Gamma = \Gamma'$.

Since $\Gamma \ne \Omega$, there is a point $P$ that lies on $\Gamma$, but not on $\Omega$. Let $P'$ be the inverse of $P$ in $\Omega$. Since $\Gamma = \Gamma'$, we have that $P' \in \Gamma$. In particular, the half-line $[OP)$ intersects $\Gamma$ at two points. By Exercise 5.6.1, $O$ lies outside of $\Gamma$.

As $\Gamma$ has points inside and outside of $\Omega$, the circles $\Gamma$ and $\Omega$ intersect. The latter follows from Exercise 3.5.1.

Let $X$ be point of their intersection. We need to show that $(OX)$ is tangent to $\Gamma$; that is, $X$ is the only intersection point of $(OX)$ and $\Gamma$.

Assume $Z$ is another point of intersection. Since $O$ is outside of $\Gamma$, the point $Z$ lies on the half-line $[OX)$.

Suppose that $Z'$ denotes the inverse of $Z$ in $\Omega$. Clearly, the three points $Z$, $Z'$, $X$ lie on $\Gamma$ and $(OX)$. The latter contradicts Lemma 5.6.1.

It is convenient to define the inversion in the line $\ell$ as the reflection across $\ell$. This way we can talk about inversion in an arbitrary circline.

Corollary $\PageIndex{1}$

Let $\Omega$ and $\Gamma$ be distinct circlines in the inversive plane. Then the inversion in $\Omega$ sends $\Gamma$ to itself if and only if $\Omega \perp \Gamma$.

Proof

By Thorem $\PageIndex{1}$, it is sufficient to consider the case when $\Omega$ or $\Gamma$ is a line.

Assume $\Omega$ is a line, so the inversion in $\Omega$ is a reflection. In this case the statement follows from Corollary 5.4.1.

If $\Gamma$ is a line, then the statement follows from Theorem 10.3.2.

Corollary $\PageIndex{2}$

Let $P$ and $P'$ be two distinct points such that $P'$ is the inverse of $P$ in the circle $\Omega$. Assume that the circline $\Gamma$ passes thru $P$ and $P'$. Then $\Gamma \perp \Omega$.

Proof

Without loss of generality, we may assume that $P$ is inside and $P'$ is outside $\Omega$. By Theorem 3.5.1, $\Gamma$ intersects $\Omega$. Suppose that A denotes a point of intersection.

Suppose that $\Gamma'$ denotes the inverse of $\Gamma$. Since $A$ is a self-inverse, the points $A, P$, and $P'$ lie on $\Gamma'$. By Exercise 8.1.1, $\Gamma'$ = $\Gamma$ and by Theorem $\PageIndex{1}$, $\Gamma \perp \Omega$.

Corollary $\PageIndex{3}$

Let $P$ and $Q$ be two distinct points inside the circle $\Omega$. Then there is a unique circline $\Gamma$ perpendicular to $\Omega$ that passes thru $P$ and $Q$.

Proof

Let $P'$ be the inverse of the point $P$ in the circle $\Omega$. According to Corollary $\PageIndex{2}$, the circline is passing thru $P$ and $Q$ is perpendicular to $\Omega$ if and only if it passes thru $P'$.

Note that $P'$ lies outside of $\Omega$. Therefore, the points $P$, $P'$, and $Q$ are distinct.

According to Exercise Exercise 8.1.1, there is a unique circline passing thru $P, Q$, and $P'$. Hence the result.

Exercise $\PageIndex{1}$

Let $P, Q, P'$, and $Q'$ be points in the Euclidean plane. Assume $P'$ and $Q'$ are inverses of $P$ and $Q$ respectively. Show that the quadrangle $PQP'Q'$ is inscribed.

Hint

Apply Theorem 10.2.1, Theorem 7.4.5 and Theorem 9.2.1.

Exercise $\PageIndex{2}$

Let $\Omega_1$ and $\Omega_2$ be two perpendicular circles with centers at $O_1$ and $O_2$ respectively. Show that the inverse of $O_1$ in $\Omega_2$ coincides with the inverse of $O_2$ in $\Omega_1$.

Hint

Suppose that $T$ denotes a point of intersection of $\Omega_1$ and $\Omega_2$. Let $P$ be the foot point of $T$ on $(O_1O_2)$. Show that $\triangle O_1PT \sim \triangle O_1TO_2 \sim \triangle TPO_2$. Consider that $P$ coincides with the inverses of $O_1$ in $\Omega_2$ and of $O_2$ in $\Omega_1$.

Exercise $\PageIndex{3}$

Three distinct circles — $\Omega_1$, $\Omega_2$ and $\Omega_3$, intersect at two points — $A$ and $B$. Assume that a circle $\Gamma$ is perpendicular to $\Omega_1$ and $\Omega_2$. Show that $\Gamma \perp \Omega_3$.

Hint

Since $\Gamma \perp \Omega_1$ and $\Gamma \perp \Omega_2$, Corollary $PageIndex{1}$ implies that the circles $\Omega_1$ and $\Omega_2$ are inverted in $\Gamma$ to themselves. Conclude that the points $A$ and $B$ are inverse of each other. Since $\Omega_3 \ni A, B$, Corollary $\PageIndex{2}$ implies that $\Omega_3 \perp \Gamma$.

Let us consider two new construction tools: the circumtool that constructs a circline thru three given points, and the inversion-tool — a tool that constructs an inverse of a given point in a given circline.

Exercise $\PageIndex{4}$

Given two circles $\Omega_1$, $\Omega_2$ and a point $P$ that does not lie on the circles, use only circum-tool and inversion-tool to construct a circline $\Gamma$ that passes thru $P$, and perpendicular to both $\Omega_1$ and $\Omega_2$.

Hint

Let $P_1$ and $P_2$ be the inverse of $P$ in $\Omega_1$ and $\Omega_2$. Apply Corollary $\PageIndex{2}$ and Theorem $\PageIndex{1}$ to show that a circline $\Gamma$ that pass thru

$P, P_1$, and $P_3$ is a solution.

Advanced Exercise $\PageIndex{5}$

Given three disjoint circles $\Omega_1$, $\Omega_2$ and $\Omega_3$, use only circum-tool and inversion-tool to construct a circline $\Gamma$ that perpendicular to each circle $\Omega_1$, $\Omega_2$ and $\Omega_3$.

Think what to do if two of the circles intersect.

Hint

All circles that perpendicular to $\Omega_1$ and $\Omega_2$ pass thru a fixed point $P$. Try to construct $P$.

If two of the circles intersect, try to apply Corollary 10.6.1.